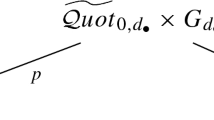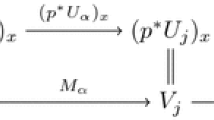Multi-Gieseker semistability and moduli of quiver sheaves

Abstract

We generalize the notion of multi-Gieseker semistability for coherent sheaves, introduced by Greb, Ross, and Toma, to quiver sheaves for a quiver Q. We construct coarse moduli spaces for semistable quiver sheaves using a functorial method that realizes these as subschemes of moduli spaces of representations of a twisted quiver, depending on Q, with relations. We also show the projectivity of the moduli space in the case when Q has no oriented cycles. Further, we construct moduli spaces of quiver sheaves which satisfy a given set of relations as closed subvarieties. Finally, we investigate the parameter dependence of the moduli.

Appendices

Appendices

Quivers and their representations

In this section, we outline how the notions of representations and stability conditions (as introduced in King (1994) for unlabeled quivers) can be generalized to the case of labeled quivers with relations, i.e. ordinary quivers with labeling vector spaces assigned to the arrows. This is a mostly straightforward procedure, but even though special cases have been studied (e.g. in Álvarez-Cónsul and King (2007) or Greb et al. (2016)), there seems to be no such concise treatment in the literature.

Apart from the foundational paper King (1994), we also refer the reader to the survey article Reineke (2008) for an introduction to the case of an unlabeled quiver.

We recall that an (unlabeled) quiver $Q=(Q_0,Q_1)$ consists of a set of vertices $Q_0$ and a set of arrows $\alpha :i\rightarrow j$. We assume both sets to be finite.

Definition A.1

A labeling for a quiver Q is a collection of vector spaces

$$\begin{aligned} H=\left( H_\alpha \mid \alpha \in Q_1\right) \end{aligned}$$

of finite dimension for each arrow in Q. An arrow $\alpha$ with label $H_\alpha =k$ is considered to be unlabeled. The pair (Q, H) is called a labeled quiver.

1.1 The category of representations

A representation M of an (unlabeled) quiver Q in some category ${\mathcal {C}}$ consists of a tuple of objects $\left( M_i\mid i\in Q_0\right)$ for each vertex, and a tuple of morphisms

$$\begin{aligned} \left( M_\alpha :M_i\rightarrow M_j \mid (\alpha :i\rightarrow j)\in Q_1\right) \end{aligned}$$

for each arrow. Together with the appropriate notion of morphisms, these representations form a category.

Definition A.2

The category of representations of Q in the category C is denoted as $Q-{\mathrm {rep}}_{\mathcal {C}}.$

The special cases of representations in the category $k-{\mathrm {vect}}$ of vector spaces of finite dimension, and in the category of coherent sheaves ${\mathrm {Coh}}(X)$ on some scheme X over k deserve the special notations

$$\begin{aligned} Q-{\mathrm {rep}}=Q-{\mathrm {rep}}_{k-{\mathrm {vect}}},\quad Q-{\mathrm {Coh}}(X)=Q-{\mathrm {rep}}_{{\mathrm {Coh}}(X)}. \end{aligned}$$

Objects in $Q-{\mathrm {Coh}}(X)$ are called quiver sheaves.

A representation of a labeled quiver is given in a slightly different manner.

Definition A.3

A representation M of a labeled quiver (Q, H) consists of a tuple $\left( M_i \mid i\in Q_0\right)$ of finite-dimensional vector spaces, and a tuple

$$\begin{aligned} \left( M_\alpha : M_i\otimes _k H_\alpha \rightarrow M_j \mid (\alpha :i\rightarrow j)\in Q_1\right) \end{aligned}$$

of linear maps. A morphism of representations $\varphi : M\rightarrow N$ consists of a tuple of linear maps $\left( \varphi _i:M_i\rightarrow N_i\mid i\in Q_0\right)$, such that for all arrows $\alpha :i\rightarrow j$ in Q the diagram

commutes.

Again, the representations form a category.

Definition A.4

The category of representations of (Q, H) is denoted as $(Q,H)-{\mathrm {rep}}$.

We also need the dimension vector of a representation.

Definition A.5

The dimension vector of a vector space representation M is defined as

$$\begin{aligned} \underline{\dim }(M)=\left( \dim (M_i)\mid i\in Q_0\right) , \end{aligned}$$

both for the labeled and unlabeled case.

In the unlabeled case, relations on the quiver are a well-established concept. To the best knowledge of the authors, there is no previous treatment of relations in the case of a labeled quiver in the literature.

For the construction of the moduli space, we need such relations. More precisely, we restrict ourselves to the discussion of one very special case involving non-trivial labels, and relations only involving unlabeled arrows.

Convention A.6

Suppose that (Q, H) contains a subquiver

that is two unlabeled arrows $\beta ,\gamma$ and two labeled arrows $\alpha ,\delta$, with the same label H on opposite sides of a square. We then say that a representation M satisfies the relation $\gamma \alpha -\delta \beta$ if there is a commuting diagram

Another special case we need is that of relations which only involve unlabeled arrows. They can be treated in exactly the same way as in the well-known case of relations on an unlabeled quiver.

We remark that relations in the unlabeled case also make sense for arbitrary k-linear categories. In particular, we can consider quiver sheaves satisfying a set of relations I.

Definition A.7

The full subcategory of quiver sheaves satisfying the relations I is denoted as

$$\begin{aligned} (Q,I)-{\mathrm {Coh}}(X)\subset Q-{\mathrm {Coh}}(X). \end{aligned}$$

A labeled quiver with relations is now defined as a triple (Q, H, I), where (Q, H) is a labeled quiver and I is a set of relations of a form as discussed above.

Definition A.8

The category

$$\begin{aligned} (Q,H,I)-{\mathrm {rep}}\subset (Q,H)-{\mathrm {rep}}\end{aligned}$$

is the full subcategory of representations which satisfy the relations I.

The connection of the notion of labeled representations to the unlabeled ones is via a choice of basis for each label $H_\alpha$. This also allows us to inherit many already established results.

Specifically, let (Q, H, I) denote any labeled quiver with relations. Construct a new (unlabeled) quiver $Q'$ by setting

$$\begin{aligned} Q_0'=Q_0,~Q_1'=\left\{ \alpha _k:i\rightarrow j \mid (\alpha :i\rightarrow j)\in Q_1,~k=1,\ldots ,\dim (H_\alpha )\right\} . \end{aligned}$$

Roughly speaking, we replace each arrow by $\dim (H_\alpha )$ copies. For a relation $\gamma \alpha -\delta \beta$ in a form as explained above, we equip $Q'$ with $\dim (H)$ relations of the form

$$\begin{aligned} \gamma \alpha _k-\delta _k\beta ,\quad k=1,\ldots ,\dim (H). \end{aligned}$$

Relations only involving unlabeled arrows can be imposed on $Q'$ in a straight-forward way. The set of all such relations is denoted as $I'$.

Now, choose a basis for each label $H_\alpha$. We restrict this choice by assuming the following.

• (1) If the label is trivial, i.e. $H_\alpha =k$, the canonical basis $1\in k$ is chosen.

• (2) If two labels are exactly the same, i.e. $H_\alpha =H_\beta$, the same bases are chosen.

It is straightforward to check that these two quivers essentially have the same categories of representations.

Proposition A.9

The choice of bases induces an isomorphism of categories

$$\begin{aligned} (Q,H,I)-{\mathrm {rep}}\rightarrow (Q',I')-{\mathrm {rep}}. \end{aligned}$$

This identification respects dimension vectors.

The paths in a labeled quiver (Q, H) are simply paths in the underlying quiver Q, and the label of a path $\gamma =\alpha _1\alpha _2\ldots \alpha _l$ is defined as

$$\begin{aligned} H_\gamma =H_{\alpha _l}\otimes _k\ldots \otimes _k H_{\alpha _1}. \end{aligned}$$

By convention, the paths $e_i$ of length zero should be labeled by k.

Definition A.10

The path algebra of (Q, H) is

$$\begin{aligned} A=A(Q,H)=\bigoplus _{\gamma \mathrm {~path}}k\cdot \gamma \otimes _k H_\gamma \end{aligned}$$

as a vector space, and multiplication is given on homogeneous elements by

$$\begin{aligned} \left( \gamma \otimes h\right) \cdot \left( \gamma '\otimes h'\right) =\gamma \gamma '\otimes (h'\otimes h) \end{aligned}$$

if the concatenation $\gamma \gamma '$ is possible, and zero otherwise. Relations of the form $\delta \beta -\gamma \alpha$ in (Q, H) give rise to the relations

$$\begin{aligned} \left( \delta \otimes h_\delta \right) \cdot \left( \beta \otimes h_\beta \right) -\left( \gamma \otimes h_\gamma \right) \cdot \left( \alpha \otimes h_\alpha \right) =0 \end{aligned}$$

independent of the elements $h_\alpha ,h_\delta \in H$ and $h_\beta ,h_\gamma \in k$. The path algebra with relations is then simply the path algebra of (Q, H) modulo the ideal generated by these relations.

We remark that there is an isomorphism

$$\begin{aligned} A\simeq A' \end{aligned}$$

between the path algebra A of (Q, H, I) and the path algebra $A'$ of $(Q',I')$.

1.2 Stability conditions

A stability condition on a quiver, labeled or unlabeled, is a tuple $\theta \in {\mathbb {R}}^{Q_0}.$

Definition A.11

The slope of a nonzero representation with respect to $\theta$ is given as

$$\begin{aligned} \mu (M)=\frac{\sum _{i\in Q_0}\theta _i \dim (M_i)}{\sum _{i\in Q_0}\dim (M_i)}, \end{aligned}$$

and M is said to be $\theta$-semistable if and only if

$$\begin{aligned} \mu (N)\le \mu (M) \end{aligned}$$

holds for all non-trivial subrepresentations $N\subset M$. If strict inequality holds for all subrepresentations we say that M is stable. Consequently, for some representation M we say that $N\subset M$ is destabilizing if $\mu (N)\ge \mu (M)$.

Just as in the unlabeled case, we can show the existence of Jordan–Hölder filtrations for semistable representations, and thus we obtain the notion of S-equivalence.

Consider the identification of Proposition A.9. Since Q and $Q'$ have the same set of vertices, a stability condition $\theta$ can be used on both simultaneously. Subrepresentations and dimension vectors are preserved, so that stability is preserved as well.

Corollary A.12

The identification

$$\begin{aligned} (Q,H,I)-{\mathrm {rep}}\simeq (Q',I')-{\mathrm {rep}}\end{aligned}$$

preserves stability, semistability and S-equivalence.

1.3 Moduli spaces

We briefly sketch the construction of moduli spaces of semistable representations. This is a slight variation of the program carried out by King (1994) for the case of unlabeled quivers.

Let (Q, H, I) denote a labeled quiver with relations.

Definition A.13

The representation variety for dimension vector $d\in {\mathbb {N}}^{Q_0}$ is given as

$$\begin{aligned} R_d(Q,H)=\bigoplus _{\alpha :i\rightarrow j}{\mathrm {Hom}}(k^{d_i}\otimes H_\alpha ,k^{d_j}), \end{aligned}$$

and the relations I define a closed subvariety $R_d(Q,H,I)\subset R_d(Q,H)$.

On $R_d(Q,H)$ we have an action of the group

$$\begin{aligned} G_d=\prod _{i\in Q_0}{\mathrm {GL}}(d_i,k) \end{aligned}$$

via conjugation, that is

$$\begin{aligned} \left( g_i\mid i\in Q_0\right) *\left( f_\alpha \mid \alpha :i\rightarrow j\right) =(g_j^{-1}f_\alpha \left( g_i\otimes id\right) \mid \alpha :i\rightarrow j). \end{aligned}$$

Note that the diagonally embedded scalars ${\mathbb {G}_m}\subset G_d$ act trivially, so that we can equivalently pass to the action of the group

$$\begin{aligned} PG_d=G_d/{\mathbb {G}_m}. \end{aligned}$$

Furthermore, there is a tautological bundle ${\mathbb {M}}$ of A-modules on $R_d(Q,H,I)$, where A denotes the path algebra of (Q, H, I). As a vector bundle,

$$\begin{aligned} {\mathbb {M}}=\left( \bigoplus _{i\in Q_0}k^{d_i}\right) \times R_d(Q,H,I)\rightarrow R_d(Q,H,I) \end{aligned}$$

is trivial. Each fiber ${\mathbb {M}}_x$ carries the structure of an A-module, by declaring that each arrow acts via the morphisms encoded in x.

The identification of representations of (Q, H, I) with those of an unlabeled quiver via choice of bases implies that the GIT-construction of the moduli space transfers to the labeled case. Here, we use the abbreviations ${\mathrm {st}}=\theta -{\mathrm {st}}$ and ${\mathrm {sst}}=\theta -{\mathrm {sst}}$.

Theorem A.14

There is a commuting diagram

and the following assertions hold.

• (1) The vertical arrows are good quotients, and the leftmost quotient is even geometric.

• (2) The inclusions are open, and p is a projective morphism.

• (3) The varieties $M^{\mathrm {st}}_d(Q,H,I), M^{\mathrm {sst}}_d(Q,H,I)$ and $M^{\mathrm {ssp}}_d(Q,H,I)$ are the moduli spaces of stable, semistable and semisimple representations of (Q, H, I) of dimension vector d, respectively.

• (4) The variety $M^{\mathrm {ssp}}_d(Q,H,I)$ is affine. It is trivial if and only if Q does not contain oriented cycles.

• (5) Closed points in $M_d^{\mathrm {sst}}(Q,H,I)$ correspond to S-equivalence classes of semistable representations, and closed points in $M^{\mathrm {st}}_d(Q,H,I)$ correspond to isomorphism classes of stable representations.

Proof

The special case of an unlabeled quiver without relations is settled by King (1994), and the general theory of GIT (see eg. Reineke (2008), Section 3.5), and the question when $M^{\mathrm {ssp}}_d(Q)$ is affine is settled by Bruyn (1990).

Relations in the unlabeled case can be handled, once we note that S-equivalence respects relations. This is because orbit closure respects S-equivalence and

$$\begin{aligned} R_d(Q,I)^{\mathrm {sst}}=R_d(Q,I)\cap R_d(Q)^{\mathrm {sst}}. \end{aligned}$$

Finally, our identifications allow a transfer to the labeled case. Note that $Q'$ contains oriented cycles if and only if Q does. $\square$

Denote by A the path algebra of (Q, H, I), by R the representation variety $R_d(Q,H,I)$, and by G the group $G_d$. Consider the functor

$$\begin{aligned} {\mathcal {M}}_A:(Sch/k)^{\mathrm {op}}\rightarrow Sets, \end{aligned}$$

which maps a scheme S to the set of isomorphism classes of $A\otimes {\mathcal {O}}_S$-modules which are locally free as ${\mathcal {O}}_S$-modules.

By sending a morphism $f:S\rightarrow R$ to the pullback $f^*{\mathbb {M}}$ of the tautological family, we obtain a natural transformation

$$\begin{aligned} h:\underline{R}\rightarrow {\mathcal {M}}_A. \end{aligned}$$

Just like in Álvarez-Cónsul and King (2007), Proposition 4.4, we can prove that ${\mathcal {M}}_A$ is locally isomorphic to a quotient functor.

Proposition A.15

The natural transformation h induces a local isomorphism

$$\begin{aligned} h':\underline{R}/\underline{G}\rightarrow {\mathcal {M}}_A. \end{aligned}$$

Quiver quot-schemes

We construct the quiver sheaf version of the Quot-scheme, which is helpful as a technical tool throughout this chapter. This section is mostly independent of the other sections.

First, we need a version of the Quot-scheme for sheaves which uses the topological type instead of the Hilbert polynomials.

Recall the strategy of proof in the construction of the sheaf version of the Quot-scheme ${\mathrm {Quot}}^P_{E/X/S}$, parametrizing flat quotients of the sheaf E with Hilbert polynomial P (eg. consider Huybrechts and Lehn (2010), Theorem 2.2.4). Reducing to the case

$$\begin{aligned} X={\mathbb {P}}^n\rightarrow S={\mathrm {Spec}}(k), \end{aligned}$$

one constructs an embedding of functors

$$\begin{aligned} {\mathcal {Q}\mathrm {uot}}^P_{E/X/S}\rightarrow {\mathcal {G}\mathrm {rass}}_k (H^0\left( E(m)\right) ,P), \end{aligned}$$

where the right hand side is represented by the Grassmannian scheme. The Quot-scheme is then given as the component corresponding to P of the flattening stratification of some suitable sheaf F on the Grassmannian.

This proof carries over to the case of the Quot-scheme ${\mathrm {Quot}}^\tau _{E/X/S}$, parameterizing flat quotients of E with topological type $\tau \in B(X)_{\mathbb {Q}}$. More precisely, the same construction as above gives an embedding

$$\begin{aligned} {\mathcal {Q}\mathrm {uot}}^\tau _{E/X/S}\rightarrow {\mathcal {G}\mathrm {rass}}_k (H^0\left( E(m)\right) ,P), \end{aligned}$$

where $P=P(\tau )$ is the Hilbert polynomial determined by $\tau$ (and the implicitly fixed relatively very ample line bundle). Since $\tau$ is locally constant in flat families, we may consider the component of the flattening stratification corresponding to $\tau$. The same argument as above shows that this component represents the Quot-functor.

We will mainly need the generalization to the Quiver Quot-scheme in the version using the topological type. Though all arguments hold for the version using Hilbert polynomials equally well.

Definition B.1

Consider a projective scheme $X\rightarrow S$ over a noetherian base scheme S, a topological type $\tau \in B(X)_{\mathbb {Q}}^{Q_0}$ and a quiver sheaf ${\mathcal {E}}$ on X. The Quiver Quot-functor

$$\begin{aligned} {\mathcal {Q}\mathrm {uot}}^\tau _{{\mathcal {E}}/X/S}:(Sch/S)^{\mathrm {op}}\rightarrow Sets \end{aligned}$$

assigns to a scheme $T\rightarrow S$ the set of equivalence classes of quotient quiver sheaves $q:{\mathcal {E}}_T\rightarrow {\mathcal {F}}$ on $X_T$ where ${\mathcal {F}}$ is flat over T and the topological type of the fiberwise quiver sheaves $({\mathcal {E}})_t$ are equal to $\tau$.

Definition B.2

Consider a projective scheme $X\rightarrow S$ over a noetherian base scheme S, a tuple of polynomials $P \in {\mathbb {Q}}[T]^{Q_0}$ and a quiver sheaf ${\mathcal {E}}$ on X. Also, implicitly fix a relatively very ample line bundle on X. The Quiver Quot-functor

$$\begin{aligned} {\mathcal {Q}\mathrm {uot}}^P_{{\mathcal {E}}/X/S}:(Sch/S)^{\mathrm {op}}\rightarrow Sets \end{aligned}$$

assigns to a scheme $T\rightarrow S$ the set of equivalence classes of quotient quiver sheaves $q:{\mathcal {E}}_T\rightarrow {\mathcal {F}}$ on $X_T$ where ${\mathcal {F}}$ is flat over T and the Hilbert polynomials of the fibrewise sheaves at the vertices $({\mathcal {E}}_i)_t$ are equal to $P_i$.

Proposition B.3

Let $X\rightarrow S$ denote a projective scheme over a noetherian base scheme S, and let E and F denote coherent sheaves on X such that F is flat over S. Consider a morphism $f:E\rightarrow F$ and the functor

$$\begin{aligned} F:(Sch/S)^{\mathrm {op}}\rightarrow Sets,~ \left( T\rightarrow S\right) \mapsto \left\{ \phi \in {\mathrm {Hom}}_S(T,X) \mid {\phi }^*(f)=0\right\} . \end{aligned}$$

This functor is represented by a closed subscheme of X.

Proof

Consider the functor

$$\begin{aligned} F':(Sch/S)^{\mathrm {op}}\rightarrow Sets,~\left( T\rightarrow S\right) \mapsto {\mathrm {Hom}}_{X_T}(E_T,F_T) \end{aligned}$$

as in Nitsure (2005), Theorem 5.8. It is represented by a scheme $V\!=\!{\mathrm {Spec}}\left( {\mathrm {Sym}}_{{\mathcal {O}}_S}(Q)\right)$ for a suitable sheaf Q on S.

In our situation, $f\in F'(X)$ corresponds to a morphism $\phi _f:X\rightarrow V$, so that Remark 5.9 in Nitsure (2005) implies that our functor F is represented by the closed subscheme $\phi _f^{-1}(V_0)$, where $V_0\subset V$ is the image of the zero section $S\rightarrow V$. $\square$

Theorem B.4

The Quiver Quot-functor is represented by a projective scheme ${\mathrm {Quot}}^\tau _{{\mathcal {E}}/X/S}$, the so called Quiver Quot-scheme. It is given as the closed subscheme

$$\begin{aligned} {\mathrm {Quot}}^\tau _{{\mathcal {E}}/X/S}\subset \prod _{i\in Q_0} {\mathrm {Quot}}^{\tau _i}_{{\mathcal {E}}_i/X/S} \end{aligned}$$

which satisfies the conditions to be compatible with the morphisms ${\mathcal {E}}_\alpha$.

A similar statement holds for the version using Hilbert polynomials.

Proof

By projection to the quotient sheaves at the vertices we get a natural transformation of functors

$$\begin{aligned} {\mathcal {Q}\mathrm {uot}}^\tau _{{\mathcal {E}}/X/S}\rightarrow \prod _{i\in Q_0} {\mathcal {Q}\mathrm {uot}}^{\tau _i}_{{\mathcal {E}}_i/X/S}, \end{aligned}$$

where the right hand side is clearly represented by the projective scheme

$$\begin{aligned} G=\prod _{i\in Q_0}G_i=\prod _{i\in Q_0} {\mathrm {Quot}}^{\tau _i}_{{\mathcal {E}}_i/X/S}. \end{aligned}$$

Consider the universal quotients at the vertices

$$\begin{aligned} q_i:({\mathcal {E}}_i)_{G_i}\rightarrow {\mathcal {U}}_i \end{aligned}$$

on the Quot-schemes $G_i$, and denote by $e_i:{\mathcal {K}}_i\rightarrow ({\mathcal {E}}_i)_{G_i}$ their kernels.

For a morphism

$$\begin{aligned} \phi :T\rightarrow G \end{aligned}$$

of noetherian schemes over S and any arrow $\alpha :i\rightarrow j$ we obtain the following exact diagram via pullback.

Hence the morphism $({\mathcal {E}}_\alpha )_T$ descends to the quotients if and only if

$$\begin{aligned} 0=\Phi ^*q_j({\mathcal {E}}_\alpha )_T\Phi ^* e_i=\Phi ^*(q_j({\mathcal {E}}_\alpha )_G e_i), \end{aligned}$$

which shows that the Quiver Quot-functor is given as the subfunctor of ${\mathrm {Hom}}_S(*,G)$ which satisfies finitely many pullback equations. It is thus represented by the intersection of the finitely many closed subschemes provided by Proposition B.3. $\square$

Langton’s theorem

This rather technical section is devoted to proving a version of Langton’s theorem for multi-Gieseker semistable quiver sheaves. Suppose that we have a flat family of quiver sheaves over the spectrum of a discrete valuation ring. The theorem then states that if the fiber over the open point is semistable, the family can be modified over the closed point such that the fiber there becomes semistable as well.

For the proof we first introduce some technicalities, which are perhaps not of general interest outside of this section. For the proof of the theorem itself, we mostly follow the reasoning of Huybrechts and Lehn (2010), Theorem 2.B.1.

Fix a quiver Q, a projective and smooth scheme X over k, a dimension $d\le \dim (X)$ and a stability condition $\left( {\underline{L}},\sigma \right)$ on Q.

For a quiver sheaf ${\mathcal {E}}$ we consider the number

$$\begin{aligned} s({\mathcal {E}})=\max (\dim ({\mathcal {E}}_i))\in {\mathbb {N}}. \end{aligned}$$

Following Huybrechts and Lehn (2010) Definition 1.6.1, the category $Q-{\mathrm {Coh}}(X)_s$ is given as the full subcategory of the category $Q-{\mathrm {Coh}}(X)$ of quiver sheaves ${\mathcal {E}}$ with $s({\mathcal {E}})\le s$. Clearly,

$$\begin{aligned} Q-{\mathrm {Coh}}(X)_s\subset Q-{\mathrm {Coh}}(X)_t \end{aligned}$$

for $s\le t$ is a full subcategory, which obviously is closed under subobjects, quotients and extensions. Hence it forms a Serre subcategory, and we can consider the quotient category

$$\begin{aligned} Q-{\mathrm {Coh}}(X)_{d,d'}=\left( Q-{\mathrm {Coh}}(X)_d\right) /\left( Q-{\mathrm {Coh}}(X)_{d'-1}\right) . \end{aligned}$$

For background on this construction we refer to Gabriel (1962). The quotient category is again abelian and the canonical functor

$$\begin{aligned} Q-{\mathrm {Coh}}(X)_d\rightarrow Q-{\mathrm {Coh}}(X)_{d,d'} \end{aligned}$$

is exact (Gabriel 1962, Lemma III.1). Note that two objects ${\mathcal {E}},{\mathcal {F}}$ in $Q-{\mathrm {Coh}}(X)_{d,d'}$ are isomorphic if and only if there exists an ordinary morphism $\phi :{\mathcal {E}}\rightarrow {\mathcal {F}}$ such that kernel and cokernel of $\phi$ are contained in $Q-{\mathrm {Coh}}(X)_{d'-1}$ (Gabriel (1962) Lemma III.4). In this case we say that they are isomorphic in dimension $d'$.

By additivity of Hilbert polynomials on exact sequences we thus get a well-defined map

$$\begin{aligned} P^\sigma :Q-{\mathrm {Coh}}(X)_{d,d'}\rightarrow {\mathbb {R}}[T]_{d,d'}, \end{aligned}$$

assigning to a quiver sheaf its multi-Hilbert polynomial with respect to the fixed stability condition. Here, ${\mathbb {R}}[T]_{d,d'}$ denotes the ordered vector space of polynomials of degree at most d modulo polynomials of degree at most $d'-1$. By

$$\begin{aligned} p({\mathcal {E}})=p^\sigma ({\mathcal {E}}) \end{aligned}$$

we denote the reduced version.

We say that ${\mathcal {E}}$ is pure in $Q-{\mathrm {Coh}}(X)_{d,d'}$ if $T_{d-1}({\mathcal {E}})=T_{d'-1}({\mathcal {E}})$. The definition of semistability and stability applies to the relative setting, where we replace the multi-Hilbert polynomial by its class in ${\mathbb {R}}[T]_{d,d'}$. This satisfies the properties of a stability condition in the sense of Rudakov (1997).

Remark C.1

Clearly, for $d'=0$ we recover the definition of semistability in $Q-{\mathrm {Coh}}(X)$, and for $d'=d-1$ we obtain slope semistability. The case $d'=d$ is trivial because the reduced Hilbert polynomial is just the monomial $\frac{1}{d!}T^d$.

Remark C.2

Unless emphasized differently, we are concerned with ordinary quiver sheaves in the proof of Theorem C.7. That is, in writing ${\mathcal {F}}\in Q-{\mathrm {Coh}}(X)_{d,d'}$ we refer to an object in $Q-{\mathrm {Coh}}(X)_{d,d'}$ which is represented by the quiver sheaf ${\mathcal {F}}$.

By Proposition 1.9 of Rudakov (1997), there exist maximally destabilizing subobjects for objects in $Q-{\mathrm {Coh}}(X)_{d,d'}$. For technical reasons we want to make sure that these subobjects are represented by saturated quiver subsheaves.

Lemma C.3

The maximally destabilizing subobject

$$\begin{aligned} {\mathcal {G}}\subset {\mathcal {F}}\end{aligned}$$

of any quiver sheaf ${\mathcal {F}}\in Q-{\mathrm {Coh}}(X)_{d,d'}$ is represented by an actual quiver subsheaf ${\mathcal {G}}\subset {\mathcal {F}}$ which is saturated.

Proof

First consider any representative and the map $i:{\mathcal {G}}\rightarrow {\mathcal {F}}$ giving the inclusion. This provides the diagram

Since ${\mathrm {ker}}(i)$ is contained in $Q-{\mathrm {Coh}}(X)_{d'-1}$, we may replace ${\mathcal {G}}$ by ${\mathcal {G}}/{\mathrm {ker}}(i)$. In the second step consider the saturation

$$\begin{aligned} {\mathcal {G}}\subset {\mathcal {G}}_{{\mathrm {sat}}}\subset {\mathcal {F}}. \end{aligned}$$

Assuming ${\mathcal {G}}_{{\mathrm {sat}}}/{\mathcal {G}}$ not to be contained in $Q-{\mathrm {Coh}}(X)_{d'-1}$, we have a strict inclusion ${\mathcal {G}}\subset {\mathcal {G}}_{sat}$ in the quotient category. But this contradicts the maximality of ${\mathcal {G}}$ because ${\mathcal {G}}_{{\mathrm {sat}}}$ has larger multi-Hilbert polynomial (both ordinary and modulo smaller degrees). Hence, ${\mathcal {G}}$ and ${\mathcal {G}}_{{\mathrm {sat}}}$ are isomorphic in ${\mathrm {Coh}}_{d,d'}(X)$. $\square$

We need another preparatory lemma.

Lemma C.4

Suppose that ${\mathcal {E}}$ and ${\mathcal {F}}$ are quiver sheaves projective scheme X of dimension n such that $s({\mathcal {E}}),s({\mathcal {F}})\le d$ and such that there is an isomorphism $\varphi :{\mathcal {E}}\rightarrow {\mathcal {F}}$ in dimension $d-1$. Then the induced morphism

$$\begin{aligned} \varphi ^D:{\mathcal {F}}^D\rightarrow {\mathcal {E}}^D \end{aligned}$$

is an isomorphism.

Proof

It is sufficient to prove the corresponding assertion about sheaves on X in a functorial way. To that end, consider the exact sequence

$$\begin{aligned} 0\rightarrow {\mathrm {ker}}(\varphi ) \rightarrow F \rightarrow {\mathrm {im}}(\varphi ) \rightarrow 0, \end{aligned}$$

where by assumption the codimension c of the kernel is greater equal to $n-d$. Consider the induced exact sequence

$$\begin{aligned} {\mathrm {Ext}}^{c-1}({\mathrm {ker}}(\varphi ),\omega _X)&\rightarrow {\mathrm {Ext}}^{c}({\mathrm {im}}(\varphi ),\omega _X)\\&\rightarrow {\mathrm {Ext}}^{c}(F,\omega _X)\rightarrow {\mathrm {Ext}}^{c}({\mathrm {ker}}(\varphi ),\omega _X). \end{aligned}$$

By Huybrechts and Lehn (2010) Proposition 1.1.6 both terms involving the kernel vanish. Hence there is an isomorphism

$$\begin{aligned} \varphi ^D:{\mathrm {Ext}}^{c}({\mathrm {im}}(\varphi ),\omega _X)\rightarrow F^D. \end{aligned}$$

Applying the same argument to the cokernel sequence shows that

$$\begin{aligned} G^D \rightarrow {\mathrm {Ext}}^{c}({\mathrm {im}}(\varphi ),\omega _X) \end{aligned}$$

is also an isomorphism, which finishes the proof. $\square$

Let X denote a projective scheme over an algebraically closed field k of characteristic 0, and consider a field extension $k\subset K$. Denote by ${\mathcal {E}}_K$ the base change of a quiver sheaf ${\mathcal {E}}$ on X, and by ${\underline{L}}_K$ the base change of the tuple ${\underline{L}}$. We now show that semistability is preserved by field extensions. This is a variant of Huybrechts and Lehn (2010), Theorem 1.3.7.

Proposition C.5

Let ${\mathcal {E}}$ denote a pure quiver sheaf on a projective scheme X over k which is semistable with respect to some stability condition $({\underline{L}},\sigma )$. Consider a finitely generated field extension $k\subset K$. The pullback ${\mathcal {E}}_K$ is a semistable quiver sheaf on $X_K$ with respect to $\left( {\underline{L}}_K,\sigma \right)$.

Proof

We even claim that the Harder–Narasimhan filtrations are compatible in the sense that

$$\begin{aligned} {\mathrm {HN}}_i\left( {\mathcal {E}}_K\right) ={\mathrm {HN}}_i\left( {\mathcal {E}}\right) _K. \end{aligned}$$

First note that any morphism $l\rightarrow L$ of fields induces a flat morphism ${\mathrm {Spec}}(L)\rightarrow {\mathrm {Spec}}(l)$, so that

$$\begin{aligned} h^0\left( X_L,E_L\right) =h^0\left( X_l,E_l\right) \end{aligned}$$

for any coherent sheaf $E_l$ on $X_l$ (compare with the proof of Hartshorne (1977) Proposition III.9.3). In particular, this implies that the Hilbert polynomials for quiver sheaves ${\mathcal {E}}$ on $X=X_k$ remain identical in the sense that

$$\begin{aligned} p^{\left( {\underline{L}},\sigma \right) }\left( {\mathcal {E}}\right) =p^{\left( {\underline{L}}_K,\sigma \right) }\left( {\mathcal {E}}_K\right) . \end{aligned}$$

Further, the flatness implies that quiver subsheaves and quiver subquotients of ${\mathcal {E}}$ get mapped to quiver subsheaves and quiver subquotients of ${\mathcal {E}}_K$ respectively.

A first consequence of these remarks is that if ${\mathcal {E}}_K$ is semistable, so is ${\mathcal {E}}$. If we can then show that the Harder–Narasimhan filtration of ${\mathcal {E}}_K$ is induced by some filtration ${\mathcal {F}}^*$ of ${\mathcal {E}}$ in the sense that ${\mathrm {HN}}_i\left( {\mathcal {E}}_K\right) =\left( {\mathcal {F}}^i\right) _K$, then ${\mathcal {F}}^*$ satisfies the properties of the Harder–Narasimhan filtration of ${\mathcal {E}}$.

By induction on the number of generators of the field extension $k\subset K$ we reduce to the case that $K=k(x)$, where x is either transcendental or algebraic and hence separable over k (note that k is perfect).

In the separable case we pass to the normal hull, so that we may assume the extension to be Galois. Thus, ${\mathrm {HN}}_i({\mathcal {E}}_K)$ is induced by a quiver subsheaf ${\mathcal {F}}^i\subset {\mathcal {E}}$ if and only if ${\mathrm {HN}}_i({\mathcal {E}}_K)$ is invariant under the induced action of $G={\mathrm {Gal}}\left( K/k\right)$ on ${\mathcal {E}}_K$. To see this, we note that the corresponding descent question for sheaves is locally a question whether a submodule $N\subset M\otimes _k K$ over $R\otimes _k K$, where M is a module over some k-algebra R, is induced by a submodule $N'\subset M$ if N is invariant under the induced action of G on $M\otimes _k K$. This is true by Milne (2015), Proposition 16.7, with $N'=M\otimes _k k\cap N$. Clearly, these descents are also respected by induced morphisms $f\otimes _k K:M_1\otimes _k K\rightarrow M_2\otimes _k K$.

In our situation, we can see that for any $g\in G$ the $g^*{\mathrm {HN}}_i\left( {\mathcal {E}}_K\right)$ satisfy the properties of the Harder–Narasimhan filtration by applying our initial remarks to the induced morphism $g:{\mathrm {Spec}}(K)\rightarrow {\mathrm {Spec}}(K)$. Hence the Harder–Narasimhan filtration is invariant and we are done.

In the case where x is transcendental over k, i.e. $K=k(x)$ is the field of rational functions, we can use a similar argument using the relative automorphism group $G=\mathrm {Aut}\left( K/k\right)$, once we note that the relative automorphisms

$$\begin{aligned} x\mapsto ax \end{aligned}$$

for $a\in k^*$ have k as their fixed point field (this follows from the fact that there are no invariant polynomials by using Mukai (2003) Proposition 6.2). $\square$

Lemma C.6

Let $X\rightarrow S$ denote a morhpism of finite type between noetherian schemes. Suppose that $S_0\subset S$ is a closed subscheme defined by a nilpotent ideal sheaf ${\mathcal {I}}\subset {\mathcal {O}}_S$. Then a quiver sheaf ${\mathcal {F}}$ on X is flat over S if and only if it is flat over $S_0$ and the natural multiplication map

$$\begin{aligned} {\mathcal {I}}\otimes _S {\mathcal {F}}\rightarrow {\mathcal {I}}F \end{aligned}$$

is an isomorphism.

Proof

This follows from the sheaf version Huybrechts and Lehn (2010) Lemma 2.1.3 once we note that the notion of flatness can be checked at each vertex and the natural multiplication map for sheaves extends to quiver sheaves. $\square$

We are ready to prove Langton’s theorem for families of semistable quiver sheaves.

Theorem C.7

Let R denote a discrete valuation ring with maximal ideal ${\mathfrak {m}}=(\pi )$, field of fractions K, and residue field k.

Let ${\mathcal {F}}$ be an R-flat family of d-dimensional quiver sheaves on X such that ${\mathcal {F}}_K={\mathcal {F}}\otimes _R K$ is semistable in $Q-{\mathrm {Coh}}(X_K)_{d,d'}$ for some $d'<d$. Then there exists a quiver subsheaf ${\mathcal {E}}\subset {\mathcal {F}}$ such that ${\mathcal {E}}_K={\mathcal {F}}_K$ and such that ${\mathcal {E}}_k$ is also semistable in $Q-{\mathrm {Coh}}(X)_{d,d'}$.

Proof

We prove the following (stronger) auxiliary statement:

Suppose that for $d'\le \delta <d$ we have that ${\mathcal {F}}_k$ is semistable in $Q-{\mathrm {Coh}}(X)_{d,\delta +1}$ in addition to the assumptions of the theorem. Then there is a quiver subsheaf ${\mathcal {E}}\subset {\mathcal {F}}$ such that ${\mathcal {E}}_K={\mathcal {F}}_K$ and ${\mathcal {E}}_k$ is semistable in $Q-{\mathrm {Coh}}(X)_{d,\delta }$.

Assuming that the auxiliary statement is true we obtain the statement of the theorem by induction on $\delta$, where the case $\delta =d-1$ is trivial (compare with Remark C.1). From now on we assume that the auxiliary statement is false. By recursion we will define a descending sequence of quiver sheaves on $X_R$

$$\begin{aligned} {\mathcal {F}}={\mathcal {F}}^0\supset {\mathcal {F}}^1 \supset {\mathcal {F}}^2 \supset \ldots , \end{aligned}$$

where ${\mathcal {F}}^n_K={\mathcal {F}}_K$ for all n. Under the assumption that the auxiliary statement is false each ${\mathcal {F}}^n_k$ is unstable in $Q-{\mathrm {Coh}}(X)_{d,\delta }$.

Suppose that ${\mathcal {F}}^n$ was already defined. Let ${\mathcal {K}}^n\subset {\mathcal {F}}^n_k$ be a saturated representative for the maximally destabilizing quiver subsheaf (given by Lemma C.3) and define ${\mathcal {G}}^n={\mathcal {F}}^n_k/{\mathcal {K}}^n$ (note that ${\mathcal {G}}^n$ is pure). Then ${\mathcal {F}}^{n+1}$ is given as

$$\begin{aligned} {\mathcal {F}}^{n+1}={\mathrm {ker}}\left( {\mathcal {F}}^n\rightarrow {\mathcal {F}}^n_k\rightarrow {\mathcal {G}}^n \right) . \end{aligned}$$

Note for later use that this implies ${\mathcal {K}}^{n-1}={\mathcal {F}}^n/\pi {\mathcal {F}}^{n-1}$. Because outside of the closed point ${\mathrm {Spec}}(k)$, that is on $X_K$, every section gets mapped to zero we have ${\mathcal {F}}^{n+1}_K={\mathcal {F}}^n_K$.

There is an obvious exact sequence

$$\begin{aligned} (S1): 0 \rightarrow {\mathcal {K}}^n \rightarrow {\mathcal {F}}^n_k \rightarrow {\mathcal {G}}^n \rightarrow 0. \end{aligned}$$

Furthermore, we may now construct a second exact sequence

$$\begin{aligned} (S2): 0 \rightarrow {\mathcal {G}}^n \rightarrow {\mathcal {F}}^{n+1}_k \rightarrow {\mathcal {K}}^n \rightarrow 0. \end{aligned}$$

Note that using induction these two exact sequences imply that

$$\begin{aligned} (EQ1): P^\sigma ({\mathcal {F}}^n_k)=P^\sigma ({\mathcal {F}}_k)\in {\mathbb {R}}[T] \end{aligned}$$

for all n.

To construct the second sequence, first note that ${\mathcal {F}}^n_k={\mathcal {F}}^n/\pi {\mathcal {F}}^n$, where we denote the projection map as

$$\begin{aligned} q:{\mathcal {F}}^n\rightarrow {\mathcal {F}}^n_k={\mathcal {F}}^n/\pi {\mathcal {F}}^n. \end{aligned}$$

By construction of ${\mathcal {F}}^{n+1}$ and the universal property of the kernel we have an induced map $q_0:{\mathcal {F}}^{n+1}\rightarrow {\mathcal {K}}^n$. Note that $\pi {\mathcal {F}}^n\subset {\mathcal {F}}^{n+1}$, and $\pi {\mathcal {F}}^n$ clearly gets annihilated by q. Hence we obtain an induced morphism

Further note that $\pi {\mathcal {F}}^{n+1}\subset \pi {\mathcal {F}}^n$, so that $q''$ induces a map

$$\begin{aligned} q':{\mathcal {F}}^{n+1}_k={\mathcal {F}}^{n+1}/\pi {\mathcal {F}}^{n+1}\rightarrow {\mathcal {K}}^n. \end{aligned}$$

The kernel of this map consists of (classes of) sections of ${\mathcal {F}}^{n+1}$ which get annihilated by $q_0$. Hence

$$\begin{aligned} {\mathrm {ker}}(q')={\mathrm {ker}}(q_0)/\pi {\mathcal {F}}^{n+1}=\pi {\mathcal {F}}^n/\pi {\mathcal {F}}^{n+1}. \end{aligned}$$

By flatness, we further know that $\pi {\mathcal {F}}^n\simeq {\mathcal {F}}^n$ and $\pi {\mathcal {F}}^{n+1}\simeq {\mathcal {F}}^{n+1}$. Since the maps used for the construction of ${\mathcal {F}}^{n+1}$ are surjective we also have ${\mathcal {G}}^n\simeq {\mathcal {F}}^n/{\mathcal {F}}^{n+1}$. This yields ${\mathrm {ker}}(q')\simeq {\mathcal {G}}^n$.

To finish the construction of (S2) observe that the surjectivity of $q'$ is inherited from $q_0$.

We define ${\mathcal {C}}^n={\mathcal {G}}^n\cap {\mathcal {K}}^{n+1}$ considered as quiver subsheaves of ${\mathcal {F}}_k^{n+1}$ via the exact sequences above. Then the exact sequence (S2) and the obvious inclusions ${\mathcal {C}}^n\subset {\mathcal {G}}^n$ and ${\mathcal {C}}^n\subset {\mathcal {K}}^{n+1}$ induce a map ${\mathcal {K}}^{n+1}/{\mathcal {C}}^n\rightarrow {\mathcal {K}}^n$

A proof by diagram chasing shows that i is an inclusion (note that ${\mathcal {C}}^n$ is a pullback). In a similar fashion, using sequence (S1), we get a monomorphism ${\mathcal {G}}^n/{\mathcal {C}}^n\rightarrow {\mathcal {G}}^{n+1}$.

Assuming that ${\mathcal {C}}^n$ is not isomorphic to zero in $Q-{\mathrm {Coh}}(X_k)_{d,\delta }$ yields a contradiction as follows. In case ${\mathcal {C}}^n={\mathcal {K}}^{n+1}$ we get the inequalities

$$\begin{aligned} (IE1): p^\sigma ({\mathcal {K}}^{n+1})=p^\sigma ({\mathcal {C}}^n)\le p^\sigma _{\max }({\mathcal {G}}^n)<p^\sigma ({\mathcal {K}}^n)\in {\mathbb {R}}[T]_{d,\delta }. \end{aligned}$$

The rightmost inequality holds because $p^\sigma _{\max }({\mathcal {G}}^n)$ and $p^\sigma ({\mathcal {K}}^n)$ are the second and first Harder–Narasimhan weights of ${\mathcal {F}}^n_k$ respectively. In case ${\mathcal {C}}^n\ne {\mathcal {K}}^{n+1}$ we have the inequalities

$$\begin{aligned} (IE2): p^\sigma ({\mathcal {C}}^n)< p^\sigma ({\mathcal {K}}^{n+1})< p^\sigma ({\mathcal {K}}^{n+1}/{\mathcal {C}}^n)\le p^\sigma ({\mathcal {K}}^n)\in {\mathbb {R}}[T]_{d,\delta }. \end{aligned}$$

The first and last inequalities hold by the defining property of a destabilizing subobject. Note that the first inequality is strict because of the assumption, and the latter inequality also uses the inclusion constructed above. The inequality in the middle is implied by the first one using the obvious exact sequence.

In any case we have

$$\begin{aligned} (IE3): p^\sigma ({\mathcal {K}}^{n+1})\le p^\sigma ({\mathcal {K}}^n)\in {\mathbb {R}}[T]_{d,\delta }. \end{aligned}$$

If ${\mathcal {C}}^n$ is not isomorphic to zero this holds by (IE1) and (IE2). And if ${\mathcal {C}}^n\simeq 0$ this holds because we have the inclusion ${\mathcal {K}}^{n+1}={\mathcal {K}}^{n+1}/{\mathcal {C}}^n\subset {\mathcal {K}}^n$.

Since we assume ${\mathcal {F}}_k$ to be semistable in $Q-{\mathrm {Coh}}(X)_{d,\delta +1}$ we have that $p^\sigma ({\mathcal {K}}^n)\le p^\sigma ({\mathcal {F}}_k^n)\in {\mathbb {R}}[T]_{d,\delta +1}$. But strict inequality would also imply

$$\begin{aligned} p^\sigma ({\mathcal {K}}^n)<p^\sigma ({\mathcal {F}}_k^n)\in {\mathbb {R}}[T]_{d,\delta }, \end{aligned}$$

contradicting the fact that ${\mathcal {F}}^n_k$ is not semistable. Together with (EQ1) we thus arrive at

$$\begin{aligned} (EQ2): p^\sigma ({\mathcal {K}}^n)=p^\sigma ({\mathcal {F}}_k)\in {\mathbb {R}}[T]_{d,\delta +1}. \end{aligned}$$

Hence

$$\begin{aligned} p^\sigma ({\mathcal {K}}^n)-p^\sigma ({\mathcal {F}}_k)=\beta _n T^{\delta }\in {\mathbb {R}}[T]_{d,\delta } \end{aligned}$$

for some $\beta _n\in {\mathbb {R}}$. We need some properties of the sequence $\beta _n\in {\mathbb {R}}$.

Because $p^\sigma ({\mathcal {K}}^n)>p^\sigma ({\mathcal {F}}^n_k)=p^\sigma ({\mathcal {F}}_k)\in {\mathbb {R}}[T]_{d,\delta }$, which holds by unstability of ${\mathcal {F}}^n_k$ and (EQ1), the $\beta _n$ are strictly positive, and by (IE3) their sequence is decreasing. Finally, the possible values for $\beta _n$ are contained in discrete set (consider Lemma C.8). Hence, $\beta _n$ must become stationary for $n\gg 0$, and without loss of generality we restrict ourselves to such n in the following.

This implies $p^\sigma ({\mathcal {K}}^n)=p^\sigma ({\mathcal {K}}^{n+1})$ in ${\mathbb {R}}[T]_{d,\delta }$, and because this contradicts both (IE1) and (IE2) it implies furthermore that

$$\begin{aligned} {\mathcal {G}}^n\cap {\mathcal {K}}^{n+1}={\mathcal {C}}^n\simeq 0. \end{aligned}$$

Observe that ${\mathcal {C}}^n$ is a quiver subsheaf of a pure quiver sheaf of dimension d (${\mathcal {G}}^n$ or ${\mathcal {K}}^{n+1}$). But since it can not have dimension d it must equal zero, so that there are inclusions $i:{\mathcal {K}}^{n+1}\rightarrow {\mathcal {K}}^n$ as well as $j:{\mathcal {G}}^n\rightarrow {\mathcal {G}}^{n+1}$ in $Q-{\mathrm {Coh}}(X_k)_{d,\delta }$.

Note that $P^\sigma ({\mathcal {K}}^n)=P^\sigma ({\mathcal {K}}^{n+1})\in {\mathbb {R}}[T]_{d,\delta }$ as well for large enough n because the rank of the ${\mathcal {K}}^n$ can not descend forever. Combined with (EQ1) this gives

$$\begin{aligned} (EQ3): P^\sigma ({\mathcal {G}}^n)=P^\sigma ({\mathcal {G}}^{n+1})\in {\mathbb {R}}[T]_{d,\delta }. \end{aligned}$$

Since ${\mathcal {G}}^n$ is pure the kernel of j is either zero or of dimension d. Clearly the latter is absurd, and hence there are actual inclusions $j:{\mathcal {G}}^n\subset {\mathcal {G}}^{n+1}$. Thus we get exact sequences

$$\begin{aligned} 0\rightarrow {\mathcal {G}}^n \rightarrow {\mathcal {G}}^{n+1} \rightarrow {\mathcal {G}}^n/{\mathcal {G}}^{n+1} \rightarrow 0, \end{aligned}$$

where (EQ3) implies that the rightmost term is isomorphic to zero in the category $Q-{\mathrm {Coh}}(X_k)_{d,\delta }$. So the ${\mathcal {G}}^n$ are isomorphic in dimension $\delta$ and thus in particular in dimension $d-1$. By Lemma C.4 their reflexive hulls $({\mathcal {G}}^n)^{DD}$ are all isomorphic, so that the sequence

$$\begin{aligned} {\mathcal {G}}^0\subset {\mathcal {G}}^1 \subset {\mathcal {G}}^2 \subset \cdots \end{aligned}$$

is an increasing sequence of quiver subsheaves of the fixed quiver sheaf which is given as this reflexive hull, and must thus become stationary. Again we restrict to the case where $n\gg 0$ is such that this is the case and set ${\mathcal {G}}={\mathcal {G}}^n$.

We can see that this implies that the sequences (S1) and (S2) split as follows. Consider the diagram

where the first row is given by (S1) and the middle morphism as the sum of the injective morphisms in (S1) and (S2). Thus there is an induced morphism ${\mathcal {G}}^n\rightarrow {\mathcal {G}}^{n+1}$. By construction, this is exactly the inclusion morphism ${\mathcal {G}}^n\subset {\mathcal {G}}^{n+1}$, which turned out to be an isomorphism, so that

$$\begin{aligned} {\mathcal {F}}^{n+1}_k\simeq {\mathcal {K}}^{n+1}\oplus {\mathcal {G}}^n \simeq {\mathcal {K}}\oplus {\mathcal {G}}\end{aligned}$$

for all n large enough.

Define ${\mathcal {E}}^n={\mathcal {F}}/{\mathcal {F}}^n$. Because $\pi {\mathcal {F}}^n\subset {\mathcal {F}}^{n+1}$, and so by induction $\pi ^n{\mathcal {F}}\subset {\mathcal {F}}^n$, there is a well-defined quotient map

$$\begin{aligned} {\mathcal {F}}/\pi ^n{\mathcal {F}}\rightarrow {\mathcal {F}}/{\mathcal {F}}^n={\mathcal {E}}^n. \end{aligned}$$

By the local flatness criterion for quiver sheaves C.6 we thus know that ${\mathcal {E}}^n$ is flat over $R/\pi ^n$.

Next, we want to show ${\mathcal {E}}^n_k\simeq {\mathcal {G}}$, which gives us the topological type of ${\mathcal {E}}^n$.

Using Noether’s isomorphism theorem we obtain

$$\begin{aligned} {\mathcal {E}}^n_k&=({\mathcal {F}}/{\mathcal {F}}^n)/(\pi ({\mathcal {F}}/{\mathcal {F}}^n))=({\mathcal {F}}/{\mathcal {F}}^n)/((\pi {\mathcal {F}}+{\mathcal {F}}^n)/{\mathcal {F}}^n)\simeq {\mathcal {F}}/(\pi {\mathcal {F}}+{\mathcal {F}}^n)\\&\simeq ({\mathcal {F}}/\pi {\mathcal {F}})/(({\mathcal {F}}^n+\pi {\mathcal {F}})/\pi {\mathcal {F}})={\mathcal {F}}_k/{\mathrm {im}}(\alpha ), \end{aligned}$$

where $\alpha$ is the composition of the morphisms

$$\begin{aligned} \alpha _n:{\mathcal {F}}^n_k\rightarrow {\mathcal {F}}^{n-1}_k,~f+\pi {\mathcal {F}}^n\mapsto f+\pi {\mathcal {F}}^{n-1}. \end{aligned}$$

Note that

$$\begin{aligned} {\mathrm {ker}}(\alpha _n)&=\pi {\mathcal {F}}^{n-1}/\pi {\mathcal {F}}^n\simeq {\mathcal {F}}^{n-1}/{\mathcal {F}}^n\simeq {\mathcal {G}}^{n-1}\\ {\mathrm {im}}(\alpha _n)&={\mathcal {F}}^n/\pi {\mathcal {F}}^{n-1}={\mathcal {K}}^{n-1}, \end{aligned}$$

which implies that we get decompositions

$$\begin{aligned} {\mathcal {F}}^n_k={\mathcal {K}}^n\oplus {\mathcal {G}}^{n-1}={\mathrm {im}}(\alpha _{n+1}) \oplus {\mathrm {ker}}(\alpha _n). \end{aligned}$$

Hence ${\mathrm {im}}(\alpha )={\mathrm {im}}(\alpha _1)={\mathcal {K}}^0$ and ${\mathcal {E}}^n_k={\mathcal {G}}^0\simeq {\mathcal {G}}$ as desired.

Summarizing these results we have shown that ${\mathcal {E}}^n$ is a quotient

$$\begin{aligned} {\mathcal {F}}_{R_n}\rightarrow {\mathcal {E}}^n \rightarrow 0, \end{aligned}$$

which is flat over $R_n=R/\pi ^n$. This corresponds to a morphism $\phi$ over ${\mathrm {Spec}}(R)$ which fits into a diagram

Hence the image of $\sigma$ contains the closed subschemes ${\mathrm {Spec}}(R_n)$ of ${\mathrm {Spec}}(R)$ for all n, which is only possible if $\sigma$ is surjective.

The morphism ${\mathrm {Spec}}(K)\rightarrow {\mathrm {Spec}}(R)$ corresponds to a point $y\in {\mathrm {Spec}}(R)$ such that $k(y)\subset K$. By surjectivity of $\sigma$ we can find an inverse image $x\in {\mathrm {Quot}}^{\tau ({\mathcal {G}})}_{{\mathcal {F}}/X_R/R}({\mathcal {F}},\tau ({\mathcal {G}}))$. Let $K'$ denote the common extension of the induced field extension $k(x)\subset k(y)$ and the extension $k(x)\subset K$, so that

Reversing the correspondences used above the extension $k(y)\subset K'$ gives a morphism ${\mathrm {Spec}}(K')\rightarrow {\mathrm {Quot}}^{\tau ({\mathcal {G}})}_{{\mathcal {F}}/X_R/R}$ over ${\mathrm {Spec}}(R)$ and hence a quotient

$$\begin{aligned} {\mathcal {F}}_{K'}\rightarrow {\mathcal {U}}\rightarrow 0 \end{aligned}$$

with topological type $\tau ({\mathcal {G}})$. By Proposition C.5 we know that ${\mathcal {F}}_{K'}$ is semistable. But by our assumptions, the Hilbert polynomials satisfy the inequality

$$\begin{aligned} p^\sigma ({\mathcal {U}})=p^\sigma ({\mathcal {G}})>p^\sigma ({\mathcal {F}}^n_k)=p^\sigma ({\mathcal {F}}_{K'}). \end{aligned}$$

This is a contradiction. $\square$

The fact that the stability condition $\sigma$ is rational is crucial for the validity of the proof of Theorem C.7. Because of its significance, we state the relevant step as a separate lemma.

Lemma C.8

With notation as in the proof of Theorem C.7, the numbers $\beta _n$ such that

$$\begin{aligned} p^\sigma ({\mathcal {K}}^n)-p^\sigma ({\mathcal {F}}_k)=\beta _nT^\delta \end{aligned}$$

are contained in a discrete set, if $\sigma$ is rational.

Proof

Since the polynomial $p^\sigma ({\mathcal {F}}_k)$ is independent of n, it remains to show that the coefficients of $p^\sigma ({\mathcal {K}}^n)$ take values in a discrete set. Such a coefficient is given as

$$\begin{aligned} \frac{\sum _{i\in Q_0}\sum _{j=1}^N \sigma _{ij}\alpha ^{L_j}_\delta ({\mathcal {K}}^n_i)}{\sum _{i\in Q_0}\sum _{j=1}^N \sigma _{ij}\alpha ^{L_j}_d({\mathcal {K}}^n_i)}. \end{aligned}$$

By construction, ${\mathcal {K}}^n$ is a quiver subsheaf of ${\mathcal {F}}^n_k$, so that each $\alpha ^{L_j}_d({\mathcal {K}}^n_i)$ is an integer between 1 and $\alpha ^{L_j}_d({\mathcal {K}}^n_i)$. The upper bound is independent of n because ${\mathcal {F}}^n_K={\mathcal {F}}^{n+1}_K$ and ${\mathcal {F}}^n$ is flat, so that there are only finitely many possible values for the denominator.

For $\delta <d$ it is well-known that the coefficients $\alpha ^{L_j}_\delta ({\mathcal {K}}^n_i)$ take value in some lattice $(1/r!){\mathbb {Z}}$. The numerator thus takes values in a set of the form

$$\begin{aligned} {\mathbb {Z}}a_1+\cdots +{\mathbb {Z}}a_r \end{aligned}$$

for finitely many rational numbers $a_r$. By factoring out the denominators of the $a_i$, we see that this set is contained in a cyclic subgroup ${\mathbb {Z}}\alpha \subset {\mathbb {R}}$, and is thus discrete. $\square$