Steady periodic rotational gravity waves with negative surface tension

Abstract

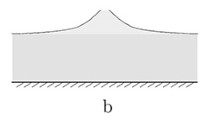
Consideration in this paper is the two-dimensional steady periodic rotational gravity waves with negative surface tension. Local curves of small amplitude solutions of the resulting problem are obtained by using the Crandall–Rabinowitz local bifurcation theory. By means of the global bifurcation theory combined with the Schauder theory of elliptic equations with the Venttsel boundary conditions, the curves of small amplitude solutions is extended to the global continuum of solutions. Furthermore, it is shown that those waves are necessarily symmetric about the crest under the assumption that their surface profiles are monotonic between troughs and crests and locally strictly monotonic near the troughs.

Appendix

For the sake of completeness, we present the maximum principles suitable for our setting in this section.

Theorem 5.1

[14] Let $\Omega \subset \mathbb {R}^{2}$ be a rectangle, $w \in C^{2} (\overline{\Omega })$, and suppose that $\mathcal {L}w =0$ for some uniformly elliptic operator $\mathcal {L}=\sum _{i,j=1}^{2}a_{ij}\partial _{ij}+\sum _{i=1}^{2}b_i\partial _i$ with continuous coefficients in $\overline{\Omega }$. Then the following statements hold:

(i) [The maximum principle] If $min_{\overline{\Omega }}w$ or $max_{\overline{\Omega }}w$ is attained in the interior of $\Omega$, then w is a constant in $\overline{\Omega }$.

(ii) [The Hopf boundary-point lemma] Let A be a point on the smooth part of the boundary $\partial \Omega$ such that $w(A)< w(X)$ (or $w(A)> w(X)$ ) for all $X \in \Omega$. Then the outer normal derivative of w at A is strictly negative (positive).

(iii) [The Serrin edge point lemma] Let $\Theta _0$ be a corner point of $\partial \Omega$ such that $w(\Theta _0)>w(\Theta )$ (or $w(\Theta _0)<w(\Theta )$) for all $\Theta \in \Omega$. If $a_{11}(\Theta _0)-0$, and $\tau$ is a unit vector outward from $\Omega$ at $\Theta _0$, then either the first or the second derivative of w in the direction of $\tau$ is strictly positive (negative).