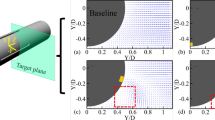
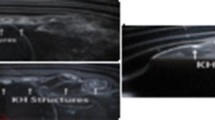
Abstract
Visualization experiments for flow around a cylinder with parallel slit placed inside a circular pipe are carried out with water as the working medium. Two different color dyes are employed to visualize the complex vortex formation mechanism behind the bluff bodies. The objective of this study is to explore the potential of cylinder with parallel slit as an improved vortex generator for various practical applications. Wake parameters, such as Strouhal Number, wake width, and vortex formation length, are calculated from the recorded images. Three different slit widths with non-dimensional separation ratio of 0.1, 0.2, and 0.4 are chosen to study the effect on the vortex formation mechanism and the corresponding wake parameters. The Reynolds number range covered in this study is Re D = 200–2300. Symmetric vortex formation from the outer surface is observed for lower slit widths, whereas for high slit widths, both in-phase and anti-phase shedding are observed. A separation bubble from each of the inner surface is formed which detaches from the bluff body to form a vortex at higher Reynolds numbers. The separation bubble is found to be both bi-stable and symmetric for lower slit widths. The interaction of the separation bubble with the outer vortices is seen to affect the strength of the primary/outer vortex. The slit width is seen to be an important parameter in determining the strength of the vortices. At higher slit width, the configuration behaves like a dual bluff body and strong crisscross vortex shedding is observed. Such novel kind of vortex formation mechanism can be utilized to improve the performance of numerous practical applications, such as flowmeters, heat exchangers, etc.
Graphical abstract















Similar content being viewed by others
Abbreviations
- B :
-
Blockage ratio (d/D)
- D :
-
Inner diameter of circular pipe (m)
- d :
-
Diameter of bluff body (m)
- f :
-
Frequency of vortex shedding (Hz)
- L′ :
-
Vortex formation length (m)
- L c :
-
Characteristic length (m)
- s :
-
Slit width (m)
- U m :
-
Mean velocity (m/s)
- W :
-
Wake width (m)
- Re D :
-
Reynolds number \(\left( {\frac{{\rho U_{\text{m}} D}}{\mu }} \right)\)
- St :
-
Strouhal number \(\left( {St = \frac{fd}{{U_{\text{m}} }}} \right)\)
- ρ :
-
Density of fluid (kg/m3)
- μ :
-
Dynamic viscosity of fluid (Pa s)
References
Agrawal A, Djenidi L, Antonia RA (2006) Investigation of flow around a pair of side-by-side square cylinders using the lattice Boltzmann method. Comput Fluids 35:1093–1107
Akaydin HD, Elvin N, Andreopoulos Y (2010) Energy harvesting from highly unsteady fluid flows using piezoelectric materials. J Intell Mater Syst Struct 21(13):1263–1278
Alam MM, Zhou Y (2007) Flow around two side-by-side closely spaced circular cylinders. J Fluids Struct 23(5):799–805
Alam MM, Zhou Y (2013) Intrinsic features of flow around two side-by-side square cylinders. Phys Fluids (1994–present) 25(8):085106
Baek H, Karniadakis GE (2009) Suppressing vortex-induced vibrations via passive means. J Fluids Struct 25(5):848–866
Bearman PW, Wadcock AJ (1973) The interaction between a pair of circular cylinders normal to a stream. J Fluid Mech 61(3):499–511
Burattini P, Agrawal A (2013) Wake interaction between two side-by-side cylinders in channel flow. Comput Fluids 77:134–142
Dhiman A, Ghosh R (2013) Computer simulation of momentum and heat transfer across an expanded trapezoidal bluff body. Int J Heat Mass Transf 59:338–352
Huang RF, Lin BH, Yen SC (2010) Time-averaged topological flow patterns and their influence on vortex shedding of a square cylinder in crossflow at incidence. J Fluids Struct 26(3):406–429
Igarashi T (1999) Flow resistance and Strouhal number of a vortex shedder in a circular pipe. JSME Int J Ser B Fluids Therm Eng 42(4):586–595
Ishigai S et al (1972) Experimental study on structure of gas flow in tube banks with tube axes normal to flow: Part 1, Karman vortex flow from two tubes at various spacings. Bull JSME 15(86):949–956
Kumar SR, Sharma A, Agrawal A (2008) Simulation of flow around a row of square cylinders. J Fluid Mech 606:369–397
Miau JJ, Wang HB, Chou JH (1996) Flopping phenomenon of flow behind two plates placed side-by-side normal to the flow direction. Fluid Dyn Res 17:311–328
Olsen JF, Rajagopalan S (2000) Vortex shedding behind modified circular cylinders. J Wind Eng Ind Aerodyn 86(1):55–63
Pankanin GL, Kulinczak A, Berlinski J (2007) Investigations of Karman vortex street using flow visualization and image processing. Sens Actuat A 138:366–375
Sumner D (2010) Two circular cylinders in cross-flow: a review. J Fluids Struct 26(6):849–899
Sumner D, Wong SST, Price SJ, Paidoussis MP (1999) Fluid behaviour of side-by-side circular cylinders in steady cross-flow. J Fluids Struct 13(3):309–338
Suzuki H et al (1993) Unsteady flow in a channel obstructed by a square rod (crisscross motion of vortex). Int J Heat Fluid Flow 14(1):2–9
Takamoto M, Terao Y (1999) Standardization of vortex shedding flowmeter. In: Proceedings of the 4th international symposium on fluid flow measurement, Denver
Venugopal A, Agrawal A, Prabhu SV (2010) Influence of blockage and upstream disturbances on the performance of a vortex flowmeter with a trapezoidal bluff body. Measurement 43(4):603–616
Venugopal A, Agrawal A, Prabhu SV (2011) Review on vortex flowmeter—designer perspective. Sens Actuat A 170(1):8–23
Venugopal A, Agrawal A, Prabhu SV (2012) Frequency detection in vortex flowmeter for low Reynolds number using piezoelectric sensor and installation effects. Sens Actuat A 184:78–85
Venugopal A, Agrawal A, Prabhu SV (2014) Note: a vortex cross-correlation flowmeter with enhanced turndown ratio. Rev Sci Instrum 85(6):066109
Wang ZJ, Zhou Y, Li H (2002) Flow-visualization of a two side-by-side cylinder wake. J Flow Vis Image Process 9:123–138
Williamson CHK (1985) Evolution of a single wake behind a pair of bluff bodies. J Fluid Mech 159:1–18
Zdravkovich MM (2003) Flow around circular cylinders, vol 2. Applications. Oxford University Press, Oxford
Acknowledgments
The authors acknowledge funding support for this research from Department of Science and Technology, New Delhi.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Ordia, L., Venugopal, A., Agrawal, A. et al. Vortex shedding characteristics of a cylinder with a parallel slit placed in a circular pipe. J Vis 20, 263–275 (2017). https://doi.org/10.1007/s12650-016-0398-y
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s12650-016-0398-y