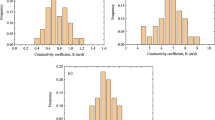
Abstract
Six series of solute transport experiments were conducted with an objective to compare scale decency of fractional dispersion coefficient (Df) in space fractional advection–dispersion equation (s-FADE) with that of dispersion coefficient (D) in advection–dispersion equation (ADE) at three flow rates. The experiments were performed in a 2.5 m × 0.1 m × 0.6 m (length × width × height) sandbox filled with a homogeneous or heterogeneous soil. The parameters of the s-FADE and ADE were estimated using an inverse method. The results indicated that the scale dependency of Df was significantly less than that of D in the heterogeneous soil. The maximum variations of Df and D for the heterogeneous soil were observed at the flow rate of 200 cm3 min–1, in which the ratios of the maximum Df and D to the minimum ones were 6.009 and 39.039, respectively. However, in the homogeneous soil, the scale dependency of Df was relatively similar to that of D. In this soil, the ratios of the maximum Df and D to the minimum ones were 3.109 and 5.041, respectively, which were observed at the flow rate of 548 cm3 min–1. Contrary to Df and D, the v values of the s-FADE and ADE for both soils varied in relatively similar ranges at the flow rates studied. Also, the fractional differentiation order (α) of the s-FADE described properly the heterogeneity degrees of the soils used. In a nutshell, the s-FADE is an efficient solute transport model to explain the solute transport process in natural porous media with different heterogeneity degrees.







Similar content being viewed by others
Abbreviations
- ADE:
-
Advection–dispersion equation
- s-FADE:
-
Space fractional advection-dispersion equation
- C :
-
Concentration
- \( \overline{C} \) :
-
Average of measured concentrations
- v :
-
Average pore-water velocity
- D :
-
Dispersion coefficient
- x :
-
Spatial coordinate (distance)
- t :
-
Temporal coordinate (time)
- L :
-
Size of spatial domain
- OF :
-
Objective function
- n :
-
Positive integer number
- N :
-
Total number of measurement points
- RMSE :
-
Root mean square error
- r 2 :
-
Determination coefficient
- meas :
-
Measured
- calc :
-
Calculated
- f :
-
Fractional
- α:
-
Order of fractional differentiation (heterogeneity degree)
- ξ :
-
Auxiliary variable
- τ :
-
Integration variable
- Γ(⋅) :
-
Gamma function
References
Benson DA, Wheatcraft SW, Meerschaert MM (2000a) Application of a fractional advection-dispersion equation. Water Resour Res 36(6):1403–1412. https://doi.org/10.1029/2000WR900031
Benson DA, Wheatcraft SW, Meerschaert MM (2000b) The fractional-order governing equation of Lévy motion. Water Resour Res 36(6):1413–1423. https://doi.org/10.1029/2000WR900032
Benson DA, Schumer R, Meerschaert MM (2001) Fractional dispersion, Lévy motion, and the MADE tracer tests. Transp Porous Med 42:211–240. https://doi.org/10.1023/A:1006733002131
Berkowitz B, Scher H (1995) On characterization of anomalous dispersion in porous and fractured media. Water Resour Res 31(6):1461–1466. https://doi.org/10.1029/95WR00483
Berkowitz B, Cortis A, Dentz M, Scher H (2006) Modeling non-Fickian transport in geological formations as a continuous time random walk. Rev Geophys 44:RG2003. https://doi.org/10.1029/2005RG000178
Chen J-S, Ni C-F, Liang C-P, Chiang C-C (2008) Analytical power series solution for contaminant transport with hyperbolic asymptotic distance dependent dispersivity. J Hydrol 362:142–149. https://doi.org/10.1016/j.jhydrol.2008.08.020
Clarke DD, Meerschaert MM, Wheatcraft SW (2005) Fractal travel time estimates for dispersive contaminants. Groundwater 43(2):401–407. https://doi.org/10.1111/j.1745-6584.2005.0025.x
Cushman JH (1987) Development of stochastic partial differential equations for subsurface hydrology. Stoch Hydrol Hydraul Risk Assess 1(4):241–262. https://doi.org/10.1007/BF01543097
Gao G, Zhan H, Feng SH, Huang G, Mao X (2009) Comparison of alternative models for simulating anomalous solute transport in a large heterogeneous soil column. J Hydrol 377:391–404. https://doi.org/10.1016/j.jhydrol.2009.08.036
Gelhar LW, Welty C, Rehfeldt KR (1992) A critical review of data on fieldscale dispersion in aquifer. Water Resour Res 28(7):1955–1974. https://doi.org/10.1029/92WR00607
Ghavanloo E, Rafii-Tabar H, Fazelzadeh SA (2019) Essential concepts from nonlocal elasticity theory. In: Computational continuum mechanics of nanoscopic structures. Springer Tracts in Mechanical Engineering. Springer, Cham, pp 241–260
Guerrero JSP, Skaggs TH (2010) Analytical solution for one-dimensional advection–dispersion transport equation with distance-dependent coefficients. J Hydrol 390:57–65. https://doi.org/10.1016/j.jhydrol.2010.06.030
Haggerty R, Gorelick SM (1995) Multiple-rate mass transfer for modeling diffusion and surface reactions in media with pore-scale heterogeneity. Water Resour Res 31(10):2383–2400. https://doi.org/10.1029/95WR10583
Hokmabadi NN, Sarfarazi V, Moshrefifar MR (2016) Investigation of separation non-persistent faults in fracture mechanism of rock bridge. Civil Eng J 2(7):348–357
Huang K, Toride N, Van Genuchten MT (1995) Experimental investigation of solute transport in large, homogeneous and heterogeneous, saturated soil columns. Transp Porous Med 18:283–302. https://doi.org/10.1007/BF00616936
Huang G, Huang Q, Zhan H (2006) Evidence of one-dimensional scale-dependent fractional advection–dispersion. J Contam Hydrol 85(1):53–71. https://doi.org/10.1016/j.jconhyd.2005.12.007
Huang Q, Huang G, Zhan H (2008) A finite element solution for the fractional advection–dispersion equation. Adv Water Resour 31:1578–1589. https://doi.org/10.1016/j.advwatres.2008.07.002
Kelly JF, Meerschaert MM (2019) The fractional advection-dispersion equation for contaminant transport. In: Tarasov VE (ed) Application in physics, part B, 1st edn. De Gruyter, Berlin, Boston, pp 129–150. https://doi.org/10.1515/9783110571721-006
Khafagy MM, Abd-Elmegeed MA, Hassan AE (2020) Simulation of reactive transport in fractured geologic media using random-walk particle tracking method. Arab J Geosci. 13. https://doi.org/10.1007/s12517-019-4952-5
Khan N, Gaurav D, Kandl T (2013) Performance evaluation of Levenberg-Marquardt technique in error reduction for diabetes condition classification. Procedia Comput Sci 18(2629):2637–2637. https://doi.org/10.1016/j.procs.2013.05.455
Kilbas AA, Srivastava HM, Trujillo JJ (2006) Theory and applications of fractional differential equations. Elsevier, Amsterdam
Lee J, Rolle M, Kitanidis PK (2018) Longitudinal dispersion coefficients for numerical modeling of groundwater solute transport in heterogeneous formations. J Contam Hydrol 212:41–54. https://doi.org/10.1016/j.jconhyd.2017.09.004
Lu S, Molz FJ (2002) Possible problems of scale dependency in applications of the three-dimensional fractional advection-dispersion equation to natural porous media. Water Resour Res. 38:4-1–4-7. https://doi.org/10.1029/2001WR000624
Martinez FSJ, Pachepsky YA, Rawls WJ (2010) Modelling solute transport in soil columns using advective–dispersive equations with fractional spatial derivatives. Adv Eng Softw 41:4–8. https://doi.org/10.1016/j.advengsoft.2008.12.015
Mehdinejadiani B (2017) Estimating the solute transport parameters of the spatial fractional advection-dispersion equation using Bees Algorithm. J Contam Hydrol 203:51–61. https://doi.org/10.1016/j.jconhyd.2017.06.004
Mehdinejadiani B, Naseri AA, Jafari H, Ghanbarzadeh A, Baleanu D (2013) A mathematical model for simulation of a water table profile between two parallel subsurface drains using fractional derivatives. Comput Math Appl 66:785–794. https://doi.org/10.1016/j.camwa.2013.01.002
Moradi G, Mehdinejadiani B (2018) Modeling solute transport in homogeneous and heterogeneous porous media using spatial fractional advection-dispersion equation. Soil Water Res 13:18–28. https://doi.org/10.17221/245/2016-SWR
Pachepsky Y, Benson D, Rawls W (2000) Simulating scale-dependent solute transport in soils with the fractional advective–dispersive equation. Soil Sci Soc Am J 64(4):1234–1243. https://doi.org/10.2136/sssaj2000.6441234x
Pang L, Hunt B (2001) Solutions and verification of a scale-dependent dispersion model. J Contam Hydrol 53:21–39. https://doi.org/10.1016/S0169-7722(01)00134-6
Pickens JF, Grisak GE (1981) Modeling of scale-dependent dispersion in hydrogeologic systems. Water Resour Res 17(4):1191–1211. https://doi.org/10.1029/WR017i006p01701
Saleem HA, Subyani AM, Elfeki A (2019) Solute transport model for groundwater contamination in Wadi Bani Malik, Jeddah. Saudi Arabia. Arab J Geosci. 12. https://doi.org/10.1007/s12517-019-4319-y
Sanskrityayn A, Kumar N (2016) Analytical solution of advection–diffusion equation in heterogeneous infinite medium using Green’s function method. J Earth Syst Sci 125(8):1713–1723. https://doi.org/10.1007/s12040-016-0756-0
Schumer R, Benson DA, Meerschaert MM, Wheatcraft SW (2001) Eulerian derivation of the fractional advection–dispersion equation. J Contam. Hydrol 48(1):69–88. https://doi.org/10.1016/S0169-7722(00)00170-4
Schumer R, Meerschaert MM, Baemuer B (2009) Fractional advection-dispersion equations for modeling transport at Earth surface. J Geophys Res 114:F00A07. https://doi.org/10.1029/2008JF001246
Sharma PK, Agarwal P, Mehdinejadiani B (2020) Study on non-Fickian behavior for solute transport through porous media. ISH J Hydraul Eng.:1–9. https://doi.org/10.1080/09715010.2020.1727783
Singh MK, Das P (2015) Scale dependent solute dispersion with linear isotherm in heterogeneous medium. J Hydrol 520:289–299. https://doi.org/10.1016/j.jhydrol.2014.11.061
Wałowski G (2018) Experimental assessment of porous material anisotropy and its effect on gas permeability. Civil Eng J 4(4):906–915. https://doi.org/10.28991/cej-0309143
Xiong Y, Huang G, Huang Q (2006) Modeling solute transport in one-dimensional homogeneous and heterogeneous soil columns with continuous time random walk. J Contam Hydrol 86(3):163–175. https://doi.org/10.1016/j.jconhyd.2006.03.001
Yates SR (1992) An analytical solution for one-dimensional transport in porous media with an exponential dispersion function. Water Resour Res 52:2149–2154. https://doi.org/10.1029/92WR01006
Zhang Y, Benson DA, Reeves DM (2009) Time and space nonlocality underlying fractional-derivative models: distinction and literature review of filed applications. Adv Water Resour 32:561–581. https://doi.org/10.1016/j.advwatres.2009.01.008
Zhang Y, Meerschaert MM, Neupauer RM (2016) Backward fractional advection dispersion model for contaminant source prediction. Water Resour Res 52:2462–2473. https://doi.org/10.1002/2015WR018515
Zhou L, Selim HM (2003) Application of the fractional advection-dispersion equation in porous media. Soil Sci Soc Am J 67:1079–1084. https://doi.org/10.2136/sssaj2003.1079
Zhou R, Zhan H, Chen K, Peng X (2018) Transport in a fully coupled asymmetric stratified system: comparison of scale dependent and independent dispersion schemes. J Hydrol X 1:100001. https://doi.org/10.1016/j.hydroa.2018.10.001
Acknowledgments
The authors would like to express thanks for financial support from University of Kurdistan (UOK). The authors also greatly appreciate anonymous reviewers for their valuable comments and suggestions to improve the manuscript.
Author information
Authors and Affiliations
Corresponding author
Additional information
Responsible Editor: Broder J. Merkel
Rights and permissions
About this article
Cite this article
Moradi, G., Mehdinejadiani, B. An experimental study on scale dependency of fractional dispersion coefficient. Arab J Geosci 13, 409 (2020). https://doi.org/10.1007/s12517-020-05438-z
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s12517-020-05438-z