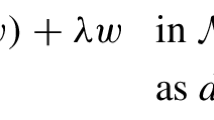Abstract
Let (M, g) be a closed Riemannian manifold of dimension \(n\ge 3\) and \(x_0 \in M\) be an isolated local minimum of the scalar curvature \(s_\mathrm{{g}}\) of g. For any positive integer k we prove that for \(\epsilon >0\) small enough the subcritical Yamabe equation \(-\epsilon ^2 \Delta u +(1+ c_{N} \ \epsilon ^2 s_\mathrm{{g}}) u = u^\mathrm{{q}}\) has a positive k-peaks solution which concentrate around \(x_0\), assuming that a constant \(\beta \) is non-zero. In the equation \(c_N = \frac{N-2}{4(N-1)}\) for an integer \(N>n\) and \(q= \frac{N+2}{N-2}\). The constant \(\beta \) depends on n and N, and can be easily computed numerically, being negative in all cases considered. This provides solutions to the Yamabe equation on Riemannian products \((M\times X , g+ \epsilon ^2 h )\), where (X, h) is a Riemannian manifold with constant positive scalar curvature. We also prove that solutions with small energy only have one local maximum.
Similar content being viewed by others
References
Akutagawa, K., Florit, L., Petean, J.: On Yamabe constants of Riemannian products. Commun. Anal. Geom. 15, 947–969 (2007)
Aubin, T.: Equations differentielles non-lineaires et probleme de Yamabe concernant la courbure scalaire. J. Math. Pures Appl. 55, 269–296 (1976)
Bettiol, R., Piccione, P.: Bifurcation and local rigidity of homogeneous solutions to the Yamabe problem on spheres. Calc. Var. Partial Differ. Equ. 4(7), 789–807 (2013)
Bettiol, R., Piccione, P.: Multiplicity of solutions to the Yamabe problem on collapsing Riemannian submersions. Pac. J. Math. 266, 1–21 (2013)
Brendle, S.: Blow-up phenomena for the Yamabe problem. J. Am. Math. Soc. 15, 951–979 (2008)
Dancer, E.N., Micheletti, A.M., Pistoia, A.: Multipeak solutions for some singularly perturbed nonlinear elliptic problems on Riemannian manifolds. Manuscr. Math. 128, 163–193 (2009)
de Lima, L.L., Piccione, P., Zedda, M.: On bifurcation of solutions of the Yamabe problem in product manifolds. Ann. de L’inst. Henri Poincare (C) Non Linear Anal. 29, 261–277 (2012)
Deng, S., Musso, M., Pistoia, A.: Concentration on minimal submanifolds for a Yamabe-type problem. Commun. Partial Differ. Equ. 41(9), 1379–1425 (2016)
Deng, S., Khemiri, Z., Mahmoudi, F.: On spike solutions for a singularly perturbed problem in a compact Riemannian manifold. Commun. Pure Appl. Anal. 17(5), 2063–2084 (2018)
Floer, A., Weinstein, A.: Nonspreading wave packets for the cubic Schrodinger equation with a bounded potential. J. Funct. Anal. 69, 397–408 (1986)
Gilbarg, D., Trudinger, N.: Elliptic Partial Differential Equations of Second Order. Springer, Berlin (1983)
Gray, A.: The volume of a small geodesic ball of a Riemannian manifold. Michigan Math. J. 20(4), 329–344 (1974)
Henry, G., Petean, J.: Isoparametric hypersurfaces and metrics of constant scalar curvature. Asian J. Math. 18, 53–68 (2014)
Kwong, M.K.: Uniqueness os positive solutions of \(\Delta u -u +u^p =0\) in \({\mathbb{R}}^n\). Arch. Ration. Mech. Anal. 105, 243–266 (1989)
Li, Y.: On a singularly perturbed equation with Neumann boundary condition. Commun. Partial Differ. Equ. 23, 487–545 (1998)
Micheletti, A.M., Pistoia, A.: The role of the scalar curvature in a nonlinear elliptic problem on Riemannian manifolds. Calc. Var. 34, 233–265 (2009)
Micheletti, A.M., Pistoia, A.: Generic properties of critical points of the scalar curvature on a Riemannian manifold. Proc. Am. Math. Soc. 138, 3277–3284 (2010)
Otoba, N., Petean, J.: Solutions of the Yamabe equation on harmonic Riemannian submersions, arXiv:1611.06709
Parker, T.H., Lee, J.M.: The Yamabe Problem. Bull. Am. Math. Soc. 17(1), 37–91 (1987)
Petean, J.: Multiplicity results for the Yamabe equation by Lusternik-Schnirelmann theory. J. Funct. Anal. 276, 1788–1805 (2019)
Petean, J.: Metrics of constant scalar curvature conformal to Riemannian products. Proc. Am. Math. Soc. 138, 2897–2905 (2010)
Pollack, D.: Nonuniqueness and high energy solutions for a conformally invariant scalar equation. Commun. Anal. Geom. 1, 347–414 (1993)
Schoen, R.: Conformal deformation of a Riemannian metric to constant scalar curvature. J. Differ. Geom. 20, 479–495 (1984)
Schoen, R.: Variational theory for the total scalar curvature functional for Riemannian metrics and related topics. Lecture Notes in Math, vol. 1365. Springer-Verlag, Berlin (1989)
Trudinger, N.: Remarks concerning the conformal deformation of Riemannian structures on compact manifolds. Ann. Scuola Norm. Sup. Pisa 22(3), 265–274 (1968)
Yamabe, H.: On a deformation of Riemannian structures on compact manifolds. Osaka Math. J. 12, 21–37 (1960)
Acknowledgements
The authors would like to thank Prof. Jimmy Petean for many helpful discussions on the subject. The second author was supported by program UNAM-DGAPA-PAPIIT IA106918.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix
Appendix
In this section we compute numerically \(\beta \) of Sect. 3, for low dimensions. Namely
which plays an important role in the asymptotic expansion of the energy \(\overline{J_{\epsilon }}\).
\(\beta \) is a dimensional constant that requires knowledge of the unique (up to translations) positive solution \(U \in H^1(\mathbb {R}^n)\) that vanishes at infinity of
with \(p=\frac{2(m+n)}{m+n-2}\). The solution is known to exist, and to be unique and radial, see [14] for details.
Hence, we consider the solution \(h=h_{\alpha }\) of
with \(h(0)=\alpha >0\), \(h'(0)=0\). By the aforementioned existence and uniqueness results, there exists only one value \(\alpha =\alpha _0=\alpha _0(m,n)\) that gives a positive solution \(h_{\alpha _0}\) that vanishes at infinity. Our approach is to find \(h_{\alpha _0}\) numerically as the solution of (110) that vanishes at infinity, and then to integrate it numerically to find \(V_{n-1} \ \mathbf{c} \int _{0}^{\infty } u^2 r^{n-1}dr\) and \(\frac{V_{n-1}}{n(n+2)} \int _{0}^{\infty } u'^2 r^{n+1} dr \), the two terms involved in (108). Of course \(u(r)=h_{\alpha _0}(r)\).
In Table 1 we show the numerical results, where \(\beta \) is negative for \(m+n\le 9\).
Rights and permissions
About this article
Cite this article
Rey, C.A., Ruiz, J.M. Multipeak Solutions for the Yamabe Equation. J Geom Anal 31, 1180–1222 (2021). https://doi.org/10.1007/s12220-019-00258-4
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s12220-019-00258-4