Skip to main content
SpringerLink
Log in

Metrological performance investigation of swirl flowmeter affected by vortex inflow

  • Published:
Journal of Mechanical Science and Technology Aims and scope Submit manuscript

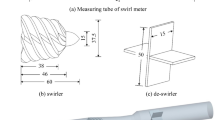
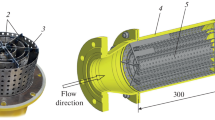
Abstract

In fluid transportation industries, long straight transition pipelines upstream of flowmeters are required to eliminate the influence of disturbed inflow on flow metering accuracy. To ensure an accurate flow measurement, the straight transition pipeline, which is often lengthy, can be shortened, particularly when the installation space is limited. However, the characteristics of disturbed flow (mainly characterized by vortex inflow) evolving along the straight transition pipeline to affect the metrological performance must be investigated for optimizing the pipeline length. The objective of this study was to investigate the metrological performance of a swirl flowmeter affected by the vortex inflow caused by flow regulation with a sleeve valve. The effect of a straight transition pipeline length upstream of a swirl flowmeter on the evolving characteristics of the vortex inflow was investigated experimentally and by conducting a numerical simulation under flow regulation parameters, including valve openings and flow velocities. The flow coefficients for different straight transition pipeline lengths were investigated to verify the flow simulation. The periodic pressure variation in the swirl flowmeter was monitored and its corresponding characteristic frequency was analyzed. It was found that the metrological characteristics of the swirl flowmeter were extremely affected by a smaller valve opening, which can be improved by lengthening the straight transition pipeline. By analyzing the velocity vector and vorticity in the internal flow field, the vortex inflow was found to be vulnerable to dissipation within a longer straight transition pipeline. The average vorticity at the cross-section of the entrance to the swirl flowmeter was adopted to evaluate the inflow vortex intensity. Additionally, a power curve model was established to assess the critical characteristic frequency determined by the maximum unaffected inflow vorticity for different straight transition pipeline lengths. This study can provide helpful insights to the metrological performance and pipeline arrangement in the field of fluid transportation engineering.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. R. Steven and A. Hall, Orifice plate meter wet gas flow performance, Flow Measurement and Instrumentation, 20 (4–5) (2009) 141–151.

    Article  Google Scholar 

  2. R. S. Ettouney and M. A. El-Rifai, Sensitivity of orifice meter gas flow computations, Journal of Petroleum Science and Engineering, 80 (1) (2012) 102–106.

    Article  Google Scholar 

  3. A. Venugopal, A. Agrawal and S. V. Prabhu, Review on vortex flowmeter-Designer perspective, Sensors and Actuators A: Physical, 170 (2011) 8–23.

    Article  Google Scholar 

  4. D. He and B. Bai, Numerical investigation of wet gas flow in Venturi meter, Flow Measurement and Instrumentation, 28 (2012) 1–6.

    Article  Google Scholar 

  5. P. Gajan, Q. Decaudin and J. P. Couput, Analysis of high pressure tests on wet gas flow metering with a venturi meter, Flow Measurement and Instrumentation, 44 (2015) 126–131.

    Article  Google Scholar 

  6. L. Qin, L. Hu, K. Mao, W. Chen and X. Fu, Application of extreme learning machine to gas flow measurement with multipath acoustic transducers, Flow Measurement and Instrumentation, 49 (2016) 31–39.

    Article  Google Scholar 

  7. L. Qin, L. Hu, K. Mao, W. Chen and X. Fu, Flow profile identification with multipath transducers, Flow Measurement and Instrumentation, 52 (2016) 148–156.

    Article  Google Scholar 

  8. J. Peng, X. Fu and Y. Chen, Response of a swirl flowmeter to oscillatory flow, Flow Measurement and Instrumentation, 19 (2) (2008) 107–115.

    Article  Google Scholar 

  9. A. Ukil and A. Andenna, Harmonic analysis-based diagnostics of noflow pulsation in vortex and swirl flowmeter, Digital Signal Processing, 19 (4) (2009) 700–707.

    Article  Google Scholar 

  10. C. Hua and Y. Geng, Investigation on the swirl flowmeter performance in low pressure wet gas flow, Measurement, 44 (5) (2011) 881–887.

    Article  Google Scholar 

  11. C. Hua and Y. Geng, Wet gas meter based on the vortex precession frequency and differential pressure combination of swirl flowmeter, Measurement, 45 (4) (2012) 763–768.

    Article  Google Scholar 

  12. D. Chen, B. Cui and Z. Zhu, Research on internal flow and performance of swirl flowmeter with different swirler cone angle, Measurement & Control, 49 (4) (2016) 136–142.

    Article  Google Scholar 

  13. D. Chen, B. Cui and Z. Zhu, Numerical simulations for swirl flowmeter on flow fields and metrological performance, Transactions of the Institute of Measurement and Control, 40 (4) (2018) 1072–1081.

    Article  Google Scholar 

  14. X. Li, P. Gao, Z. Zhu and Y. Li, Effect of the blade loading distribution on hydrodynamic performance of a centrifugal pump with cylindrical blades, Journal of Mechanical Science and Technology, 32 (3) (2018) 1161–1170.

    Article  Google Scholar 

  15. Y. Li, G. Feng, X. Li, Q. Si and Z. Zhu, An experimental study on the cavitation vibration characteristics of a centrifugal pump at normal flow rate, Journal of Mechanical Science and Technology, 32 (10) (2018) 4711–4720.

    Article  Google Scholar 

  16. S. Zhang, X. Li, B. Hu, Y. Liu and Z. Zhu, Numerical investigation of attached cavitating flow in thermo-sensitive fluid with special emphasis on thermal effect and shedding dynamics, International Journal of Hydrogen Energy, 44 (5) (2019) 3170–3184.

    Article  Google Scholar 

  17. M.-J. Chern, C.-C. Wang and C.-H. Ma, Performance test and flow visualization of ball valve, Experimental Thermal and Fluid Science, 31 (6) (2007) 505–512.

    Article  Google Scholar 

  18. B. Cui, Z. Lin, Z. Zhu, H. Wang and G. Ma, Influence of opening and closing process of ball valve on external performance and internal flow characteristics, Experimental Thermal and Fluid Science, 80 (2017) 193–202.

    Article  Google Scholar 

  19. Z. Leutwyler and C. Dalton, A CFD study of the flow field, resultant force, and aerodynamic torque on a symmetric disk butterfly valve in a compressible fluid, Journal of Pressure Vessel Technology, 130 (2) (2008) 021302.

    Google Scholar 

  20. L. Wang, X. G. Song and Y. C. Park, Dynamic analysis of three-dimensional flow in the opening process of a singledisc butterfly valve, Proceedings of the Institution of Mechanical Engineers, Part C: Journal of Mechanical Engineering Science, 224 (2) (2010) 329–336.

    Article  Google Scholar 

  21. H. Chattopadhyay, A. Kundu, B. K. Saha and T. Gangopadhyay, Analysis of flow structure inside a spool type pressure regulating valve, Energy Conversion and Management, 53 (1) (2012) 196–204.

    Article  Google Scholar 

  22. B. K. Saha, H. Chattopadhyay, P. B. Mandal and T. Gangopadhyay, Dynamic simulation of a pressure regulating and shut-off valve, Computers & Fluids, 101 (2014) 233–240.

    Article  Google Scholar 

  23. Z. Lin, G. Ma, B. Cui, Y. Li, Z. Zhu and N. Tong, Influence of flashboard location on flow resistance properties and internal features of gate valve under the variable condition, Journal of Natural Gas Science and Engineering, 33 (2016) 108–117.

    Article  Google Scholar 

  24. Z. Lin, C. Ma, H. Xu, X. Li, B. Cui and Z. Zhu, Numerical and experimental studies on hydrodynamic characteristics of sleeve regulating valves, Flow Measurement and Instrumentation, 53 (2017) 279–285.

    Article  Google Scholar 

  25. S. Chun, B.-R. Yoon and K.-B. Lee, Diagnostic flow metering using ultrasound tomography, Journal of Mechanical Science and Technology, 25 (6) (2011) 1475–1482.

    Article  Google Scholar 

  26. S. Chun, B.-R. Yoon, D.-K. Lee and H.-M. Choi, Correction of flow metering coefficients by using multidimensional non-linear curve fitting, Journal of Mechanical Science and Technology, 26 (1) (2012) 3479–3489.

    Article  Google Scholar 

  27. A. Venugopal, A. Agrawal and S. V. Prabhu, Frequency detection in vortex flowmeter for low Reynolds number using piezoelectric sensor and installation effects, Sensors and Actuators A: Physical, 184 (2012) 78–85.

    Article  Google Scholar 

  28. A. Venugopal, A. Agrawal and S. V. Prabhu, Performance evaluation of piezoelectric and differential pressure sensor for vortex flowmeters, Measurement, 50 (2014) 10–18.

    Article  Google Scholar 

  29. G. Haller, A. Hadjighasem, M. Farazmand and F. Huhn, Defining coherent vortices objectively from the vorticity, Journal of Fluid Mechanics, 795 (2016) 136–173.

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgments

This work is supported by the National Natural Science Foundation of China (No. 51876193 and No. 51806196), Natural Science Foundation of Zhejiang Province (No. LQ17A02 0002), the Young Researchers Foundation of Zhejiang Provincial Top Key Academic Discipline of Mechanical Engineering of Zhejiang Sci-Tech University (No. ZSTUME02B03).

Author information

Authors and Affiliations

  1. Key Laboratory of Fluid Transmission Technology of Zhejiang Province, Zhejiang Sci-Tech University, Hangzhou, 310018, China

    Qi Liu, Jia-hui Ye, Guang Zhang, Zhe Lin, Hong-guang Xu & Zu-chao Zhu

  2. National-Provincial Joint Engineering Laboratory for Fluid Transmission System Technology, Zhejiang Sci-Tech University, Hangzhou, 310018, China

    Qi Liu, Zhe Lin & Zu-chao Zhu

Authors
  1. Qi Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jia-hui Ye
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Guang Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Zhe Lin
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Hong-guang Xu
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Zu-chao Zhu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Zhe Lin.

Additional information

Recommended by Associate Editor Gyuhae Park

Qi Liu received his B.S. from Zhejiang University of Technology, China, in 2008. He received his Ph.D. from Zhejiang University, China, in 2015. Currently, he is a lecturer in Zhejiang Sci-Tech University, China. His research interests include internal flow stability in pipeline and optimal design in fluid machinery.

Zhe Lin received his B.S. from Zhejiang Sci-Tech University, China, in 2008. He received his Ph.D. from Zhejiang University, China, in 2013. Currently, he is an Associate Professor in Zhejiang Sci-Tech University, China. His research interests include multiphase flow, flow wear, numerical simulation and experimental measurement in fluid machinery.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Liu, Q., Ye, Jh., Zhang, G. et al. Metrological performance investigation of swirl flowmeter affected by vortex inflow. J Mech Sci Technol 33, 2671–2680 (2019). https://doi.org/10.1007/s12206-019-0515-7

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12206-019-0515-7

Keywords

Search

Navigation