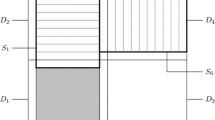
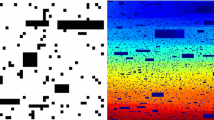
Abstract
Bootstrap percolation transition may be first order or second order, or it may have a mixed character where a first-order drop in the order parameter is preceded by critical fluctuations. Recent studies have indicated that the mixed transition is characterized by power-law avalanches, while the continuous transition is characterized by truncated avalanches in a related sequential bootstrap process. We explain this behaviour on the basis of an analytical and numerical study of the avalanche distributions on a Bethe lattice.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
J Chalupa, P L Leath and G R Reich, J. Phys. C12, L31 (1979)
M E Fisher and J W Essam, J. Math. Phys. 2, 609 (1961)
S S Manna, Physica A261, 351 (1998)
C Farrow, P M Duxbury and C F Moukarzel, Phys. Rev. E72, 066109 (2005)
C L Farrow, P Shukla and P M Duxbury, J. Phys. A40, F581 (2007)
G Parisi and T Rizzo, cond-mat 609777 (2006)
D Dhar, P Shukla and J P Sethna, J. Phys. A30, 5259 (1977)
S Sabhapandit, P Shukla and D Dhar, J. Stat. Phys. 98, 103 (2000)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Shukla, P. Dynamics of bootstrap percolation. Pramana - J Phys 71, 319–329 (2008). https://doi.org/10.1007/s12043-008-0165-2
Published:
Issue Date:
DOI: https://doi.org/10.1007/s12043-008-0165-2