Skip to main content
SpringerLink
Log in

Bayesian framework for satellite rechargeable lithium battery synthesizing bivariate degradation and lifetime data

卫星蓄电池二元性能退化和寿命数据的贝叶斯模型

  • Published:
Journal of Central South University Aims and scope Submit manuscript

Abstract

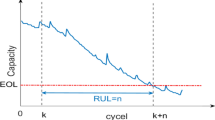
Reliability and remaining useful life (RUL) estimation for a satellite rechargeable lithium battery (RLB) are significant for prognostic and health management (PHM). A novel Bayesian framework is proposed to do reliability analysis by synthesizing multisource data, including bivariate degradation data and lifetime data. Bivariate degradation means that there are two degraded performance characteristics leading to the failure of the system. First, linear Wiener process and Frank Copula function are used to model the dependent degradation processes of the RLB’s temperature and discharge voltage. Next, the Bayesian method, in combination with Markov Chain Monte Carlo (MCMC) simulations, is provided to integrate limited bivariate degradation data with other congeneric RLBs’ lifetime data. Then reliability evaluation and RUL prediction are carried out for PHM. A simulation study demonstrates that due to the data fusion, parameter estimations and predicted RUL obtained from our model are more precise than models only using degradation data or ignoring the dependency of different degradation processes. Finally, a practical case study of a satellite RLB verifies the usability of the model.

摘要

卫星蓄电池剩余剩余寿命预测是卫星系统故障诊断与健康管理的重要一环。 本文创新性的提出了一个融合二元性能退化数据和寿命数据的可靠性分析贝叶斯模型, 对卫星锂电池的剩余寿命进行预测。 二元性能退化是指系统存在两个相关的性能退化现象, 二者的共同作用下可能导致系统故障。 模型中首先利用 Copula 函数和线性维纳过程对锂电池的二元性能退化数据进行建模。 而后, 利用贝叶斯模型融合小子样寿命数据, 采用马尔科夫蒙特卡洛仿真估计模型参数, 从而对剩余寿命进行预测, 并通过仿真和实例分析对所提模型的性能进行分析。 结果表明融合了二元性能退化数据和寿命数据后, 剩余寿命的预测精度能够有效提高。

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Similar content being viewed by others

References

  1. GAO Li-jun, LIU Sheng-yi, DOUGAL R A. Dynamic lithium-ion battery model for system simulation [J]. IEEE Transactions on Components and Packaging Technologies, 2002, 25(3): 495–505.

    Article  Google Scholar 

  2. ERDINC O, VURAL B, UZUNOGLU M. A dynamic lithium-ion battery model considering the effects of temperature and capacity fading [C]//International Conference on Clean Electrical Power. Capri, Italy: IEEE, 2009: 383–386.

    Google Scholar 

  3. JIN Guang, MATTHEWS D E, ZHOU Zhong-bao. A Bayesian framework for on-line degradation assessment and residual life prediction of secondary batteries in spacecraft [J]. Reliability Engineering & System Safety, 2013, 113: 7–20.

    Article  Google Scholar 

  4. PATEL M R. Spacecraft power systems [M]. Boca Raton: CRC Press, 2005.

    Google Scholar 

  5. SI Xiao-sheng. An adaptive prognostic approach via nonlinear degradation modelling: Application to battery data [J]. IEEE Transactions on Industrial Electronics, 2014, 62(8): 5082–5096.

    Article  Google Scholar 

  6. TANG Sheng-jin, YU Chuan-qiang, WANG Xue, et al. Remaining useful life prediction of lithium-ion batteries based on the Wiener process with measurement error [J]. Energies, 2014, 7(2): 520–547.

    Article  Google Scholar 

  7. HU Chao, JAIN G, TAMIRISA P, et al. Method for estimating capacity and predicting remaining useful life of lithium-ion battery [J]. Applied Energy, 2014, 126: 182–189.

    Article  Google Scholar 

  8. SONG L, EVANS J W. Electrochemical-thermal model of lithium polymer batteries [J]. Journal of the Electrochemical Society, 2000, 147(6): 2086–2095.

    Article  Google Scholar 

  9. GU W B, WANG C Y. Thermal-electrochemical modeling of battery systems [J]. Journal of the Electrochemical Society, 2000, 147(8): 2910–2922.

    Article  Google Scholar 

  10. LIAW B Y, JUNGST R G, NAGASUBRAMANIAN G, et al. Modeling capacity fade in lithium-ion cells [J]. Journal of Power Sources, 2005, 140(1): 157–161.

    Article  Google Scholar 

  11. SHIM J, KOSTECKI R, RICHARDSON T, et al. Electrochemical analysis for cycle performance and capacity fading of a lithium-ion battery cycled at elevated temperature [J]. Journal of Power Sources, 2002, 112(1): 222–230.

    Article  Google Scholar 

  12. SPOTNITZ R. Simulation of capacity fade in lithium-ion batteries [J]. Journal of Power Sources, 2003, 113(1): 72–80.

    Article  Google Scholar 

  13. LIU Da-tong, WANG Hong, PENG Yu, et al. Satellite lithium-ion battery remaining cycle life prediction with novel indirect health indicator extraction [J]. Energies, 2013, 6(8): 3654–3668.

    Article  Google Scholar 

  14. VETTER J, NOV K P, WAGNER M R, et al. Ageing mechanisms in lithium-ion batteries [J]. Journal of Power Sources, 2005, 147(1): 269–281.

    Article  Google Scholar 

  15. WANG Xiao-lin, GUO Bo, CHENG Zhi-jun. Residual life estimation based on bivariate Wiener degradation process with time-scale transformations [J]. Journal of Statistical Computation and Simulation, 2012, 84(3): 545–563.

    Article  MathSciNet  Google Scholar 

  16. CHEN S C, WAN C C, WANG Y Y. Thermal analysis of lithium-ion batteries [J]. Journal of Power Sources, 2005, 140(1): 11–24.

    Article  Google Scholar 

  17. ZHANG Jing-liang, LEE J. A review on prognostics and health monitoring of Li-ion battery [J]. Journal of Power Sources, 2011, 196(15): 6007–6014.

    Article  Google Scholar 

  18. CHOI Young-jin, WANG Jin-suk. Stability analysis of a voltage-temperature (V/T) limit circuit for satellite power system [C]//Proceedings of the Energy Conversion Engineering Conference (IECEC 1996). Washington, DC, USA: IEEE, 1996, 1: 316–321.

    Google Scholar 

  19. COX D R, MILLER H D. The theory of stochastic process [M]. London: Chapman and Hall, 1965.

    MATH  Google Scholar 

  20. PAN Zheng-qiang, BALAKRISHNAN N, SUN Q, et al. Bivariate degradation analysis of products based on Wiener processes and copulas [J]. Journal of Statistical Computation and Simulation, 2013, 83(7): 1316–1329.

    Article  MathSciNet  Google Scholar 

  21. WANG Xiao-lin, GUO Bo, CHENG Zhi-jun, et al. Residual life estimation based on bivariate Wiener degradation process with measurement errors [J]. Journal of Central South University, 2013, 20(1): 844–851.

    Google Scholar 

  22. SAHA B, GOEBEL K, POLL S, et al. Prognostics methods for battery health monitoring using a Bayesian framework [J]. IEEE Transactions on Instrumentation and Measurement, 2009, 58(2): 291–296.

    Article  Google Scholar 

  23. HE Wei, WILLIARD N, OSTERMAN M, et al. Prognostics of lithium-ion batteries based on Dempster–Shafer theory and the Bayesian Monte Carlo method [J]. Journal of Power Sources, 2011, 196(23): 10314–10321.

    Article  Google Scholar 

  24. WANG Li-zhi, PAN Rong, LI Xiao-yang, et al. A Bayesian reliability evaluation method with integrated accelerated degradation testing and field information [J]. Reliability Engineering & System Safety, 2013, 112: 38–47.

    Article  Google Scholar 

  25. LEHMANN A. Joint modeling of degradation and failure time data [J]. Journal of Statistical Planning and Inference, 2009, 139(5): 1693–1706.

    Article  MathSciNet  MATH  Google Scholar 

  26. GUO Ji-qiang, WILSON A G. Bayesian methods for estimating system reliability using heterogeneous multilevel information [J]. Technometrics, 2013, 55(4): 461–472.

    Article  MathSciNet  Google Scholar 

  27. NELSEN R B. An introduction to copulas [M]. Second ed. New York: Springer Science+Business Media, Inc, 2006.

    MATH  Google Scholar 

  28. PENG Wei-wen, HUANG Hong-zhong, LI Yan-feng, et al. Life cycle reliability assessment of new products—A Bayesian model updating approach [J]. Reliability Engineering & System Safety, 2013, 112(10): 9–19.

    Google Scholar 

  29. SKLAR A. Random variables, joint distributions, and copulas [J]. Kybernetica, 1973, 9(6): 449–460.

    MathSciNet  MATH  Google Scholar 

  30. SAHA B, GOEBEL K. Modeling Li-ion battery capacity depletion in a particle filtering framework [C]//The Annual Conference of the Prognostics and Health Management Society. San Diego, CA: PHMsociety, 2009: 2909–2924.

    Google Scholar 

  31. PENG C Y, TSENG S T. Mis-specification analysis of linear prediction models [J]. IEEE Transactions on Reliability, 2009, 58(3): 444–455.

    Article  Google Scholar 

  32. NTZOUFRAS I. Bayesian modeling using WinBUGS [M]. Canada: John Wiley & Sons, Inc, 2009.

    Book  MATH  Google Scholar 

  33. PENG Wei-wen, LIU Yu, LI Yan-feng, et al. A Bayesian optimal design for degradation tests based on the inverse Gaussian process [J]. Journal of Mechanical Science and Technology, 2014, 28(10): 3937–3946.

    Article  Google Scholar 

  34. WANG Zhong-lai, HUANG Hong-zhong, DU Li. Reliability analysis on competitive failure processes under fuzzy degradation data [J]. Applied Soft Computing, 2011, 11(3): 2964–2973.

    Article  Google Scholar 

  35. YUAN T, JI Y. A Hierarchical bayesian degradation model for heterogeneous data [J]. IEEE Transactions on Reliability, 2015, 64(1): 63–70.

    Article  Google Scholar 

  36. PAN Zheng-qiang, BALAKRISHNAN N, SUN Quan. Bivariate constant-stress accelerated degradation model and inference [J]. Communications in Statistics-Simulation and Computation, 2010, 40(2): 247–257.

    Article  MathSciNet  MATH  Google Scholar 

  37. SPIEGELHALTER D J, BEST N G, CARLIN B P, et al. Bayesian measures of model complexity and fit [J]. Journal of the Royal Statistical Society, 2002, 64(4): 583–639.

    Article  MathSciNet  MATH  Google Scholar 

  38. SPIEGELHALTER D J, BEST N G, CARLIN B P, et al. The deviance information criterion: 12 years on [J]. Journal of the Royal Statistical Society: Series B, 2014, 76(3): 485–493.

    Article  MathSciNet  Google Scholar 

  39. HAO Hui-bing, SU Chun. A Bayesian framework for reliability assessment via wiener process and MCMC [J]. Mathematical Problems in Engineering, 2014, 2014 Article ID 486368. DOI: 101155120141486368.

    Google Scholar 

  40. SANTOS C A, ACHCAR J A. A Bayesian analysis in the presence of covariates for multivariate survival data: An example of application [J]. Revista Colombinana De Estadistica, 2011, 34(1): 111–131.

    MathSciNet  Google Scholar 

  41. TSAI C C, TSENG S T, BALAKRISHNAN N. Mis-specification analyses of gamma and Wiener degradation processes [J]. Journal of Statistical Planning and Inference, 2011, 141(12): 3725–3735.

    Article  MathSciNet  MATH  Google Scholar 

  42. MASSEY J R, FRANK J. The Kolmogorov-Smirnov test for goodness of fit [J]. Journal of the American Statistical Association, 1951, 46(253): 68–78.

    Article  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. College of Information Systems and Management, National University of Defense Technology, Changsha, 410073, China

    Yang Zhang  (张洋), Xiang Jia  (贾祥) & Bo Guo  (郭波)

Authors
  1. Yang Zhang  (张洋)
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Xiang Jia  (贾祥)
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Bo Guo  (郭波)
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yang Zhang  (张洋).

Additional information

Foundation item: Project(71371182) supported by the National Natural Science Foundation of China

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhang, Y., Jia, X. & Guo, B. Bayesian framework for satellite rechargeable lithium battery synthesizing bivariate degradation and lifetime data. J. Cent. South Univ. 25, 418–431 (2018). https://doi.org/10.1007/s11771-018-3747-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11771-018-3747-2

Key words

关键词

Search

Navigation