Skip to main content
SpringerLink
Log in

A theoretical model for the estimation of maximum impact force from a rockfall based on contact theory

  • Published:
Journal of Mountain Science Aims and scope Submit manuscript

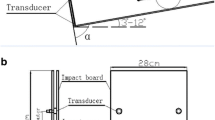
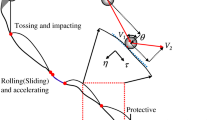
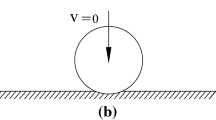
Abstract

Rockfall poses a great threat to buildings and personal security. To understand the dynamic characteristics of rockfalls is a prerequisite for disaster prevention and assessment. Models for rockfalls in different forms are established based on the theory of rigid body motion. The equivalent velocity considering the rotational effect is determined by the energy ratio. Besides, considering plastic deformation and nonlinear hardening, the maximum impact force is estimated based on the Hertz contact theory. Then, a case study is carried out to illustrate the applicability of the model and sensitive analyses on some affecting parameters are also made. Calculation results show that the maximum impact force increases with the increasing of incident velocity, angle and slope gradient reflected by the changing of energy ratio. Moreover, the model for the estimation of maximum impact force is validated by two different scales of experiments and compared with other theoretical models. Simulated maximum impact forces agree well with the experiments.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Abbreviations

v x :

Initial horizontal velocity (m/s)

v y :

Initial vertical velocity (m/s)

v :

Final velocity (m/s)

g :

Gravitational acceleration (m2/s)

H :

Vertical falling height (m)

L :

Horizontal distance during freefall (m)

e n :

Normal coefficient of restitution

e τ :

Tangential coefficient of restitution

v rn :

Normal rebound velocity (m/s)

v in :

Normal incident velocity (m/s)

v rτ :

Tangential rebound velocity (m/s)

v iτ :

Tangential incident velocity (m/s)

v 0 :

Initial velocity (m/s)

α:

Slope gradient (°)

f 1 :

Rolling friction coefficient

R 1 :

Radius of the rockfall (m)

ß :

Energy ratio

E r :

Rotational energy (J)

E v :

Translational energy (J)

E :

Total energy (J)

m :

Quality of rockfall (kg)

v n :

Normal velocity (m/s)

v τ :

Tangential velocity (m/s)

v n ':

Equivalent normal velocity (m/s)

v τ ':

Equivalent tangential velocity (m/s)

θ :

Incident angle (°)

σ (r) :

Contact stress on the contact surface (Pa)

P :

Normal impact force (N)

δ :

Maximum indentation depth (m)

References

  • ASTRA S (2008) Actions due to falling rocks on protective galleries. Guideline, Federal Roads Office, Building Directorate SBB, Swiss Federal Institute of Printing and Materials, Bern. (In Italian)

    Google Scholar 

  • Azzoni A, Barbera GL, Zaninetti A (1995) Analysis and prediction of rockfalls using a mathematical model. International Journal of Rock Mechanics and Mining Sciences & Geomechanics Abstracts 32(7): 709–724. https://doi.org/10.1016/0148-9062(95)00018-C

    Article  Google Scholar 

  • Bozzolo D, Pamini R (1986) Simulation of rock falls down a valley side. Acta Mech 63(1): 113–130. https://doi.org/10.1007/BF01182543

    Article  Google Scholar 

  • Brizmer V, Kligerman Y, Etsion I (2006) The effect of contact conditions and material properties on the elasticity terminus of a spherical contact. International Journal of Solids and Structures 43(18–19): 5736–5749. https://doi.org/10.1016/j.ijsolstr.2005.07.034

    Article  Google Scholar 

  • Buzzi O, Spadari M, Giacomini A, et al. (2013) Experimental testing of rockfall barriers designed for the low range of impact energy. Rock Mechanics and Rock Engineering 46(4): 701–712. https://doi.org/10.1007/s00603-012-0295-1

    Article  Google Scholar 

  • Calvetti F, Di Prisco C, Vecchiotti M (2005) Experimental and numerical study of rock-fall impacts on granular soils. Rivista Italiana Di Geotecnica 4: 95–109.

    Google Scholar 

  • Chau KT, Wong RHC, Wu JJ (2002) Coefficient of restitution and rotational motions of rockfall impacts. International Journal of Rock Mechanics and Mining Sciences 39(1): 69–77. https://doi.org/10.1016/S1365-1609(02)00016-3

    Article  Google Scholar 

  • Cui YF, Nouri A, Chan D, Rahmati E (2016) A new approach to DEM simulation of sand production. Journal of Petroleum Science and Engineering 147: 56–67. https://doi.org/10.1016/j.petrol.2016.05.007

    Article  Google Scholar 

  • Cui YF, Chan D, Nouri A (2017) Discontinuum modeling of solid deformation pore-water diffusion coupling. International Journal of Geomechanics 17: 04017033. https://doi.org/10.1061/(ASCE)GM.1943-5622.0000903

    Article  Google Scholar 

  • Delhomme F, Mommessin M, Mougin JP, et al. (2007) Simulation of a block impacting a reinforced concrete slab with a finite element model and a mass-spring system. Engineering Structures 29: 2844–2852. https://doi.org/10.1016/j.engstruct.2007.01.017

    Article  Google Scholar 

  • Dorren LKA (2003) A review of rockfall mechanics and modelling approaches. Progress in Physical Geography 27(1): 69–87. https://doi.org/10.1191/0309133303pp359ra

    Article  Google Scholar 

  • Dorren LKA, Berger F, Putters US (2006) Real-size experiments and 3-D simulation of rockfall on forested and non-forested slopes. Natural Hazards and Earth System Sciences 6(1): 145–153. https://doi.org/10.5194/nhess-6-145-2006

    Article  Google Scholar 

  • Genis M, Sakız U, Aydıner BC (2017) A stability assessment of the rockfall problem around the Gökgöl Tunnel (Zonguldak, Turkey). Bulletin of Engineering Geology and the Environment 76(4): 1237–1248. https://doi.org/10.1007/s10064

    Article  Google Scholar 

  • Gentilini C, Govoni L, de Miranda S, et al. (2012) Threedimensional numerical modelling of falling rock protection barriers. Computers and Geotechnics 44: 58–72. https://doi.org/10.1016/j.compgeo.2012.03.011

    Article  Google Scholar 

  • Giacomini A, Thoeni K, Lambert C, et al. (2012) Experimental study on rockfall drapery systems for open pit highwalls. International Journal of Rock Mechanics and Mining Sciences 56(12): 171–181. https://doi.org/10.1016/j.ijrmms.2012.07.030

    Article  Google Scholar 

  • Guzzetti F, Reichenbach P, Ghigi S (2004) Rockfall hazard and risk assessment along a transportation corridor in the Nera Valley, central Italy. Environmental Management, 34(2):191–208. https://doi.org/10.1007/s00267-003-0021-6

    Article  Google Scholar 

  • He S, Wu Y, Li X (2009) Research on restitution coefficient of rock fall. Rock and Soil Mechanics 30(3): 623–627 (In Chinese)

    Google Scholar 

  • Hertz H (1882) Über die Berührung fester elastischer Körper. Journal Für Die Reine Und Angewandte Mathematik 92: 156–171

    Google Scholar 

  • Hou TX, Xu Q, Zhou JW (2015) Size distribution, morphology and fractal characteristics of brittle rock fragmentations by the impact loading effect. Acta Mechanica 226: 3623–3637. https://doi.org/10.1007/s00707

    Article  Google Scholar 

  • Hou TX, Xu Q, Xie HQ, et al. (2017). An estimation model for the fragmentation properties of brittle rock block due to the impacts against an obstruction. Journal of Mountain Science 14: 1161–1173. https://doi.org/10.1007/s11629-017-4398-8

    Article  Google Scholar 

  • Hou TX, Yang XG, Huang C, et al. (2015) A calculation method based on impulse theorem to determine impact force of rockfall on structure. Journal of rock mechanics and Engineering 34: 3116–3122 (In Chinese)

    Google Scholar 

  • Huang RQ, Liu W (2009) In-situ test study of characteristics of rockfall rock blocks based on orthogonal design. Journal of rock mechanics and Engineering 28: 882–891. (In Chinese)

    Google Scholar 

  • Japan Road Association (JRA) (1983) Rockfall Handbook. Tokyo:Maruzen Publisher (Japan): 1–359.

    Google Scholar 

  • Kawahara S, Muro T (2006) Effects of dry density and thickness of sandy soil on impact response due to rockfall. Journal of Terramechanics 43 (3): 329–340. https://doi.org/10.1016/j.jterra.2005.05.009

    Article  Google Scholar 

  • Labiouse V, Descoeudres F, Montani S (1996) Experimental study of rock sheds impacted by rock blocks. Structural Engineering International 6 (3): 171–176

    Article  Google Scholar 

  • Mangwandi C, Cheong YS, Adams MJ, et al. (2007) The coefficient of restitution of different representative types of granules. Chemical Engineering Science 62(1–2): 437–450. https://doi.org/10.1016/j.ces.2006.08.063

    Article  Google Scholar 

  • Matsukura Y (2001) Rockfall at Toyohama Tunnel, Japan, in 1996: effect of notch growth on instability of a coastal cliff. Bulletin of Engineering Geology and the Environment 60(4): 285–289. https://doi.org/10.1007/s100640100123

    Article  Google Scholar 

  • Ministry of Transport of the People’s Republic of China (1995) Specifications for Design of Highway Subgrades (JTJ013-95). (In Chinese)

    Google Scholar 

  • Parise M (2002) Landslide hazard zonation of slopes susceptible to rock falls and topples. Natural Hazards and Earth System Sciences 2(1/2): 37–49. https://doi.org/10.5194/nhess-2-37-2002

    Article  Google Scholar 

  • Pichler B, Hellmich C, Mang HA (2005) Impact of rocks onto gravel Design and evaluation of experiments. International Journal of Impact Engineering 31(5): 559–578. https://doi.org/10.1016/j.ijimpeng.2004.01.007

    Article  Google Scholar 

  • Preh A, Mitchell A, Hungr O, et al. (2015) Stochastic analysis of rock fall dynamics on quarry slopes. International Journal of Rock Mechanics and Mining Sciences 80(8): 57–66. https://doi.org/10.1016/j.ijrmms.2015.09.010

    Article  Google Scholar 

  • Ritchie AM (1963) Evaluation of rockfall and its control. Journal of Experimental Psychology Applied 2(4): 291–304.

    Google Scholar 

  • Spadari M, Giacomini A, Buzzi O, et al. (2012) In situ rockfall testing in New South Wales, Australia. International Journal of Rock Mechanics and Mining Sciences 49: 84–93. https://doi.org/10.1016/j.ijrmms.2011.11.013

    Article  Google Scholar 

  • Thornton C (1997) Coefficient of restitution for collinear collisions of elastic-perfectly plastic spheres. Journal of Applied Mechanics 64(2): 383. https://doi.org/10.1115/1.2787319

    Article  Google Scholar 

  • Yuan JK, Li YR, Huang RQ, et al. (2015) Impact of rockfalls on protection measures: an experimental approach. Natural Hazards and Earth System Sciences 15: 885–893. https://doi.org/10.5194/nhessd-3-337-2015

    Article  Google Scholar 

  • Zhang G, Tang H, Xiang X, et al. (2015) Theoretical study of rockfall impacts based on logistic curves. International Journal of Rock Mechanics and Mining Science 78: 133–143. https://doi.org/10.1016/j.ijrmms.2015.06.001

    Article  Google Scholar 

  • Zhou JW, Jiao MY, Xing HG, et al. (2016) A reliability analysis method for rock slope controlled by weak structural surface. Geosciences Journal 21: 453–467. https://doi.org/10.1007/s12303-016-0058-1

    Article  Google Scholar 

Download references

Acknowledgement

This work was supported by the National Natural Science Foundation of China (41472272) and the Youth Science and Technology Fund of Sichuan Province (2016JQ0011). Critical comments by the anonymous reviewers greatly improved the initial manuscript.

Author information

Authors and Affiliations

  1. State Key Laboratory of Hydraulics and Mountain River Engineering, Sichuan University, Chengdu, 610065, China

    Shi-lin Zhang & Jia-wen Zhou

  2. College of Water Resource and Hydropower, Sichuan University, Chengdu, 610065, China

    Xing-guo Yang

Authors
  1. Shi-lin Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Xing-guo Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jia-wen Zhou
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jia-wen Zhou.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhang, Sl., Yang, Xg. & Zhou, Jw. A theoretical model for the estimation of maximum impact force from a rockfall based on contact theory. J. Mt. Sci. 15, 430–443 (2018). https://doi.org/10.1007/s11629-017-4606-6

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11629-017-4606-6

Keywords

Search

Navigation