Abstract
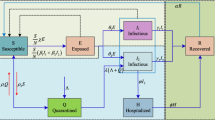
Infectious disease outbreaks occurred many times in the past and are more likely to happen in the future. In this paper the problem of allocating and scheduling limited multiple, identical or non-identical, resources employed in parallel, when there are several infected areas, is considered. A heuristic algorithm, based on Shih’s (1974) and Pappis and Rachaniotis’ (2010) algorithms, is proposed as the solution methodology. A numerical example implementing the proposed methodology in the context of a specific disease outbreak, namely influenza, is presented. The proposed methodology could be of significant value to those drafting contingency plans and healthcare policy agendas.
Article PDF
Similar content being viewed by others
References
Aleman, D. M., Wibisono, T. G. & Schwartz, B. (2011). A nonhomogeneous agent-based simulation approach to modeling the spread of disease in a pandemic outbreak. Interfaces, 41(3): 301–315.
Alexander, M. E., Moghadas, S. M., Röst, G. & Wu, J. (2008). A delay differential model for pandemic influenza with antiviral treatment. Bulletin of Mathematical Biology, 70(2): 382–397.
Boëlle, P. Y., Ansart, S., Cori, A. & Valleron, A. J. (2011). Transmission parameters of the A/H1N1 (2009) influenza virus pandemic: a review. Influenza and other Respiratory Viruses, 5(5): 306–316.
Brandeau, M. (2005). Allocating resources to control infectious diseases. Operations Research and Health Care, Vol. 70: 443–464.
Bretthauer, K. & Shetty, B. (1995). The nonlinear resource allocation problem. Operations Research, 43(4): 670–683.
Burke, D. S., Epstein, J. M., Cummings, D. A. T., Parker, J. I., Cline, K. C., Singa, R. M. & Chakravarty, S. (2006). Individual-based computational modeling of smallpox epidemic control strategies. Academic Emergency Medicine, 13(11): 1142–1149.
Carr, S. & Roberts, S. (2010). Planning for infectious disease outbreaks: a geographic disease spread, clinic location, and resource allocation simulation. Baltimore, MD, 2171–2184.
Carrat, F., Luong, J., Lao, H., Sallé, A. V., Lajaunie, C. & Wackernagel, H. (2006). A ‘small-world-like’ model for comparing interventions aimed at preventing and controlling influenza pandemics. BMC Medicine, 4(1):26.
Chowell, G., Ammon, C. E., Hengartner, N. W. & Hyman, J. M. (2006). Transmission dynamics of the great influenza pandemic of 1918 in Geneva, Switzerland: assessing the effects of hypothetical interventions. Journal of Theoretical Biology, 241(2): 193–204.
Chowell, G., Viboud, C., Wang, X., Bertozzi, S. M. & Miller, M. A. (2009). Adaptive vaccination strategies to mitigate pandemic influenza: Mexico as a case study. PLoS ONE, 4(12): e8164: 1-9.
Ciofi degli Atti, M. L., Merler, S., Rizzo, C., Ajelli, M., Massari, M., Manfredi, P., Furlanello, C., Tomba, G. S. & Iannelli, M. (2008). Mitigation measures for pandemic influenza in Italy: an individual based model considering different scenarios. PLoS ONE, 3(3):811–827.
Coburn, B. J., Wagner, B. G. & Blower, S. (2009). Modeling influenza epidemics and pandemics: insights into the future of swine flu (H1N1). BMC Medicine, 7.
Colizza, V., Barrat, A., Barthelemy, M., Valleron, A. J. & Vespignani, A. (2007). Modeling the worldwide spread of pandemic influenza: baseline case and containment interventions. PloS Medicine, 4(1): 0095–0110.
Cooper, B. S., Pitman, R. J., Edmunds, W. J. & Gay, N. J. 2006). Delaying the international spread of pandemic influenza. PLoS Medicine, 3(6): 0845–0855.
Cruz-Aponte, M., McKiernan, E. C. & Herrera-Valdez, M. A. (2011). Mitigating effects of vaccination on influenza outbreaks given constraints in stockpile size and daily administration capacity. BMC Infectious Diseases, 11.
Das, T. K., Savachkin, A. & Zhu, Y. (2008). A large-scale simulation model of pandemic influenza outbreaks for development of dynamic mitigation strategies. IIE Transactions (Institute of Industrial Engineers), 40(9): 893–905.
Department of Health. (2005). Smallpox mass vaccination -an operational planning framework. Department of Health, Scottish Government. Retrieved June 11, 2013 from http://www.scotland.gov.uk/Publications/2005/09/20160232/02428.
Epstein, J. M., Goedecke, D. M., Yu, F., Morris, R. J., Wagener, D. K. & Bobashev, G. V. (2007). Controlling pandemic flu: the value of international air travel restrictions. PLoS ONE, 2(5): e401:1-11.
Eubank, S., Guclu, H., Kumar, V. S. A., Marathe, M. V., Srinivasan, A., Toroczkai, Z. & Wang, N. (2004). Modelling disease outbreaks in realistic urban social networks. Nature, 429(6988): 180–184.
Ferguson, N., Keeling, M., Edmunds, W., Gani, R., Grenfell, B., Anderson, R. & Leach, S. (2003). Planning for smallpox outbreaks. Nature, 425(6959): 681–685.
Ferguson, N. M., Cummings, D. A. T., Cauchemez, S., Fraser, C., Riley, S., Meeyai, A., Iamsirithaworn, S. & Burke, D. S. (2005). Strategies for containing an emerging influenza pandemic in Southeast Asia. Nature, 437(7056): 209–214.
Flahault, A., Vergu, E., Coudeville, L. & Grais, R. F. (2006). Strategies for containing a global influenza pandemic. Vaccine, 24(44-46): 6751–6755.
Glasser, J., Taneri, D., Feng, Z., Chuang, J. H., Tüll, P., Thompson, W., McCauley, M. M. & Alexander, J. (2010). Evaluation of targeted influenza vaccination strategies via population modeling. PLoS ONE, 5(9): 1–8.
Hall, I. M., Egan, J. R., Barrass, I., Gani, R. & Leach, S. (2007). Comparison of smallpox outbreak control strategies using a spatial metapopulation model. Epidemiology and Infection, 135(7): 1133–1144.
Hansen, E. & Day, T. (2011). Optimal control of epidemics with limited resources. Journal of Mathematical Biology, 62(3): 423–451.
Hethcote, H. (2000). The mathematics of infectious diseases. SIAM Review, 42(4): 599–653.
Hollingsworth, T. D., Klinkenberg, D., Heesterbeek, H. & Anderson, R. M. (2011). Mitigation strategies for pandemic influenza a: balancing conflicting policy objectives. PLoS Computational Biology, 7(2): e1001076: 1-11.
Hupert, N., Cuomo, J., Callahan, M., Mushlin, A. & Morse, S. (2004). Community-Based Mass Prophylaxis: A Planning Guide for Public Health Preparedness. Prepared by Weill Medical College of Cornell University, Department of Public Health under Contract No. 290-02-0013-3. AHRQ Pub No. 04-0044. Rockville, MD: Agency for Healthcare Research and Quality. Retrieved June 08, 2010 from http://archive.ahrq.gov/downloads/pub/biotertools/cbmprophyl.pdf.
Ibaraki, T. & Katoh, N. (1988). Resource Allocation Problems: Algorithmic Approaches. The MIT Press.
Kaplan, E. H., Craft, D. L. & Wein, L. M. (2002). Emergency response to a smallpox attack: the case for mass vaccination. Proceedings of the National Academy of Sciences of the United States of America, 99(16): 10935–10940.
Kaplan, E. H., Craft, D. L. & Wein, L. M. (2003). Analyzing bioterror response logistics: the case of smallpox. Mathematical Biosciences, 185(1): 33–72.
Koyuncu, M. & Erol, R. (2010). Optimal resource allocation model to mitigate the impact of pandemic influenza: a case study for Turkey. Journal of Medical Systems, 34(1): 61–70.
Krumkamp, R., Kretzschmar, M., Rudge, J. W., Ahmad, A., Hanvoravongchai, P., Westenhoefer, J., Stein, M., Putthasri, W. & Coker, R. (2011). Health service resource needs for pandemic influenza in developing countries: a linked transmission dynamics, interventions and resource demand model. Epidemiology and Infection, 139(1): 59–67.
Lee, B. Y., Brown, S. T., Korch, G. W., Cooley, P. C., Zimmerman, R. K., Wheaton, W. D., Zimmer, S. M., Grefenstette, J. J., Bailey, R. R., Assi, T. M. & Burke, D. S. (2010). A computer simulation of vaccine prioritization, allocation, and rationing during the 2009 H1N1 influenza pandemic. Vaccine, 28(31): 4875–4879.
Lee, S., Golinski, M. & Chowell, G. (2012). Modeling optimal age-specific vaccination strategies against pandemic influenza. Bulletin of Mathematical Biology, 74(4): 958–980.
Matrajt, L., Halloran, M. E. & Longini, I. M. (2013). Optimal vaccine allocation for the early mitigation of pandemic influenza. PLoS Computational Biology, 9(3): e1002964: 1-15.
Matrajt, L. & Longini, I. M. (2010). Optimizing vaccine allocation at different points in time during an epidemic. PLoS ONE, 5(11): e13767: 1-11.
Mbah, M. L. N. & Gilligan, C. A. (2011). Resource allocation for epidemic control in metapopulations. PLoS ONE, 6(9): e24577:1-10.
Medlock, J. & Galvani, A. P. (2009). Optimizing influenza vaccine distribution. Science, 325(5948): 1705–1708.
Meyers, L., Galvani, A. & Medlock, J. (2009). Optimizing allocation for a delayed influenza vaccination campaign. PLoS Currents (DEC).
Mjelde, K. (1978). Discrete resource allocation by a branch and bound method. Journal of the Operational Research Society, 29(10): 1021–1023.
Mylius, S. D., Hagenaars, T. J., Lugnér, A. K. & Wallinga, J. (2008). Optimal allocation of pandemic influenza vaccine depends on age, risk and timing. Vaccine, 26(29-30): 3742–3749.
Nishiura, H. & Chowell, G. (2009). The effective reproduction number as a prelude to statistical estimation of time-dependent epidemic trends. In G. Chowell, J. Hyman, L. A. Bettencourt & C. Castillo-Chavez (eds.), Mathematical and Statistical Estimation Approaches in Epidemiology: 103–121, Springer Netherlands.
Ompad, D. C., Galea, S. & Vlahov, D. (2006). Distribution of influenza vaccine to high-risk groups. Epidemiologic Reviews, 28(1): 54–70.
Pappis, C. P. & Rachaniotis, N. P. (2010). Scheduling in a multi-processor environment with deteriorating job processing times and decreasing values: the case of forest fires. Journal of Heuristics, 16(4): 617–632.
R Core Team. (2013). R: a language and environment for statistical computing. R Foundation for Statistical Computing, Vienna, Austria. URL http://www.R-project.org/.
Rachaniotis, N. P., Dasaklis, T. K. & Pappis, C. P. (2012). A deterministic resource scheduling model in epidemic control: a case study. European Journal of Operational Research, 216(1): 225–231.
Riley, S., Fraser, C., Donnelly, C. A., Ghani, A. C., Abu-Raddad, L. J., Hedley, A. J., Leung, G. M., Ho, L. M., Lam, T. H., Thach, T. Q., Chau, P., Chan, K. P., Lo, S. V., Leung, P. Y., Tsang, T., Ho, W., Lee, K. H., Lau, E. M. C., Ferguson, N. M. & Anderson, R. M. (2003). Transmission dynamics of the etiological agent of SARS in Hong Kong: impact of public health interventions. Science, 300(5627): 1961–1966.
Samsuzzoha, M., Singh, M. & Lucy, D. (2012). A numerical study on an influenza epidemic model with vaccination and diffusion. Applied Mathematics and Computation, 219(1): 122–141.
Samsuzzoha, M., Singh, M. & Lucy, D. (2013). Uncertainty and sensitivity analysis of the basic reproduction number of a vaccinated epidemic model of influenza. Applied Mathematical Modelling, 37(3): 903–915.
Sander, B., Nizam, A., Garrison Jr, L. P., Postma, M. J., Halloran, M. E. & Longini, I. M. (2009). Economic evaluation of influenza pandemic mitigation strategies in the United States using a stochastic microsimulation transmission model. Value in Health, 12(2): 226–233.
Shih, W. (1974). A new application of incremental analysis in resource allocations. Operational Research Quarterly, 25(4): 587–597.
Shih, W. (1977). Branch and bound procedure for a class of discrete resource allocation problems with several constraints. Operational Research Quarterly, 28(2): 439–451.
Stein, M. L., Rudge, J. W., Coker, R., Weijden, C., Krumkamp, R., Hanvoravongchai, P., Chavez, I., Putthasri, W., Phommasack, B., Adisasmito, W., Touch, S., Sat, L. M., Hsu, Y. C., Kretzschmar, M. & Timen, A. (2012). Development of a resource modelling tool to support decision makers in pandemic influenza preparedness: the AsiaFluCap Simulator. BMC Public Health: 870.
Tuite, A. R., Fisman, D. N., Kwong, J. C. & Greer, A. L. (2010). Optimal pandemic influenza vaccine allocation strategies for the Canadian population. PLoS ONE, 5(5): e10520:1-11.
Uribe-Sánchez, A., Savachkin, A., Santana, A., Prieto-Santa, D. & Das, T. K. (2011). A predictive decision-aid methodology for dynamic mitigation of influenza pandemics. OR Spectrum, 33(3): 751–786.
Van Der Weijden, C. P., Stein, M. L., Jacobi, A. J., Kretzschmar, M. E. E., Reintjes, R., van Steenbergen, J. E. & Timen, A. (2013). Choosing pandemic parameters for pandemic preparedness planning: a comparison of pandemic scenarios prior to and following the influenza A(H1N1) 2009 pandemic. Health Policy, 109(1): 52–62.
Wallinga, J., Van Bovena, M. & Lipsitch, M. (2010). Optimizing infectious disease interventions during an emerging epidemic. Proceedings of the National Academy of Sciences of the United States of America, 107(2): 923–928.
Wichmann, O., Stöcker, P., Poggensee, G., Altmann, D., Walter, D., Hellenbrand, W., Krause, G. & Eckmanns, T. (2010). Pandemic influenza A(H1N1) 2009 breakthrough infections and estimates of vaccine effectiveness in Germany 2009-2010. Eurosurveillance, 15(18): 1–4.
Yaesoubi, R. & Cohen, T. (2011). Dynamic health policies for controlling the spread of emerging infections: influenza as an example. PLoS ONE, 6(9): e24043:1-11.
Yang, Y., Atkinson, P. M. & Ettema, D. (2011). Analysis of CDC social control measures using an agent-based simulation of an influenza epidemic in a city. BMC Infectious Diseases, 11.
Yarmand, H., Ivy, J. S., Denton, B. & Lloyd, A. L. (2014). Optimal two-phase vaccine allocation to geographically different regions under uncertainty. European Journal of Operational Research, 233(1): 208–219.
Zhou, L. & Fan, M. (2012). Dynamics of an SIR epidemic model with limited medical resources revisited. Nonlinear Analysis: Real World Applications, 13(1): 312–324.
Acknowledgments
We would like to thank the two anonymous referees for their help to improve the quality of this paper.
Author information
Authors and Affiliations
Corresponding author
Additional information
Nikolaos P. Rachaniotis is an Assistant Professor in Business Administration, Democritus University of Thrace, Department of Economics, Greece. He graduated from the National and Kapodistrian University of Athens, Dept. of Mathematics, Greece. He holds a M.Sc. degree from the same university in statistics and operational research and a Ph.D. degree from University of Piraeus, Dept. of Industrial Management, Greece, in operational research. His research interests lie in the areas of scheduling deteriorating jobs with time dependent parameters, humanitarian logistics, decision support systems, reverse logistics and routing and scheduling of fuel supply vessels and he has published papers in many international journals. He has participated in several national and European research projects. He has worked in Zayed University, Dubai, UAE, in INSEAD Social Innovation Centre, Humanitarian Research Group, Fontainebleau, France and in Otto von Guericke University, Faculty of Economics and Management, Department of Production and Logistics, Magdeburg, Germany.
Thomas K. Dasaklis is a seasonal lecturer in the Department of Industrial Management, University of Piraeus, Greece. He graduated from University of Piraeus, Department of Industrial Management. He holds a M.Sc. degree from the same department and a Ph.D. degree from the same department in emergency supply chain management. His research interests lie in the area of humanitarian logistics and he has published papers in several international journals. He has participated in National and European Research projects. He has previously worked in European Commission and in University of Piraeus Research Centre.
Costas P. Pappis is Emeritus Professor in production management at the University of Piraeus, Greece. He received his BS in production engineering from the National Technical University of Athens, his diploma in management studies from the Polytechnic of Central London and his PhD in engineering from the University of London. Prior to joining the university in 1993, he was an Associate Professor of production/operations management at the University of Patras, Greece. He has also worked as an Operations Analyst, as an Engineer in the Technical Services of the National Bank of Greece and as a Director of the Offsets Department of the Ministry of National Economy of Greece. He has been the President of the Hellenic OR Society and has published papers in many international journals.
Rights and permissions
About this article
Cite this article
Rachaniotis, N., Dasaklis, T.K. & Pappis, C. Controlling infectious disease outbreaks: A deterministic allocation-scheduling model with multiple discrete resources. J. Syst. Sci. Syst. Eng. 26, 219–239 (2017). https://doi.org/10.1007/s11518-016-5327-z
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11518-016-5327-z