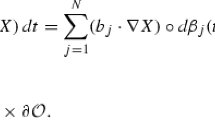Abstract
In this paper, we study the following stochastic Hamiltonian system in ℝ2d (a second order stochastic differential equation):
where b(x; v) : ℝ2d → ℝd and σ(x; v): ℝ2d → ℝd ⊗ ℝd are two Borel measurable functions. We show that if σ is bounded and uniformly non-degenerate, and b ∈ H 2/3,0 p and ∇σ ∈ Lp for some p > 2(2d+1), where H α, β p is the Bessel potential space with differentiability indices α in x and β in v, then the above stochastic equation admits a unique strong solution so that (x, v) ↦ Zt(x, v) := (Xt, Ẋt)(x, v) forms a stochastic homeomorphism flow, and (x, v) ↦ Zt(x, v) is weakly differentiable with ess.supx, v E(supt∈[0, T] |∇Zt(x, v)|q) < ∞ for all q ⩾ 1 and T ⩾ 0. Moreover, we also show the uniqueness of probability measure-valued solutions for kinetic Fokker-Planck equations with rough coefficients by showing the well-posedness of the associated martingale problem and using the superposition principle established by Figalli (2008) and Trevisan (2016).
Similar content being viewed by others
References
Bass R, Chen Z-Q. Brownian motion with singular drift. Ann Probab, 2003, 31: 791–817
Bergh J, Löfström J. Interpolation Spaces: An Introduction. Berlin: Springer-Verlag, 1976
Bogachev V I, Krylov N V, Röckner M. On regularity of transition probabilities and invariant measures of singular diffusions under minimal conditions. Comm Partial Differential Equations, 2001, 26: 2037–2080
Bogachev V I, Krylov N V, Röckner M. Elliptic and parabolic equations for measures. Russian Math Surveys, 2009, 64: 973–1078
Bouchut F. Hypoeliptic regularity in kinetic equations. J Math Pures Appl (9), 2002, 81: 1135–1159
Bramanti M, Cupini G, Lanconelli E, et al. Global L p-estimate for degenerate Ornstein-Uhlenbeck operators. Math Z, 2010, 266: 789–816
Chaudru de Raynal P E. Strong existence and uniqueness for stochastic differential equation with Hölder drift and degenerate noise. Ann Inst H Poincaré, 2017, 53: 259–286
Chen Z Q, Zhang X. L p-maximal hypoelliptic regularity of nonlocal kinetic Fokker-Planck operators. ArXiv: 1608.05502, 2016
Cherny A S. On the uniqueness in law and the pathwise uniqueness for stochastic differential equations. Theory Probab Appl, 2006, 46: 483–497
Crippa G, De Lellis C. Estimates and regularity results for the DiPerna-Lions ow. J Reine Angew Math, 2008, 616: 15–46
Fedrizzi E, Flandoli F. Noise prevents singularities in linear transport equations. J Funct Anal, 2013, 264: 1329–1354
Fedrizzi E, Flandoli F. Hölder flow and differentiability for SDEs with non regular drift. Stoch Anal Appl, 2013, 31: 708–736
Fedrizzi E, Flandoli F, Priola E, et al. Regularity of stochastic kinetic equations. ArXiv:1606.01088, 2016
Figalli A. Existence and uniqueness of martingale solutions for SDEs with rough or degenerate coeffcients. J Funct Anal, 2008, 254: 109–153
Jin P. Brownian motion with singular time-dependent drift. J Theoret Probab, 2017, 30: 1499–1538
Karatza I, Shreve S E. Brownian Motion and Stochastic Calculus. New York: Springer-Verlag, 1988
Krylov N V. Lectures on Elliptic and Parabolic Equations in Sobolev Spaces. Graduate Studies in Mathematics, vol. 96. Providence: Amer Math Soc, 2008
Krylov N V, Röckner M. Strong solutions of stochastic equations with singular time dependent drift. Probab Theory Related Fields, 2005, 131: 154–196
Kunita H. Stochastic flows and stochastic differential equations. Cambridge Studies in Advanced Mathematics, vol. 24. Cambridge: Cambridge University Press, 1990
Menoukeu-Pamen O, Meyer-Brandis T, Nilssen T, et al. A variational approach to the construction and Malliavin differentiability of strong solutions of SDE’s. Math Ann, 2013, 357: 761–799
Menozzi S. Martingale problems for some degenerate Kolmogorov equations. Stochastic Process Appl, 2017, 128: 756–802
Mohammed S E A, Nilssen T, Proske F. Sobolev differentiable stochastic flows for SDEs with singular coeffcients: Applications to the transport equation. Ann Probab, 2015, 43: 1535–1576
Priola E. On weak uniqueness for some degenerate SDEs by global Lp-estimate. Potential Anal, 2015, 42: 247–281
Röckner M, Zhang X. Weak uniqueness of Fokker-Planck equations with degenerate and bounded coeffcients. C R Math Acad Sci Paris, 2010, 348: 435–438
Soize C. The Fokker-Planck Equation for Stochastic Dynamical Systems and Its Explicit Steady State Solutions. Series on Advances in Mathematics for Applied Sciences, vol. 17. Singapore: World Scientic, 1994
Stein E M. Singular Integrals and Differentiability Properties of Functions. Princeton: Princeton University Press, 1970
Stroock D, Varadhan S R S. Multidimensional Diffusion Processes. Berlin: Springer-Verlag, 1997
Talay D. Stochastic Hamiltonian systems: Exponential convergence to the invariant measure and discretization by the implicit Euler scheme. Markov Process Related Fields, 2002, 8: 1–36
Trevisan D. Well-posedness of multidimensional diffusion processes with weakly differentiable coeffcients. Electron J Probab, 2016, 21, doi: 10.1214/16-EJP4453
Wang F, Zhang X. Degenerate SDE with Hölder-Dini drift and non-Lipschitz noise coeffcient. SIAM J Math Anal, 2016, 48: 2189–2222
Xie L, Zhang X. Sobolev differentiable flows of SDEs with local Sobolev and super-linear growth coeffcients. Ann Probab, 2016, 44: 3661–3687
Zhang X. Strong solutions of SDEs with singular drift and Sobolev diffusion coeffcients. Stochastic Process Appl, 2005, 115: 1805–1818
Zhang X. Stochastic homeomorphism flows of SDEs with singular drifts and Sobolev diffusion coeffcients. Electron J Probab, 2011, 16: 1096–1116
Zhang X. Stochastic partial differential equations with unbounded and degenerate coeffcients. J Differential Equations, 2011, 250: 1924–1966
Zhang X. Stochastic differential equations with Sobolev diffusion and singular drift. Ann Appl Probab, 2016, 26: 2697–2732
Zvonkin A K. A transformation of the phase space of a diffusion process that removes the drift. Mat Sb, 1974, 93: 129–149
Author information
Authors and Affiliations
Corresponding author
Additional information
Dedicated to the 60th Birthday of Professor Michael Röckner
Rights and permissions
About this article
Cite this article
Zhang, X. Stochastic Hamiltonian flows with singular coefficients. Sci. China Math. 61, 1353–1384 (2018). https://doi.org/10.1007/s11425-017-9127-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11425-017-9127-0
Keywords
- stochastic Hamiltonian system
- weak differentiability
- Krylov’s estimate
- Zvonkin’s transformation
- kinetic Fokker-Planck operator