Skip to main content
SpringerLink
Log in

Optimal Multimodel Representation by Laguerre Filters Applied to a Communicating Two Tank System

  • Published:
Journal of Systems Science and Complexity Aims and scope Submit manuscript

Abstract

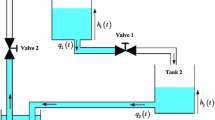
This paper presents the development of a new nonlinear representation by exploiting the multimodel approach and the new linear representation ARX-Laguerre for each operating region. The resulting multimodel, entitled ARX-Laguerre multimodel, is characterized by the parameter number reduction with a recursive representation. However, a significant reduction of this multimodel is subject to an optimal choice of Laguerre poles characterizing each local linear model ARX-Laguerre. Therefore, the authors propose an optimization algorithm to estimate, from input/output measurements, the optimal values of Laguerre poles. The ARX-Laguerre multimodel as well as the proposed optimization algorithm are tested on a continuous stirred tank reactor system (CSTR). Moreover, the authors take into account a practical validation on an experimental communicating two tank system (CTTS).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Bouzrara K, Mbarek A, Garna T, et al., Nonlinear predictive controller for uncertain process modeled by GOBF-Volterra models, International Journal of Modelling, Identification and Control, 2013, 19(4): 307–322.

    Article  Google Scholar 

  2. Diouf C, Telescu M, Cloastre P, et al., On the use of equality constraints in the identification of volterra-laguerre models, IEEE Signal Processing Letters, 2012, 19(12): 857–860.

    Article  Google Scholar 

  3. Ricardo J G B Campello, Gard Favier, andWagner C do Amaralc, Optimal expansions of discretetime Volterra models using Laguerre functions, Automatica, 2004, 40(5): 815–822.

    Article  MathSciNet  Google Scholar 

  4. Dumont G and Fu Y, Non-linear adaptive control via Laguerre expansion of Volterra kernels, Int. J. Adapt. Contr. Signal Process., 1993, 7(5): 367–382.

    Article  MATH  Google Scholar 

  5. Boyd S and Chua L O, Fading memory and the problem of approximating nonlinear operators with Volterra series, IEEE Tr. Circuits and Systems, 1985, 32(11): 1150–1161.

    Article  MathSciNet  MATH  Google Scholar 

  6. Kibangou A Y, Favier G, and Hassani M M, Laguerre-Volterra filters optimization based on Laguerre spectra, EURASIP Journal on Advances in Signal Processing, 2005, 17: 2874–2887.

    MATH  Google Scholar 

  7. Garna T, Bouzrara K, Ragot J, et al., Nonlinear system modeling based on bilinear Laguerre orthonormal bases, ISA transactions, 2013, 52(3): 301–317.

    Article  MATH  Google Scholar 

  8. Garna T, Bouzrara K, Ragot J, et al., Optimal expansions of discrete-time bilinear models using Laguerre functions, IMA Journal of Mathematical Control & Information, 2013, 31(3): 313–343.

    Article  MathSciNet  MATH  Google Scholar 

  9. Ghassen M, Abdelkader M, Tarek G, et al., Nonlinear model based predictive control using multiple models approach expanded on Laguerre bases, Wseas Transactions on Systems and Control, 2015, 10: 113–126.

    Google Scholar 

  10. Sbarbaro D and Johansen T A, Multiple local Laguerre models for modelling nonlinear dynamic systems of the Wiener class, IEE Proceedings, Control Theory Applications, 1997, 144(5): 375–380.

    Article  MATH  Google Scholar 

  11. Sbarbaro D, Context sensitive networks for modelling nonlinear dynamic systems, Proceedings of 3rd European Control Conference, 1995, 3b: 2420–2425.

    Google Scholar 

  12. Boukhris A, Mourot G, and Ragot J, Non-linear dynamic system identification: A multi-model approach, International Journal of Control, 1999, 72(7–8): 591–604.

    Article  MathSciNet  MATH  Google Scholar 

  13. Orjuela R, Marx B, Ragot J, et al., State estimation for nonlinear systems using decoupled multiple model, International Journal of Modelling Identification and Control, 2008, 4(1): 59–67.

    Article  Google Scholar 

  14. Bouzrara K, Garna T, Ragot J, et al., Decomposition of an ARX model on orthonormal Laguerre bases, ISA Transactions, 2012, 51(6): 848–860.

    Article  Google Scholar 

  15. Demuth H, Beale M, and Hagan M, Neural Network Toolbox 5, User’s Guide, The MathWorks, 2007.

    Google Scholar 

  16. Filev D, Fuzzy modeling of complex systems, International Journal of Approximate Reasoning, 199, 5(3): 281–290.

  17. Johansen T A and Foss A B, Constructing NARMAX using ARMAX models, International Journal of Control, 1993, 58(5): 1125–1153.

    Article  MathSciNet  MATH  Google Scholar 

  18. Johansen T A and Foss B A, Identification of non-linear system structure and parameters using regime decomposition, Automatica, 1995, 31(2): 321–326.

    Article  MathSciNet  MATH  Google Scholar 

  19. Narendra K S and Cheng X, Adaptive control of discrete-time systems using multiple models, IEEE Transactions on Automatic Control, 2000, 45(9): 1669–1686.

    Article  MathSciNet  MATH  Google Scholar 

  20. Bouzrara K, Garna T, Ragot J, et al., Online identification of the ARX model expansion on Laguerre orthonormal bases with filtres on model input and output, International Journal of Control, 2013, 86(3): 369–385.

    Article  MathSciNet  MATH  Google Scholar 

  21. Tanguy N, Morvan R, Vilbé P, et al., Online optimization of the time scale in adaptive Laguerrebased filters, IEEE Transactions on Signal Processing, 2000, 48(4): 1184–1187.

    Article  Google Scholar 

  22. Seborg D E, Edger T F, and Millichamp D A, Process Dynamics and Control, John Wiley & Sons, 2004.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Laboratory of Automatic Control, Signal and Image Processing, National Engineering School of Monastir, University of Monastir, Monastir, 5019, Tunisia

    Adaily Sameh, Mbarek Abdelkader & Garna Tarek

  2. Higher Institute of Applied Science and Technology of Sousse, University of Sousse, Sousse, 4003, Tunisia

    Garna Tarek

  3. Center of Research on Automatic of Nancy, CNRS, 2 Avenue de la for de Haye, 54516, Vandoeuvre Cedex, France

    Ragot José

Authors
  1. Adaily Sameh
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mbarek Abdelkader
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Garna Tarek
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Ragot José
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mbarek Abdelkader.

Additional information

This paper was recommended for publication by Editor CHEN Jie.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sameh, A., Abdelkader, M., Tarek, G. et al. Optimal Multimodel Representation by Laguerre Filters Applied to a Communicating Two Tank System. J Syst Sci Complex 31, 621–646 (2018). https://doi.org/10.1007/s11424-017-6047-2

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11424-017-6047-2

Keywords

Search

Navigation