Abstract
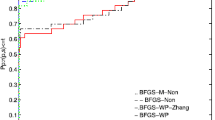
This paper presents a new nonmonotone filter line search technique in association with the MBFGS method for solving unconstrained minimization. The filter method, which is traditionally used for constrained nonlinear programming (NLP), is extended to solve unconstrained NLP by converting the latter to an equality constrained minimization. The nonmonotone idea is employed to the filter method so that the restoration phrase, a common feature of most filter methods, is not needed. The global convergence and fast local convergence rate of the proposed algorithm are established under some reasonable conditions. The results of numerical experiments indicate that the proposed method is efficient.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Li D H and Fukushima M, A modified BFGS method and its global convergence in nonconvex minimization, J. Comput. Appl. Math., 2001, 129(1): 15–35.
Fletcher R and Leyffer S, Nonlinear programming without a penaty function, Math. Program., 2002, 91(2): 239–269.
Gould N I M, Sainvitu C, and Toint P L, A filter-trust-region method for unconstrained optimization, SIAM J. Optim., 2005, 16(2): 341–357.
Krejić N, Lužanin Z, and Stojkovska I, Gauss-Newton-based BFGS method with filter for unconstrained minimization, Appl. Math. Comput., 2009, 211(2): 354–362.
Li S J and Liu Z H, A new trust region filter algorithm, Appl. Math. Comput., 2008, 204(1): 485–489.
Wu T, Solving unconstrained optimization problem with a filter-based nonmonotone pattern search algorithm, Appl. Math. Comput., 2008, 203(1): 380–386.
Wu T and Sun L P, A filter-based pattern search method for unconstrained optimization, Numer. Math. J. Chinese Univ., 2006, 15(3): 209–216.
Wang Z J and Zhu D T, A filter-line-search method for unconstrained optimization, J. Appl. Math. Comput., 2010, 34(1): 329–324.
Grippo L, Lampariello F, and Lucidi S, A nonmonotone line search technique for Newton’s method, SIAM J. Numer. Anal., 1986, 23(4): 707–716.
Dai Y H, A nonmonotone conjugate gradient algorithm for unconstrained optimization, Journal of Systems Science and Complexity, 2002, 15(2): 139–145.
Liu G H, Han J Y, and Sun D F, Global convergence of the BFGS algorithm with nonmonotone line search, Optimization, 1995, 34(1): 147–159.
Zhang H C and Hager W W, A nonmonotone line search technique and its application to unconstrained optimization, SIAM J. Optim., 2004, 14(4): 1043–1056.
Dai Y H, Convergence properties of the BFGS algorithm, SIAM J. Optim., 2002, 13(3): 693–701.
Mascarenhas W F, The BFGS method with exact line searches fails for non-convex objective functions, Math. Program., 2004, 99(1): 49–61.
Nocedal J and Wright S, Numerical Optimization, Springer-Verlag, Berlin, 1999.
Andrei N, Unconstrained optimization test functions, http://www.ici.rol/camo/neculai/SCALCG/testuo.pdf.
Author information
Authors and Affiliations
Corresponding author
Additional information
This research is supported by the National Science Foundation under Grant No. 11371253 and the Science Foundation under Grant No. 11C0336 of Provincial Education Department of Hunan.
This paper was recommended for publication by Editor ZHANG Hanqin.
Rights and permissions
About this article
Cite this article
Wang, Z., Zhu, D. A nonmonotone filter line search technique for the MBFGS method in unconstrained optimization. J Syst Sci Complex 27, 565–580 (2014). https://doi.org/10.1007/s11424-014-1081-9
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11424-014-1081-9