Skip to main content
SpringerLink
Log in

Quantum coherence behaviors of fermionic system in non-inertial frame

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

Abstract

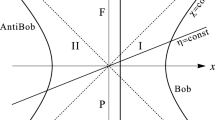
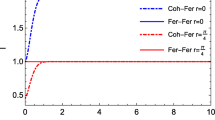
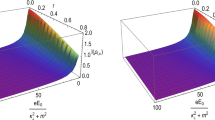
In this paper, we analyze the quantum coherence behaviors of a single qubit in the relativistic regime beyond the single-mode approximation. Firstly, we investigate the freezing condition of quantum coherence in fermionic system. We also study the quantum coherence tradeoff between particle and antiparticle sector. It is found that there exists quantum coherence transfer between particle and antiparticle sector, but the coherence lost in particle sector is not entirely compensated by the coherence generation of antiparticle sector. Besides, we emphatically discuss the cohering power and decohering power of Unruh channel with respect to the computational basis. It is shown that cohering power is vanishing and decohering power is dependent of the choice of Unruh mode and acceleration. Finally, we compare the behaviors of quantum coherence with geometric quantum discord and entanglement in relativistic setup. Our results show that this quantifiers in two region converge at infinite acceleration limit, which implies that this measures become independent of Unruh modes beyond the single-mode approximations. It is also demonstrated that the robustness of quantum coherence and geometric quantum discord are better than entanglement under the influence of acceleration, since entanglement undergoes sudden death.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Peres, A., Terno, D.R.: Quantum information and relativity theory. Rev. Mod. Phys. 74, 93 (2004)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  2. Friis, N., Bruschi, D.E., Louko, J., Fuentes, I.: Motion generates entanglement. Phys. Rev. D 85, 081701(R) (2012)

    Article  ADS  Google Scholar 

  3. Bruschi, D.E., Fuentes, I., Louko, J.: Voyage to Alpha Centauri: entanglement degradation of cavity modes due to motion. Phys. Rev. D 85, 061701(R) (2012)

    Article  ADS  Google Scholar 

  4. Wang, J.C., Tian, Z.H., Jing, J.L., Fan, H.: Irreversible degradation of quantum coherence under relativistic motion. Phys. Rev. A 93, 062105 (2016)

    Article  ADS  Google Scholar 

  5. Wang, J.C., Tian, Z.H., Jing, J.L., Fan, H.: Influence of relativistic effects on satellite-based clock synchronization. Phys. Rev. D 93, 065008 (2016)

    Article  ADS  MathSciNet  Google Scholar 

  6. Wang, J.C., Jing, J.L., Fan, H.: Quantum discord and measurement-induced disturbance in the background of dilaton black holes. Phys. Rev. D 90, 025032 (2014)

    Article  ADS  Google Scholar 

  7. Martin-Martinez, E., León, J.: Fermionic entanglement that survives a black hole. Phys. Rev. A 80, 042318 (2009)

    Article  ADS  Google Scholar 

  8. Martin-Martinez, E., Garay, L.J., León, J.: Unveiling quantum entanglement degradation near a Schwarzschild black hole. Phys. Rev. D 82, 064006 (2010)

    Article  ADS  Google Scholar 

  9. Martin-Martinez, E., Garay, L.J., León, J.: Quantum entanglement produced in the formation of a black hole. Phys. Rev. D 82, 064028 (2010)

    Article  ADS  Google Scholar 

  10. Alsing, P.M., Fuentes-Schulle, I., Mann, R.B., Tessier, T.E.: Entanglement of Dirac fields in noninertial frames. Phys. Rev. A 74, 032326 (2006)

    Article  ADS  Google Scholar 

  11. Pan, Q., Jing, J.: Degradation of nonmaximal entanglement of scalar and Dirac fields in noninertial frames. Phys. Rev. A 77, 024302 (2008)

    Article  ADS  Google Scholar 

  12. Hwang, M.R., Park, D., Jung, E.: Tripartite entanglement in a noninertial frame. Phys. Rev. A 83, 012111 (2010)

    Article  ADS  Google Scholar 

  13. Wang, J.C., Cao, H.X., Jing, J.L., Fan, H.: Gaussian quantum steering and its asymmetry in curved spacetime. Phys. Rev. D 93, 125011 (2016)

    Article  ADS  MathSciNet  Google Scholar 

  14. Montero, M., Martn-Martnez, E.: Entanglement of arbitrary spin fields in non-inertial frames. Phys. Rev. A 84, 012337 (2011)

    Article  ADS  Google Scholar 

  15. Ramzan, M., Khan, M.K.: Decoherence and entanglement degradation of a qubit-qutrit system in non-inertial frames. Quantum Inf. Process. 11, 443 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  16. Wang, J., Deng, J., Jing, J.: Classical correlation and quantum discord sharing of Dirac fields in noninertial frames. Phys. Rev. A 81, 052120 (2010)

    Article  ADS  Google Scholar 

  17. Brown, E.G., Cormier, K., Martn-Martnez, E., Mann, R.B.: Vanishing geometric discord in non-inertial frames. Phys. Rev. A 86, 032108 (2012)

    Article  ADS  Google Scholar 

  18. Ramzan, M.: Decoherence dynamics of geometric measure of quantum discord and measurement induced nonlocality for noninertial observers at finite temperature. Quantum Inf. Process. 12, 2721 (2013)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  19. Bruschi, D.E., Louko, J., Martín-Martínez, E., Dragan, A., Fuentes, I.: Unruh effect in quantum information beyond the single-mode approximation. Phys. Rev. A 82, 042332 (2010)

    Article  ADS  Google Scholar 

  20. Montero, M., Martín-Martínez, E.: Fermionic entanglement ambiguity in non-inertial frames. Phys. Rev. A 83, 062323 (2011)

    Article  ADS  Google Scholar 

  21. Martín-Martínez, E., Fuentes, I.: Redistribution of particle and antiparticle entanglement in noninertial frames. Phys. Rev. A 83, 052306 (2011)

    Article  ADS  Google Scholar 

  22. Montero, M., Martin-Martinez, E.: Convergence of fermionic field entanglement at infinite acceleration in relativistic quantum information. Phys. Rev. A 85, 024301 (2012)

    Article  ADS  Google Scholar 

  23. Chang, J., Kwon, Y.: Entanglement behavior of quantum states of fermionic system in accelerated frame. Phys. Rev. A 85, 032302 (2012)

    Article  ADS  Google Scholar 

  24. Ramzan, M.: Quantum discord amplification of fermionic systems in an accelerated frame. Quantum Inf. Process. 13, 259 (2014)

    Article  MATH  Google Scholar 

  25. Glauber, R.J.: Coherent and incoherent states of the radiation field. Phys. Rev. 131, 2766 (1963)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  26. Sudarshan, E.C.G.: Equivalence of semiclassical and quantum mechanical descriptions of statistical light beams. Phys. Rev. Lett. 10, 277 (1963)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  27. Scully, M.O.: Enhancement of the index of refraction via quantum coherence. Phys. Rev. Lett. 67, 1855 (1991)

    Article  ADS  Google Scholar 

  28. Mandel, L., Wolf, E.: Optical Coherence and Quantum Optics. Cambridge University Press, Cambridge (1995)

    Book  Google Scholar 

  29. Asbóth, J.K., Calsamiglia, J., Ritsch, H.: Computable measure of nonclassicality for light. Phys. Rev. Lett. 94, 173602 (2005)

    Article  ADS  Google Scholar 

  30. Vogel, W., Sperling, J.: Unified quantification of nonclassicality and entanglement. Phys. Rev. A 89, 052302 (2014)

    Article  ADS  Google Scholar 

  31. Mraz, M., Sperling, J., Vogel, W., Hage, B.: Witnessing the degree of nonclassicality of light. Phys. Rev. A 90, 033812 (2014)

    Article  ADS  Google Scholar 

  32. Roßnagel, J., Abah, O., Schmidt-Kaler, F., Singer, K., LutzNanoscale, E.: Heat engine beyond the carnot limit. Phys. Rev. Lett. 112, 030602 (2014)

    Article  ADS  Google Scholar 

  33. Åberg, J.: Catalytic coherence. Phys. Rev. Lett. 113, 150402 (2014)

    Article  Google Scholar 

  34. Correa, L.A., Palao, J.P., Alonso, D., Adesso, G.: Quantum-enhanced absorption refrigerators. Sci. Rep. 4, 3949 (2014)

    Article  ADS  Google Scholar 

  35. Narasimhachar, V., Gour, G.: Low-temperature thermodynamics with quantum coherence. Nat. Commun. 6, 7689 (2015)

    Article  ADS  Google Scholar 

  36. Lostaglio, M., Jennings, D., Rudolph, T.: Description of quantum coherence in thermodynamic processes requires constraints beyond free energy. Nat. Commun. 6, 6383 (2015)

    Article  ADS  Google Scholar 

  37. Nielsen, M., Chuang, I.: Quantum Computation and Quantum Information. Cambridge University Press, Cambridge (2000)

    MATH  Google Scholar 

  38. Huang, Z.M., Situ, H.Z.: Dynamics of quantum correlation and coherence for two atoms coupled with a bath of fluctuating massless scalar feld. Ann. Phys. 377, 484 (2017)

    Article  ADS  MATH  Google Scholar 

  39. Huang, Z.M., Situ, H.Z., Zhao, L.H.: Payoffs and coherence of a quantum two-player game under noisy environment. Eur. Phys. J. Plus 132, 152 (2017)

    Article  Google Scholar 

  40. Huang, Z.M.: Dynamics of quantum correlation and coherence in de Sitter universe. Quantum Inf. Process. 16, 207 (2017)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  41. Huang, Z.M., Situ, H.Z.: Optimal protection of quantum coherence in noisy environment. Int. J. Theor. Phys. 56, 503 (2017)

    Article  MATH  Google Scholar 

  42. Huang, Z.M.: Quantum correlation and coherence in the background of dilaton black hole. J. Phys. Soc. Jpn. 86, 124007 (2017)

    Article  ADS  Google Scholar 

  43. Huang, Z.M., Situ, H.Z.: Non-Markovian dynamics of quantum coherence of two-level system driven by classical field. Quantum Inf. Process. 16, 222 (2017)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  44. Situ, H.Z., Hu, X.Y.: Dynamics of relative entropy of coherence under Markovian channels. Quantum Inf. Process. 15, 4649 (2016)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  45. Deveaud-Plédran, B., Quattropani, A., Schwendimann P. (Eds.), Quantum coherence in solid state systems. In: Proceedings of the International School of Physics Enrico Fermi, vol. 171, IOS Press, Amsterdam, ISBN: 978-1-60750-039-1 (2009)

  46. Li, C.-M., Lambert, N., Chen, Y.-N., Chen, G.-Y., Nori, F.: Witnessing quantum coherence: from solid-state to biological systems. Sci. Rep. 2, 885 (2012)

    Article  Google Scholar 

  47. Engel, G.S., Calhoun, T.R., Read, E.L., Ahn, T.-K., Manc̆al, T., Cheng, Y.-C., Blakenship, R.E., Fleming, G.R.: Evidence for wavelike energy transfer through quantum coherence in photosynthetic systems. Nature (London) 446, 782 (2007)

    Article  ADS  Google Scholar 

  48. Collini, E., Wong, C.Y., Wilk, K.E., Curmi, P.M.G., Brumer, P., Scholes, G.D.: Coherently wired light-harvesting in photosynthetic marine algae at ambient temperature. Nature (London) 463, 644 (2010)

    Article  ADS  Google Scholar 

  49. Lambert, N., Chen, Y.-N., Cheng, Y.-C., Li, C.-M., Chen, G.-Y., Nori, F.: Quantum biology. Nat. Phys. 9, 10 (2013)

    Article  Google Scholar 

  50. Chin, A.W., Prior, J., Rosenbach, R., Caycedo-Soler, F., Huelga, S.F., Plenio, M.B.: The role of non-equilibrium vibrational structures in electronic coherence and recoherence in pigment–protein complexes. Nat. Phys. 9, 113 (2013)

    Article  Google Scholar 

  51. Cai, J., Plenio, M.B.: Chemical compass model for avian magnetoreception as a quantum coherent device. Phys. Rev. Lett. 111, 230503 (2013)

    Article  ADS  Google Scholar 

  52. Baumgratz, T., Cramer, M., Plenio, M.B.: Quantifying coherence. Phys. Rev. Lett. 113, 140401 (2014)

    Article  ADS  Google Scholar 

  53. Levi, F., Mintert, F.: A quantitative theory of coherent delocalization. New J. Phys. 16, 033007 (2014)

    Article  ADS  Google Scholar 

  54. Girolami, D.: Observable measure of quantum coherence in finite dimensional systems. Phys. Rev. Lett. 113, 170401 (2014)

    Article  ADS  Google Scholar 

  55. Smyth, C., Scholes, G.D.: Method of developing analytical multipartite delocalization measures for mixed W-like states. Phys. Rev. A 90, 032312 (2014)

    Article  ADS  Google Scholar 

  56. Pires, D.P., Celeri, L.C., Soares-Pinto, D.O.: Geometric lower bound for a quantum coherence measure. Phys. Rev. A 91, 042330 (2015)

    Article  ADS  Google Scholar 

  57. Streltsov, A., Singh, U., Dhar, H.S., Bera, M.N., Adesso, G.: Measuring quantum coherence with entanglement. Phys. Rev. Lett. 115, 020403 (2015)

    Article  ADS  MathSciNet  Google Scholar 

  58. Rana, S., Parashar, P., Lewenstein, M.: Trace-distance measure of coherence. Phys. Rev. A 93, 012110 (2016)

    Article  ADS  MathSciNet  Google Scholar 

  59. Yao, Y., Xiao, X., Ge, L., Sun, C.P.: Quantum coherence in multipartite systems. Phys. Rev. A 92, 022112 (2015)

    Article  ADS  Google Scholar 

  60. Chitambar, E., Hsieh, M.-H.: Relating the resource theories of entanglement and quantum coherence. arXiv:1509.07458 (2015)

  61. Ma, J.J., Yadin, B., Girolami, D., Vedral, V., Gu, M.: Converting coherence to quantum correlations. Phys. Rev. Lett. 116, 160407 (2016)

    Article  ADS  Google Scholar 

  62. Xi, Z., Li, Y., Fan, H.: Quantum coherence and correlations in quantum system. Sci. Rep. 5, 10922 (2015)

    Article  ADS  Google Scholar 

  63. Hu, X., Fan, H.: Coherence extraction from measurement-induced disturbance. arXiv:1508.01978 (2015)

  64. Mani, A., Karimipour, V.: Cohering and decohering power of quantum channels. Phys. Rev. A 92, 032331 (2015)

    Article  ADS  Google Scholar 

  65. Xi, Z., Hu, M., Li, Y., Fan, H.: Cohering power of unitary operations and de-cohering of quantum operations. arXiv:1510.06473 (2015)

  66. Garca-Daz, M., Egloff, D., Plenio, M.B.: A note on coherence power of N-dimensional unitary operators. arXiv:1510.06683 (2015)

  67. Martín-Martínez, E.: Relativistic quantum information: developments in quantum information in general relativistic scenarios. arXiv:1106.0280 (2011)

  68. Ollivier, H., Zurek, W.H.: Quantum discord: a measure of the quantumness of correlations. Phys. Rev. Lett. 88, 017901 (2001)

    Article  ADS  MATH  Google Scholar 

  69. Henderson, L., Vedral, V.: Classical, quantum and total correlations. J. Phys. A Math. Gen. 34, 6899 (2001)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  70. Dakić, B., Vedral, V., Brukner, Č.: Necessary and sufficient condition for nonzero quantum discord. Phys. Rev. Lett. 105, 190502 (2010)

    Article  ADS  MATH  Google Scholar 

  71. Luo, S.L., Fu, S.S.: Geometric measure of quantum discord. Phys. Rev. A 82, 034302 (2010)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  72. Lu, X.M., Xi, Z.J., Sun, Z., Wang, X.: Geometric measure of quantum discord under decoherence. Quantum Inf. Comput. 10, 0994 (2010)

    MathSciNet  MATH  Google Scholar 

  73. Wootters, W.K.: Entanglement of formation of an arbitrary state of two qubits. Phys. Rev. Lett. 80, 2245 (1998)

    Article  ADS  MATH  Google Scholar 

Download references

Acknowledgements

This work is supported by the Science Foundation for Young Teachers of Wuyi University (2015zk01), the Doctoral Research Foundation of Wuyi University (2017BS07) and the National Natural Science Foundation of China (No. 61502179).

Author information

Authors and Affiliations

  1. School of Economics and Management, Wuyi University, Jiangmen, 529020, China

    Zhiming Huang

  2. College of Mathematics and Informatics, South China Agricultural University, Guangzhou, 510642, China

    Haozhen Situ

Authors
  1. Zhiming Huang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Haozhen Situ
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Zhiming Huang.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Huang, Z., Situ, H. Quantum coherence behaviors of fermionic system in non-inertial frame. Quantum Inf Process 17, 95 (2018). https://doi.org/10.1007/s11128-018-1867-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-018-1867-0

Keywords

Search

Navigation