Skip to main content
SpringerLink
Log in

Disentanglement and quantum states transitions dynamics in spin-qutrit systems: dephasing random telegraph noise and the relevance of the initial state

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

Abstract

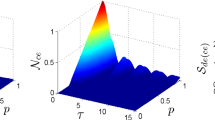
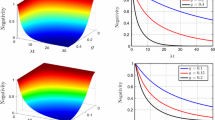
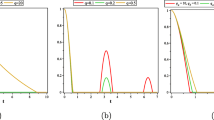
Using negativity and realignment criterion as quantifiers of free and bound entanglements respectively, we present in details the analytical study of the entanglements and quantum states transitions dynamics in a two-qutrit system driven by dephasing random telegraph noise channel(s). Both collective and independent system–environment couplings as well as the Markovian and the non-Markovian regimes of the noise channel(s) are considered. Two non-equivalent initial states and their locally equivalent through a local unitary operation (LUO) are also considered. We demonstrate a stronger entanglement under independent Markovian environments than with a collective one; meanwhile, for the non-Markovian regime, entanglement is stronger under a collective environment than with independent ones. States transitions as well as the (re)activation of bound entanglement (for initially free entangled states) can be found for a specific class of initial states, but can, however, be avoided by means of a LUO on the initial state. While unavoidable disentanglement occurs for independents coupling, we demonstrate the possibility of indefinite free entanglement survival in the qutrit system under a common environment by converting the initial entangled state using the local unitary operation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. Nielsen, M.A., Chuang, I.L.: Quantum Computation and Quantum Information. Cambridge University Press, Cambridge (2000)

    MATH  Google Scholar 

  2. Cui, J., Gu, M., Kwek, L.C., Santos, M.F., Fan, H., Vedral, V.: Quantum phases with differing computational power. Nat. Commun. 3, 812 (2012)

    Article  ADS  Google Scholar 

  3. Ekert, A.K.: Quantum cryptography based on Bells theorem. Phys. Rev. Lett. 67(6), 661 (1991)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  4. Bennett, C.H., Brassard, G., Crpeau, C., Jozsa, R., Peres, A., Wootters, W.K.: Teleporting an unknown quantum state via dual classical and Einstein–Podolsky–Rosen channels. Phys. Rev. Lett. 70(13), 1895 (1993)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  5. Murao, M., Jonathan, D., Plenio, M.B., Vedral, V.: Quantum telecloning and multiparticle entanglement. Phys. Rev. A 59(1), 156 (1999)

    Article  ADS  Google Scholar 

  6. Raussendorf, R., Briegel, H.J.: A one-way quantum computer. Phys. Rev. Lett. 86(22), 5188 (2001)

    Article  ADS  Google Scholar 

  7. Richter, T., Vogel, W.: Nonclassical characteristic functions for highly sensitive measurements. Phys. Rev. A 76(5), 053835 (2007)

    Article  ADS  Google Scholar 

  8. Hu, C.Y., Rarity, J.G.: Loss-resistant state teleportation and entanglement swapping using a quantum-dot spin in an optical microcavity. Phys. Rev. B 83(11), 115303 (2011)

    Article  ADS  Google Scholar 

  9. Chekhova, M., Kulik, S., Chekhova, M., Kulik, S.: Physical Foundations of Quantum Electronics by David Klyshko, 1st edn. WS, Singpore (2011)

    Book  Google Scholar 

  10. Moreva, E., Brida, G., Gramegna, M., Giovannetti, V., Maccone, L., Genovese, M.: Time from quantum entanglement: an experimental illustration. Phys. Rev. A 89(5), 052122 (2014)

    Article  ADS  Google Scholar 

  11. Grassani, D., Azzini, S., Liscidini, M., Galli, M., Strain, M.J., Sorel, M., Sipe, J.E., Bajoni, D.: Micrometer-scale integrated silicon source of time-energy entangled photons. Optica 2(2), 88 (2015)

    Article  Google Scholar 

  12. Zurek, W.H.: Decoherence, einselection, and the quantum origins of the classical. Rev. Mod. Phys. 75(3), 715 (2003)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  13. Yu, T., Eberly, J.H.: Finite-time disentanglement via spontaneous emission. Phys. Rev. Lett. 93(14), 140404 (2004)

    Article  ADS  Google Scholar 

  14. Yu, T., Eberly, J.: Sudden death of entanglement: classical noise effects. Opt. Commun. 264(2), 393 (2006)

    Article  ADS  Google Scholar 

  15. Yu, T., Eberly, J.H.: Sudden death of entanglement. Science 323(5914), 598 (2009)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  16. Lopez, C.E., Romero, G., Lastra, F., Solano, E., Retamal, J.C.: Sudden birth versus sudden death of entanglement in multipartite systems. Phys. Rev. Lett. 101(8), 080503 (2008)

    Article  ADS  Google Scholar 

  17. Lo Franco, R., Bellomo, B., Andersson, E., Compagno, G.: Revival of quantum correlations without system environment back-action. Phys. Rev. A 85(3), 032318 (2012)

    Article  ADS  Google Scholar 

  18. Xu, J.S., Sun, K., Li, C.F., Xu, X.Y., Guo, G.C., Andersson, E., Franco, R.L., Compagno, G.: Experimental recovery of quantum correlations in absence of system environment back-action. Nat. Commun. 4, 2851 (2013)

    Google Scholar 

  19. Bellomo, B., Lo Franco, R., Compagno, G.: Entanglement dynamics of two independent qubits in environments with and without memory. Phys. Rev. A 77(3), 032342 (2008)

    Article  ADS  Google Scholar 

  20. Mazzola, L.: Sudden death and sudden birth of entanglement in common structured reservoirs. Phys. Rev. A 79(4), 042302 (2009)

    Article  ADS  Google Scholar 

  21. Hu, J.: Entanglement dynamics for uniformly accelerated two-level atoms. Phys. Rev. A 91(1), 012327 (2015)

    Article  MathSciNet  ADS  Google Scholar 

  22. Huang, Z., Qiu, D., Mateus, P.: Geometry and dynamics of one-norm geometric quantum discord. Quantum Inf. Process. 15(1), 301 (2016)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  23. Situ, H., Hu, X.: Dynamics of relative entropy of coherence under Markovian channels. Quantum Inf. Process. 15(11), 4649 (2016)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  24. Huang, Z., Situ, H.: Non-Markovian dynamics of quantum coherence of two-level system driven by classical field. Quantum Inf. Process. 16(9), 222 (2017)

    Article  MathSciNet  ADS  Google Scholar 

  25. Huang, Z., Tian, Z.: Dynamics of quantum entanglement in de Sitter spacetime and thermal Minkowski spacetime. Nucl. Phys. B 923, 458 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  26. Bellomo, B., Lo Franco, R., Compagno, G.: Non-Markovian effects on the dynamics of entanglement. Phys. Rev. Lett. 99(16), 160502 (2007)

    Article  ADS  Google Scholar 

  27. Girolami, D., Adesso, G.: Quantum discord for general two-qubit states: analytical progress. Phys. Rev. A 83(5), 052108 (2011)

    Article  ADS  Google Scholar 

  28. Ciccarello, F., Giovannetti, V.: Creating quantum correlations through local nonunitary memoryless channels. Phys. Rev. A 85(1), 010102 (2012)

    Article  ADS  Google Scholar 

  29. Kuznetsova, E., Zenchuk, A.: Quantum discord versus second-order MQ NMR coherence intensity in dimers. Phys. Lett. A 376(10–11), 1029 (2012)

    Article  MATH  ADS  Google Scholar 

  30. Benedetti, C., Buscemi, F., Bordone, P., Paris, M.G.A.: Dynamics of quantum correlations in colored-noise environments. Phys. Rev. A 87(5), 052328 (2013)

    Article  ADS  Google Scholar 

  31. Javed, M., Khan, S., Ullah, S.A.: The dynamics of quantum correlations in mixed classical environments. J. Russ. Laser Res. 37(6), 562 (2016)

    Article  Google Scholar 

  32. Kaszlikowski, D., Gnaciski, P., ukowski, M., Miklaszewski, W., Zeilinger, A.: Violations of local realism by two entangled N-dimensional systems are stronger than for two qubits. Phys. Rev. Lett. 85(21), 4418 (2000)

    Article  ADS  Google Scholar 

  33. Collins, D., Gisin, N., Linden, N., Massar, S., Popescu, S.: Bell inequalities for arbitrarily high-dimensional systems. Phys. Rev. Lett. 88(4), 040404 (2002)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  34. Walborn, S.P., Lemelle, D.S., Almeida, M.P., Ribeiro, P.H.S.: Quantum key distribution with higher-order alphabets using spatially encoded qudits. Phys. Rev. Lett. 96(9), 090501 (2006)

    Article  ADS  Google Scholar 

  35. Bourennane, M., Karlsson, A., Bjrk, G.: Quantum key distribution using multilevel encoding. Phys. Rev. A 64(1), 012306 (2001)

    Article  ADS  Google Scholar 

  36. Da-Sheng, D., Cheng-Jie, Z., Yong-Sheng, Z., Guang-Can, G.U.O.: Class of unlockable bound entangled states and their applications, class of unlockable bound entangled states and their applications. Chin. Phys. Lett. 25(7), 2366 (2008)

    Article  ADS  Google Scholar 

  37. Horodecki, P., Horodecki, M., Horodecki, R.: Bound entanglement can be activated. Phys. Rev. Lett. 82(5), 1056 (1999)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  38. Cerf, N.J., Bourennane, M., Karlsson, A., Gisin, N.: Security of quantum key distribution using \(\mathit{d}\)-level systems. Phys. Rev. Lett. 88(12), 127902 (2002)

    Article  Google Scholar 

  39. Durt, T., Cerf, N.J., Gisin, N., Żukowski, M.: Security of quantum key distribution with entangled qutrits. Phys. Rev. A 67(1), 012311 (2003)

    Article  ADS  Google Scholar 

  40. Jafarpour, M.: An entanglement study of superposition of qutrit spin-coherent states. J. Sci. Islam. Repub. Iran 22(2), 165 (2011)

    Google Scholar 

  41. Ali, M.: Distillability sudden death in qutrit\(-\)qutrit systems under global and multilocal dephasing. Phys. Rev. A 81(4), 042303 (2010)

    Article  Google Scholar 

  42. Ali, M.: Distillability sudden death in qutrit-qutrit systems under amplitude damping. J. Phys. B: At. Mol. Opt. Phys. 43(4), 045504 (2010)

    Article  ADS  Google Scholar 

  43. Jafarpour, M., Ashrafpour, M.: Entanglement dynamics of a two-qutrit system under DM interaction and the relevance of the initial state. Quantum Inf. Process. 12(2), 761 (2013)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  44. Yang, Y., Wang, A.M.: Quantum discord for a qutrit\(-\)qutrit system under depolarizing and dephasing noise. Chin. Phys. Lett. 30(8), 080302 (2013)

    Article  Google Scholar 

  45. Li, X.J., Ji, H.H., Hou, X.W.: Thermal discord and negativity in a two-spin-qutrit system under different magnetic fields. Int. J. Quantum Inf. 11(08), 1350070 (2013)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  46. Doustimotlagh, N., Guo, J.L., Wang, S.: Quantum correlations in qutrit-qutrit systems under local quantum noise channels. Int. J. Theor. Phys. 54(6), 1784 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  47. Tsokeng, A.T., Tchoffo, M., Fai, L.C.: Quantum correlations and decoherence dynamics for a qutrit-qutrit system under random telegraph noise. Quantum Inf. Process. 16(8), 191 (2017)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  48. Arthur, T.T., Martin, T., Fai, L.C.: Quantum correlations and coherence dynamics in qutrit–qutrit systems under mixed classical environmental noises. Int. J. Quantum Inform. 15(06), 1750047 (2017)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  49. Horodecki, M., Horodecki, P., Horodecki, R.: Mixed-state entanglement and distillation: is there a bound entanglement in nature? Phys. Rev. Lett. 80(24), 5239 (1998)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  50. Horodecki, R., Horodecki, P., Horodecki, M., Horodecki, K.: Quantum entanglement. Rev. Mod. Phys. 81(2), 865 (2009)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  51. Smolin, J.A.: Four-party unlockable bound entangled state. Phys. Rev. A 63(3), 032306 (2001)

    Article  ADS  Google Scholar 

  52. Acin, A., Cirac, J.I., Masanes, L.: Multipartite bound information exists and can be activated. Phys. Rev. Lett. 92(10), 107903 (2004)

    Article  ADS  Google Scholar 

  53. Shor, P.W., Smolin, J.A., Thapliyal, A.V.: Superactivation of bound entanglement. Phys. Rev. Lett. 90(10), 107901 (2003)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  54. Horodecki, K., Horodecki, M., Horodecki, P., Oppenheim, J.: Secure key from bound entanglement. Phys. Rev. Lett. 94(16), 160502 (2005)

    Article  MathSciNet  MATH  ADS  Google Scholar 

  55. Murao, M., Vedral, V.: Remote information concentration using a bound entangled state. Phys. Rev. Lett. 86(2), 352 (2001)

    Article  ADS  Google Scholar 

  56. Ishizaka, S.: Bound entanglement provides convertibility of pure entangled states. Phys. Rev. Lett. 93(19), 190501 (2004)

    Article  ADS  Google Scholar 

  57. Song, W., Chen, L., Zhu, S.L.: Sudden death of distillability in qutrit-qutrit systems. Phys. Rev. A 80(1), 012331 (2009)

    Article  ADS  Google Scholar 

  58. Fujisawa, T., Hirayama, Y.: Charge noise analysis of an AlGaAs/GaAs quantum dot using transmission type radio-frequency single-electron transistor technique. Appl. Phys. Lett. 77(4), 543 (2000)

    Article  ADS  Google Scholar 

  59. Paladino, E., Faoro, L., Falci, G., Fazio, R.: Decoherence and \(1/\mathit{f}\) noise in Josephson qubits. Phys. Rev. Lett. 88(22), 228304 (2002)

    Article  Google Scholar 

  60. Rau, A.R.P., Ali, M., Alber, G.: Hastening, delaying, or averting sudden death of quantum entanglement. EPL 82(4), 40002 (2008)

    Article  ADS  Google Scholar 

  61. Ali, M., Alber, G., Rau, A.R.P.: Manipulating entanglement sudden death of two-qubit X-states in zero- and finite-temperature reservoirs. J. Phys. B: At. Mol. Opt. Phys. 42(2), 025501 (2009)

    Article  ADS  Google Scholar 

  62. Vidal, G., Werner, R.F.: Computable measure of entanglement. Phys. Rev. A 65(3), 032314 (2002)

    Article  ADS  Google Scholar 

  63. Chen, K., Wu, L.A.: A matrix realignment method for recognizing entanglement. arXiv:quant-ph/0205017 (2002)

  64. Rudolph, O.: Further results on the cross norm criterion for separability. Quantum Inf. Process. 4(3), 219 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  65. Clarisse, L.: Entanglement distillation; a discourse on bound entanglement in quantum information theory. arXiv:quant-ph/0612072 (2006)

  66. Vaziri, A., Weihs, G., Zeilinger, A.: Experimental twophoton, three-dimensional entanglement for quantum communication. Phys. Rev. Lett. 89(24), 240401 (2002)

    Article  ADS  Google Scholar 

  67. Thew, R.T., Acin, A., Zbinden, H., Gisin, N.: Experimental realization of entangled qutrits for quantum communication. arXiv:quant-ph/0307122 (2003)

  68. Gutierrez-Esparza, A.J., Pimenta, W.M., Marques, B., Matoso, A.A., Pdua, S.: Experimental characterization of two spatial qutrits using entanglement witnesses. Opt. Express 20(24), 26351 (2012)

    Article  ADS  Google Scholar 

  69. Jaeger, G., Ann, K.: Disentanglement and decoherence in a pair of qutrits under dephasing noise. J. Mod. Opt. 54(16–17), 2327 (2007)

    Article  ADS  Google Scholar 

  70. Xiao-San, M., Ming-Fan, R., Guang-Xing, Z., An-Min, W.: Dynamics of entanglement of qutrit-qutrit states with stochastic dephasing. Commun. Theor. Phys. 56(2), 258 (2011)

    Article  MATH  ADS  Google Scholar 

  71. Karpat, G., Gedik, Z.: Invariant quantum discord in qubit–qutrit systems under local dephasing. Phys. Scr. 2013(T153), 014036 (2013)

    Article  Google Scholar 

  72. Bergli, J., Galperin, Y.M., Altshuler, B.L.: Decoherence in qubits due to low-frequency noise. New J. Phys. 11(2), 025002 (2009)

    Article  ADS  Google Scholar 

  73. Bordone, P., Buscemi, F., Benedetti, C.: Effect of Markov and non-Markov classical noise on entanglement dynamics. Fluctuat. Noise Lett. 11(03), 1242003 (2012)

    Article  Google Scholar 

  74. Faoro, L., Ioffe, L.B.: Microscopic origin of low-frequency flux noise in Josephson circuits. Phys. Rev. Lett. 100(22), 227005 (2008)

    Article  ADS  Google Scholar 

  75. Buscemi, F., Bordone, P.: Time evolution of tripartite quantum discord and entanglement under local and nonlocal random telegraph noise. Phys. Rev. A 87(4), 042310 (2013)

    Article  ADS  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Mesoscopic and Multilayer Structures Laboratory, Department of Physics, Faculty of Science, University of Dschang, PO Box: 67, Dschang, Cameroon

    Tsamouo Tsokeng Arthur, Tchoffo Martin & Lukong Cornelius Fai

Authors
  1. Tsamouo Tsokeng Arthur
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Tchoffo Martin
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Lukong Cornelius Fai
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Tsamouo Tsokeng Arthur.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Arthur, T.T., Martin, T. & Fai, L.C. Disentanglement and quantum states transitions dynamics in spin-qutrit systems: dephasing random telegraph noise and the relevance of the initial state. Quantum Inf Process 17, 37 (2018). https://doi.org/10.1007/s11128-017-1800-y

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-017-1800-y

Keywords

Search

Navigation