Skip to main content
SpringerLink
Log in

Controlled teleportation with the control of two groups of agents via entanglement

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

    We’re sorry, something doesn't seem to be working properly.

    Please try refreshing the page. If that doesn't work, please contact support so we can address the problem.

Abstract

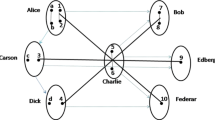
We present a way for implementing controlled teleportation of an arbitrary unknown pure state of a qutrit with the control of two groups of agents via entanglement. In our proposal, the sender can successfully teleport the qutrit state to a distant receiver with the help of all agents. However, if one agent in each group does not cooperate, the receiver cannot gain any information (including amplitude information or phase information or both) about the qutrit state to be teleported. Since a qubit is a special case of a qutrit when the state lies in a fixed two-dimensional subspace of the qutrit, the present proposal can be also applied in the implementation of controlled teleportation of an arbitrary unknown pure state of a qubit with many control agents in two groups. We note that our proposal is the first one to use two groups of agents to achieve controlled teleportation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Bennett, C.H., Brassard, G., Crepeau, C., Jozsa, R., Peres, A., Wootters, W.K.: Teleporting an unknown quantum state via dual classical and Einstein–Podolsky–Rosen channels. Phys. Rev. Lett 70, 1895 (1993)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  2. Fujii, M.: Continuous-variable quantum teleportation with a conventional laser. Phys. Rev. A 68, 050302(R) (2003)

    Article  ADS  Google Scholar 

  3. An, N.B.: Teleportation of coherent-state superpositions within a network. Phys. Rev. A 68, 022321 (2003)

    Article  ADS  Google Scholar 

  4. Bowen, W.P., Treps, N., Buchler, B.C., Schnabel, R., Ralph, T.C., Bachor, H.A., Symul, T., Lam, P.K.: Experimental investigation of continuous-variable quantum teleportation. Phys. Rev. A 67, 032302 (2003)

    Article  ADS  Google Scholar 

  5. Johnson, T.J., Bartlett, S.D., Sanders, B.C.: Continuous-variable quantum teleportation of entanglement. Phys. Rev. A 66, 042326 (2002)

    Article  ADS  Google Scholar 

  6. Fang, J., Lin, Y., Zhu, S., Chen, X.: Probabilistic teleportation of a three-particle state via three pairs of entangled particles. Phys. Rev. A 67, 014305 (2003)

    Article  ADS  Google Scholar 

  7. Son, W., Lee, J., Kim, M.S., Park, Y.J.: Conclusive teleportation of a d-dimensional unknown state. Phys. Rev. A 64, 064304 (2001)

    Article  ADS  Google Scholar 

  8. Galvao, E.F., Hardy, L.: Building multiparticle states with teleportation. Phys. Rev. A 62, 012309 (2000)

    Article  ADS  MathSciNet  Google Scholar 

  9. Yang, C.P., Guo, G.C.: A Proposal of teleportation for three-particle entangled state. Chin. Phys. Lett. 16, 628 (1999)

    Article  ADS  Google Scholar 

  10. Gordon, G., Rigolin, G.: Generalized teleportation protocol. Phys. Rev. A 73, 042309 (2006)

    Article  ADS  Google Scholar 

  11. Bouwmeester, D., Pan, J.W., Mattle, Kl, Eibl, M., Weinfurter, H., Zeilinger, A.: Experimental quantum teleportation. Nature (London) 390, 575 (1997)

    Article  ADS  Google Scholar 

  12. Furusawa, A., Sørensen, J.L., Braunstein, S.L., Fuchs, C.A., Kimble, H.J., Polzik, E.S.: Unconditional quantum teleportation. Science 282, 706 (1998)

    Article  ADS  Google Scholar 

  13. Lee, N., Benichi, H., Takeno, Y., Takeda, S., Webb, J., Huntington, E., Furusawa, A.: Teleportation of nonclassical wave packets of light. Science 332, 330 (2011)

    Article  ADS  Google Scholar 

  14. Nielsen, M.A., Knill, E., Laflamme, R.: Complete quantum teleportation using nuclear magnetic resonance. Nature (London) 396, 52 (1998)

    Article  ADS  Google Scholar 

  15. Barrett, M.D., Chiaverini, J., Schaetz, T., Britton, J., Itano, W.M., Jost, J.D., Knill, E., Langer, C., Leibfried, D., Ozeri, R., Wineland, D.J.: Deterministic quantum teleportation of atomic qubits. Nature (London) 429, 737 (2004)

    Article  ADS  Google Scholar 

  16. Olmschenk, S., Matsukevich, D.N., Maunz, P., Hayes, D., Duan, L.-M., Monroe, C.: Quantum teleportation between distant matter qubits. Science 323, 486 (2009)

    Article  ADS  Google Scholar 

  17. Jin, X.M., Ren, J.G., Yang, B., Yi, Z.H., Zhou, F., Xu, X.F., Wang, S.K., Yang, D., Hu, Y.F., Jiang, S., Yang, T., Yin, H., Chen, K., Peng, C.Z., Wei Pan, J.: Experimental free-space quantum teleportation. Nat. Photonics 87, 376 (2010)

    Article  ADS  Google Scholar 

  18. Yin, J., et al.: Quantum teleportation and entanglement distribution over 100-kilometre free-space channels. Nature (London) 488, 185 (2012)

  19. Karlsson, A., Bourennane, M.: Quantum teleportation using three-particle entanglement. Phys. Rev. A 58, 4394 (1998)

    Article  ADS  MathSciNet  Google Scholar 

  20. Hillery, M., Buzek, V., Berthiaume, A.: Quantum secret sharing. Phys. Rev. A 59, 1829 (1999)

    Article  ADS  MathSciNet  Google Scholar 

  21. Deng, F.G., Li, X.H., Li, C.Y., Zhou, P., Zhou, H.Y.: Multiparty quantum-state sharing of an arbitrary two-particle state with Einstein–Podolsky–Rosen pairs. Phys. Rev. A 72, 044301 (2005)

    Article  ADS  Google Scholar 

  22. Zheng, S.B.: Splitting quantum information via W states. Phys. Rev. A 74, 054303 (2006)

    Article  ADS  Google Scholar 

  23. Muralidharan, S., Panigrahi, P.K.: Quantum-information splitting using multipartite cluster states. Phys. Rev. A 78, 062333 (2008)

    Article  ADS  Google Scholar 

  24. Nie, Y.Y., Li, Y.H., Liu, J.C., Sang, M.H.: Quantum state sharing of an arbitrary three-qubit state by using four sets of W-class states. Opt. Commun. 284, 1457 (2011)

    Article  ADS  Google Scholar 

  25. Tittel, W., Zbinden, H., Gisin, N.: Experimental demonstration of quantum secret sharing. Phys. Rev. A 63, 042301 (2001)

    Article  ADS  Google Scholar 

  26. Bogdanski, J., Rafiei, N., Bourennane, M.: Experimental quantum secret sharing using telecommunication fiber. Phys. Rev. A 78, 062307 (2008)

    Article  ADS  Google Scholar 

  27. Gaertner, S., Kurtsiefer, C., Bourennane, M., Weinfurter, H.: Experimental demonstration of four-Party quantum secret sharing. Phys. Rev. Lett. 98, 020503 (2007)

    Article  ADS  Google Scholar 

  28. Yang, C.P., Chu, S.I., Han, S.: Efficient many-party controlled teleportation of multiqubit quantum information via entanglement. Phys. Rev. A 70, 022329 (2004)

    Article  ADS  Google Scholar 

  29. Yang, C.P., Han, S.: A scheme for the teleportation of multiqubit quantum information via the control of many agents in a network. Phys. Lett. A 343, 267 (2005)

    Article  ADS  MATH  Google Scholar 

  30. Deng, F.G., Li, C.Y., Li, Y.S., Zhou, H.Y., Wang, Y.: Symmetric multiparty-controlled teleportation of an arbitrary two-particle entanglement. Phys. Rev. A 72, 022338 (2005)

    Article  ADS  Google Scholar 

  31. Man, Z.X., Xia, Y.J., An, N.B.: Genuine multiqubit entanglement and controlled teleportation. Phys. Rev. A 75, 052306 (2007)

    Article  ADS  Google Scholar 

  32. Li, X.H., Deng, F.G., Zhou, H.Y.: Controlled teleportation of an arbitrary multi-qudit state in a general form with d-dimensional Greenberger–Horne–Zeilinger States. Chin. Phys. Lett. 24, 1151 (2007)

    Article  ADS  Google Scholar 

  33. Liu, J.C., Li, Y.H., Nie, Y.Y.: Controlled teleportation of an arbitrary two-particle pure or mixed state by using a five-qubit cluster state. Int. J. Theor. Phys. 49, 1976 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  34. Shi, R.H., Huang, L.S., Yang, W., Zhong, H.: Controlled quantum perfect teleportation of multiple arbitrary multi-qubit states. Chin. Phys. 54(12), 2208 (2011)

    Google Scholar 

  35. Wang, T.Y., Wen, Q.Y.: Controlled quantum teleportation with Bell states. Chin. Phys. B 20, 040307 (2011)

    Article  ADS  Google Scholar 

  36. Guo, Z.Y., Shang, X.X., Fang, J.X., Xiao, R.H.: Controlled teleportation of an arbitrary two-particle state by one EPR pair and cluster state. Commun. Theor. Phys. 56, 819 (2011)

    Article  ADS  MATH  Google Scholar 

  37. Li, Z., Long, L.R., Zhou, P., Yin, C.L.: Probabilistic multiparty-controlled teleportation of an arbitrary m-qubit state with a pure entangled quantum channel against collective noise. Sci. China Phys. 55, 2445 (2012)

    Article  Google Scholar 

  38. Han, L.F., Xu, H.F.: Probabilistic and controlled teleportation of an arbitrary two-qubit state via one dimensional five-qubit cluster-class state. Int. J. Theor. Phys. 51, 2540 (2012)

    Article  MATH  Google Scholar 

  39. Zha, X.W., Zou, Z.C., Qi, J.X., Song, H.Y.: Bidirectional quantum controlled teleportation via five-qubit cluster state. Int. J. Theor. Phys. 52, 1740 (2013)

    Article  MathSciNet  Google Scholar 

  40. Yan, A.: Bidirectional controlled teleportation via six-qubit cluster state. Int. J. Theor. Phys. 52, 3870 (2013)

    Article  MATH  Google Scholar 

  41. Peng, Z.H., Zou, J., Liu, X.J., Kuang, L.M.: Construction of general quantum channel for quantum teleportation. Quantum Inf. Process. 12, 2803 (2013)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  42. Zhan, Y.B.: Controlled teleportation of high-dimension quantum-states with generalized Bell-state measurement. Chin. Phys. B 16, 2557 (2008)

    Google Scholar 

  43. Cao, H.J., Chen, Z.H., Song, H.S.: Controlled teleportation of a 3-dimensional bipartite quantum state. Phys. Scr. 78, 015002 (2008)

    Article  ADS  MathSciNet  Google Scholar 

  44. Dai, H.Y., Zhang, M., Kuang, L.M.: Teleportation of the three-level three-particle entangled state and classical communication cost. Phys. A 387, 3811 (2008)

    Article  Google Scholar 

  45. Shi, J., Zhan, Y.B.: Controlled probabilistic teleportation of an unknown multi-particle high-dimensional entangled state. Commun. Theor. Phys. 51, 1027 (2009)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  46. Zhang, Z.Y., Sun, Q.: Realization of superposition and entanglement of coherent and squeezed states in circuit quantum electrodynamics. Sci. China Phys. Mech. Astron. 54, 1476 (2011)

    Article  ADS  Google Scholar 

  47. Li, X.H., Zhou, P., Li, C.Y., Zhou, H.Y., Deng, F.G.: Efficient symmetric multiparty quantum state sharing of an arbitrary m-qubit state. J. Phys. B 39, 1975 (2006)

    Article  ADS  Google Scholar 

  48. Zhou, P., Li, X.H., Deng, F.G., Zhou, H.Y.: Multiparty-controlled teleportation of an arbitrary m-qudit state with a pure entangled quantum channel. J. Phys. A Math. Theor. 40, 13121 (2007)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  49. Dong, J., Teng, J.F.: Controlled teleportation of an arbitrary n-qudit state using nonmaximally entangled GHZ states. Eur. Phys. J. D 49, 129 (2008)

    Article  ADS  Google Scholar 

  50. Xia, Y., Song, J., Song, H.S., Wang, B.Y.: Generalized Teleportation of a d-Level N-Particle GHZ State with one pair of entangled particles as the quantum channel. Int. J. Theor. Phys. 47, 2835 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  51. Wang, J., Hou, K., Yuan, H., Shi, S.H.: An efficient scheme for generalized tripartite controlled teleportation of a two-qubit entangled state. Phys. Scr. 80, 015004 (2009)

    Article  ADS  Google Scholar 

  52. Zuo, X.Q., Liu, Y.M., Xu, C.J., Zhang, Z.Y., Zhang, Z.J.: Generalized tripartite scheme for sharing arbitrary 2n-qudit state. Opt. Commun. 283, 4108 (2010)

    Article  ADS  Google Scholar 

  53. Long, L.R., Li, H.W., Zhou, Pi, Fan, C., Yin, C.L.: Multiparty-controlled teleportation of an arbitrary GHZ-class state by using a d-dimensional (N+2)-particle nonmaximally entangled state as the quantum channel. Sci. China Phys. Mech. Astron. 54, 484 (2011)

    Article  ADS  Google Scholar 

Download references

Acknowledgments

X.L.H. acknowledges the funding support from the Zhejiang Natural Science Foundation under Grant No. LY12A04008. C.P.Y. was supported in part by the National Natural Science Foundation of China under Grant Nos. 11074062 and 11374083, the Zhejiang Natural Science Foundation under Grant No. LZ13A040002, and the funds from Hangzhou Normal University under Grant Nos. HSQK0081 and PD13002004. This work was also supported by the funds from Hangzhou City for the Hangzhou-City Quantum Information and Quantum Optics Innovation Research Team.

Author information

Authors and Affiliations

  1. School of Science, Zhejiang University of Science and Technology, Hangzhou, 310023, Zhejiang, China

    Xiao-Ling He

  2. Department of Physics, Hangzhou Normal University, Hangzhou, 310036, Zhejiang, China

    Man Liu & Chui-Ping Yang

Authors
  1. Xiao-Ling He
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Man Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Chui-Ping Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Chui-Ping Yang.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

He, XL., Liu, M. & Yang, CP. Controlled teleportation with the control of two groups of agents via entanglement. Quantum Inf Process 14, 1055–1068 (2015). https://doi.org/10.1007/s11128-014-0894-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11128-014-0894-8

Keywords

Search

Navigation