Abstract
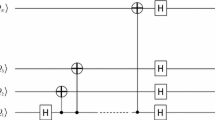
We present a three-party quantum secret sharing (QSS) scheme via the entangled Greenberger–Horne–Zeilinger state. In this scheme, the sender Alice encodes her arbitrary secret information by means of preparing a single-particle quantum state. The agent Bob obtains his shared information according to his hobby, while Charlie can easily calculate his shared information. The proposed scheme is secure. It is shown that even a dishonest agent, who may avoid the security checking, cannot obtain any useful information. Moreover, we further investigate the multi-party QSS scheme which allows most agents to predetermine their information.
Similar content being viewed by others
References
Bennett, C.H., Brassard, G.: Quantum cryptography: public-key distribution and coin tossing. In: IEEE International Conference on Computers, Systems and Signal Processing, pp. 175–179 (1984)
Chen X.B., Wang T.Y., Du J.Z., Wen Q.Y., Zhu F.C.: Controlled quantum secure direct communication with quantum encryption. Int. J. Quant. Inform. 6, 543–551 (2008)
Chen X.B., Wen Q.Y., Guo F.Z., Sun Y., Xu G., Zhu F.C.: Controlled quantum secure direct communication with w state. Int. J. Quant. Inform. 6, 899–906 (2008)
Chong S.K., Hwang T.: The enhancement of three-party simultaneous quantum secure direct communication scheme with epr pairs. Opt. Commun. 284, 515–518 (2011)
Bennett C.H., Brassard G., Crepeau C., Jozsa R., Peres A., Wootters W.K.: Teleporting an unknown quantum state via dual classical and einstein-podolsky-rosen channels. Phys. Rev. Lett. 70, 1895–1899 (1993)
Chen X.B., Wen Q.Y., Zhu F.C.: Probabilistic teleportation of a non-symmetric three-particle state. Chin. Phys. 15, 2240–2245 (2006)
Chen X.B., Zhang N., Lin S., Wen Q.Y., Zhu F.C.: Quantum circuits for controlled teleportation of two-particle entanglement via a w state. Opt. Commun. 281, 2331–2335 (2008)
Chen X.B., Wen Q.Y., Xu G., Yang Y.X., Zhu F.C.: Comment on “General relation between the transformation operator and an invariant under stochastic local operations and classical communication in quantum teleportation”. Phys. Rev. A 79, 036301 (2009)
Chen, X.B., Ma, S.Y., Su, Y., Zhang, R., Yang, Y.X.: Controlled remote state preparation of an arbitrary two and three qubit states via the brown state. Quantum Inf. Process (Online). doi:10.1007/s11128-11011-10326-y (2012)
Hillery M., Buzek V., Berthiaume A.: Quantum secret sharing. Phys. Rev. A 59, 1829–1834 (1999)
Cleve R., Gottesman D., Lo H.K.: How to share a quantum secret. Phys. Rev. Lett. 83, 648–651 (1999)
Karimipour V., Bahraminasab A., Bagherinezhad S.: Entanglement swapping of generalized cat states and secret sharing. Phys. Rev. A 65, 042320 (2002)
Zhang Z.J., Man Z.X.: Multiparty quantum secret sharing of classical messages based on entanglement swapping. Phys. Rev. A 72, 022303 (2005)
Markham D., Sanders B.C.: Graph states for quantum secret sharing. Phys. Rev. A 78, 042309 (2008)
Li Q., Long D.Y., Chan W.H., Qiu D.W.: Sharing a quantum secret without a trusted party. Quantum Inf. Process. 10, 97–106 (2011)
Gaertner S., Kurtsiefer C., Bourennane M., Weinfurter H.: Experimental demonstration of four-party quantum secret sharing. Phys. Rev. Lett. 98, 020503 (2007)
Bogdanski J., Rafiei N., Bourennane M.: Experimental quantum secret sharing using telecommunication fiber. Phys. Rev. A 78, 062307 (2008)
Shi R.H., Huang L.S., Yang W., Zhong H.: Multi-party quantum state sharing of an arbitrary two-qubit state with bell states. Quantum Inf. Process. 10, 231–239 (2011)
Zhang Z.J., Li Y., Man Z.X.: Multiparty quantum secret sharing. Phys. Rev. A 71, 044301 (2005)
Qin S.J., Gao F., Wen Q.Y., Zhu F.C.: Improving the security of multiparty quantum secret sharing against an attack with a fake signal. Phys. Lett. A 357, 101–103 (2006)
Sun Y., Wen Q.Y., Gao F., Chen X.B., Zhu F.C.: Multiparty quantum secret sharing based on bell measurement. Opt. Commun. 282, 3647–3651 (2009)
Shi R., Huang L.S., Yang W., Zhong H.: Multiparty quantum secret sharing with bell states and bell measurements. Opt. Commun. 283, 2476–2480 (2010)
Lin S., Wen Q.Y., Qin S.J., Zhu F.C.: Multiparty quantum secret sharing with collective eavesdropping-check. Opt. Commun. 282, 4455–4459 (2009)
Gao G.: Cryptanalysis of multiparty quantum secret sharing with collective eavesdropping-check. Opt. Commun. 283, 2997–3000 (2010)
Zhang Z.: Robust multiparty quantum secret key sharing over two collective-noise channels. Phys. A: Stat. Mech. Appl. 361, 233–238 (2006)
Chen X.B., Xu G., Niu X.X., Wen Q.Y., Yang Y.X.: An efficient protocol for the private comparison of equal information based on the triplet entangled state and single-particle measurement. Opt. Commun. 283, 1561–1565 (2010)
Sun Y., Wen Q.y., Zhu F.C.: Improving the multiparty quantum secret sharing over two collective-noise channels against insider attack. Opt. Commun. 283, 181–183 (2010)
Gan G.: Multiparty quantum secret sharing using two-photon three-dimensional bell states. Commun. Theor. Phys. 52, 421–424 (2009)
Deutsch D., Ekert A., Jozsa R., Macchiavello C., Popescu S., Sanpera A.: Quantum privacy amplification and the security of quantum cryptography over noisy channels. Phys. Rev. Lett. 77, 2818–2821 (1996)
Shor P.W., Preskill J.: Simple proof of security of the bb84 quantum key distribution protocol. Phys. Rev. Lett. 85, 441–444 (2000)
Wang T.Y., Wen Q.Y., Chen X.B., Guo F.Z., Zhu F.C.: An efficient and secure multiparty quantum secret sharing scheme based on single photons. Opt. Commun. 281, 6130–6134 (2008)
Shannon C.E.: Communication theory of secret systems. Bell Syst. Tech. J. 28, 656–715 (1949)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Chen, XB., Niu, XX., Zhou, XJ. et al. Multi-party quantum secret sharing with the single-particle quantum state to encode the information. Quantum Inf Process 12, 365–380 (2013). https://doi.org/10.1007/s11128-012-0379-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11128-012-0379-6