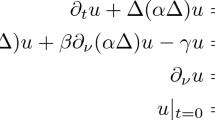Abstract
In this note, we concentrate on the sub-Laplace operator on the nilpotent Lie group of rank two, which is the infinitesimal generator of the diffusion generated by n Brownian motions and their \(\frac{n(n-1)}2\) Lévy area processes, which is the simple extension of the sub-Laplacian on the Heisenberg group ℍ. In order to study contraction properties of the associated heat kernel, we show that, as in the cases of the Heisenberg group and the three Brownian motions model, the restriction of the sub-Laplace operator acting on radial functions (see Definition 3.5) satisfies a positive Ricci curvature condition (more precisely a CD(0, ∞ ) inequality), see Theorem 4.5, whereas the operator itself does not satisfy any CD(r, ∞ ) inequality. From this we may deduce some useful, sharp gradient bounds for the associated heat kernel. It can be seen a generalization of the paper (Qian, Bull Sci Math 135:262–278, 2011).
Similar content being viewed by others
References
Bakry D.: On Sobolev and logarithmic Sobolev inequalities for Markov semigroups. Taniguchi symposium. New trends in stochastic analysis (Charingworth, 1994), World Sci. Publ. River Edge, NJ, 1997: 43-75.
Bakry, D., Baudoin, F., Bonnefont, M., Chafaï, D.: On gradient bounds for the heat kernel on the Heisenberg group. J. Funct. Anal. 255, 1905–1938 (2008)
Bakry, D., Baudoin, F., Bonnefont, M., Qian, B.: Subelliptic Li-Yau estimates on three dimensional model spaces. Potential Theory and Stochastics in Albac: Aurel Cornea Memorial Volume (2009)
Baudoin, F., Bonnefont, M.: The subelliptic heat kernel on SU(2): representations, asymptotics and gradient bounds. Math. Zeit. 263, 647–672 (2009)
Baudoin, F., Bonnefont, M.: Log-Sobolev inequalities for subelliptic operators satisfying a generalized curvature dimension inequality. J. Funct. Anal. 262(6), 2646–2676 (2012)
Baudoin, F., Garofalo, N.: Curvature-dimension inequalities and Ricci lower bounds for sub-Riemannian manifolds with transverse symmetries. Arxiv:1101.3590
Beals, R., Gaveau B., Greiner, P.C.: Hamilton-Jacobi theory and the heat kernel on Heisenberg groups. J. Math. Pures Appl. 79(9), 633–689 (2000)
Driver, B.K., Melcher, T.: Hypoelliptic heat kernel inequalities on the Heisenberg group. J. Funct. Anal. 221(2), 340–365 (2005)
Engoulatov, A.: A universal bound on the gradient of logtithm of the heat kernel for manifolds with bounded Ricci curvature. J. Funct. Anal. 238, 518–529 (2006)
Gaveau B.: Principe de moindre action, propagation de la chaleur et estimees souselliptiques sur certains groupes nilpotents. Acta Math. 139 95–153 (1977)
Hu, J.Q., Li, H.Q.: Gradient estimates for the heat semigroup on H-type groups. Potential Anal. 33, 355–386 (2010)
Juillet, N.: Geometric inequalities and generalized Ricci bounds on the Heisenberg group. Int. Math. Res. Not. 13, 2347–2373 (2009)
Ledoux, M.: The geometric of Markov diffusion geretators, probability theroy. Ann. Fac. Sci. Toulouse Math. 9(6), 305–366 (2000)
Li, H.Q.: Estimation optimale du gradient du semi-groupe de la chaleur sur le groupe de Heisenberg. J. Funct. Anal. 236, 369–394 (2006)
Li, H.Q.: Esimations opitmale du noyau de la chaleur sur les groupes de Heisenberg. CRAS Ser. I 497–502 (2007)
Li, H.Q.: Estimations optimales du noyau de la chaleur sur les groupes de type Heisenberg. J. Reine Angew. Math. 646, 195–233 (2010)
Li, H.Q.: Estimations asymptotiques du noyau de la chaleur pour l’operateur de Grushin. Comm. P. D. E. 37, 794–832 (2012)
Li, P., Yau, S.T.: On the parabolic kernel of the Schrödinger operator. Acta. Math. 156, 153–201 (1986)
Li, X.D.: Perelman’s entropy formula for theWitten Laplacian on Riemanian manifolds via Bakry-Emery Ricci curvature. Math. Ann. 353, 403–437 (2012)
Melcher, T.: Hypoelliptic heat kernel inequalities on Lie groups. Stoch. Proc. Anal. 118(3), 368–388 (2008)
Melcher, T.: Hypoelliptic heat kernel inequalities on Lie groups. Ph.D. thesis, UC San Diego, pp. 120 (2004)
Qian, B.: Gradient estimates for the heat kernels in the high dimensional Heisenberg groups. Chin. Ann. Math. 31B(3), 305–314 (2010)
Qian, B.: Positive curvature for some hypoelliptic operators. Bull. Sci. Math. 135, 262–278 (2011)
Varopolous, N.Th., Saloff-Coste, L., Coulhon, Th.: Analysis and Geometry on Groups. Cambridge Tracts in Mathematics 100, Cambridge University Press (1992)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Qian, B. Positive Curvature Property for Sub-Laplacian on Nilpotent Lie Group of Rank Two. Potential Anal 39, 325–340 (2013). https://doi.org/10.1007/s11118-013-9332-2
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11118-013-9332-2