Abstract
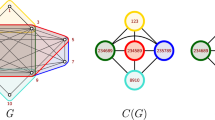
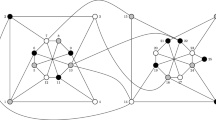
We introduce the notion of a properly ordered coloring (POC) of a weighted graph, that generalizes the notion of vertex coloring of a graph. Under a POC, if xy is an edge, then the larger weighted vertex receives a larger color; in the case of equal weights of x and y, their colors must be different. In this paper, we shall initiate the study of this special coloring in graphs. For a graph G, we introduce the function f(G) which gives the maximum number of colors required by a POC over all weightings of G. We show that f(G) = ℓ(G), where ℓ(G) is the number of vertices of a longest path in G. Another function we introduce is χPOC(G; t) giving the minimum number of colors required over all weightings of G using t distinct weights. We show that the ratio of χPOC(G; t) − 1 to χ(G) − 1 can be bounded by t for any graph G; in fact, the result is shown by determining χPOC(G; t) when G is a complete multipartite graph. We also determine the minimum number of colors to give a POC on a vertex-weighted graph in terms of the number of vertices of a longest directed path in an orientation of the underlying graph. This extends the so called Gallai-Hasse-Roy-Vitaver theorem, a classical result concerning the relationship between the chromatic number of a graph G and the number of vertices of a longest directed path in an orientation of G.
Similar content being viewed by others
Data Availability
Data sharing not applicable to this article as no datasets were generated or analysed during the current study.
References
Chaitin, G.J.: Register allocation & spilling via graph colouring. In: Proceedings of the 1982 SIGPLAN Symposium on Compiler Construction, pp 98–105 (1982)
Gallai, T.: On directed graphs and circuits. In: Theory of Graphs (Proceedings of the Colloquium held at Tihany 1966), pp 115–118 (1968)
Hasse, M.: Zur algebraischen Begründung der Graphentheorie. I. Math. Nachr. (in German) 28, 275–290 (1965)
Holzer, C.: The future of polymer processing. POLIMERI 32(3–4), 124–129 (2011)
Kishimoto, A., Buesser, B., Botea, A.: AI meets chemistry. In: The Thirty-Second AAAI Conference on Artificial Intelligence (AAAI-18), pp 7978–7982 (2018)
Malaguti, E.: The Vertex Coloring Problem and its Generalizations. PhD thesis, Universita Degli Studi di Bologna (2009)
Malaguti, E., Toth, P.: A survey on vertex coloring problems. Int. Trans. Oper. Res. 17, 1–34 (2010)
Marx, D.: Graph colouring problems and their applications in scheduling. Period. Polytech. Electr. Eng. 48(1–2), 11–16 (2004)
Roy, B.: Nombre chromatique et plus longs chemins d’un graphe. Rev. Fr. Inf. Rech. Opér. (in French) 1, 129–132 (1967)
Schweidtmanna, A.M., Clayton, A.D., Holmes, N., Bradforda, E., Bournec, R.A., Lapkina, A.A.: Machine learning meets continuous flow chemistry: automated optimization towards the Pareto front of multiple objectives. Chem. Eng. J. 352, 277–282 (2018)
Spalding, M.A., Chatterjee, A.M.: Handbook of Industrial Polyethylene and Technology: Definitive Guide to Manufacturing, Properties, Processing, Applications and Markets. Wiley, New York (2017)
Vitaver, L.M.: Determination of minimal coloring of vertices of a graph by means of Boolean powers of the incidence matrix. Dokl. Akad. Nauk. SSSR 147, 758–759 (in Russian) (1962)
Acknowledgments
The authors would like to thank the referees for carefully reading our article and for many helpful comments. The first author’s research was supported by JSPS KAKENHI (19K03603).
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Fujita, S., Kitaev, S., Sato, S. et al. On Properly Ordered Coloring of Vertices in a Vertex-Weighted Graph. Order 38, 515–525 (2021). https://doi.org/10.1007/s11083-021-09554-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11083-021-09554-7