A polynomial model of transmission and reflection of electromagnetic monochromatic plane waves in lossless, non-magnetic multilayer thin films subjected to an external transverse voltage

Abstract

A polynomial approach is adopted to simulate the optical-electrical response of a multilayer thin film subjected to an applied transverse voltage. Each layer in the thin film is modelled by a propagation matrix, while the coupling between any two adjacent layers at an interface is modelled by an interface matrix. Each layer in the multilayer thin film in the present approach is treated as a capacitor coupled to the next so that the multilayer film is modelled as an effective capacitor which is constructed by a series of coupled capacitors. The electric charges accumulated at the interface between adjacent ‘capacitors’ can be modulated by the transverse voltage. The model is constructed to describe only lossless and nonmagnetic materials. The reflection and transmission of multilayer MgO/CaF₂ thin films with a selected number of layers and structure, subjected to a tunable transverse potential, are simulated with a home-grown code implementing the model. The numerical results indicate that non-trivial optical responses can be conveniently predicted with the code, providing a handy simulator for designing and optimizing desired optical functionality of an arbitrary lossless multilayer thin film by tuning the transverse potential and the geometrical and electrical parameter of the structure.

Appendix

1.1 Derivation of Fresnel’s coefficients of polarized EM waves for both TM and TE modes in a lossless, non-magnetic medium

To begin with, Maxwell’s equations for a general plane wave in a homogeneous medium can be written in the frequency domain as the following (Markos and Soukoulis 2008),

$$\overrightarrow{k}\times \overrightarrow{E}=\frac{\mu \omega }{c}\overrightarrow{H},$$

(36)

$$\overrightarrow{k}\times \overrightarrow{H}=-\frac{\varepsilon \omega }{c}\overrightarrow{E},$$

(37)

$$\overrightarrow{k}\cdot \overrightarrow{E}=0,$$

(38)

$$\overrightarrow{k}\cdot \overrightarrow{H}=0,$$

(39)

where $\overrightarrow{k}$ is the wave vector of the EM fields, $\overrightarrow{E}$ is the electrical field, $\overrightarrow{H}$ is the magnetic field, $\omega$ and $c$ are the angular frequency and the speed of light respectively; $\mu$ and $\varepsilon$ are the permeability and permittivity of the medium respectively. Note that in general, $\mu$ and $\varepsilon$ are complex. $\eta$, which is defined as the intrinsic impedance of the medium via $\eta =\sqrt{\mu /\varepsilon }$, can be related to the electromagnetic fields for planes waves propagates through a homogeneous medium via $\eta =\overrightarrow{E}/\overrightarrow{H}$. The ratio $\overrightarrow{E}/\overrightarrow{H}$ is also known as the wave impedance, but for a transverse-electric–magnetic (TEM) plane wave travelling through a homogeneous medium, the ratio is equal to the intrinsic impedance of the medium (Pozar 2012).

The configuration of two adjacent semi-infinite dielectric slabs is illustrated in Fig. 11, where the z = 0 (the x−y plane) represents the interface between medium 1 (z < 0) and medium 2 (z > 0). Consider a polarized electromagnetic wave incident from media 1 to the interface with an angle of incidence ${\theta }_{i}$. The incident, transmitted and reflected fields are denoted with subscripts ‘i’, ‘t’ and ‘r’ respectively. These fields can be expressed in the form of (Markos and Soukoulis 2008)

$${\overrightarrow{E_{i}}}\left(\overrightarrow{r},t\right)={\overrightarrow{E_{oi}}}{e}^{\mathrm{i}({\overrightarrow{k_{i}}}\cdot \overrightarrow{r} -\omega t)},$$

(40)

$${\overrightarrow{E_{t}}}\left(\overrightarrow{r},t\right)={\overrightarrow{E_{ot}}}{e}^{\mathrm{i}({\overrightarrow{k_{t}}}\cdot \overrightarrow{r}-\omega t)},$$

(41)

$${\overrightarrow{E_{r}}}\left(\overrightarrow{r},t\right)={\overrightarrow{E_{or}}}{e}^{\mathrm{i}({\overrightarrow{k_{r}}}\cdot \overrightarrow{r}-\omega t)},$$

(42)

$${\overrightarrow{H_{i}}}\left(\overrightarrow{r},t\right)={\overrightarrow{H_{oi}}}{e}^{\mathrm{i}({\overrightarrow{k_{i}}}\cdot \overrightarrow{r}-\omega t),}$$

(43)

$${\overrightarrow{H_{t}}}\left(\overrightarrow{r},t\right)={\overrightarrow{H_{ot}}}{e}^{\mathrm{i}\left({\overrightarrow{k_{t}}}\cdot \overrightarrow{r}-\omega t\right)},$$

(44)

$${\overrightarrow{H_{r}}}\left(\overrightarrow{r},t\right)={\overrightarrow{H_{or}}}{e}^{\mathrm{i}({\overrightarrow{k_{r}}}\cdot \overrightarrow{r}-\omega t)},$$

(45)

where ${\overrightarrow{E_{oi}}}$, ${\overrightarrow{E_{ot}}}$, ${\overrightarrow{E_{or}}}$ (${\overrightarrow{H_{oi}}}$, ${\overrightarrow{H_{ot}}}$, ${\overrightarrow{H_{or}}}$) are vectors which define the amplitudes and directions of the incident, transmitted, and reflected electric (magnetic) fields $. \overrightarrow{r}=x\widehat{x}+y\widehat{y}+z\widehat{z}$ is a position vector denoting spatial location, ${\overrightarrow{k_{i}}}$, ${\overrightarrow{k_{t}}}$, ${\overrightarrow{k_{r}}}$ are the wave vectors of the incident, transmitted and reflected fields respectively. In this “Appendix”, all wave vectors ${\overrightarrow{k_{\gamma }}},\gamma \in \{i,t,r\}$ are assumed real, i.e.$\varepsilon ={\varepsilon }^{*}$, and $\mu = \mu^{*} \Rightarrow {\vec{k}} = {\vec{k}}^{*}$ (Pozar 2012), so that the rest of the derivation in this “Appendix” applies to lossless materials only, unless otherwise specified. By referring to Fig. 11, $\left|{\overrightarrow{k_{i}}}\right|=\left|{\overrightarrow{k_{r}}}\right|={k_{1}}$,$\left|{\overrightarrow{k_{t}}}\right|={k}_{2}$, where ${{k}_{1}=2\pi /{\lambda }_{1},\;k}_{2}=2\pi /{\lambda }_{2}$ are the magnitude of the wave vectors in medium 1 and 2 respectively. Without loss of generality, the phase factor ${e}^{\mathrm{i}\omega t}$ in equations (Eqs. 40–45) will be switched off (by setting$t=0$) from this point onwards to ease the derivation of the transmission and reflection coefficients. The dropping of the factor ${e}^{\mathrm{i}\omega t}$ shall not cause any quantitative difference to the following analysis regarding the reflection and transmission of EM waves.

Now, let us consider the TM mode, which geometrical configuration is depicted in Fig. 11. In the TM mode, due to the translational invariance along the y-direction, the fields’ strength depends only on x and z but not on y. Hence, we write $\overrightarrow{E}=\overrightarrow{E}\left(x,z\right), \overrightarrow{H}=\overrightarrow{H}\left(x,z\right)$. The wave vector and electric field have only the x- and z-components but no y component, $\overrightarrow{k}={k}_{x}\widehat{x}+{k}_{z}\widehat{z}, \overrightarrow{E}={E}_{x}\widehat{x}+{E}_{z}\widehat{z}$, whereas the magnetic field has only y-component but no x- or z-components, $\overrightarrow{H}={H}_{y}\widehat{y}$.

The amplitude vector ${\overrightarrow{E_{oi}}}$ in Eq. (40) is resolved into ${\overrightarrow{E_{oi}}}={E}_{oi}\mathrm{cos}{\theta }_{i}\widehat{x}-{E}_{oi}\mathrm{sin}{\theta }_{i}\widehat{z}$. So is the wave vector, ${\overrightarrow{k_{i}}}={k}_{i}\mathrm{sin}{\theta }_{i}\widehat{x}+{k}_{i}\mathrm{cos}{\theta }_{i}\widehat{z}$ (see the geometry as illustrated in Fig. 11). Hence, by omitting the ${e}^{\mathrm{i}\omega t}$ terms, Eq. (40) becomes (Khorasani and Rashidian 2001)

$${\overrightarrow{E_{i}}}\left(x,z\right)={E}_{oi}\left(\mathrm{cos}{\theta }_{i}\widehat{x}-\mathrm{sin}{\theta }_{i}\widehat{z}\right){e}^{\mathrm{i}{k}_{1}\left(x\mathrm{sin}{\theta }_{i}+z\mathrm{cos}{\theta }_{i}\right)},$$

(46)

Fig. 11

Configuration of the incident (‘i’), reflected (‘r’) and transmitted (‘t’) waves at an interface between two adjacent dielectric semi-infinite slabs for a TM wave. Note that in the TM mode, the electric field has only components in the x- and z-directions, whereas the magnetic field has only the component in the y-direction

The incident magnetic field in medium 1 is ${\overrightarrow{H_{i}}}\left(x,z\right)={H}_{oi}{e}^{\mathrm{i}{k}_{1}\left(x\mathrm{sin}{\theta }_{i}+z\mathrm{cos}{\theta }_{i}\right)}\widehat{y}$. Using the relation $\eta =\overrightarrow{E}/\overrightarrow{H}$, ${\overrightarrow{H_{i}}}\left(x,z\right)$ is expressed in terms of ${E}_{oi}$ in the following form without loss of generality (Khorasani and Rashidian 2001),

$${\overrightarrow{H_{i}}}\left(x,z\right)=\frac{{E}_{oi}}{{\eta }_{1}}{e}^{\mathrm{i}{k}_{1}\left(x\mathrm{sin}{\theta }_{i}+z\mathrm{cos}{\theta }_{i}\right)}\widehat{y} .$$

(47)

The vector ${\overrightarrow{E_{or}}}$ of the reflected electric field in medium 1, by referring to Fig. 11 is ${\overrightarrow{E_{or}}}={E}_{or}\left(\mathrm{cos}{\theta }_{r}\widehat{x}+\mathrm{sin}{\theta }_{r}\widehat{z}\right)$. In terms of the resolved components, the reflected electric field in medium 1, Eq. (42), now reads

$${\overrightarrow{E_{r}}}\left(x,z\right)={E}_{or}\left(\mathrm{cos}{\theta }_{r}\widehat{x}+\mathrm{sin}{\theta }_{r}\widehat{z}\right){e}^{\mathrm{i}{k}_{1}\left(x\mathrm{sin}{\theta }_{r}-z\mathrm{cos}{\theta }_{r}\right)},$$

(48)

By the same token used for arriving at ${\overrightarrow{H_{i}}}\left(x,z\right)$ in Eq. (47), the reflected magnetic field ${\overrightarrow{H_{r}}}$, Eq. (45) can be expressed in terms of ${E}_{or}$ as

$${\overrightarrow{H_{r}}}\left(x,z\right)=-\frac{{E}_{or}}{{\eta }_{1}}{e}^{\mathrm{i}{k}_{1}\left(x\mathrm{sin}{\theta }_{r}-z\mathrm{cos}{\theta }_{r}\right)}\widehat{y}.$$

(49)

The negative sign in the RHS of Eq. (49) arises from the simple rules that the magnetic field ${\overrightarrow{H_{r}}}$ is the result of the cross product ${\overrightarrow{k_{r}}}\times {\overrightarrow{E_{r}}}$ (see Fig. 11).

By the same token, the transmitted electric field, Eq. (41), is written as

$${\overrightarrow{E_{t}}}\left(x,z\right)={E}_{ot}\left(\mathrm{cos}{\theta }_{t}\widehat{x}-\mathrm{sin}{\theta }_{t}\widehat{z}\right){e}^{\mathrm{i}{k}_{2}\left(x\mathrm{sin}{\theta }_{t}+z\mathrm{cos}{\theta }_{t}\right)}.$$

(50)

The transmitted magnetic field, Eq. (44), is expressed in terms of ${E}_{ot}$ via

$${\overrightarrow{H_{t}}}\left(x,z\right)=\frac{{E}_{ot}}{{\eta }_{2}}{e}^{\mathrm{i}{k}_{2}\left(x\mathrm{sin}{\theta }_{t}+z\mathrm{cos}{\theta }_{t}\right)}\widehat{y}.$$

(51)

Next, we impose the boundary condition of the continuity of the tangential components of the electric field to the boundary $z=0$, ${\overrightarrow{E_{1}}}^{\parallel }-{\overrightarrow{E_{2}}}^{\parallel }=0$. This yields (Jackson 1998; Cheng 1989; Griffiths 2014)

$${\left.\left({\overrightarrow{E_{i}}}^{\parallel }+{\overrightarrow{E_{r}}}^{\parallel }-{\overrightarrow{E_{t}}}^{\parallel }\right)\right|}_{z=0}=0,$$

(52)

where ${\overrightarrow{E_{i}}}^{\parallel }$, ${\overrightarrow{E_{r}}}^{\parallel }$, and ${\overrightarrow{E_{t}}}^{\parallel }$ are tangential components of the incident, reflected, and transmitted electric fields, respectively. Upon substituting Eqs. (46), (48), and (50) for the expression of ${\overrightarrow{E_{i}}}^{\parallel },{\overrightarrow{E_{r}}}^{\parallel }$ and ${\overrightarrow{E_{t}}}^{\parallel }$, at the surface $z=0$, using the Snell’s law ${k}_{1}\mathrm{sin}{\theta }_{i}={k}_{2}\mathrm{sin}{\theta }_{t}$ and the symmetric condition ${\theta }_{r}={\theta }_{i}$, Eq. (52) is cast into the following form,

$$\left({E}_{oi}+{E}_{or}\right)\mathrm{cos}{\theta }_{i} ={E}_{ot}\mathrm{cos}{\theta }_{t}.$$

(53)

Consider an interface located around the coordinate $z=0$, with an effective thickness much less than the wavelength of the light, and with a surface conductivity $\sigma$. An interface current density ${\overrightarrow{J_{s}}}$ at this layer is generated according to Pozar (2012), Khorasani and Rashidian (2001), Cheng (1989)

$${\overrightarrow{J_{s}}}\left(x\right)=\sigma {\overrightarrow{E}}^{\parallel }\left(x,0\right)$$

(54)

where $\overrightarrow{J}$, $\sigma$, and $\overrightarrow{E}$ are current density, conductivity, and electric field, respectively. The magnitude $\left|{\overrightarrow{E}}^{\parallel }\left(x,0\right)\right|\equiv {E}^{\parallel }\left(x,0\right)$ is given by Eq. (53), i.e., ${E}^{\parallel }\left(x,0\right)=\left({E}_{oi}+{E}_{or}\right)\mathrm{cos}{\theta }_{i} ={E}_{ot}\mathrm{cos}{\theta }_{t}.$ In TM mode, Eq. (54) reads

$${\overrightarrow{J_{s}}}\left(x\right)=\sigma {\overrightarrow{E}}^{\parallel }\left(x,0\right)=\sigma \cdot {E}_{ot}\mathrm{cos}{\theta }_{t}{e}^{\mathrm{i}{k}_{2}x\mathrm{sin}{\theta }_{t}} \widehat{x}.$$

(55)

The magnetic field $\overrightarrow{H}$ fulfils the boundary condition of discontinuity at the interface boundary $z=0$ for its tangential components (Fig. 11),

$$\left({\overrightarrow{H_{1}}}-{\overrightarrow{H_{2}}}\right)\times \widehat{z}={\overrightarrow{J_{s}}}.$$

(56)

In this case,

$${\overrightarrow{H_{1}}}={\overrightarrow{H_{i}}}+{\overrightarrow{H_{r}}} ; {\overrightarrow{H_{2}}}={\overrightarrow{H_{t}}}.$$

(57)

Substituting ${\overrightarrow{H_{i}}}$ [from Eq. (47)], ${\overrightarrow{H_{r}}}$ [from Eq. (49)] and ${\overrightarrow{H_{t}}}$ [from Eq. (51)] into the LHS of Eq. (56) at the surface $z=0$, using the Snell’s law ${k}_{1}\mathrm{sin}{\theta }_{i}={k}_{2}\mathrm{sin}{\theta }_{t}$ and the symmetric condition ${\theta }_{r}={\theta }_{i}$ result in the following expression,

$${\overrightarrow{J_{s}}}=\left[\frac{1}{{\eta }_{1}}\left({E}_{oi}-{E}_{or}\right)-\frac{{E}_{ot}}{{\eta }_{2}}\right]{e}^{\mathrm{i}{k}_{2}x\mathrm{sin}{\theta }_{t}}\widehat{x}.$$

(58)

Equating Eqs. (55)–(58) (due to Eq. 56), resulting in

$$\left({E}_{oi}-{E}_{or}\right)\frac{1}{{\eta }_{1}}=\left(\sigma \mathrm{cos}{\theta }_{t}+\frac{1}{{\eta }_{2}}\right){E}_{ot}.$$

(59)

The Fresnel’s reflection and transmission coefficients in TM mode are defined as (Fowles 2012)

$${r}^{\mathrm{TM}}={\left(\frac{{E}_{or}}{{E}_{oi}}\right)}_{\mathrm{TM}}, {t}^{\mathrm{TM}}={\left(\frac{{E}_{ot}}{{E}_{oi}}\right)}_{\mathrm{TM}}.$$

(60)

These coefficients can be re-expressed in terms of the measurable parameters of the medium. From Eqs. (53), and (59) the Fresnel’s transmission coefficient, and Fresnel’s reflection coefficient ${r}^{\mathrm{TM}}$ read, in terms of physically measurable parameters (Pozar 2012; Griffiths 2014)

$${t}^{\mathrm{TM}}=\frac{{E}_{ot}}{{E}_{oi}}=\frac{2\cdot {\eta }_{2}\cdot \mathrm{cos}{\theta }_{i}}{{\eta }_{2}\mathrm{cos}{\theta }_{t}+{\eta }_{1}\mathrm{cos}{\theta }_{i}(1+{\eta }_{2}\sigma \mathrm{cos}{\theta }_{t})}.$$

(61)

$${r}^{\mathrm{TM}}=\frac{{E}_{or}}{{E}_{oi}}=\frac{{\eta }_{2}\mathrm{cos}{\theta }_{t}-{\eta }_{1}\mathrm{cos}{\theta }_{i}\left(1+{\eta }_{2}\sigma \mathrm{cos}{\theta }_{t}\right)}{{\eta }_{2}\mathrm{cos}{\theta }_{t}+{\eta }_{1}\mathrm{cos}{\theta }_{i}\left(1+{\eta }_{2}\sigma \mathrm{cos}{\theta }_{t}\right)}.$$

(62)

Equations (61), (62) are the Fresnel’s transmission and reflection coefficient for lossless materials in which the condition $\overrightarrow{k}={\overrightarrow{k}}^{*}$ holds.

A nonmagnetic material is a medium with ${\mu }_{r}=1$. So, for lossless non-magnetic material, the intrinsic impedance $\eta$ is purely real and contains no complex impedance. Hence, for a lossless, non-magnetic medium, ${\eta }_{j}\to {\eta }_{0}/{n}_{j}$, where ${\eta }_{0}=\sqrt{{\mu }_{0}/{\varepsilon }_{0}}$ is the intrinsic impedance of the vacuum, ${n}_{j}$ the refractive index of the material which labelled $j$. ${\varepsilon }_{0}$ and ${\mu }_{0}$ are the vacuum permittivity and permeability respectively. As such, the Fresnel’s coefficients for lossless, non-magnetic materials can be easily deduced from Eqs. (61) and (62) by setting ${\eta }_{j}\to {\eta }_{0}/{n}_{j}$,

$${t}^{\mathrm{TM}}=\frac{2\cdot {n}_{1}\cdot \mathrm{cos}{\theta }_{i}}{{n}_{1}\cdot \mathrm{cos}{\theta }_{t}+{n}_{2}\cdot \mathrm{cos}{\theta }_{i}(1+\frac{{\eta }_{0}}{{n}_{2}}\cdot \sigma \cdot \mathrm{cos}{\theta }_{t})},$$

(63)

$${r}^{\mathrm{TM}}=\frac{{n}_{1}\mathrm{cos}{\theta }_{t}-{n}_{2}\mathrm{cos}{\theta }_{i}\left(1+\frac{{\eta }_{0}}{{n}_{2}}\sigma \mathrm{cos}{\theta }_{t}\right)}{{n}_{1}\mathrm{cos}{\theta }_{t}{+n}_{2}\mathrm{cos}{\theta }_{i}\left(1+\frac{{\eta }_{0}}{{n}_{2}}\sigma \mathrm{cos}{\theta }_{t}\right)}.$$

(64)

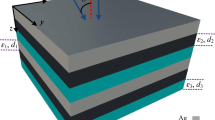
Note that, in the expressions of ${r}^{\mathrm{TM}},{t}^{\mathrm{TM}}$ in Eqs. (61), (62) and Eqs. (63) and (64), the subscripts “1” and “i” refer to the medium in which the incident light is propagating, while “2” and “t” refer to the medium in which the transmitted light is propagating. Light propagates from the left medium (with a subscript “1”) to the right (with a subscript “2”) across a virtual boundary. The indexing scheme adopted in this paper is such that the layers of the medium is denoted with the index j, counting from the left to the right, with j runs from 1 to N. The index of the previous layer (j − 1) is one less than that in the right (j). See Fig. 1 in the main manuscript for an example of a medium labeled using this index scheme. In terms of the index j, the subscripts in ${r}^{\mathrm{TM}},{t}^{\mathrm{TM}}$ in Eqs. (63, 64) are to be replaced via the following substitutions: ${\theta }_{i}\to {\theta }_{j-1}$; ${n}_{1}\to {n}_{j-1}$; ${\theta }_{t}\to {\theta }_{j}$; ${n}_{2}\to {n}_{j}$. In terms of the index j, Eqs. (63), (64) read

$${t}_{j}^{\mathrm{TM}}=\frac{2\cdot {n}_{j-1}\cdot \mathrm{cos}{\theta }_{j-1}}{{n}_{j-1}\cdot \mathrm{cos}{\theta }_{j}+{n}_{j}\cdot \mathrm{cos}{\theta }_{j-1}(1+\frac{{\eta }_{0}}{{n}_{j}}\cdot {\sigma }_{j}\cdot \mathrm{cos}{\theta }_{j})},$$

(65)

$${r}_{j}^{\mathrm{TM}}=\frac{{n}_{j-1}\mathrm{cos}{\theta }_{j}-{n}_{j}\mathrm{cos}{\theta }_{j-1}\left(1+\frac{{\eta }_{0}}{{n}_{j}}{\sigma }_{j}\mathrm{cos}{\theta }_{j}\right)}{{n}_{j-1}\mathrm{cos}{\theta }_{j}+{n}_{j}\mathrm{cos}{\theta }_{j-1}\left(1+\frac{{\eta }_{0}}{{n}_{j}}{\sigma }_{j}\mathrm{cos}{\theta }_{j}\right)}.$$

(66)

Now, we shall derive the expressions for the Fresnel coefficients for the EM wave in the TE mode. This is done by following similar steps as for the TM mode. Due to the translational invariance along the y-direction, the EM fields in the TE mode are x- and z-dependent only, $\overrightarrow{E}=\overrightarrow{E}\left(x,z\right), \overrightarrow{H}=\overrightarrow{H}\left(x,z\right)$. The geometrical configuration of the TE mode is depicted in Fig. 12. In the TE mode, the wave vector and magnetic field have only x- and z-components, $\overrightarrow{k}={k}_{x}\widehat{x}+{k}_{z}\widehat{z}$, $\overrightarrow{H}={H}_{x}\widehat{x}+{H}_{z}\widehat{z}$, whereas the electric field has only y-component, $\overrightarrow{E}={E}_{y}\widehat{y}$, as illustrated in Fig. 12. The incident field in medium 1 can be expressed as

$${\overrightarrow{E_{i}}}\left(x,z\right)={E}_{oi}{e}^{\mathrm{i}{k}_{1}\left(x\mathrm{sin}{\theta }_{i}+z\mathrm{cos}{\theta }_{i}\right)}\widehat{y} .$$

(67)

Fig. 12

Configuration of the reflected and refracted waves at an interface between two adjacent dielectric semi-infinite slabs for the TE mode. Note that in TE mode, the magnetic field has components in the x- and z-directions, whereas the electric field has only component in the y-direction

The incident magnetic field in the TE mode reads

$${\overrightarrow{H_{i}}}\left(x,z\right)=\frac{{E}_{oi}}{{\eta }_{1}}\left(-\mathrm{cos}{\theta }_{i}\widehat{x}+\mathrm{sin}{\theta }_{i}\widehat{z}\right){e}^{\mathrm{i}{k}_{1}\left(x\mathrm{sin}{\theta }_{i}+z\mathrm{cos}{\theta }_{i}\right)}$$

(68)

For the reflected field ($z<0$) in medium 1, one can write (and impose the reflection symmetry ${\theta }_{r}={\theta }_{i}$)

$${\overrightarrow{E_{r}}}\left(x,z\right)={E}_{or}{e}^{\mathrm{i}{k}_{1}\left(x\mathrm{sin}{\theta }_{i}-z\mathrm{cos}{\theta }_{i}\right)}\widehat{y} .$$

(69)

The reflected magnetic field intensity can be written as

$${\overrightarrow{H_{r}}}\left(x,z\right)=\frac{{E}_{or}}{{\eta }_{1}}\left(\mathrm{cos}{\theta }_{r}\widehat{x}+\mathrm{sin}{\theta }_{r}\widehat{z}\right){e}^{\mathrm{i}{k}_{1}\left(x\mathrm{sin}{\theta }_{i}-z\mathrm{cos}{\theta }_{i}\right)}$$

(70)

For the transmitted electric and magnetic fields in medium 2, the following relations can be derived:

$${\overrightarrow{E_{t}}}\left(x,z\right)={E}_{ot}{e}^{\mathrm{i}{k}_{2}\left(x\mathrm{sin}{\theta }_{t}+z\mathrm{cos}{\theta }_{t}\right)}\widehat{y},$$

(71)

$${\overrightarrow{H_{t}}}\left(x,z\right)=\frac{{E}_{ot}}{{\eta }_{2}}\left(-\mathrm{cos}{\theta }_{t}\widehat{x}+\mathrm{sin}{\theta }_{t}\widehat{z}\right){e}^{\mathrm{i}{k}_{2}\left(x\mathrm{sin}{\theta }_{t}+z\mathrm{cos}{\theta }_{t}\right)}$$

(72)

Similarly as for the TM mode and in terms of the indexing scheme j adopted in this paper, ${r}^{\mathrm{TE}},{t}^{\mathrm{TE}}$ for lossless, non-magnetic materials, read (Fig. 12)

$${t}_{j}^{\mathrm{TE}}=\frac{2{n}_{j-1}\mathrm{cos}{\theta }_{j-1}}{{n}_{j-1}\mathrm{cos}{\theta }_{j-1}+{n}_{j}\mathrm{cos}{\theta }_{j}\left(1+\frac{{\eta }_{0}{\sigma }_{j}}{{n}_{j}\mathrm{cos}{\theta }_{j}}\right)},$$

(73)

$${r}_{j}^{\mathrm{TE}}=\frac{{n}_{j-1}\mathrm{cos}{\theta }_{j-1}-{n}_{j}\mathrm{cos}{\theta }_{j}\left(1+\frac{{\eta }_{0}{\sigma }_{j}}{{n}_{j}\mathrm{cos}{\theta }_{j}}\right)}{{n}_{j-1}\mathrm{cos}{\theta }_{j-1}+{n}_{j}\mathrm{cos}{\theta }_{j}\left(1+\frac{{\eta }_{0}{\sigma }_{j}}{{n}_{j}\mathrm{cos}{\theta }_{j}}\right)}.$$

(74)

1.2 Implementation of a transverse voltage to the multilayer thin films in practice

It is worth commenting on how the structure as proposed here could be realized in practice. There are two requirements the outer electrodes must fulfil. Firstly, the electrodes should cover all the region above and under the multilayer thin films and secondly, they should allow EM waves to pass through the multilayer structure. One of the possible ways to satisfy these requirements is by making a small rectangular furrow in the electrodes so that the EM wave can pass through them as illustrated in Fig. 13. If the interface area of the thin film is “a” (as appeared in Eq. 23), and the furrow dimensions are given by $\delta d\times l$, then the electrode area will be $a-\delta d\times l$. The area of furrow should not make a considerable change in the total area, i.e., $a-\delta d\times l\approx a$. With such a design, the incident EM wave should align and propagates, at least in an approximated manner, through the thin film in a way that conforms to the model as proposed. We acknowledge the possibility of other more superior implementation of the model in practice than as proposed above but leave the technical details of such implementation as a future avenue to pursue.

Fig. 13

Implementation of a transverse voltage to the multilayer thin films in practice