Skip to main content
SpringerLink
Log in

Mollow spectrum influenced by collisional fluctuations described by a stochastic model of collisions

  • Published:
Optical and Quantum Electronics Aims and scope Submit manuscript

Abstract

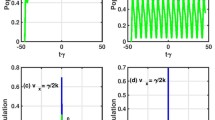
In this paper, we consider the influence of collisional fluctuations on optical phenomena. The fluctuations are taken into account by a simple shift of the constant detuning, involved in a set of optical Bloch equations by collision frequency noise in which this noise is modelled by a two-step random telegraph signal. Following that, the stochastic Bloch equations can be solved analytically in an exact way. As an example, we consider in detail the Mollow spectrum of resonance fluorescence for the case of an arbitrary detuning of the laser frequency, where velocity of the emitters simply has a deterministic value or is distributed with the Maxwell–Boltzmann density in which volume of the buffer gas is arbitrary.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

References

  • Bayrakçeken, F.: Highly sensitive detection of optical discrete absorption and resonance fluorescence of fused silica in the far ultraviolet. Spectrochim. Acta A 60, 923–925 (2004)

    Article  ADS  Google Scholar 

  • Cao Long, V.: Stimulated Raman scattering of pre-Gaussian light. Z. Phys. B 65, 535–538 (1987)

    Article  Google Scholar 

  • Cao Long, V., Doan Quoc, K.: An exact soluble equation for the steady state probability distribution in a nonlinear system: application to the noise reduction in Raman Ring Laser. Opt. Quantum Electron. 43, 137–145 (2012)

    Article  Google Scholar 

  • Cao Long, V.: stochastic models of isolated collisions: applications to optical phenomena. In: Paper Presented in LAMP Conference, 89, 02 Trieste, Italy (1989)

  • Cao Long, V., Wódkiewicz, K.: Multiphoton ionization in the presence of pre-Gaussian light. J. Phys. B 19, 1925–1933 (1986)

    Article  ADS  Google Scholar 

  • Carlsten, J.L., Szöke, A., Raymer, M.G.: Collisional redistribution and saturation of near-resonance scattered light. Phys. Rev. A 15, 1029–1045 (1977)

    Article  ADS  Google Scholar 

  • Doan Quoc, K., Bui Dinh, T., Cao Long, V., Leoński, W.: A stochastic model of the influence of buffer gas collisions on Mollow spectra. Eur. Phys. J. Spec. Top. 222, 2241–2245 (2013)

    Article  Google Scholar 

  • Eberly, J.H., Wódkiewicz, K.: The time-dependent physical spectrum of light. J. Opt. Soc. Am. 67, 1252–1261 (1977)

    Article  ADS  Google Scholar 

  • Eberly, J.H., Wódkiewicz, K., Shore, B.W.: Noise in strong laser–atom interactions: phase telegraph noise. Phys. Rev. A 30, 2381–2389 (1984)

    Article  ADS  Google Scholar 

  • Gerber, S., Rotter, D., Slodička, L., Eschner, J., Carmichael, H.J., Blatt, R.: Intensity-field correlation of single-atom resonance fluorescence. Phys. Rev. Lett. 102, 183601–183604 (2009)

    Article  ADS  Google Scholar 

  • Heitler, W.: The Quantum Theory of Radiation. Oxford University Press, London (1954)

    MATH  Google Scholar 

  • Leonov, A.G., Panteleev, A.A., Starostin, A.N., Chekhov, D.I.: Resonance fluorescence spectrum of a dense three-level medium (sodium vapor) in an intense laser field. Pis’ma Zh. Eksp. Teor. Fiz. 58, 959–963 (1993)

    Google Scholar 

  • Liu, R.H., Tan, W.H.: Resonance fluorescence spectrum by two-level system without the rotating wave approximation. Chin. Phys. Lett. 16, 23–25 (1999)

    Article  ADS  Google Scholar 

  • Loudon, R.: The Quantum Theory of Light. Clarendon Press, Oxford (1983)

    Google Scholar 

  • Mandel, L., Wolf, E.: Optical Coherence and Quantum Optics. Cambridge University Press, New York (1995)

    Book  Google Scholar 

  • Mollow, B.R.: Power spectrum of light scattered by two-level systems. Phys. Rev. 188, 1969–1975 (1969)

    Article  ADS  Google Scholar 

  • Mollow, B.R.: Elastic and inelastic collisional and radiative damping effects on saturated line shapes in the limit of well-separated spectral lines. Phys. Rev. A 15, 1023–1028 (1977)

    Article  ADS  Google Scholar 

  • Nakayama, K., Yoshikawa, Y., Matsumoto, H., Torii, Y., Kuga, T.: Precise intensity correlation measurement for atomic resonance fluorescence from optical molasses. Opt. Express 18, 6604–6612 (2010)

    Article  ADS  Google Scholar 

  • Schuda, F., Stroud Jr, C.R., Hercher, M.: Observation of the resonant Stark effect at optical frequencies. J. Phys. B 7, L198–L202 (1974)

    Article  ADS  Google Scholar 

  • Vamivakas, A.N., Zhao, Y., Lu, C.-Y., Atatüre, M.: Spin-resolved quantum-dot resonance fluorescence. Nat. Phys. 5, 198–202 (2009)

    Article  Google Scholar 

  • Wódkiewicz, K.: Stochastic incoherences of optical Bloch equations. Phys. Rev. A 19, 1686–1696 (1979)

    Article  ADS  MathSciNet  Google Scholar 

  • Wódkiewicz, K.: Noise in strong laser-atom interaction. In: Proceedings of the 6th International School of Coherent Optics, Ustroń 19–26 Sept (1985)

  • Wódkiewicz, K., Shore, B.W., Eberly, J.H.: Pre-Gaussian noise in strong laser–atom interactions. J. Opt. Soc. Am. B 1, 398–405 (1984a)

    Article  ADS  Google Scholar 

  • Wódkiewicz, K., Shore, B.W., Eberly, J.H.: Noise in strong laser–atom interactions: frequency fluctuations and nonexponential correlations. Phys. Rev. A 30, 2390–2398 (1984b)

    Article  ADS  Google Scholar 

  • Zhang, X.H., Hu, X.M.: Sideband entanglement in collective resonance fluorescence. Chin. Phys. B 20, 114205–114206 (2011)

    Article  ADS  Google Scholar 

Download references

Acknowledgments

This research is funded by Vietnam National Foundation for Science and Technology Development (NAFOSTED) under grant number 103.03-2014.13.

Author information

Authors and Affiliations

  1. Institute of Research and Development, Duy Tan University, Da Nang, Vietnam

    Khoa Doan Quoc & Hieu Nguyen Ngoc

  2. Quang Tri Teacher Training College, Km 3, Highway No 9, Dong Ha, Quang Tri, Vietnam

    Khoa Doan Quoc

  3. Quantum Optics and Engineering Division, Institute of Physics, University of Zielona Góra, Prof. A. Szafrana 4a, 65-516, Zielona Góra, Poland

    Van Cao Long

  4. Faculty of Physics, Warsaw University, Hoża 69, 00-681, Warsaw, Poland

    Van Cao Long

  5. Vinh University, 182 Le Duan, Vinh, Nghe An, Vietnam

    Lanh Chu Van

  6. Hong Duc University, 565 Quang Trung, Thanh Hoa, Vietnam

    Hoa Nguyen Van

Authors
  1. Khoa Doan Quoc
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Van Cao Long
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Lanh Chu Van
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Hoa Nguyen Van
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Hieu Nguyen Ngoc
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Khoa Doan Quoc.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Doan Quoc, K., Cao Long, V., Chu Van, L. et al. Mollow spectrum influenced by collisional fluctuations described by a stochastic model of collisions. Opt Quant Electron 48, 45 (2016). https://doi.org/10.1007/s11082-015-0324-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11082-015-0324-x

Keywords

Search

Navigation