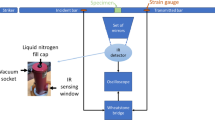
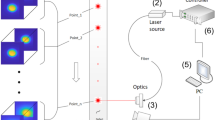
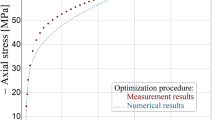
Abstract
An implementation of updating techniques similar to finite element updating in structural dynamics is developed for thermal material inspection using adaptive response surfaces to approximate experimental parameters. In general, thermal models contain high nonlinearities in their parameters, which influences updating accuracies. This is further investigated in this work. Several adaptive response surface regression methods are compared: interpolation, piecewise spline and polynomial regression functions. Next, the influence of the choice of optimisation parameters is discussed and compared with several global and local optimisation routines. Finally, a well-suited regression technique is investigated which transforms the dataset to a smaller, focused response model in each optimisation loop and delivers a proper regression accuracy. This results in data-reduction for the model to be optimised.









Similar content being viewed by others
References
Abd El-Ghany KM, Farag MM (2000) Expert system to automate the finite element analysis for non-destructive testing. NDT E Int 33(6):409–415. doi:10.1016/S0963-8695(00)00009-8
Alfeld P (1989) Scattered data interpolation in three or more variables. Math Methods Comput Aided Geom Des 1–33. http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.35.2949&rep=rep1&type=pdf%5Cnpapers://d4024fd7-a3f3-42d0-af66-5e8b9b0d2151/Paper/p7013
Azadeh A, Ghaderi SF, Sheikhalishahi M, Nokhandan BP (2014) Optimization of short load forecasting in electricity market of Iran using artificial neural networks. Optim Eng. doi:10.1007/s11081-012-9200-8
Bajzer Z, Penzar I (1999) Strategic simplex algorithm. Mayo Clinic and Foundation, Rochester, Minnesota. Software documentation Matlab toolbox: https://nl.mathworks.com/matlabcentral/fileexchange/102-simps
Bergman TL, Lavine AS, Incropera FP, DeWitt DP (2011) Fundamentals of heat and mass transfer, 7th edn. Wiley, Hoboken
Brown P, Hindmarsh A, Petzold L (1994) Using Krylov methods in the solution of large-scale differential-algebraic systems. SIAM J Sci Comput 15:1467–1488
Busse G (1979) Optoacoustic phase angle measurement for probing a metal. Appl Phys Lett 35(10):759–760
Cannavó F (2012) Sensitivity analysis for volcanic source modeling quality assessment and model selection. Comput Geosci 44:52–59. doi:10.1016/j.cageo.2012.03.008
Chapman AJ (1984) Heat transfer, 4th edn. Macmillan, New York
Chaudhuri P, Santra P, Yoele S, Prakash A, Reddy D, Lachhvani L, Govindarajan J, Saxena Y (2006) Non-destructive evaluation of brazed joints between cooling tube and heat sink by IR thermography and its verification using FE analysis. NDT E Int 39(2):88–95. doi:10.1016/j.ndteint.2005.08.002
Cheng H-C, Tsai Y-H, Chen K-N, Fang J (2010) Thermal placement optimization of multichip modules using a sequential metamodeling-based optimization approach. Appl Therm Eng 30(17–18):2632–2642. doi:10.1016/j.applthermaleng.2010.07.004
Coleman T, Li Y (1996) An interior trust region approach for nonlinear minimization subject to bounds. SIAM J Optim. doi:10.1137/0806023
Comsol Multiphysics (2015) Comsol documentation
Cukier RI, Fortuin CM, Shuler KE, Petschek AG, Schaibly JH (1973) Study of the sensitivity of coupled reaction systems to uncertainties in rate coefficients. I theory. J Chem Phys 59(8):3873–3878. doi:10.1063/1.1680571
Darabi A, Maldague X (2002) Neural network based defect detection and depth estimation in TNDE. NDT E Int 35(3):165–175. http://ac.els-cdn.com/S096386950100041X/1-s2.0-S096386950100041X-main.pdf?_tid=48a5f622-9d30-11e3-b900-00000aacb360&acdnat=1393231825_c8c438208474ca17a066479cb5d67f35 http://www.sciencedirect.com/science/article/pii/S096386950100041X
Dashora P, Gupta G, Dashora J (2005) Thermal conductivity, diffusivity and heat capacity of plasticized polyvinyl chloride. Indian J Pure Appl Phys 43(February):132–136. http://nopr.niscair.res.in/handle/123456789/8720
Fang SE, Perera R (2009) A response surface methodology based damage identification technique. Smart Mater Struct 18(6):065009. doi:10.1088/0964-1726/18/6/065009
Faravelli L, Casciati S (2004) Structural damage detection and localization by response change diagnosis. Prog Struct Eng Mater 6(2):104–115. doi:10.1002/pse.171
Foti D, Diaferio M, Giannoccaro NI, Mongelli M (2012) Ambient vibration testing, dynamic identification and model updating of a historic tower. NDT E Int 47:88–95. doi:10.1016/j.ndteint.2011.11.009
Granta Design Limited (2013) CES Edupack 2013
Hagan MT, Menhaj MB (1994) Training feedforward networks with the Marquardt algorithm. IEEE Trans Neural Netw 5(6):989–993. doi:10.1109/72.329697
Hansen N, Kern S (2004) Evaluating the CMA evolution strategy on multimodal test functions. In: 8th international conference on parallel problem solving from nature PPSN VIII, Springer, pp 282–291
Hosder S, Watson LT, Grossman B, Mason WH, Kim H, Haftka RT, Cox SE (2001) Polynomial response surface approximations for the multidisciplinary design optimization of a high speed civil transport. Optim Eng 2(4):431–452
Ibarra-Castanedo C, Maldague XPV (2005) Interactive methodology for optimized defect characterization by quantitative pulsed phase thermography. Res Nondestruct Eval 16(4):175–193. doi:10.1080/09349840500351846
Ibarra-Castanedo C, Genest M, Piau J-M, Guibert S, Bendada A, Maldague XPV (2007) Active infrared thermography techniques for the non-destructive testing of materials. In: Ultrasonic and advanced methods for nondestructive testing and material characterization, Chen, CH, pp 325–348. http://medcontent.metapress.com/index/A65RM03P4874243N.pdf
Igel C, Hansen N, Roth S (2007) Covariance matrix adaptation for multi-objective optimization. Evol Comput 15(1):1–28. doi:10.1162/evco.2007.15.1.1
Isakov V (2006) Inverse problems for partial differential equations, 2nd edition, vol. 127 of applied mathematical sciences. Springer, New York. doi:10.1007/0-387-32183-7
Iwasaki A, Todoroki A, Sugiya T (2003) Remote smart damage detection via internet with unsupervised statistical diagnosis. Springer, Dordrecht, pp 157–166. doi:10.1007/978-94-017-0371-0_16
Jekabsons G (2011) ARESLab adaptive regression Splines toolbox for Matlab/Octave. http://www.cs.rtu.lv/jekabsons/
Kim C, Wang S, Choi KK (2005) Efficient response surface modeling by using moving least-squares method and sensitivity. AIAA J 43(11):2404–2411
Lau S, Almond D, Milne J (1991) A quantitative analysis of pulsed video thermography. NDT E Int 24(4):195–202. doi:10.1016/0963-8695(91)90267-7
Levin R, Lieven N (1998) Dynamic finite element model updating using simulated annealing and genetic algorithms. Mech Syst Signal Process 12:1195–1201. http://www.sciencedirect.com/science/article/pii/S0888327096901363
Louaayou M, Naït-Saïd N, Louai FZ (2008) 2D finite element method study of the stimulation induction heating in synchronic thermography NDT. NDT E Int 41(8):577–581. doi:10.1016/j.ndteint.2008.07.001
Mabrouki F, Genest M, Shi G, Fahr A (2009) Numerical modeling for thermographic inspection of fiber metal laminates. NDT E Int 42(7):581–588. doi:10.1016/j.ndteint.2009.02.010
Maldague X, Marinetti S (1996) Pulse phase infrared thermography. J Appl Phys 79(5):2694. doi:10.1063/1.362662
Maldague X, Galmiche F, Ziadi A (2002) Advances in pulsed phase thermography. Infrared Phys Technol 1(418):1–11. http://www.sciencedirect.com/science/article/pii/S135044950200138X
Marquardt D (1963) An algorithm for least-squares estimation of nonlinear parameters. J Soc Ind Appl 151(3712):859–60. doi:10.1126/science.151.3712.859-b
Marwala T (2010) Finite-element-model updating using computational intelligence techniques. Springer. http://books.google.com/books?id=ObtwUF1uAqMC&pgis=1
MathWorks (2017) The Matlab user manual
Meo M, Zumpano G (2008) Damage assessment on plate-like structures using a global–local optimization approach. Optim Eng 9(2):161–177. doi:10.1007/s11081-007-9016-0
Mottershead J, Friswell M (1993) Model updating in structural dynamics: a survey. J Sound Vib 167(2):347–375. doi:10.1006/jsvi.1993.1340
Nagle RK, Saff EB, Snider AD (2012) Fundamentals of differential equations and boundary value problems. Pearson Education, Boston
Nguyen XS, Sellier A, Duprat F, Pons G (2009) Adaptive response surface method based on a double weighted regression technique. Probab Eng Mech 24(2):135–143. doi:10.1016/j.probengmech.2008.04.001
Nocedal J, Wright SJ (1999) Numerical optimization, springer series in operations research and financial engineering. Springer, New York. doi:10.1007/b98874
Offord,C.,Bajzer,Ž.(2001) A hybrid global optimization algorithm involving simplex and inductive search. In: Computational science—ICCS 2001, vol. 2074. Springer, pp 680–688. doi:10.1007/3-540-45718-6_73
Peeters J, Arroud G, Ribbens B, Dirckx J, Steenackers G (2015) Updating a finite element model to the real experimental setup by thermographic measurements and adaptive regression optimization. Mech Syst Signal Process 64–65:428–440. doi:10.1016/j.ymssp.2015.04.010
Peeters J, Ribbens B, Dirckx JJJ, Steenackers G (2016) Determining directional emissivity: numerical estimation and experimental validation by using infrared thermography. Infrared Phys Technol. doi:10.1016/j.infrared.2016.06.016
Peeters J, Steenackers G, Ribbens B, Arroud G, Dirckx J (2014) Finite element optimization by pulsed thermography with adaptive response surfaces. In: Proceedings of the 2014 international conference on quantitative InfraRed thermography, University of Antwerp, QIRT Council, Bordeaux, p 10. doi:10.21611/qirt.2014.039. http://qirt.org/archives/qirt2014doi/papers/QIRT-2014-039.pdf
Perera R, Ruiz A, Manzano C (2009) Performance assessment of multicriteria damage identification genetic algorithms. Comput Struct 87(1–2):120–127. doi:10.1016/j.compstruc.2008.07.003
Professional Thermographers Association (2014) Emissivity values for common materials.http://www.infrared-thermography.com/material.htm
Ren W-X, Chen H-B (2010) Finite element model updating in structural dynamics by using the response surface method. Eng Struct 32(8):2455–2465. doi:10.1016/j.engstruct.2010.04.019
Rosencwaig A, Busse G (1980) High-resolution photoacoustic thermal-wave microscopy. Appl Phys Lett 36(9):725–727
Sfarra S, Ibarra-Castanedo C, Lambiase F, Paoletti D, Di Ilio A, Maldague X (2012) From the experimental simulation to integrated non-destructive analysis by means of optical and infrared techniques: results compared. Meas Sci Technol 23(11):115601. doi:10.1088/0957-0233/23/11/115601
Steenackers G, Guillaume P (2006) Finite element model updating taking into account the uncertainty on the modal parameters estimates. J Sound Vib 296(4–5):919–934. doi:10.1016/j.jsv.2006.03.023
Steenackers G, Presezniak F, Guillaume P (2009) Development of an adaptive response surface method for optimization of computation-intensive models. Comput Ind Eng 57(3):847–855. doi:10.1016/j.cie.2009.02.016
Susa M, Ibarra-Castanedo C (2007) Pulse thermography applied on a complex structure sample: comparison and analysis of numerical and experimental results. In: IV Conferencia Panamericana de END Buenos Aires, p 12. http://212.8.206.21/article/panndt2007/papers/54.pdf
Thacker WI, Zhang J, Watson LT, Birch JB, Iyer MA, Berry MW (2010) Algorithm 905: SHEPPACK: modified shepard algorithm for interpolation of scattered multivariate data. ACM Trans Math Softw 37(3):34:1–34:20. doi:10.1145/1824801.1824812
Thomas RL, Pouch JJ, Wong YH, Favro LD, Kuo PK, Rosencwaig A (1980) Subsurface flaw detection in metals by photoacoustic microscopya. J Appl Phys 51(2):1152–1156
Tu Z, Lu Y (2008) FE model updating using artificial boundary conditions with genetic algorithms. Comput Struct 86(7–8):714–727. doi:10.1016/j.compstruc.2007.07.005
Vollmer M, Möllmann K(2010) Infrared thermal imaging: fundamentals, research and applications. Wiley, Berlin. http://books.google.com/books?hl=en&lr=&id=5SSiZAMwxtYC&oi=fnd&pg=PR15&dq=Infrared+thermal+imaging:+fundamentals,+research+and+applications&ots=ClTOKRc2sH&sig=oRgWvTf06VZj2g0iXLoElTWHaxs
Wang GG, Dong Z, Aitchison P (2001) Adaptive response surface method—a global optimization scheme for approximation-based design problems. Eng Optim 33(6):707–733. doi:10.1080/03052150108940940
Xenics Vision (2008) D. Uwaerts, gobi interface control document XP Gobi 384-1471
Xenics Vision (2010) Sensor-Gobi 384-1471
Acknowledgements
This research has been funded by the University of Antwerp and the Institute for the Promotion of Innovation by Science and Technology in Flanders (IWT) by the support to the TETRA project ‘SINT’ with Project Number HBC.2017.0032. Furthermore, the research leading to these results has received funding from Industrial Research Fund FWO Krediet aan navorsers 1.5.240.13N and the FWO travel Grant V4.010.16N. The authors also acknowledge the Flemish government (GOA-Optimech) and the research council of the University of Antwerp (fti-OZC) for its funding.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Peeters, J., Louarroudi, E., Bogaerts, B. et al. Active thermography setup updating for NDE: a comparative study of regression techniques and optimisation routines with high contrast parameter influences for thermal problems. Optim Eng 19, 163–185 (2018). https://doi.org/10.1007/s11081-017-9368-z
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11081-017-9368-z