Characterization of Combinatorially Independent Permutation Separability Criteria

Abstract

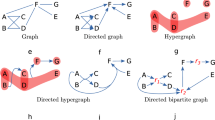
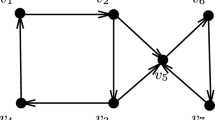
The so-called permutation separability criteria are simple operational conditions that are necessary for separability of mixed states of multipartite systems: (1) permute the indices of the density matrix and (2) check if the trace norm of at least one of the resulting operators is greater than one. If it is greater than one then the state is necessarily entangled. A shortcoming of the permutation separability criteria is that many permutations give rise to equivalent separability criteria. Therefore, we introduce a necessary condition for two permutations to yield independent criteria called combinatorial independence. This condition basically means that the map corresponding to one permutation cannot be obtained by concatenating the map corresponding to the second permutation with a norm-preserving map. We characterize completely combina-torially independent criteria, and determine simple permutations that represent all independent criteria. The representatives can be visualized by means of a simple graphical notation. They are composed of three basic operations: partial transpose, and two types of so-called reshufflings. In particular, for a four-partite system all criteria except one are composed of partial transpose and only one type of reshuffling; the exceptional one requires the second type of reshuffling. Furthermore, we show how to obtain efficiently a simple representative for every permutation. This method allows to check easily if two permutations are Combinatorially equivalent or not.