International Capital Flows in the Model with Limited Commitment and Incomplete Markets

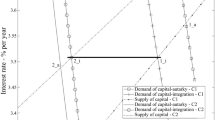
Abstract

Recent literature has proposed two alternative types of financial frictions, i.e., limited commitment and incomplete markets, to explain the empirical patterns of international capital flows between developed and developing countries in the past two decades. This paper integrates these two frictions into a two-country overlapping-generations framework to facilitate a direct comparison of their respective effects. In our model, limited commitment distorts the investment made by agents with different productivity, which creates a wedge between the interest rates on equity capital vs. credit capital; while incomplete markets distort the investment among projects with different riskiness, which creates a wedge between the risk-free rate and the mean rate of return to risky capital. We show that the two approaches are observationally equivalent with respect to their implications for international capital flows, production efficiency, and aggregate output.

Appendix

1.1 Proof of Proposition 2.1

Proof

According to Eq. 7b, the relative price is rewritten as $\chi ^{i}_{t+1}\equiv \frac {V^{i,A}_{t+1}}{V^{i,B}_{t+1}}= \frac {R^{i,h}_{t}}{V^{i,B}_{t+1}}$. Combining Eqs. 3, 7a, d, and e, we get the lending of individual household $d^{i,h}_t=\frac {\theta ^{i}}{\theta ^i+1}\beta \omega ^{i}_{t}$. Combining it with Eqs. 7a and d, we get

$$ \chi^{i}_{t+1}=\theta^{i}\left[1+\frac{\eta}{1-\eta}\frac{d^{i,e}_{t}} {d^{i,e}_{t}}\right]=\frac{\eta+\theta^{i}}{1-\eta}=1-\frac{\bar\theta-\theta^{i}}{1-\eta} $$

(21)

Thus, the relative price is time invariant $\chi ^{i}_{t+1}=\chi ^{i}_{IFA}$ and positively related with θ ⁱ, under IFA. According to Eq. 7b, the equity rate of entrepreneurs in sector B is rewritten as $R^{i,e}_{t}=R^{i,h}_{t}\frac {1-\theta ^{i}}{\chi ^{i}_{t+1}-\theta ^{i}}$. Combining it with Eq. 6 and using Eq. 21 to substitute away θ ⁱ with $\chi ^{i}_{IFA}$, we get the solution to the loan rate as specified in Eq. 8b. Plugging it back to Eq. 6, we get the solution to the equity rate as specified in Eq. 8f. The price of intermediate good B is obtained by definition. Given the Cobb-Douglas production function as specified in Eq. 2, $\left (\omega ^{i}_{t+1}\right )^{1-\alpha }\left (V^{i,A}_{t+1}\right )^{\frac {\alpha }{2}} \left (V^{i,B}_{t+1}\right )^{\frac {\alpha }{2}}=1$ and the dynamic equation of wages is obtained as specified in Eq. 8e. □

1.2 Proof of Proposition 2.2

Proof

A second-order Taylor approximation of $\ln \zeta ^{i}_{t}$ around σ = 0 gives,

$$ \ln\zeta^{i}_t=\frac{\ln E_{t}(\hat\zeta^{i}_{t+1})^{1-\gamma}}{1-\gamma} \approx E_{t}\ln \hat\zeta^{i}_{t+1}+\frac{(1-\gamma)\text{Var}_{t}\ln\hat\zeta^{i}_{t+1}}{2} $$

(22)

$$ \approx\phi^{i}_{t} \ln V^{i,A}_{t+1}+(1-\phi^{i}_t)\ln V^{i,B}_{t+1}-\frac{(\phi^{i}_t)^{2}}{2}\gamma[(1-\lambda^i)\sigma]^2 $$

(23)

The agent chooses $\phi ^{i}_{t}$ to maximize the ex ante risk-adjusted rate of portfolio return, $\ln \zeta ^{i}_{t}$, and the first order condition gives the optimal portfolio choice,

$$ \phi^{i}_{t}\approx\frac{\ln V^{i,A}_{t+1}-\ln V^{i,B}_{t+1}}{\gamma\left[\left(1-\lambda^{i}\right)\sigma\right]^{2}}=\frac{\ln \chi^{i}_{t+1}}{\gamma\left[\left(1-\lambda^{i}\right)\sigma\right]^{2}}\approx \frac{\chi^{i}_{t+1}-1}{\gamma\left[\left(1-\lambda^{i}\right)\sigma\right]^{2}}. $$

(24)

Let $\xi ^{i}_{t}\equiv \frac {\chi ^{i}_{t+1}-1}{(1-\lambda ^i)\sigma }$ denote the Sharpe Ratio. Plugging the solution of $\phi ^{i}_{t}$ into Eq. 22,

$$ \ln\zeta^{i}_{t}\approx \ln V^{i,B}_{t+1}+{\frac{\left(\xi^{i}_{t}\right)^{2}}{2\gamma}}, \Rightarrow \zeta^{i}_{t}\approx V^{i,B}_{t+1}e^{\frac{(\xi^{i}_t)^{2}}{2\gamma}}\approx V^{i,B}_{t+1}\left[1+{\frac{(\xi^{i}_t)^{2}}{2\gamma}}\right]. $$

(25)

Using Eqs. 3, 9d, and 26, we get the relative price as a constant depending on the degree of market completeness,

$$ \chi^{i}_{t+1}=\frac{V^{i,A}_{t+1}}{V^{i,B}_{t+1}}=\frac{M^{i,B}_{t+1}} {M^{i,A}_{t+1}}=\frac{1-\phi^{i}_{t}}{\phi^{i}_{t}}\;\;\Rightarrow\;\; \chi^{i}_{t+1}=\chi^{i}_{IFA}\approx\sqrt{1+\gamma[(1-\lambda^i)\sigma]^{2}}>1 $$

(26)

Use $\left (\chi ^{i}_{IFA}\right )^2-1=\gamma [(1-\lambda ^i)\sigma ]^{2}$ to substitute away γ(1 − λ ⁱ)σ ² from Eq. 26, we get the portfolio choice $\phi ^{i}_{t}\approx \frac {\chi ^{i}_{IFA}-1}{\left (\chi ^{i}_{IFA}\right )^2-1}= \frac {1}{\chi ^{i}_{IFA}+1}$.

Plugging the solution to $\phi ^{i}_{t}$ and $V^{i,A}_{t+1}=\chi ^{i}_{IFA}R^{i}_{t}$ into Eq. 10, we solve the risk-free interest rate as specified in Eq. 11b. Other variables can be solved as in the proof of Proposition 2.1. □

1.3 Proof of Lemma 3.1

Proof

The proof consists of three steps. First, we prove that Eq. 15a is the solution to the equity rate under full capital mobility. Define $\Delta \chi ^{i}_{t+1}\equiv \chi ^{i}_{t+1}-\chi ^{i}_{IFA}$. If the borrowing constraint is binding, it holds under IFA and under full capital mobility,

$$ \chi^{i}_{t+1}=\frac{R^{i,h}_{t}\left(1-\theta^{i}\right)}{R^{i,e}_{t}}+\theta^{i}, \Rightarrow \frac{\Delta\chi^{i}_{t+1}}{1-\theta^{i}}=\frac{R^{i,h}_{t}}{R^{i,e}_{t}}- \frac{R^{i,h}_{IFA}}{R^{i,e}_{IFA}}. $$

(27)

According to Eq. 6, $(1-\eta )R^{i,h}_{IFA} +\eta R^{i,e}_{IFA}=\Psi _{IFA}$. Substituting $R^{i,h}_{t}$ and $R^{i,h}_{IFA}$ with $R^{i,e}_{t}$ and $R^{i,e}_{IFA}$ using Eq. 14 and $R^{i,h}_{IFA}=\frac {1}{(1-\eta )}\left (\Psi _{IFA}-\eta R^{i,e}_{IFA}\right )$, we solve the equity rate from Eq. 27. Plug in the solution to the equity rate in Eq. 14 to solve the loan rate $R^{i,h}_{t}$.

Second, we prove that $\chi ^{i}_{t+1}$ is constant under full capital mobility. Let us assume that $\chi ^{i}_{t+1}$ is time variant and so is the auxiliary variable $Z^{i}_{t+1}$ defined in Eq. 15a. According to Eq. 15a, the equity rate equalization in country i and N implies that

$$ R^{S,e}_{IFA}-\mathcal{Z}^{S}_{t+1} =R^{N,e}_{IFA}-\mathcal{Z}^{N}_{t+1}, \\ $$

(28)

$$\Delta\chi^{S}_{t+1} =\frac{1-\theta^{S}}{1-\theta^{N}}\Delta\chi^{N}_{t+1}+ \left(\frac{1}{p^{N}_{IFA}}-\frac{1}{p^{S}_{IFA}}\right) \frac{1-\theta^{S}}{1-\eta},\\ $$

(29)

$$ \frac{\partial\Delta\chi^{i}_{t+1}} {\partial\Delta\chi^{N}_{t+1}} = \frac{1-\theta^{i}}{1-\theta^{N}}>0. $$

(30)

Using Eqs. 15a, e, and 29, we rewrite the condition, $\Omega ^{S}_{t}+\Omega ^{N}_{t}=0$, into

$$ \omega^{S}_{t+1}\Delta\chi^{S}_{t+1} \frac{p^{S}_{IFA}(1-\eta)}{1-\theta^{S}}+\omega^{N}_{t+1}\Delta\chi^{N}_{t+1} \frac{p^{N}_{IFA}(1-\eta)}{1-\theta^{N}}=0 $$

(31)

Given the Cobb-Douglas production function, $\omega ^{i}_{t+1}=\left (\chi ^{i}_{t+1}\right )^{\frac {\rho }{2}}\left (R^{i}_{t}\right )^{-\rho }$. Combining it with the loan rate equalization, $R^{i,h}_t=R^{*,h}_{t}$, we simplify Eq. 31 as

$$ \mathrm{K}^{S}_{t+1}+\mathrm{K}^{N}_{t+1} =0, \text{where}{\kern3pt} \mathrm{K}^{i}_{t+1}\equiv\left(\Delta\chi^{i}_{t+1}+\chi^{i}_{IFA}\right)^{\frac{\rho} {2}}\Delta\chi^{i}_{t+1} \frac{p^{i}_{IFA}\left(1-\eta\right)}{1-\theta^{i}} ,\\ $$

(32)

$$ \frac{\partial \mathrm{K}^{i}_{t+1}}{\partial \Delta\chi^{i}_{t+1}} =\left(\chi^{i}_{t+1}\right)^{\frac{\rho} {2}-1} \left(\chi^{i}_{t+1}+\frac{\rho} {2}\Delta\chi^{i}_{t+1}\right) \frac{p^{i}_{IFA}\left(1-\eta\right)}{1-\theta^{i}}>0. $$

(33)

Using Eq. 29 to substitute $\Delta \chi ^{i}_{t+1}$ with $\Delta \chi ^{N}_{t+1}$, the left-hand side of Eq. 32 becomes a monotonically increasing function of $\Delta \chi ^{N}_{t+1}$,

$$ \frac{\partial\left(\mathcal{K}^{S}_{t+1}+\mathcal{K}^{N}_{t+1}\right)}{\partial \Delta\chi^{N}_{t+1}}=\frac{\partial\mathcal{K}^{S}_{t+1}}{\partial \Delta\chi^{S}_{t+1}}\frac{\partial \Delta\chi^{S}_{t+1}}{\partial \Delta\chi^{N}_{t+1}}+\frac{\partial\mathcal{K}^{N}_{t+1}}{\partial \Delta\chi^{N}_{t+1}}>0. $$

(34)

Suppose that $\Delta \chi ^{N}_{t+1}\geq 0$. Equation 29 implies that $\Delta \chi ^{i}_{t+1}>0$. According to the definition of $\mathcal {K}^{i}_{t+1}$, $\Delta \chi ^{i}_{t+1}>0$ implies that $\mathcal {K}^{i}_{t+1}>0$. Thus, the left-hand side of Eq. 32 is larger than zero, which contradicts Eq. 32. Thus, there exits a unique solution of $\Delta \chi ^{N}_{t+1}$ smaller than zero and time-invariant. Using Eq. 29, we can then solve $\Delta \chi ^{S}_{t+1}$, accordingly.

Finally, we prove the existence of a unique and stable steady state under full capital mobility. $\chi ^{i}_{t+1}$ is time-invariant and so is $\mathcal {Z}^{i}_{t+1}$. Let $R^{i,h}_{FCM}\equiv R^{i,h}_{IFA}+\frac {\eta } {1-\eta }\mathcal {Z}^{i}_{FCM}$ which is same across countries, $R^{i,h}_{FCM}=R^{*,h}_{FCM}$. Thus, the loan rate depends on the dynamics of the world-average wages, according to Eq. 15b. So is the wage in country i,

$$\begin{array}{*{20}l} \omega^{i}_{t+1}=\left( \frac{\omega^{w}_{t+1}}{\omega^{w}_{t}}R^{*}_{FCM}\right)^{-\rho} \left(\chi^{i}_{FCM}\right)^{\frac{\rho}{2}}. \end{array} $$

The dynamics of the world-average wages are

$$\begin{array}{*{20}l} \omega^{w}_{t+1}&=\frac{\omega^{S}_{t+1}+\omega^{N}_{t+1}}{2}= \left( \frac{\omega^{w}_{t+1}}{\omega^{w}_{t}}R^{*,h}_{FCM}\right)^{-\rho} \frac{\left(\chi^{S}_{FCM}\right)^{\frac{\rho}{2}}+\left(\chi^{N}_{FCM}\right)^{\frac{\rho} {2}}}{2},\\ \omega^{w}_{t+1}&=\left(\frac{\omega^{w}_{t}}{R^{*,h}_{FCM}}\right)^{\alpha} \left[\frac{\left(\chi^{S}_{FCM}\right)^{\frac{\rho}{2}}+\left(\chi^{N}_{FCM}\right)^{\frac{\rho} {2}}}{2}\right]^{1-\alpha} \end{array} $$

Given α ∈ (0, 1), the phase diagram of the world-average wage is concave. Thus, there exists a unique and stable steady state. Proportional to the wage, aggregate output in country i is determined by the world output dynamics. □

1.4 Proof of Lemma 3.2

Proof

The proof consists of three steps.

First, we prove that FDI equalizes the Sharpe ratio across the border. An agent born in country i can choose between investing its single project domestically or abroad. According to the solution to the optimal portfolio choices in Angeletos (2007) and Angeletos and Panousi (2011), three factors determine the agent’s optimal portfolio share of risky investment and the risk-adjusted rate of portfolio return, i.e., the mean rate of return in the risky sector, $\ln V^{i,A}_{t+1}$, the risk-free interest rate, $\ln R^{i}_{t}$, and the risk-sharing factor λ ⁱ. By assumption, agents obtain risk sharing in the country where they make the risky investment. Given the world risk-free interest rate $R^{*}_{t}$, the portfolio share of risky investment and the risk-adjusted rate of portfolio return are $\phi ^{i,l}_{t}\approx \frac {\zeta ^{i,l}_{t}}{\gamma (1-\lambda ^l)\sigma }$ and $\xi ^{i,l}_{t}\approx R^{*}_{t}\left [1+\frac {\left (\zeta ^{i,l}_{t}\right )^{2}}{2\gamma }\right ]$, if the agent born in country i makes the risky investment abroad in country l ≠ i, where the Sharpe ratio is $\zeta ^{i,l}_{t}\equiv \frac {\ln V^{l,A}_{t+1}-\ln R^{*}_{t}}{(1-\lambda ^l)\sigma }$. In equilibrium, an agent is indifferent between investing the risky project domestically or abroad. Given $R^{i}_t=R^{l}_t=R^{*}_{t}$, the no-arbitrage condition $\xi ^{i,l}_t=\xi ^{i}_{t}$ is simplified as the equalization of the Sharpe ratio, $\zeta ^{l}_t=\zeta ^{i}_{t}$, and the portfolio share is simplified as $\phi ^{i,l}_t=\phi ^{l}_{t}$.

Suppose that FDI flows are from country N to country S, i.e., $\Omega ^{N}_t>0>\Omega ^{S}_{t}$ and $\Omega ^{N}_t+\Omega ^{S}_t=0$. The total savings of agents born in country N but making the risky investment abroad is $\frac {\Omega ^{N}_{t}}{\phi ^{S}_{t}}$, while the total savings of agents born in country N and making the risky investment domestically is $\frac {M^{N,A}_{t+1}}{\phi ^{N}_{t}}$. Thus, the aggregate savings of agents born in country N and in country S are specified as in Eqs. 17b, respectively.

Second, we prove by contradiction that $\chi ^{i}_{t+1}$ is time-invariant under full capital mobility. Assume that $\chi ^{i}_{t+1}$ is time-variant. The equalization of the Sharpe Ratio $\zeta ^{S}_{t+1}=\zeta ^{N}_{t+1}=\zeta ^{*}_{t+1}$ implies that $\chi ^{S}_{t+1}$ is linear and increasing in $\chi ^{N}_{t+1}$,

$$ \frac{\chi^{S}_{t+1}-1}{1-\lambda^{S}}=\frac{\chi^{N}_{t+1}-1}{1-\lambda^{N}}. $$

(35)

Net capital flows sum up to zero at the world level,

$$\begin{array}{*{20}l} \beta\left(\omega^{S}_t+\omega^{N}_{t}\right)=\frac{\rho}{2R^{*}_{t}}\left[ \omega^{S}_{t+1}\frac{\left(1+\chi^{S}_{t+1}\right)}{\chi^{S}_{t+1}}+ \omega^{N}_{t+1}\frac{\left(1+\chi^{N}_{t+1}\right)}{\chi^{N}_{t+1}}\right],\\ \end{array} $$

(36)

$$ 2\left(\omega^{S}_{t+1}+\omega^{N}_{t+1}\right)=\left[1+\frac{\left(\xi^{S}_{t+1}\right)^{2}}{\gamma}\right] \omega^{S}_{t+1}\frac{\left(1+\chi^{S}_{t+1}\right)}{\chi^{S}_{t+1}}+\left[1+ \frac{\left(\xi^{N}_{t+1}\right)^{2}}{\gamma}\right] \omega^{N}_{t+1}\frac{\left(1+\chi^{N}_{t+1}\right)}{\chi^{N}_{t+1}}. $$

(37)

Since $\omega ^{i}_{t+1}=\left (\chi ^{i}_{t+1}\right )^{-\frac {\rho }{2}}\left (R^{i}_{t}\right )^{-\rho }$ and $R^{S}_t=R^{N}_t=R^{*}_{t}$, Eq. 37 can be rewritten as

$$ 2\left[\left(\chi^{S}_{t+1}\right)^{-\frac{\rho}{2}}+\left(\chi^{N}_{t+1}\right)^{-\frac{\rho}{2}}\right] = \left[1+\frac{\left(\xi^{S}_{t+1}\right)^{2}}{\gamma}\right] \left[\left(\chi^{S}_{t+1}\right)^{-\frac{\rho}{2}}+ \left(\chi^{S}_{t+1}\right)^{-\frac{\rho}{2}-1}\right]+ $$

(38)

$$ \left[1+\frac{\left(\xi^{N}_{t+1}\right)^{2}}{\gamma}\right] \left[\left(\chi^{N}_{t+1}\right)^{-\frac{\rho}{2}}+\left(\chi^{N}_{t+1}\right)^{-\frac{\rho}{2}-1}\right]. $$

(39)

Since $\frac {(\xi ^{i}_{t+1})^{2}}{\gamma }=\frac {(\chi ^{i}_{t+1}-1)^{2}}{(\chi ^{i}_{IFA})^2-1}$ is a function of $\chi ^{i}_{t+1}$, given $\chi ^{i}_{IFA}$ as a constant. Thus, according to Eq. 39, $\chi ^{S}_{t+1}$ is an implicit function of $\chi ^{N}_{t+1}$ and it can be proved that $\frac {\partial \chi ^{S}_{t+1}}{\partial \chi ^{N}_{t+1}}<0$. Thus, according to Eqs. 35 and 39, there exists a unique and time-invariant solution to $\chi ^{N}_{t+1}$ and $\chi ^{S}_{t+1}$.

Finally, we prove the existence of a unique and stable steady state under full capital mobility. The relative price and the Sharpe ratio are time invariant which are denoted by $\chi ^{i}_{FCM}$ and $\xi ^{i}_{FCM}$, respectively. Define $R^{i}_{FCM}\equiv \frac {\rho }{\beta }\frac {1}{1+\frac {(\xi ^{*}_{FCM})^{2}}{\gamma }}$, which is same across countries, $R^{i}_{FCM}=R^{*}_{FCM}$. Thus, the loan rate depends on the dynamics of the world-average wages, $R^{i}_{t+1}=\frac {\omega ^{w}_{t+1}}{\omega ^{w}_{t}}R^{*}_{FCM}$ and so does the wage in country i,

$$\begin{array}{*{20}l} \omega^{i}_{t+1}=\left( \frac{\omega^{w}_{t+1}}{\omega^{w}_{t}}R^{*}_{FCM}\right)^{-\rho} (\chi^{i}_{FCM})^{-\frac{\rho}{2}}. \end{array} $$

The dynamics of the world-average wages are

$$\begin{array}{*{20}l} \omega^{w}_{t+1}&=\frac{\omega^{S}_{t+1}+\omega^{N}_{t+1}}{2}= \left( \frac{\omega^{w}_{t+1}}{\omega^{w}_{t}}R^{*}_{FCM}\right)^{-\rho} \frac{\left(\chi^{S}_{FCM}\right)^{-\frac{\rho}{2}}+\left(\chi^{N}_{FCM}\right)^{-\frac{\rho}{2}}}{2},\\ \omega^{w}_{t+1}&=\left(\frac{\omega^{w}_{t}}{R^{*}_{FCM}}\right)^{\alpha} \left[\frac{\left(\chi^{S}_{FCM}\right)^{-\frac{\rho}{2}}+\left(\chi^{N}_{FCM}\right)^{-\frac{\rho}{2}}}{2}\right]^{1-\alpha} \end{array} $$

Given α ∈ (0, 1), the phase diagram of the world-average wage is concave. Thus, there exists a unique and stable steady state. Proportional to the wage, aggregate output in country i is determined by the world output dynamics. □

1.5 Proof of Proposition 2.3

Proof

The relative price of intermediate goods $\chi ^{i}_{IFA}$ reflects the distortion of two financial frictions on aggregate allocation under IFA. Let $\chi ^{i}_{IFA,LC}$ and $\chi ^{i}_{IFA,IM}$ denote the respective relative price of intermediate goods under the model setting of limited commitment and that of incomplete markets. According to Eqs. 8e and 11e, the wage rate has the same functional form with respect to $\chi ^{i}_{IFA}$ and so does aggregate output. Obviously, the steady-state aggregate output is same across the two model settings and so are the loan rate in the setting of limited commitment and the risk-free interest rate in the setting of incomplete markets, as long as $\chi ^{i}_{IFA,LC}=\frac {1}{\chi ^{i}_{IFA,IM}}$. That is, $1-\frac {\bar \theta -\theta ^{i}}{1-\eta }=\frac {1}{\sqrt {1+\gamma [(1-\lambda ^i)\sigma ]^{2}}}$ and the solution to θ ⁱ is a function of λ ⁱ in the form, $\theta ^i=\bar \theta -(1-\eta )\left \{1-\frac {1}{\sqrt {1+\gamma [(1-\lambda ^i)\sigma ]^{2}}} \right \}$. A necessary condition for $\theta ^{i}\in [0,\bar \theta )$ is $1+\sqrt {1+\gamma [(1-\lambda ^i)\sigma ]^{2}}\leq \frac {1}{\eta }$. □