Skip to main content
SpringerLink
Log in

Stability and Hamiltonian Hopf bifurcation for a nonlinear symmetric bladed rotor

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

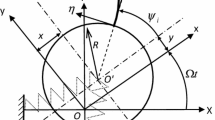
The modal interaction which leads to Hamiltonian Hopf bifurcation is studied for a nonlinear rotating bladed-disk system. The model, which is discussed in the paper, is a Jeffcott rotor carrying a number of planar blades which bend in the plane of the motion. The rigid rotating disk is supported on nonlinear bearings. It is supposed that this dynamical system is a Hamiltonian system which is perturbed by small dissipative and nonlinear forces. Krein’s theorem is employed for obtaining a stability criterion. The nonlinear eigenvalue equations on the stability boundary are turned into ordinary differential equations (ODEs) by differentiating them over the rotating speed. By solving these ODEs, the eigenmodes and the eigenvalues on the stability boundary are obtained. The bifurcation analysis is performed by applying multiple scales method around the boundary. The rotor nonlinear behavior and damping effects are studied for different conditions on the rotating speed and nonlinearity type by the bifurcation equation. It is shown that the damping distribution between the blades and bearings may shift the unstable mode. Depending on the nonlinearity type, subcritical and supercritical Hopf bifurcation are possible.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18

Similar content being viewed by others

References

  1. Seyranian, A.P., Mailybaev, A.A.: Multiparameter Stability Theory with Mechanical Applications. Word Scientific, New Jersey (2003)

    MATH  Google Scholar 

  2. Meiss, J.D.: Differential Dynamical Systems. Mathematical Modeling and Computation. Society for Industrial and Applied Mathematics, Philadelphia (2007)

    Book  Google Scholar 

  3. Crandall, S.H., Dugundji, J.: Resonant whirling of aircraft propeller-engine systems. J. Appl. Mech. 48(4), 929–935 (1981)

    Article  Google Scholar 

  4. Genta, G.: On the stability of rotating blade arrays. J. Sound Vib. 273(4–5), 805–836 (2004). doi:10.1016/s0022-460x(03)00784-3

    Article  Google Scholar 

  5. Genta, G.: Dynamics of Rotating Systems, 1st edn. Springer, Berlin (2005)

    Book  Google Scholar 

  6. Hartog, J.P.D.: Mechanical Vibrations, 4th edn. McGraw-Hill Book Company, New York (1956)

    MATH  Google Scholar 

  7. Sanches, L., Michon, G., Berlioz, A., Alazard, D.: Instability zones for isotropic and anisotropic multibladed rotor configurations. Mech. Mach. Theory 46(8), 1054–1065 (2011). doi:10.1016/j.mechmachtheory.2011.04.005

    Article  MATH  Google Scholar 

  8. Shahgholi, M., Khadem, S.: Stability analysis of a nonlinear rotating asymmetrical shaft near the resonances. Nonlinear Dyn. 70(2), 1311–1325 (2012). doi:10.1007/s11071-012-0535-7

    Article  MathSciNet  Google Scholar 

  9. Crandall, S.H., Mroszczyk, J.W.: Conservative and Nonconservative Coupling in Dynamic Systems. Institution of Mechanical Engineers, London (2003)

    Google Scholar 

  10. Leissa, A.W.: On a curve veering aberration. ZAMP (J. Appl. Math. Phys.) 25, 99–111 (1974)

    Article  MATH  Google Scholar 

  11. Afolabi, D.: Modal Interaction in Linear Dynamic Systems Near Degenerate Modes. NASA, Ohio (1991)

    Google Scholar 

  12. Afolabi, D., Mehmed, O.: On Curve Veering and Flutter of Rotating Blades, pp. 1–45. NASA, Ohio (1993)

    Google Scholar 

  13. Afolabi, D.: The cusp catastrophe and the stability problem of helicopter ground resonance. Proc. R. Soc. Lond. 441(1912), 399–406 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  14. Seiranyan, A.P.: Collision of eigenvalues in linear oscillatory systems. J. Appl. Math. Mech. 58(5), 805–813 (1994). doi:10.1016/0021-8928(94)90005-1

    Article  MathSciNet  MATH  Google Scholar 

  15. Seyranian, A.P., Mailybaev, A.A.: Interaction of eigenvalues in multi-parameter problems. J. Sound Vib. 267(5), 1047–1064 (2003). doi:10.1016/s0022-460x(03)00360-2

    Article  MathSciNet  MATH  Google Scholar 

  16. Kirillov, O.N.: Unfolding the conical zones of the dissipation-induced subcritical flutter for the rotationally symmetrical gyroscopic systems. Phys. Lett. A 373(10), 940–945 (2009). doi:10.1016/j.physleta.2009.01.013

    Article  MATH  Google Scholar 

  17. Kirillov, O.N., Seyranian, A.O.: The effect of small internal and external damping on the stability of distributed non-conservative systems. J. Appl. Math. Mech. 69(4), 529–552 (2005). doi:10.1016/j.jappmathmech.2005.07.004

    Article  MathSciNet  Google Scholar 

  18. Kirillov, O.N.: Campbell diagrams of weakly anisotropic flexible rotors. Proc. R Soc. A 465(2109), 2703–2723 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  19. Cleghorn, W.L., Fenton, R.G., Tabarrok, B.: Steady-state vibrational response of high-speed flexible mechanisms. Mech. Mach. Theory 19(4—-5), 417–423 (1984). doi:10.1016/0094-114X(84)90100-9

    Article  Google Scholar 

  20. Peletan, L., Baguet, S., Torkhani, M., Jacquet-Richardet, G.: A comparison of stability computational methods for periodic solution of nonlinear problems with application to rotordynamics. Nonlinear Dyn. 72(3), 671–682 (2013). doi:10.1007/s11071-012-0744-0

    Article  MathSciNet  Google Scholar 

  21. Sadeghi, M., Ansari Hosseinzadeh, H.: Parametric excitation of a thin ring under time-varying initial stress: theoretical and numerical analysis. Nonlinear Dyn. 74(3), 733–743 (2013). doi:10.1007/s11071-013-1001-x

    Article  MathSciNet  Google Scholar 

  22. van der Meer, J.-C.: The Hamiltonian Hopf Bifurcation. Springer, Berlin (1985)

    Book  MATH  Google Scholar 

  23. Nayfeh, A.H.: Introduction to perturbation techniques. Wiley, New York (1993)

    Google Scholar 

  24. Nayfeh, A.H., Balachandran, B.: Computational and Experimental Methods. Applied Nonlinear Dynamics: Analytical. Wiley, New York (2008)

    Google Scholar 

  25. Lahiri, A., Sinha Roy, M.: The Hamiltonian Hopf bifurcation: an elementary perturbative approach. Int. J. Non-Linear Mech. 36, 787–802 (2001). doi:10.1016/S0020-7462(00)00045-7

    Article  MATH  Google Scholar 

  26. Gils, S., Krupa, M., Langford, W.F.: Hopf bifurcation with non-semisimple 1:1 resonance. Nonlinearity 3, 825–850 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  27. Luongo, A., Egidio, A.D., Paolone, A.: Multiscale analysis of defective multiple-Hopf bifurcations. Comput. Struct. 82(31–32), 2705–2722 (2004). doi:10.1016/j.compstruc.2004.04.022

    Article  Google Scholar 

  28. Luongo, A., Egidio, A.: Bifurcation equations through multiple-scales analysis for a continuous model of a planar beam. Nonlinear Dyn. 41(1–3), 171–190 (2005). doi:10.1007/s11071-005-2804-1

    Article  MATH  Google Scholar 

  29. Luongo, A., Paolone, A.: On the reconstitution problem in the multiple time-scale method. Nonlinear Dyn. 19(2), 135–158 (1999). doi:10.1023/A:1008330423238

    Article  MathSciNet  Google Scholar 

  30. Anegawa, N., Fujiwara, H., Matsushita, O.: Vibration diagnosis featuring blade-shaft coupling effect of turbine rotor models. J. Eng. Gas Turbines Power 133(2), 022501–022508 (2011)

    Article  Google Scholar 

  31. Chiu, Y.-J., Chen, D.-Z.: The coupled vibration in a rotating multi-disk rotor system. Int. J. Mech. Sci. 53(1), 1–10 (2011). doi:10.1016/j.ijmecsci.2010.10.001

    Article  MathSciNet  Google Scholar 

  32. Diken, H., Alnefaie, K.: Effect of unbalanced rotor whirl on blade vibrations. J. Sound Vib. 330(14), 3498–3506 (2011). doi:10.1016/j.jsv.2011.02.017

    Article  Google Scholar 

  33. Wang, L., Cao, D.Q., Huang, W.: Nonlinear coupled dynamics of flexible blade-rotor-bearing systems. Tribol. Int. 43(4), 759–778 (2010). doi:10.1016/j.triboint.2009.10.016

    Article  Google Scholar 

  34. Ghazavi, M.R., Najafi, A., Jafari, A.A.: Bifurcation and nonlinear analysis of nonconservative interaction between rotor and blade row. Mech. Mach. Theory 65(0), 29–45 (2013). doi:10.1016/j.mechmachtheory.2013.02.008

  35. Younesian, D., Esmailzadeh, E.: Non-linear vibration of variable speed rotating viscoelastic beams. Nonlinear Dyn. 60(1–2), 193–205 (2010). doi:10.1007/s11071-009-9589-6

    Article  MATH  Google Scholar 

  36. Bridges, T.J.: Bifurcation of periodic solutions near a collision of eigenvalues of opposite signature. Math. Proc. Cambridge Philos. Soc. 108, 575–601 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  37. Bolotin, V.V.: Nonconservative Problems of the Theory of Elastic Stability. Pergamon, New York (1963)

    MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, Tarbiat Modares University, Tehran, Iran

    Asghar Najafi & Mohammad-Reza Ghazavi

  2. Department of Mechanical Engineering, K.N. Toosi University of Technology, Tehran, Iran

    Ali-Asghar Jafari

Authors
  1. Asghar Najafi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mohammad-Reza Ghazavi
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Ali-Asghar Jafari
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mohammad-Reza Ghazavi.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Najafi, A., Ghazavi, MR. & Jafari, AA. Stability and Hamiltonian Hopf bifurcation for a nonlinear symmetric bladed rotor. Nonlinear Dyn 78, 1049–1064 (2014). https://doi.org/10.1007/s11071-014-1495-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-014-1495-x

Keywords

Search

Navigation