Skip to main content
SpringerLink
Log in

Local symmetry dynamics in one-dimensional aperiodic lattices: a numerical study

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

A unifying description of lattice potentials generated by aperiodic one-dimensional sequences is proposed in terms of their local reflection or parity symmetry properties. We demonstrate that the ranges and axes of local reflection symmetry possess characteristic distributional and dynamical properties, determined here numerically for certain lattice types. A striking aspect of such a property is given by the return maps of sequential spacings of local symmetry axes, which typically traverse few-point symmetry orbits. This local symmetry dynamics allows for a description of inherently different aperiodic lattices according to fundamental symmetry principles. Illustrating the local symmetry distributional and dynamical properties for several representative binary lattices, we further show that the renormalized axis-spacing sequences follow precisely the particular type of underlying aperiodic order, revealing the presence of dynamical self-similarity. Our analysis thus provides evidence that the long-range order of aperiodic lattices can be characterized in a compellingly simple way by its local symmetry dynamics.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

Notes

  1. Since we perform a numerical investigation of the considered aperiodic sequences, throughout the paper the notion of asymptotic convergence is not used in a mathematically strict sense, but refers to the observed behavior for large number of corresponding iterations.

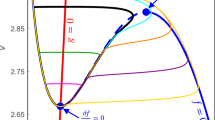
  2. The first and second range subsequences would be \(L_0, L_{0+m}, L_{0+2m}, ...\) and \(L_{1}, L_{1+m}, L_{1+2m}, ...\), respectively. Thus, in order to consider the very first \(m\)-tet bundle \(L_0, L_1, ..., L_m\), one has to include, apart from the single-letter maximal palindromes with length \(L_1 = 1\), also the empty maximal palindromes \(\epsilon \) of length \(L_0 = 0\). The latter are the “interfaces” between two different letters \(A\) and \(B\) in a word. Since \(L_0\) and \(L_1\) are not plotted throughout our analysis, we describe the \(m\)-tets starting from the second bundle \(L_{0+m}, L_{1+m}, ..., L_{m+m}\).

References

  1. Maciá, E.: The role of aperiodic order in science and technology. Rep. Prog. Phys. 69, 397 (2006)

    Article  Google Scholar 

  2. Kohmoto, M., Sutherland, B., Tang, C.: Critical wave functions and a Cantor-set spectrum of a one-dimensional quasicrystal model. Phys. Rev. B 35, 1020 (1987)

    Article  MathSciNet  Google Scholar 

  3. Maciá, E., Domínguez-Adame, F.: Physical nature of critical wave functions in Fibonacci systems. Phys. Rev. Lett. 76, 2957 (1996)

    Article  Google Scholar 

  4. Kohmoto, M., Sutherland, B., Iguchi, K.: Localization of optics: quasiperiodic media. Phys. Rev. Lett. 58, 2436 (1987)

    Article  Google Scholar 

  5. Gellermann, W., Kohmoto, M., Sutherland, B., Taylor, P.C.: Localization of light waves in Fibonacci dielectric multilayers. Phys. Rev. Lett. 72, 633 (1994)

    Article  Google Scholar 

  6. Huang, X.Q., Jiang, S.S., Peng, R.W., Hu, A.: Perfect transmission and self-similar optical transmission spectra in symmetric Fibonacci-class multilayers. Phys. Rev. B 63, 245104 (2001)

    Article  Google Scholar 

  7. Zhukovsky, S.V.: Perfect transmission and highly asymmetric light localization in photonic multilayers. Phys. Rev. A 81, 053808 (2010)

    Article  Google Scholar 

  8. Thiem, S., Schreiber, M.: Photonic properties of metallic-mean quasiperiodic chains. Eur. Phys. J. B 76, 339 (2010)

    Article  Google Scholar 

  9. Thiem, S., Schreiber, M., Grimm, U.: Light transmission through metallic-mean quasiperiodic stacks with oblique incidence. Philos. Mag. 91, 2801 (2011)

    Article  Google Scholar 

  10. Maciá, E.: Exploiting aperiodic designs in nanophotonic devices. Rep. Prog. Phys. 75, 036502 (2012)

    Article  Google Scholar 

  11. Hof, A., Knill, O., Simon, B.: Singular continuous spectrum for palindromic Schrödinger operators. Commun. Math. Phys 174, 149 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  12. Damanik, D., Ghez, J.-M., Raymond, L.: A palindromic half-line criterion for absence of eigenvalues and applications to substitution hamiltonians. Ann. Henri Poincaré 2, 927 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  13. Damanik, D., Hundertmark, D.: Reflection symmetries and absence of eigenvalues for one-dimensional Schrödinger operators. Proc. Am. Math. Soc. 132, 1957 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  14. Kalozoumis, P.A., Morfonios, C., Diakonos, F.K., Schmelcher, P.: Local symmetries in one-dimensional quantum scattering. Phys. Rev. A 87, 032113 (2013)

    Article  Google Scholar 

  15. Kalozoumis, P.A., Morfonios, C., Palaiodimopoulos, N., Diakonos, F.K., Schmelcher, P.: Local symmetries and perfect transmission in aperiodic photonic multilayers. Phys. Rev. A 88, 033857 (2013)

    Article  Google Scholar 

  16. Kalozoumis, P.A., Morfonios, C., Diakonos, F.K., Schmelcher, P.: Invariants of broken discrete symmetries. arXiv:1403.7149 (2014).

  17. Maciá, E.: Exploiting quasiperiodic order in the design of optical devices. Phys. Rev. B 63, 205421 (2001)

    Article  Google Scholar 

  18. Dal, Negro L., Oton, C.J., Gaburro, Z., Pavesi, L., Johnson, P., Lagendijk, A., Righini, R., Colocci, M., Wiersma, D.S.: Light transport through the band-edge states of Fibonacci quasicrystals. Phys. Rev. Lett. 90, 055501 (2003)

    Article  Google Scholar 

  19. Dal, Negro L., Boriskina, S.: Deterministic aperiodic nanostructures for photonics and plasmonics applications. Laser Photon. Rev. 6, 178 (2012)

    Article  Google Scholar 

  20. Poddubny, A., Ivchenko, E.: Photonic quasicrystalline and aperiodic structures. Phys. E 42, 1871 (2010)

    Article  Google Scholar 

  21. Droubay, X.: Palindromes in the Fibonacci word. Inf. Proc. Lett. 55, 217 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  22. de Luca, A.: Sturmian words: structure, combinatorics, and their arithmetics. Theor. Comput. Sci. 183, 45 (1997)

    Article  MATH  Google Scholar 

  23. Droubay, X., Pirillo, G.: Palindromes and Sturmian words. Theor. Comput. Sci. 223, 73 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  24. Allouche, J.-P., Baake, M., Cassaigne, J., Damanik, D.: Palindrome complexity. Theor. Comput. Sci. 292, 9 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  25. Borel, J.-P., Reutenauer, C.: Palindromic factors of billiard words. Theor. Comput. Sci. 340, 334 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  26. de Luca, A., De Luca, A.: Palindromes in Sturmian Words. Lect. Notes Comput. Sci. 3572, 199 (2005)

    Article  Google Scholar 

  27. Glen, A.: Occurrences of palindromes in characteristic Sturmian words. Theor. Comput. Sci. 352, 31 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  28. Glen, A., Justin, J., Widmer, S., Zamboni, L.Q.: Palindromic richness. Eur. J. Comb. 30, 510 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  29. Anisiu, M.-C., Anisiu, V., Kása, Z.: Properties of palindromes in finite words. Pure Math. Appl. 17, 183 (2006)

    MATH  Google Scholar 

  30. Damanik, D.: Local symmetries in the period-doubling sequence. Discret. Appl. Math. 100, 115 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  31. de Luca, A., De Luca, A.: Combinatorial properties of Sturmian palindromes. Int. J. Found. Comput. Sci. 17, 557 (2006)

    Article  MATH  Google Scholar 

  32. Fischler, S.: Palindromic prefixes and episturmian words. J. Comb. Theor. A 113, 1281–1282 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  33. Tomohiro, I., Inenaga, S., Bannai, H., Takeda, M.: Counting and verifying maximal palindromes. Lect. Notes Comput. Sci. 6393, 135 (2010)

    Article  Google Scholar 

  34. Kolpakov, R., Kucherov, G.: Searching for gapped palindromes. Theor. Comput. Sci. 410, 5365 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  35. Lu, L., Jia, H., Dröge, P., Li, J.: The human genome-wide distribution of DNA palindromes. Funct. Integr. Genomics 7, 221 (2007)

    Article  Google Scholar 

  36. Ravsky, O.: On the palindromic decomposition of binary words. J. Autom. Lang. Comb. 8, 75 (2003)

    MathSciNet  MATH  Google Scholar 

  37. Blondin-Massé, A., Brlek, S., Labbé, S.: Palindromic lacunas of the Thue-Morse word. In: Proceedings of 6th international conference on random generation of combinatorial structures, p. 53. Arezzo, Italia, 16–20 June 2008 .

  38. Brlek, S., Hamel, S., Nivat, M., Reutenauer, C.: On the palindromic complexity of infinite words. Int. J. Found. Comput. Sci. 15, 293 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  39. Frid, A., Puzynina, S., Zamboni, L.: On minimal factorizations of words as products of palindromes. Adv. Appl. Math. 50, 737 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  40. Zhi-Xiong, W., Zhi-Ying, W.: Some properties of the singular words of the Fibonacci word. Eur. J. Comb. 15, 587 (1994)

    Article  MATH  Google Scholar 

  41. Chuan, W.-F., Ho, H.-L.: Locating factors of a characteristic word via the generalized Zeckendorf representation of numbers. Theor. Comput. Sci. 440, 39 (2012)

    Article  MathSciNet  Google Scholar 

  42. Wang, X., Grimm, U., Schreiber, M.: Trace and antitrace maps for aperiodic sequences: extensions and applications. Phys. Rev. B 62, 14020 (2000)

    Article  Google Scholar 

  43. Allouche, J.-P., Shallit, J.: Automatic Sequences: Theory, Applications, Generalizations. Cambridge University Press, New York (2003)

    Book  Google Scholar 

  44. Baake, M.: A note on palindromicity. Lett. Math. Phys. 49, 217 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  45. Moody, R.: Model sets: a survey. In: Axel, F., Dénoyer, F., Gazeau, J.P. (eds.) From Quasicrystals to More Complex Systems, p. 145. Springer, Berlin (2000)

    Chapter  Google Scholar 

  46. Guimond, L.-S., Masáková, Z., Pelantová, E.: Combinatorial properties of infinite words associated with cut-and-project sequences. J. Theor. Nombres Bordeaux 15, 697 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  47. de Luca, A.: A division property of the Fibonacci word. Inf. Proc. Lett. 54, 307 (1995)

    Article  MATH  Google Scholar 

  48. Thiem, S., Schreiber, M.: Renormalization group approach for the wave packet dynamics in golden-mean and silver-mean labyrinth tilings. Phys. Rev. B 85, 224205 (2012)

    Article  Google Scholar 

  49. Thiem, S., Schreiber, M.: Wave packet dynamics, ergodicity, and localization in quasiperiodic chains. J. Phys. Condens. Matter 25, 075503 (2012)

    Article  Google Scholar 

  50. Bellissard, J., Bovier, A., Ghez, J.-M.: Spectral properties of a tight binding Hamiltonian with period doubling potential. Commun. Math. Phys. 135, 379 (1991)

  51. Damanik, D.: Singular continuous spectrum for the period doubling Hamiltonian on a set of full measure. Commun. Math. Phys. 196, 477 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  52. Baake, M., Grimm, U.: Surprises in aperiodic diffraction. J. Phys. Conf. Ser. 226, 012023 (2010)

    Article  Google Scholar 

  53. Allouche, J.-P., Shallit, J.: The ubiquitous Prouhet-Thue-Morse sequence. In: Ding, C., Helleseth, T., Niederreiter, H. (eds.) Sequences and their Applications, p. 1. Springer, London (1999)

    Chapter  Google Scholar 

  54. Blondin-Massé, A., Brlek, S., Garon, A., Labbé, S.: Combinatorial properties of \(f\)-palindromes in the Thue-Morse sequence. Pure Math. Appl. 19, 39 (2008)

    Google Scholar 

  55. Blondin-Massé, A., Brlek, S., Frosini, A., Labbé, S., Rinaldi, S.: Reconstructing words from a fixed palindromic length sequence. Int. Fed. Inf. Process. 273, 101 (2008)

    Google Scholar 

  56. Sengupta, S., Chakrabarti, A., Chattopadhyay, S.: Electronic properties of a Cantor lattice. Physica B 344, 307 (2004)

    Article  Google Scholar 

  57. Esaki, K., Sato, M., Kohmoto, M.: Wave propagation through Cantor-set media: chaos, scaling, and fractal structures. Phys. Rev. E 79, 056226 (2009)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgments

This research has been co-financed by the European Union (European Social Fund—ESF) and Greek national funds through the Operational Program “Education and Lifelong Learning” of the National Strategic Reference Framework (NSRF) - Research Funding Program: Heracleitus II. Investing in knowledge society through the European Social Fund. Further financial support by the Greek Scholarship Foundation IKY in the framework of an exchange program with Germany (IKYDA) is also acknowledged.

Author information

Authors and Affiliations

  1. Zentrum für Optische Quantentechnologien, Universität Hamburg, Luruper Chaussee 149, 22761 , Hamburg, Germany

    C. Morfonios & P. Schmelcher

  2. The Hamburg Centre for Ultrafast Imaging, Universität Hamburg, Luruper Chaussee 149, 22761 , Hamburg, Germany

    P. Schmelcher

  3. Department of Physics, University of Athens, 15771 , Athens, Greece

    P. A. Kalozoumis & F. K. Diakonos

Authors
  1. C. Morfonios
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. P. Schmelcher
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. P. A. Kalozoumis
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. F. K. Diakonos
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to C. Morfonios.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Morfonios, C., Schmelcher, P., Kalozoumis, P.A. et al. Local symmetry dynamics in one-dimensional aperiodic lattices: a numerical study. Nonlinear Dyn 78, 71–91 (2014). https://doi.org/10.1007/s11071-014-1422-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-014-1422-1

Keywords

Search

Navigation