Skip to main content
SpringerLink
Log in

Distributed cooperative control for multiple quadrotor systems via dynamic surface control

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

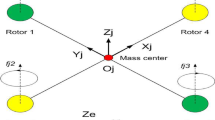
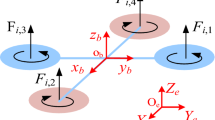
Abstract

This paper addresses the cooperative control problems of multiple quadrotor systems under fixed directed communication topologies. Dynamic surface control (DSC) is utilized to design nonlinear consensus controllers to make multiple quadrotors construct and keep a formation during flying. The control scheme is distributed so that each quadrotor updates the values of its information states based on the information states of its neighbors and its own states. Moreover, the leader–follower case is also discussed. In this case, distributed continuous schemes are also proposed with distributed sliding-mode observers, whose function is to estimate two linear combinations of the leader’s velocity and acceleration accurately in finite time. Finally, the effectiveness of the theoretical results is demonstrated via two examples.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. Altug, E., Osreiwski, J.O., Mahony, R.: Control of a quadrotor helicopter using visual feedback. In: Proc. IEEE. Conf. Robotics and Automation, Washington, DC, pp. 72–77 (2002)

    Google Scholar 

  2. Madahi, T., Benallegue, A.: Control of a quadrotor mini-helicopter via full state backstepping technique. In: Proc. IEEE. Conf. on Decision and Control, San Diego, pp. 1515–1520 (2006)

    Chapter  Google Scholar 

  3. Castillo, P., Lozano, R., Dzul, A.: Stabilization of a mini rotorcraft having four rotors. IEEE Control Syst. Mag. 25(6), 45–55 (2005)

    Article  MathSciNet  Google Scholar 

  4. Das, A., Subbarao, K., Lweis, F.: Dynamic inversion with zero-dynamics stabilisation for quadrotor control. IET Control Theory Appl. 3(3), 303–314 (2008)

    Article  Google Scholar 

  5. Xü, R., Ümit, Ö.: Sliding mode control of a class of underactuated systems. Automatica 44, 233–241 (2008)

    Article  Google Scholar 

  6. Guerreoro, J.A., Castillo, P., Salazar, S., Lozano, R.: Mini rotorcraft flight formation control using bounded inputs. J. Intell. Robot. Syst. 12(6), 175–186 (2012)

    Article  Google Scholar 

  7. Zhou, J., Wang, Q.: Distributed discrete-time nonlinear consensus protocols. In: Proc. IEEE. Conf. on Decision and Control, Shanghai, pp. 4759–4764 (2009)

    Google Scholar 

  8. Alon, D., Nadav, B., Shai, A.: Formation flight using multiple integral backstepping controllers. In: Proc. IEEE. Conf. on Cybernetics and Intelligent Systems, Qingdao, pp. 317–322 (2011)

    Google Scholar 

  9. Matthew, T., Nathan, M., Vijay, K.: Decentralized formation control with variable shapes for aerial robots. In: Proc. IEEE. Conf. on Robotics and Automation, Saint Paul, pp. 23–30 (2012)

    Google Scholar 

  10. Olfati-Saber, R., Murray, R.M.: Consensus problems in networks of agents with switching topology and time-delays. IEEE Trans. Autom. Control 49(9), 1520–1533 (2004)

    Article  MathSciNet  Google Scholar 

  11. Olfati-Saber, R., Fax, J.A., Murray, R.M.: Consensus and cooperation in networked multiagent systems. Proc. IEEE 95(1), 215–233 (2007)

    Article  Google Scholar 

  12. Liu, J., Liu, Z., Chen, Z.: Coordinative control of multi-agent systems using distributed nonlinear output regulation. Nonlinear Dyn. 67(3), 1871–1881 (2007)

    Article  Google Scholar 

  13. Ren, W., Beard, R.W., Atkins, E.M.: Information consensus in multivehicle cooperative control. IEEE Control Syst. Mag. 27(2), 71–82 (2007)

    Article  Google Scholar 

  14. Bauso, D., Giarré, L., Pesenti, R.: Non-linear protocols for optimal distributed consensus in networks of dynamic agents. Syst. Control Lett. 55(11), 918–928 (2006)

    Article  MATH  Google Scholar 

  15. Mei, J., Ren, W., Ma, G.: Distributed coordinated tracking with a dynamic leader for multiple Euler–Lagrange systems. IEEE Trans. Autom. Control 56(6), 1415–1421 (2010)

    Article  MathSciNet  Google Scholar 

  16. Mei, J., Ren, W., Ma, G.: Distributed containment control for Lagrangian networks with parametric uncertainties under a directed graph. Automatica 48(4), 653–659 (2012)

    Article  MATH  MathSciNet  Google Scholar 

  17. Cao, Y., Ren, W., Meng, Z.: Decentralized finite-time sliding mode estimators and their applications in decentralized finite-time formation tracking. Syst. Control Lett. 59(9), 522–529 (2012)

    Article  MathSciNet  Google Scholar 

  18. Arcak, P.: Passivity as a design tool for group coordination. IEEE Trans. Autom. Control 56(6), 1415–1421 (2010)

    MathSciNet  Google Scholar 

  19. Yip, P.P., Hedrick, J.K.: Adaptive dynamic surface control: a simplified algorithm for adaptive backstepping control of nonlinear systems. Int. J. Control 71(5), 959–979 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  20. Swarrop, D., Hedrick, J.K., Yip, P.P., Gerdes, J.C.: Dynamic surface control of a class of nonlinear systems. IEEE Trans. Autom. Control 45(10), 1893–1899 (2000)

    Article  Google Scholar 

  21. Das, A., Lwies, F.L.: Cooperative adaptive control for synchronization of second-order systems with unknown nonlinearities. Int. J. Robust Nonlinear Control 21(13), 1509–1524 (2011)

    Article  MATH  Google Scholar 

  22. Ren, W., Beard, R.W.: Distributed Consensus in Multi-Vehicle Cooperative Control. Springer, London (2008)

    MATH  Google Scholar 

  23. Horn, R., Johnson, C.: Matrix Analysis. Cambridge University Press, New York (1985)

    Book  MATH  Google Scholar 

  24. Godsil, C., Royle, G.: Algebraic Graph Theory. Springer, New York (2001)

    Book  MATH  Google Scholar 

Download references

Acknowledgements

This work was supported by the National Natural Science Foundation of P.R. China under Grant 61074031 and the Program for Changjiang Scholars and Innovative Research Team in University under Grant IRT1208.

Author information

Authors and Affiliations

  1. Beijing Institute of Technology, Beijing, China

    Yinqiu Wang, Qinghe Wu & Yao Wang

Authors
  1. Yinqiu Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Qinghe Wu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Yao Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yinqiu Wang.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Wang, Y., Wu, Q. & Wang, Y. Distributed cooperative control for multiple quadrotor systems via dynamic surface control. Nonlinear Dyn 75, 513–527 (2014). https://doi.org/10.1007/s11071-013-1081-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-013-1081-7

Keywords

Search

Navigation