Abstract
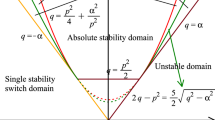
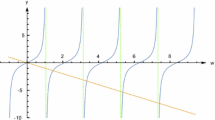
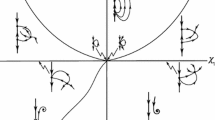
In this paper, we aim to investigate the dynamics of a system of Van der Pol–Duffing oscillators with delay coupling. First, taking the time delay as a bifurcation parameter, the stability of the equilibrium, and the existence of Hopf bifurcation are investigated. Then using the center manifold reduction technique and normal form theory, we give the direction of the Hopf bifurcation. And then by means of the symmetric bifurcation theory for delay differential equations and the representation theory of groups, we claim the bifurcation periodic solution induced by time delay is antiphase locked oscillation. Finally, at the end of the paper, numerical simulations are carried out to support our theoretical analysis.




Similar content being viewed by others
References
Belykh, V.N., Pankratova, E.V.: Chaotic dynamics of two Van der Pol–Duffing oscillators with Huygens coupling. Regul. Chaotic Dyn. 15, 274–284 (2010)
Benford, J., Sze, H., Woo, W., Smith, R.R., Harteneck, B.: Phase locking of relativistic magnetrons. Phys. Rev. Lett. 62, 969 (1989)
Bi, Q.S.: Dynamical analysis of two coupled parametrically excited Van der Pol oscillators. Int. J. Non-Linear Mech. 39, 33–54 (2004)
Chow, C., Mallet-Paret, J.: Integral averaging and bifurcation. J. Differ. Equ. 26, 112–159 (1977)
Collins, J.J., Stewart, I.N.: Coupled nonlinear oscillators and the symmetries of animal gaits. J. Nonlinear Sci. 3, 349 (1993)
Daido, H.: Multibranch entrainment and scaling in large populations of coupled oscillators. Phys. Rev. Lett. 77, 1406 (1996)
Golubitsky, M., Stewart, I., Schaeffer, D.: Singularities and Groups in Bifurcation Theory, Vol. II. Springer, New York (1988)
Hale, J.K., Lunel, S.M.V.: Introduction to Functional Differential Equations. Springer, New York (1993)
Hassard, B.D., Kazarinoff, N.D., Wan, Y.H.: Theory and Applications of Hopf Bifurcation. Cambridge University Press, Cambridge (1981)
Ioos, G., Joseph, D.: Elementary Stability and Bifurcation Theory. Springer, New York (1980)
Ji, J., Zhang, N.: Additive resonances of a controlled Van der Pol–Duffing oscillator. J. Sound Vib. 315, 22–33 (2008)
Kuznetsov, A.P., Stankevich, N.V., Turukina, L.V.: Coupled Van der Pol–Duffing oscillators: phase dynamics and structure of synchronization tongues. Physica D 238, 1203–1215 (2009)
Maccari, A.: Vibration amplitude control for a Van der Pol–Duffing oscillator with time delay. J. Sound Vib. 317, 20–29 (2008)
Nakajima, K., Sawada, Y.: Experimental studies on the weak coupling of oscillatory chemical reaction systems. J. Chem. Phys. 72, 2231 (1980)
Nayfeh, A.H.: The Method of Normal Forms Second, Updated and Enlarged Edition. Wiley-VCH, Boschstr (2011)
Nayfeh, A.H.: Order reduction of retarded nonlinear systems—the method of multiple scales versus center-manifold reduction. Nonlinear Dyn. 51, 483–500 (2008)
Njah, A.N.: Synchronization and anti-synchronization of double hump Duffing–Van der Pol oscillators via active control. J. Inf. Comput. Sci. 4(4), 243–250 (2009)
Njah, A.N., Vincent, U.E.: Chaos synchronization between single and double wells Duffing–Van der Pol oscillators using active control. Chaos Solitons Fractals 37, 1356–1361 (2008)
Norimichi, H., Slawomir, R.: Existence of limit cycles for coupled Van der Pol equations. J. Differ. Equ. 195, 194–209 (2003)
Pastor, I., Pérez-García, V.M., Encinas, F., GuerraI, J.M.: Ordered and chaotic behaviour of two coupled Van der Pol oscillators. Phys. Rev. E 48, 171–182 (1993)
Pecora, L.M.: Synchronization conditions and desynchronizing patterns in coupled limit cycle and chaotic systems. Phys. Rev. E 58, 347 (1998)
Reddy, D.V.R., Sen, A., Johnston, G.L.: Time delay induced death in coupled limit cycle oscillators. Phys. Rev. Lett. 82, 648–672 (1999)
Rompala, K., Rand, R., Howland, H.: Dynamics of three coupled Van der Pol oscillators with application to circadian rhythms. Commun. Nonlinear Sci. Numer. Simul. 12, 794803 (2007)
Shiino, M., Frankowicz, M.: Synchronization of infinitely many coupled limit-cycle oscillators. Phys. Lett. A 136, 103 (1989)
Song, Y.L.: Hopf bifurcation and spatio-temporal patterns in delay-coupled Van der Pol oscillators. Nonlinear Dyn. 63, 223–237 (2011)
Song, Y.L., Wei, J., Yuan, Y.: Stability switches and Hopf bifurcation in a pair of delay-coupled oscillations. J. Nonlinear Sci. 17, 145–166 (2007)
Wiggins, S.: Introduction to Applied Nonlinear Dynamical Systems and Chaos. Springer, New York (2010)
Wirkus, S., Rand, R.: The dynamics of two coupled Van der Pol oscillators with delay coupling. Nonlinear Dyn. 30, 205–210 (2002)
Wu, J.: Symmetric functional-differential equations and neural networks with memory. Trans. Am. Math. Soc. 350, 4799–4838 (1998)
Yamapi, R., Filatrella, G.: Strange attractors and synchronization dynamics of coupled Van der Pol–Duffing oscillators. Commun. Nonlinear Sci. Numer. Simul. 13, 1121–1130 (2008)
Zhang, J., Gu, X.: Stability and bifurcation analysis in the delay-coupled Van der Pol oscillators. Appl. Math. Model. 34, 2291–2299 (2009)
Acknowledgements
The authors thank the referees for their valuable suggestions and comments concerning improving the work. The authors would like to acknowledge the support from National Natural Science Foundation of China (11101318 and 11001212).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Zang, H., Zhang, T. & Zhang, Y. Stability and bifurcation analysis of delay coupled Van der Pol–Duffing oscillators. Nonlinear Dyn 75, 35–47 (2014). https://doi.org/10.1007/s11071-013-1047-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11071-013-1047-9