Abstract
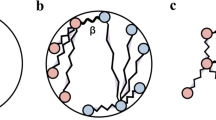
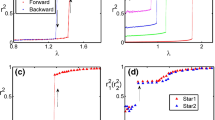
This paper presents a detailed analysis on the dynamics of a ring network with small world connection. On the basis of Lyapunov stability approach, the asymptotic stability of the trivial equilibrium is first investigated and the delay-dependent criteria ensuring global stability are obtained. The existence of Hopf bifurcation and the stability of periodic solutions bifurcating from the trivial equilibrium are then analyzed. Further studies are paid to the effects of small world connection on the stability interval and the stability of periodic solution. In particular, some complex dynamical phenomena due to short-cut strength are observed numerically, such as: period-doubling bifurcation and torus breaking to chaos, the coexistence of multiple periodic solutions, multiple quasi-periodic solutions, and multiple chaotic attractors. The studies show that small world connection may be used as a simple but efficient “switch” to control the dynamics of a system.
Similar content being viewed by others
References
Pandit, S.A., Amritkar, R.E.: Characterization and control of small-world networks. Phys. Rev. E 60, 1119–1122 (1999)
Boccaletti, S., Latora, V., Moreno, Y., Chavez, M., Hwang, D.U.: Complex networks: structure and dynamics. Phys. Rep. 424, 175–308 (2006)
Newman, M.E.J., Morre, C., Watts, D.J.: Mean-field solution of the small-world network model. Phys. Rev. Lett. 84, 3201–3204 (2000)
Wang, X.F., Chen, G.: Synchronization in small-world dynamical networks. Int. J. Bifur. Chaos 12, 187–192 (2002)
Kulkarni, R.V., Almaas, E., Stroud, D.: Exact results and scaling properties of small-world networks. Phys. Rev. E 61, 4268–4271 (2000)
Belykh, I.V., Belykh, V.N., Hasler, M.: Blinking model and synchronization in small-world networks with a time-varying coupling. Physica D 195, 188–206 (2004)
Zekri, N., Porterie, B., Clerc, J.P., Loraud, J.C.: Propagation in a two-dimensional weighted local small-world network. Phys. Rev. E 71, 046121–046125 (2005)
Xu, X., Hu, H.Y., Wang, H.L.: Dynamics of a two dimensional delayed small-world network under delayed feedback control. Int. J. Bifur. Chaos 16, 3257–3273 (2006)
Yang, X.S.: Chaos in small-world networks. Phys. Rev. E 63, 046206 (2001)
Yang, X.H.: Fractals in small-world networks with time-delay. Chaos Solitons Fractals 13, 215–219 (2002)
Li, C., Chen, G.: Local stability and Hopf bifurcation in small-world delayed networks. Chaos Solitons Fractals 20, 353–361 (2004)
Watts, D.J., Strogatz, S.H.: Collective dynamics of ‘small-world’ networks. Nature 393, 440–442 (1998)
Latora, V., Marchiori, M.: Efficient behavior of small-world networks. Phys. Rev. Lett. 87, 198701 (2001)
Marchiori, M., Latora, V.: Harmony in the small-world. Physica A 285, 539 (2000)
Barabasi, A.L., Jeong, H., Ravasz, R., Neda, Z., Viscek, T., Schubert, A.: Evolution of the social network of scientific collaborations. Physica A 311, 590–614 (2002)
Plois, G.A.: Stability is woven by complex webs. Nature 395, 744–745 (1998)
McCann, K., Hastings, A., Huxel, G.R.: Weak tropic interactions and the balance of nature. Nature 395, 794–798 (1998)
Berlow, E.L.: Strong effects of weak interactions in ecological communities. Nature 398, 330–334 (1999)
Sporns, O., Tononi, G., Edelman, G.M.: Connectivity and complexity: the relationship between neuroanatomy and brain dynamics. Neural Netw. 13, 909 (2002)
Latora, V., Marchiori, M.: Economic small-world behavior in weighted networks. Eur. Phys. J. B 32, 249–263 (2003)
Campbell, S.A.: Stability and bifurcation of a simple neural network with multiple time delays. Fields Inst. Commun. 21, 65–78 (1999)
Campbell, S.A., Yuan, Y., Bungay, S.D.: Equivariant Hopf bifurcation in a ring of identical cells with delayed coupling. Nonlinearity 18, 2827–2846 (2005)
Guo, S., Huang, L.: Hopf bifurcation periodic orbits in a ring of neurons with delays. Physica D 183, 19–44 (2003)
Xu, X.: Complicated dynamics of a ring neural network with time delays. J. Phys. A: Math. Theory 41, 035102 (2008)
Gopalsamy, K., He, X.Z.: Delay-independent stability in bidirectional associative memory networks. IEEE Trans. Neural Netw. 5, 998–1002 (1994)
Gopalsamy, K.: Stability and Oscillations in Delay Differential Equations of Population Dynamics. Kluwer Academic, Dordrecht (1992)
Wu, J.: Symmetric functional differential equations and neural networks with memory. Trans. Am. Math. Soc. 350, 4799–4838 (1998)
Hu, H.Y., Wang, Z.H.: Dynamics of Controlled Mechanical Systems with Feedback Time Delays. Springer, Heidelberg (2002)
Hassard, B.D., Kazarinoff, N.D., Wan, Y.H.: Theory and Applications of Hopf Bifurcation. Cambridge University Press, Cambridge (1981)
Ermentrout, B.: XPPAUT 3.0—The Differential Equations Tool. University of Pittsburgh, Pittsburgh (1997)
Balanov, A.G., Janson, N.B., Schöll, E.: Delayed feedback control of chaos: bifurcation analysis. Phys. Rev. E 71, 9 (2005) 016222-1
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Xu, X., Wang, Z.H. Effects of small world connection on the dynamics of a delayed ring network. Nonlinear Dyn 56, 127–144 (2009). https://doi.org/10.1007/s11071-008-9384-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11071-008-9384-9