The Damped Nonlinear Quasiperiodic Mathieu Equation Near 2:2:1 Resonance

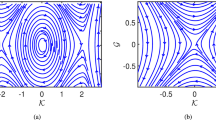
Abstract

We investigate the damped cubic nonlinear quasiperiodic Mathieu equation

$$ \frac{d^2x}{dt^2}+(\delta+\varepsilon \cos t+\varepsilon \mu \cos\omega t)x+\varepsilon \mu c\frac{dx}{dt}+\varepsilon \mu \gamma x^3=0$$

in the vicinity of the principal 2:2:1 resonance. By using a double perturbation method which assumes that both ε and μ are small, we approximate analytical conditions for the existence and bifurcation of nonlinear quasiperiodic motions in the neighborhood of the middle of the principal instability region associated with 2:2:1 resonance. The effect of damping and nonlinearity on the resonant quasiperiodic motions of the quasiperiodic Mathieu equation is also provided. We show that the existence of quasiperiodic solutions does not depend upon the nonlinearity coefficient γ, whereas the amplitude of the associated quasiperiodic motion does depend on γ.