Skip to main content
SpringerLink
Log in

Three new triangular shell elements of ANCF represented by Bézier triangles

  • Published:
Multibody System Dynamics Aims and scope Submit manuscript

Abstract

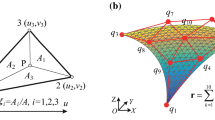
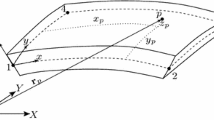
The past few years have witnessed rapid advances in the integration of Computer-Aided Design (CAD) and Finite Element Analysis (FEA), such as the Isogeometric Analysis (IGA) method and the Integration of Computer Aided Design and Analysis (I-CAD-A) technique. Recent studies indicated that the geometry description used in the Absolute Nodal Coordinate Formulation (ANCF) is consistent with the geometry description in CAD systems, making it an attractive approach for I-CAD-A. ANCF as an accurate, non-incremental finite element method to deal with the dynamics of those systems subject to large motions and large deformations has developed towards practical application in the field of flexible multibody systems. The objective of this study is to enrich the family of ANCF elements with three new triangular shell elements: the thin triangular element, the low order thick triangular element and the high order thick triangular element, which are represented by Bézier triangles. The linear mapping relationship between the geometrical representation of the Bézier triangle and that of the proposed thin triangular element is established. The invertible property of the linear mapping relationship clearly indicates that the proposed thin triangular element can be also used to perform the I-CAD-A. The conditions of the approximate \(G^{1}\) continuity between the proposed adjacent elements are also deduced in order to construct a smooth surface. Finally, several static and dynamic numerical examples are employed to validate the correctness and effectiveness of the proposed triangular shell elements.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25
Fig. 26
Fig. 27
Fig. 28

Similar content being viewed by others

References

  1. Farin, G.: Curves and Surfaces for CAGD: A Practical Guide. Morgan Kaufmann, San Mateo (2001)

    Google Scholar 

  2. Ernens, D.: Finite element methods with exact geometry representation. Master’s Thesis, Delft University of Technology (2011)

  3. Piegl, L., Tiller, W.: The NURBS Book, 2nd edn. Springer, Berlin (1997)

    Book  Google Scholar 

  4. Rogers, D.F.: An Introduction to NURBS: With Historical Perspective. Morgan Kaufmann, San Mateo (2001)

    Google Scholar 

  5. Shabana, A.A.: Computational Dynamics, 2nd edn. Wiley, New York (2001)

    MATH  Google Scholar 

  6. Shabana, A.A.: Uniqueness of the geometric representation in large rotation finite element formulations. J. Comput. Nonlinear Dyn. 5, 044501 (2010)

    Article  Google Scholar 

  7. Cottrell, J.A., Hughes, T.J.R., Bazilevs, Y.: Isogeometric Analysis—Toward Integration of CAD and FEA. Wiley, New York (2009)

    Book  Google Scholar 

  8. Hughes, T.J.R., Cottrell, J.A., Bazilevs, Y.: Isogeometric analysis: CAD, finite elements, NURBS, exact geometry and mesh refinement. Comput. Methods Appl. Mech. Eng. 194(39), 4135–4195 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  9. Sanborn, G.G., Shabana, A.A.: A rational finite element method based on the absolute nodal coordinate formulation. Nonlinear Dyn. 58(3), 565–572 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  10. Sanborn, G.G., Shabana, A.A.: On the integration of computer aided design and analysis using the finite element absolute nodal coordinate formulation. Multibody Syst. Dyn. 22(2), 181–197 (2009)

    Article  MATH  Google Scholar 

  11. Shabana, A.A.: An absolute nodal coordinates formulation for the large rotation and deformation analysis of flexible bodies. Technical Report. No. MBS96-1-UIC, University of Illinois at Chicago, (1996)

  12. Schiehlen, W.: Research trends in multibody system dynamics. Multibody Syst. Dyn. 18, 3–13 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  13. Shabana, A.A.: Flexible multibody dynamics review of past and recent developments. Multibody Syst. Dyn. 1, 189–222 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  14. Dmitrochenko, O.N., Pogorelov, D.Y.: Generalization of plate finite elements for absolute nodal coordinate formulation. Multibody Syst. Dyn. 10(1), 17–43 (2003)

    Article  MATH  Google Scholar 

  15. Dufva, K., Shabana, A.A.: Analysis of thin plate structures using the absolute nodal coordinate formulation. Proc. Inst. Mech. Eng., Proc., Part K, J. Multi-Body Dyn. 219, 345–355 (2005)

    Google Scholar 

  16. Tian, Q., Chen, L., Zhang, Y.Q., Yang, J.: An efficient hybrid method for multibody dynamics simulation based on absolute nodal coordinate formulation. J. Comput. Nonlinear Dyn. 4, 021009 (2009)

    Article  Google Scholar 

  17. Liu, C., Tian, Q., Hu, H.Y.: Dynamics of a large scale rigid–flexible multibody system composed of composite laminated plate. Multibody Syst. Dyn. 26(3), 283–305 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  18. Yan, D., Liu, C., Tian, Q., Zhang, K., Liu, X.N., Hu, G.K.: A new curved gradient deficient shell element of absolute nodal coordinate formulation for modeling thin shell structures. Nonlinear Dyn. 74, 153–164 (2013)

    Article  MathSciNet  Google Scholar 

  19. Lan, P., Shabana, A.A.: Integration of B-spline geometry and ANCF finite element analysis. Nonlinear Dyn. 61, 193–206 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  20. Mikkola, A.M., Shabana, A.A., Sanchez-Rebollo, C., Jimenez-Octavio, J.R.: Comparison between ANCF and B-spline surfaces. Multibody Syst. Dyn. 30(2), 119–138 (2013)

    Article  MathSciNet  Google Scholar 

  21. Farin, G.: Triangular Bernstein–Bézier patches. Comput. Aided Geom. Des. 3(2), 83–127 (1986)

    Article  MathSciNet  Google Scholar 

  22. Yamashita, H., Sugiyama, H.: Numerical convergence of finite element solutions of nonrational B-spline element and absolute nodal coordinate formulation. Nonlinear Dyn. 67(1), 177–189 (2012)

    Article  MATH  MathSciNet  Google Scholar 

  23. Shabana, A.A., Hamed, A.M., Mohamed, A.N.A., Jayakumar, P., Letherwood, M.D.: Use of B-spline in the finite element analysis: comparison with ANCF geometry. J. Comput. Nonlinear Dyn. 7(1), 011008 (2012)

    Article  Google Scholar 

  24. Nada, A.A.: Use of B-spline surface to model large-deformation continuum plates: procedure and applications. Nonlinear Dyn. 72, 243–263 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  25. Bauchau, O.A., Han, S.l., Mikkola, A.M., Matikainen, M.K.: Comparison of the absolute nodal coordinate and geometrically exact formulations for beams. Multibody Syst. Dyn. 32(1), 67–85 (2013)

    Article  MathSciNet  Google Scholar 

  26. Mikkola, A.M., Shabana, A.A.: A non-incremental finite element procedure for the analysis of large deformation of plates and shells in mechanical system applications. Multibody Syst. Dyn. 9(3), 283–309 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  27. Matikainen, M.K., Valkeapää, A.I., Mikkola, A.M., Schwab, A.L.: A study of moderately thick quadrilateral plate elements based on the absolute nodal coordinate formulation. Multibody Syst. Dyn. (2013). doi:10.1007/s11044-013-9383-6

    Google Scholar 

  28. Liu, C., Tian, Q., Yan, D., Hu, H.Y.: Dynamic analysis of membrane systems undergoing overall motion large deformations and wrinkles via thin shell elements of ANCF. Comput. Methods Appl. Mech. Eng. 258, 81–95 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  29. Dmitrochenko, O., Mikkola, A.M.: Two simple triangular plate elements based on the absolute nodal coordinate formulation. J. Comput. Nonlinear Dyn. 3(4), 041012-1–041012-8 (2008)

    Article  Google Scholar 

  30. Specht, B.: Modified shape functions for the three–node plate bending element passing the patch test. Int. J. Numer. Methods Eng. 26(3), 705–715 (1988)

    Article  MATH  MathSciNet  Google Scholar 

  31. Morley, L.S.D.: The constant-moment plate-bending element. J. Strain Anal. 6(1), 20–24 (1971)

    Article  Google Scholar 

  32. Mohamed, A.A.: Three-dimensional fully parameterized triangular plate element based on the absolute nodal coordinate formulation. J. Comput. Nonlinear Dyn. 8(4), 041016-1–041016-7 (2013)

    Google Scholar 

  33. Ubach, P.A., Oñate, E.: New rotation-free finite element shell triangle accurately using geometrical data. Comput. Methods Appl. Mech. Eng. 199(5), 383–391 (2010)

    Article  MATH  Google Scholar 

  34. Liu, Y.: Triangular Bézier surfaces with approximate continuity. University of Waterloo, Ph.D. Thesis (2008)

  35. Shi, F.: Computer-Aided Geometric Design and Non-uniform Rational B-spline. Higher Education Press, Beijing (2001) (in Chinese)

    Google Scholar 

  36. Mann, S.: Continuity adjustments to triangular Bézier patches that retain polynomial precision. Research Report CS-2000-01 (2000)

  37. Liu, D., Hoschek, J.: GC1 continuity conditions between adjacent rectangular and triangular Bézier surface patches. Comput. Aided Des. 21(4), 194–200 (1989)

    Article  MATH  Google Scholar 

  38. Shabana, A.A., Mikkola, A.M.: Use of the finite element absolute nodal coordinate formulation in modeling slope discontinuity. J. Mech. Des. 125, 342–350 (2003)

    Article  Google Scholar 

  39. Shabana, A.A., Maqueda, L.G.: Slope discontinuities in the finite element absolute nodal coordinate formulation: gradient deficient elements. Multibody Syst. Dyn. 20(3), 239–249 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  40. Bathe, K.J.: Finite Element Procedures. Prentice-Hall, New Jersey (1996)

    Google Scholar 

  41. Belytschko, T., Liu, W.K., Moran, B., Elkhodary, K.I.: Nonlinear Finite Element for Continua and Structures. Wiley, New York (2013)

    Google Scholar 

  42. Gerstmayr, J., Shabana, A.A.: Efficient integration of the elastic forces and thin three-dimensional beam elements in the absolute nodal coordinate formulation. In: Proceedings of the Multibody Dynamics Eccomas Thematic Conference, Madrid, Spain, 21–24 June (2005)

    Google Scholar 

  43. Liu, C., Tian, Q., Hu, H.Y.: New spatial curved beam and cylindrical shell elements of gradient-deficient absolute nodal coordinate formulation. Nonlinear Dyn. 70(3), 1903–1918 (2012)

    Article  MathSciNet  Google Scholar 

  44. Hussein, B., Negrut, D., Shabana, A.A.: Implicit and explicit integration in the solution of the absolute nodal coordinate differential/algebraic equations. Nonlinear Dyn. 54, 283–296 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  45. Shabana, A.A., Hussein, B.: A two–loop sparse matrix numerical integration procedure for the solution of differential/algebraic equations: application to multibody systems. J. Sound Vib. 327, 557–563 (2009)

    Article  Google Scholar 

  46. Hussein, B., Shabana, A.A.: Sparse matrix implicit numerical integration of the stiff differential/algebraic equation: implementation. Nonlinear Dyn. 65, 369–382 (2011)

    Article  MathSciNet  Google Scholar 

  47. Chung, J., Hulbert, G.: A time integration algorithm for structural dynamics with improved numerical dissipation: the generalized-alpha method. J. Appl. Mech. 60, 371–375 (1993)

    Article  MATH  MathSciNet  Google Scholar 

  48. Arnold, M., Brüls, O.: Convergence of the generalized-alpha scheme for constrained mechanical systems. Multibody Syst. Dyn. 18, 185–202 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  49. Tian, Q., Zhang, Y., Chen, L., Yang, J.J.: Simulation of planar flexible multibody systems with clearance and lubricated revolute joints. Nonlinear Dyn. 60, 489–511 (2010)

    Article  MATH  Google Scholar 

  50. Tian, Q., Liu, C., Machado, M., Flores, P.: A new model for dry and lubricated cylindrical joints with clearance in spatial flexible multibody systems. Nonlinear Dyn. 64, 25–67 (2011)

    Article  MATH  Google Scholar 

  51. Liu, C., Tian, Q., Hu, H.Y.: Dynamics and control of a spatial rigid–flexible multibody system with multiple cylindrical clearance joints. Mech. Mach. Theory 52, 106–129 (2012)

    Article  Google Scholar 

  52. Hermanns, M.: Parallel programming in Fortran 95 using OpenMP (2002). http://www.openmp.org/presentations/miguel/F95_OpenMPv1_v2.pdf

  53. Kulikov, G.M., Plotnikova, S.V.: Non-linear exact geometry 12-node solid-shell element with three translational degrees of freedom per node. Int. J. Numer. Methods Eng. 88(13), 1363–1389 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  54. Arciniega, R.A., Reddy, J.N.: Tensor-based finite element formulation for geometrically nonlinear analysis of shell structures. Comput. Methods Appl. Mech. Eng. 196(4), 1048–1073 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  55. Tian, Q., Sun, Y.L., Liu, C., Hu, H.Y., Flores, P.: Elastohydrodynamic lubricated cylindrical joints for rigid-flexible multibody dynamics. Comput. Struct. 114, 106–120 (2013)

    Article  Google Scholar 

  56. Sze, K.Y., Liu, X.H., Lo, S.H.: Popular benchmark problems for geometric nonlinear analysis of shells. Finite Elem. Anal. Des. 40, 1551–1569 (2004)

    Article  Google Scholar 

Download references

Acknowledgements

This work was supported in part by National Natural Science Foundations of China under Grants 11290151, 11221202 and 11302025. The work was also supported in part by Excellent Young Scholar Research Fund from Beijing Institute of Technology, and supported in part by the Beijing Higher Education Young Elite Teacher Project under Grant YETP1201.

Author information

Authors and Affiliations

  1. MOE Key Laboratory of Dynamics and Control of Flight Vehicle, School of Aerospace Engineering, Beijing Institute of Technology, Beijing, 100081, China

    Hanjiang Chang, Cheng Liu, Qiang Tian & Haiyan Hu

  2. Department of Mechanical Engineering, Lappeenranta University of Technology, Lappeenranta, Finland

    Aki Mikkola

Authors
  1. Hanjiang Chang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Cheng Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Qiang Tian
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Haiyan Hu
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Aki Mikkola
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Cheng Liu.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chang, H., Liu, C., Tian, Q. et al. Three new triangular shell elements of ANCF represented by Bézier triangles. Multibody Syst Dyn 35, 321–351 (2015). https://doi.org/10.1007/s11044-015-9462-y

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11044-015-9462-y

Keywords

Search

Navigation