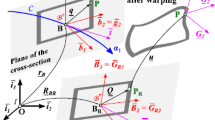
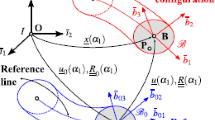
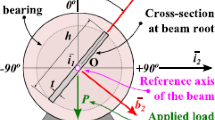
Abstract
The modeling of flexibility in multibody systems has received increase scrutiny in recent years. The use of finite element techniques is becoming more prevalent, although the formulation of structural elements must be modified to accommodate the large displacements and rotations that characterize multibody systems. Two formulations have emerged that have the potential of handling all the complexities found in these systems: the absolute nodal coordinate formulation and the geometrically exact formulation. Both approaches have been used to formulate naturally curved and twisted beams, plate, and shells. After a brief review of the two formulations, this paper presents a detailed comparison between these two approaches; a simple planar beam problem is examined using both kinematic and static solution procedures. In the kinematic solution, the exact nodal displacements are prescribed and the predicted displacement and strain fields inside the element are compared for the two methods. The accuracies of the predicted strain fields are found to differ: The predictions of the geometrically exact formulation are more accurate than those of the absolute nodal coordinate formulation. For the static solution, the principle of virtual work is used to determine the solution of the problem. For the geometrically exact formulation, the predictions of the static solution are more accurate than those obtained from the kinematic solution; in contrast, the same order of accuracy is obtained for the two solution procedures when using the absolute nodal coordinate formulation. It appears that the kinematic description of structural problems offered by the absolute nodal coordinate formulation leads to inherently lower accuracy predictions than those provided by the geometrically exact formulation. These observations provide a rational for explaining why the absolute nodal coordinate formulation computationally intensive.















Similar content being viewed by others
References
Eberhard, P., Schiehlen, W.: Computational dynamics of multibody systems: history, formalisms, and applications. J. Comput. Nonlinear Dyn. 1(1), 3–12 (2006)
Wasfy, T.M., Noor, A.K.: Computational strategies for flexible multibody systems. Appl. Mech. Rev. 56(2), 553–613 (2003)
Shabana, A.A., Wehage, R.A.: A coordinate reduction technique for dynamic analysis of spatial substructures with large angular rotations. J. Struct. Mech. 11(3), 401–431 (1983)
Agrawal, O.P., Shabana, A.A.: Application of deformable-body mean axis to flexible multibody system dynamics. Comput. Methods Appl. Mech. Eng. 56(2), 217–245 (1986)
Shabana, A.A.: Flexible multibody dynamics: review of past and recent developments. Multibody Syst. Dyn. 1(2), 189–222 (1997)
Simo, J.C.: A finite strain beam formulation. the three-dimensional dynamic problem. Part I. Comput. Methods Appl. Mech. Eng. 49(1), 55–70 (1985)
Shabana, A.A., Hussien, H.A., Escalona, J.L.: Application of the absolute nodal coordinate formulation to large rotation and large deformation problems. J. Mech. Des. 120, 188–195 (1998)
Gerstmayr, J., Sugiyama, H., Mikkola, A.: An overview on the developments of the absolute nodal coordinate formulation. In: Proceedings of the Second Joint International Conference on Multibody System Dynamics, Stuttgart, Germany, May 2012
Géradin, M., Cardona, A.: Flexible Multibody System: A Finite Element Approach. Wiley, New York (2001)
Hughes, T.J.R.: The Finite Element Method. Prentice Hall, Englewood Cliffs (1987)
Bathe, K.J.: Finite Element Procedures. Prentice Hall, Englewood Cliffs (1996)
Simo, J.C., Vu-Quoc, L.: A three dimensional finite strain rod model. Part II: Computational aspects. Comput. Methods Appl. Mech. Eng. 58(1), 79–116 (1986)
Borri, M., Merlini, T.: A large displacement formulation for anisotropic beam analysis. Meccanica 21, 30–37 (1986)
Danielson, D.A., Hodges, D.H.: Nonlinear beam kinematics by decomposition of the rotation tensor. J. Appl. Mech. 54(2), 258–262 (1987)
Danielson, D.A., Hodges, D.H.: A beam theory for large global rotation, moderate local rotation, and small strain. J. Appl. Mech. 55(1), 179–184 (1988)
Shabana, A.A.: Definition of the slopes and the finite element absolute nodal coordinate formulation. Multibody Syst. Dyn. 1, 339–348 (1997)
Romero, I.: A comparison of finite elements for nonlinear beams: the absolute nodal coordinate and geometrically exact formulations. Multibody Syst. Dyn. 20, 51–68 (2008)
Bauchau, O.A., Craig, J.I.: Structural Analysis with Application to Aerospace Structures. Springer, Dordrecht (2009)
Timoshenko, S.P.: On the correction factor for shear of the differential equation for transverse vibrations of bars of uniform cross-section. Philos. Mag. 41, 744–746 (1921)
Timoshenko, S.P.: On the transverse vibrations of bars of uniform cross-section. Philos. Mag. 43, 125–131 (1921)
Reissner, E.: The effect of transverse shear deformation on the bending of elastic plates. Z. Angew. Math. Phys. 12, A-69–A-77 (1945)
Mindlin, R.D.: Influence of rotatory inertia and shear on flexural motions of isotropic elastic plates. J. Appl. Mech. 18, 31–38 (1951)
Reissner, E.: On one-dimensional finite-strain beam theory: the plane problem. Z. Angew. Math. Phys. 23, 795–804 (1972)
Reissner, E.: On one-dimensional large-displacement finite-strain beam theory. Stud. Appl. Math. 52, 87–95 (1973)
Reissner, E.: On finite deformations of space-curved beams. Z. Angew. Math. Phys. 32, 734–744 (1981)
Malvern, L.E.: Introduction to the Mechanics of a Continuous Medium. Prentice Hall, Englewood Cliffs (1969)
Gerstmayr, J., Irschik, H.: On the correct representation of bending and axial deformation in the absolute nodal coordinate formulation with an elastic line approach. J. Sound Vib. 318(3), 461–487 (2008)
Shabana, A.A., Yakoub, R.Y.: Three dimensional absolute nodal coordinate formulation for beam elements: theory. J. Mech. Des. 123, 606–613 (2001)
Yakoub, R.Y., Shabana, A.A.: Three dimensional absolute nodal coordinate formulation for beam elements: implementation and applications. J. Mech. Des. 123, 614–621 (2001)
Bauchau, O.A.: Flexible Multibody Dynamics. Springer, Dordrecht (2011)
Crisfield, M.A., Jelenić, G.: Objectivity of strain measures in the geometrically exact three-dimensional beam theory and its finite-element implementation. Proc. R. Soc., Math. Phys. Eng. Sci. 455(1983), 1125–1147 (1999)
Bauchau, O.A., Han, S.L.: Interpolation of rotation and motion. Multibody Syst. Dyn. (2013). doi:10.1007/s11044-013-9365-8
Shabana, A.A., Mikkola, A.M.: Use of the finite element absolute nodal coordinate formulation in modeling slope discontinuity. J. Mech. Des. 125(2), 342–350 (2003)
Shabana, A.A., Maqueda, L.G.: Slope discontinuities in the finite element absolute nodal coordinate formulation: gradient deficient elements. Multibody Syst. Dyn. 20, 239–249 (2008)
Maqueda, L.G., Shabana, A.A.: Numerical investigation of the slope discontinuities in large deformation finite element formulations. Nonlinear Dyn. 58, 23–37 (2009)
Zienkiewicz, O.C., Taylor, R.L., Zhu, J.Z.: The Finite Element Method: Its Basis and Fundamentals, 6th edn. Elsevier, Butterworth-Heinemann, Amsterdam (2005)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Bauchau, O.A., Han, S., Mikkola, A. et al. Comparison of the absolute nodal coordinate and geometrically exact formulations for beams. Multibody Syst Dyn 32, 67–85 (2014). https://doi.org/10.1007/s11044-013-9374-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11044-013-9374-7