Skip to main content
SpringerLink
Log in

Dynamic simulation of crankshaft multibody systems

  • Published:
Multibody System Dynamics Aims and scope Submit manuscript

Abstract

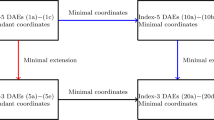
General multibody system approaches are often not sufficient for specific situations in applications to yield an efficient and accurate solution. We concentrate on the simulation of the crankshaft dynamics which is characterized by flexible bodies and force laws describing the interaction between the bodies. The use of the floating frame of reference approach in our model leads to an index-2 DAE system. The algebraic constraints originate from the reference conditions and the normalization equation for the quaternions. For the time integration of this system, two aspects have to be taken into account: firstly, for efficiency exploiting the structure of the system and using parallelization. Secondly, consistent initial values also with respect to a related index-3 system have to be computed in order to compute missing initial velocities and to reduce transient phenomena.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. ABAQUS: ABAQUS Theory Manual, Version 6.7. ABAQUS (2007)

  2. Barrett, R., Berry, M., Chan, T.F., Demmel, J., Donato, J., Dongarra, J., Eijkhout, V., Pozo, R., Romine, C., Van der Vorst, H.: Templates for the Solution of Linear Systems: Building Blocks for Iterative Methods, 2nd edn. SIAM, Philadelphia (1994)

    Google Scholar 

  3. Bottema, O., Roth, B.: Theoretical Kinematics. Dover, New York (1990)

    MATH  Google Scholar 

  4. Brenan, K.E., Campbell, S.L., Petzold, L.R.: Numerical Solution of Initial-Value Problems in Differential-Algebraic Equations. SIAM, Philadelphia (1996)

    MATH  Google Scholar 

  5. Bulirsch, R., Stoer, J.: Introduction to Numerical Analysis. Springer, New York (2002)

    MATH  Google Scholar 

  6. De Veubeke, B.F.: The dynamics of flexible bodies. Int. J. Eng. Sci. 14, 895–913 (1976)

    Article  MATH  Google Scholar 

  7. Drab, C.B.: Dynamic simulation of power train related systems. PhD Thesis. Johannes Kepler Universität Linz, Linz (2007)

    Google Scholar 

  8. Drab, C.B., Haslinger, J.R., Offner, G., Pfau, R.U.: Comparison of the classical formulation with the reference conditions formulation for dynamic flexible multibody systems. ASME J. Comput. Nonlinear Dyn. 2, 337–343 (2007)

    Article  Google Scholar 

  9. Eberhard, P.: Kontaktuntersuchungen durch hybride Mehrkörpersystem/Finite Element Simulationen. Shaker, Aachen (2000)

    Google Scholar 

  10. Eich-Soellner, E., Führer, C.: Numerical Methods in Multibody Dynamics. Teubner, Stuttgart (1998)

    MATH  Google Scholar 

  11. Eizenberger, T.: Dynamic simulation of flexible structures undergoing large gross motion. PhD Thesis. Johannes Kepler Universität Linz, Linz (2003)

    Google Scholar 

  12. AVL GmbH: AVL-EXCITE, Theory, Version 7.0.4. AVL, Graz (2007)

    Google Scholar 

  13. Führer, C., Wallrapp, O.: A computer-oriented method for reducing linearized multibody system equations by incorporating constraints. Comput. Methods Appl. Mech. Eng. 46, 169–175 (1984)

    Article  MATH  Google Scholar 

  14. Hairer, E., Nørsett, S.P., Wanner, G.: Solving Ordinary Differential Equations I: Nonstiff Problems. Springer, New York (2000)

    Google Scholar 

  15. Hairer, E., Wanner, G.: Solving Ordinary Differential Equations II: Stiff and Differential-Algebraic Problems. Springer, New York (2002)

    Google Scholar 

  16. Jackson, K.R., Sacks-Davis, R.: An alternative implementation of variable step-size multistep formulas for stiff ODEs. ACM Trans. Math. Softw. 3, 295–318 (1980)

    Article  MathSciNet  Google Scholar 

  17. Krasser, J.: Thermoelastohydrodynamische Analyse dynamisch belasteter Radialgleitlager. PhD Thesis, Technische Universität Graz, Graz (1996)

  18. Kuipers, J.B.: Quaternions and Rotation Sequences. Princeton University Press, Princeton (1999)

    MATH  Google Scholar 

  19. Lalor, N.: Experimental statistical energy analysis: A tool for the reduction of machinery noise. In: Proceedings of the E.J. Richards Memorial Session at the 131st ASA Meeting (1996)

  20. Lötstedt, P.: On the relation between singular perturbation problems and differential-algebraic equations. Uppsala University, Dept. of Computer Sciences, Report No. 100 (1985)

  21. Lubich, Ch.: Integration of stiff mechanical systems by Runge–Kutta methods. Z. Angew. Math. Phys. (ZAMP) 44, 1022–1053 (1993)

    Article  MATH  MathSciNet  Google Scholar 

  22. Nikravesh, P.E.: Initial condition correction in multibody dynamics. Multibody Syst. Dyn. 18, 107–115 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  23. Offner, G., Ma, M.-T., Karlsson, U., Wikstroem, A., Loibnegger, B., Priebsch, H.-H.: Quality and validation of cranktrain vibration predictions—Effect of hydrodynamic journal bearing models. MBD-MST, Loughborough (2004)

  24. Offner, G., Drab, C.B., Loibnegger, B.: Application oriented dynamic simulation of elastic multibody systems. In: Goicolea, J.M., Cuadrado, J., García Orden, J.C. (eds) Proceedings of the ECCOMAS Thematic Conference “Multibody Dynamics 2005”, Madrid, Spain, 21–24 June 2005

  25. Offner, G., Eizenberger, T., Priebsch, H.H.: Separation of reference motions and elastic deformations in an elastic multi-body system. Proc. IMechE, Part K, J. Multi-body Dyn. 220, 63–75 (2006)

    Google Scholar 

  26. Offner, G., Lechner, M., Mahmoud, K., Priebsch, H.H.: Surface contact analysis in axial thrust bearings based on different numerical interpolation approaches. Proc. IMechE, Part K, J. Multi-body Dyn. 221, 233–245 (2007)

    Google Scholar 

  27. Parikyan, T., Resch, T., Priebsch, H.H.: Structured model of crankshaft in the simulation of engine dynamics with AVL/EXCITE. In: ASME Fall Technical Conference, ICE-37-3 (2001)

  28. Priebsch, H.H., Krasser, J.: Simulation of the oil film behaviour in elastic engine bearings considering pressure and temperature dependent oil viscosity. In: Dowson, D., Childs, T.H.C., Taylor, C. (eds) Proceedings of the 23rd Leeds-Lyon Symposium on Tribology-Elastohydrodynamics (Leeds, UK, 1996), pp. 651–659. Elsevier, Amsterdam (1996)

    Google Scholar 

  29. Rasser, M.W., Resch, T., Priebsch, H.H.: Enhanced crankshaft stress calculation method. In: CIMAC Congress, Copenhagen, Denmark, May 18–22 (1998)

  30. Rheinboldt, W.C., Simeon, B.: Computing smooth solutions of DAEs for elastic multibody systems. Comput. Math. Appl. 37, 69–83 (1999)

    Article  MATH  MathSciNet  Google Scholar 

  31. Schiehlen, W.: Multibody system dynamics: Roots and perspectives. Multibody Syst. Dyn. 1, 149–188 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  32. Schiehlen, W.: Research trends in multibody system dynamics. Multibody Syst. Dyn. 18, 3–13 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  33. Shabana, A.A.: Flexible multibody dynamics: Review of the past and recent developments. Multibody Syst. Dyn. 1, 189–222 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  34. Shabana, A.A.: Vibration of Discrete and Continuous Systems. Springer, New York (1997)

    Google Scholar 

  35. Shabana, A.A.: Dynamics of Multibody Systems. Cambridge University Press, Cambridge (1998)

    MATH  Google Scholar 

  36. Sopouch, M., Hellinger, W., Priebsch, H.-H.: Prediction of vibro-acoustics for chains and synchronous belts of reciprocating engines. Proc. IMechE, Part K, J. Multi-body Dyn. 217, 225–240 (2003)

    Google Scholar 

  37. Wehage, R.A., Haug, E.J.: Generalized coordinate partitioning for dimension reduction in analysis of constrained dynamic systems. J. Mech. Des. 104, 247–255 (1982)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Industrial Mathematics Competence Center, Altenberger Str. 69, 4040, Linz, Austria

    C. B. Drab, J. R. Haslinger & R. U. Pfau

  2. Johann Radon Institute for Computational and Applied Mathematics, Austrian Academy of Sciences, Altenberger Str. 69, 4040, Linz, Austria

    H. W. Engl

  3. AVL List GmbH, Hans-List-Platz 1, 8020, Graz, Austria

    G. Offner

  4. Johannes Kepler Universität Linz, Altenberger Str. 69, 4040, Linz, Austria

    W. Zulehner

Authors
  1. C. B. Drab
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. H. W. Engl
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. J. R. Haslinger
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. G. Offner
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. R. U. Pfau
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. W. Zulehner
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to C. B. Drab.

Additional information

The work of C.B. Drab, J.R. Haslinger, and R.U. Pfau is supported by the “Bundesministerium für Wirtschaft und Arbeit” and by the government of Upper Austria within the framework “Industrielle Kompetenzzentren und Netzwerke”.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Drab, C.B., Engl, H.W., Haslinger, J.R. et al. Dynamic simulation of crankshaft multibody systems. Multibody Syst Dyn 22, 133–144 (2009). https://doi.org/10.1007/s11044-009-9152-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11044-009-9152-8

Keywords

Search

Navigation