Skip to main content
SpringerLink
Log in

Constitutive model for cyclic deformation of perfluoroelastomers

  • Published:
Mechanics of Time-Dependent Materials Aims and scope Submit manuscript

Abstract

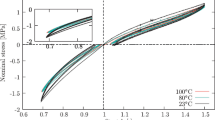
Observations are reported in uniaxial cyclic tensile tests with a strain-controlled program on perfluoroelastomer Hyflon MFA. A constitutive model is developed for its viscoplastic response and damage at three-dimensional deformations with finite strains. Adjustable parameters in the stress–strain relations are found by fitting the experimental data. Numerical simulation demonstrates that the constitutive equations adequately describe the mechanical response of perfluoroelastomer in cyclic tests with complicated deformation programs.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Ameduri, B., Boutevin, B., Kostov, G.: Fluoroelastomers: synthesis, properties and applications. Progr. Polym. Sci. 26, 105–187 (2001)

    Article  Google Scholar 

  • Bergstrom, J.S., Kurtz, S.M., Rimnac, C.M., Edidin, A.A.: Constitutive modeling of ultra-high molecular weight polyethylene under large-deformation and cyclic loading conditions. Biomaterials 23, 2329–2343 (2002)

    Article  Google Scholar 

  • Bueche, F.: Mullins effect and rubber–filler interaction. J. Appl. Polym. Sci. 5, 271–281 (1961)

    Article  Google Scholar 

  • Chagnon, G., Verron, E., Gornet, L., Marckmann, G., Charrier, P.: On the relevance of continuum damage mechanics as applied to the Mullins effect in elastomers. J. Mech. Phys. Solids 52, 1627–1650 (2004)

    Article  MATH  Google Scholar 

  • Chagnon, G., Verron, E., Marckmann, G., Gornet, L.: Development of new constitutive equations for the Mullins effect in rubber using the network alteration theory. Int. J. Solids Struct. 43, 6817–6831 (2006)

    Article  MATH  Google Scholar 

  • Chen, J., Asano, M., Maekawa, Y., Yoshida, M.: Suitability of some fluoropolymers used as base films for preparation of polymer electrolyte fuel cell membranes. J. Membr. Sci. 277, 249–257 (2006)

    Article  Google Scholar 

  • De Tommasi, D., Puglisi, G., Saccomandi, G.: A micromechanics-based model for the Mullins effect. J. Rheol. 50, 495–512 (2006)

    Article  Google Scholar 

  • Diani, J., Brieu, M., Vacherand, J.M.: A damage directional constitutive model for Mullins effect with permanent set and induced anisotropy. Eur. J. Mech. A 25, 483–496 (2006)

    Article  MATH  Google Scholar 

  • Drozdov, A.D., Christiansen, J.deC.: Cyclic viscoplasticity of thermoplastic elastomers. Acta Mech. 194, 47–65 (2007)

    Article  MATH  Google Scholar 

  • Drozdov, A.D., Dorfmann, A.: Stress–strain relations in finite viscoelastoplasticity of rigid-rod networks: Applications to the Mullins effect. Continuum Mech. Thermodyn. 13, 183–205 (2001)

    MATH  MathSciNet  Google Scholar 

  • Erman, B., Flory, P.J.: Theory of elasticity of polymer networks. II. The effect of geometric constraints on junctions. J. Chem. Phys. 68, 5363–5369 (1978)

    Article  Google Scholar 

  • Erman, B., Monnerie, L.: Theory of elasticity of amorphous networks: effects of constraints along chains. Macromolecules 22, 3342–3348 (1989)

    Article  Google Scholar 

  • Goktepe, S., Miehe, C.: A micro-macro approach to rubber-like materials. III. The micro-sphere model of anisotropic Mullins-type damage. J. Mech. Phys. Solids 53, 2259–2283 (2005)

    Article  MathSciNet  Google Scholar 

  • Govindjee, S., Simo, J.: Transition from micro-mechanics to computationally efficient phenomenology: Carbon black filled rubbers incorporating Mullins’ effect. J. Mech. Phys. Solids 40, 213–233 (1992)

    Article  MATH  Google Scholar 

  • Horgan, C.O., Ogden, R.W., Saccomandi, G.: A theory of stress softening of elastomers based on finite chain extensibility. Proc. R. Soc. A 460, 1737–1754 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  • Johnson, M.A., Beatty, M.F.: A constitutive equation for the Mullins effect in stress controlled uniaxial extension experiments. Continuum Mech. Thermodyn. 5, 301–318 (1993)

    Article  MathSciNet  Google Scholar 

  • Kaliske, M., Nasdala, L., Rothert, H.: On damage modelling for elastic and viscoelastic materials at large strain. Comp. Struct. 79, 2133–2141 (2001)

    Article  Google Scholar 

  • Kafka, V., Vokoun, D.: On backstresses, overstresses, and internal stresses represented on the mesoscale. Int. J. Plast. 21, 1461–1480 (2005)

    Article  MATH  Google Scholar 

  • Kaushiva, B.D., Wilkes, G.L., Comeaux, C., Socha, L.: Structure-property relationships of poly(tetra fluoroethylene)–poly(tetrafluoroethylene-co-vinylidene fluoride-co-hexafluoropropylene) blends. Polymer 42, 4619–4633 (2001)

    Article  Google Scholar 

  • Likozar, B., Sebenik, U., Krajnc, M.: Modeling of dynamic mechanical properties of vulcanized fluoroelastomer. Polym. Eng. Sci. 47, 2085–2094 (2007)

    Article  Google Scholar 

  • Lin, R.C., Schomburg, U.: A finite elastic-viscoelastic-elastoplastic material law with damage: Theoretical and numerical aspects. Comp. Meth. Appl. Mech. Engng. 192, 1591–1627 (2003)

    Article  MATH  Google Scholar 

  • Losi, G.U., Knauss, W.G.: Free volume theory and nonlinear thermoviscoelastictity. Polym. Eng. Sci. 32, 542–557 (1992)

    Article  Google Scholar 

  • Mullins, L.: Softening of rubber by deformation. Rubber Chem. Technol. 42, 339–362 (1969)

    Google Scholar 

  • Ogden, R.W., Roxburgh, D.G.: A pseudo-elastic model for the Mullins effect in filled rubber. Proc. R. Soc. A 455, 2861–2877 (1999)

    Article  MATH  MathSciNet  Google Scholar 

  • Popelar, C.F., Liechti, K.M.: A distortion-modified free volume theory for nonlinear viscoelastic behavior. Mech. Time-Depend. Mater. 7, 89–141 (2003)

    Article  Google Scholar 

  • Simo, J.C.: On a fully three-dimensional finite-strain viscoelastic damage model: Formulation and computational aspects. Comp. Meth. Appl. Mech. Eng. 60, 153–173 (1987)

    Article  MATH  MathSciNet  Google Scholar 

  • Souzy, R., Ameduri, B.: Functional fluoropolymers for fuel cell membranes. Progr. Polym. Sci. 30, 644–687 (2005)

    Article  Google Scholar 

  • Souzy, R., Ameduri, B., Boutevin, B., Gebel, G., Capron, P.: Functional fluoropolymers for fuel cell membranes. Solid State Ionics 176, 2839–2848 (2005)

    Article  Google Scholar 

  • Sullivan, R.W.: Development of a viscoelastic continuum damage model for cyclic loading. Mech. Time-Depend. Mater. 12, 329–342 (2008)

    Article  MathSciNet  Google Scholar 

  • Tan, J., Chao, Y.J., Van Zee, J.W., Li, X., Wang, X., Yang, M.: Assessment of mechanical properties of fluoroelastomer and EPDM in a simulated PEM fuel cell environment by microindentation test. Mater. Sci. Eng. A 496, 464–470 (2008)

    Article  Google Scholar 

  • Turri, S., Levi, M., Cristini, M., Sanguineti, A.: Dynamic and thermo-mechanical properties of some specialty fluoroelastomers for low T g seal materials. J. Polym. Res. 14, 141–145 (2007)

    Article  Google Scholar 

  • Turri, S., Valsecchi, R., Levi, M., Cristini, M., Sanguineti, A.: Microstructure to property relations in a family of millable polyurethane fluoroelastomers. Eur. Polym. J. 44, 2951–2961 (2008)

    Article  Google Scholar 

  • Wang, S., Legare, J.M.: Perfluoroelastomer and fluoroelastomer seals for semiconductor wafer processing equipment. J. Fluorine Chem. 122, 113–119 (2003)

    Article  Google Scholar 

  • Xia, Z., Shen, X., Ellyin, F.: An assessment of nonlinearly viscoelastic constitutive models for cyclic loading: The effect of a general loading/unloading rule. Mech. Time-Depend. Mater. 9, 281–300 (2005)

    Article  Google Scholar 

  • Yakimets, I., Lai, D., Guigon, M.: Model to predict the viscoelastic response of a semi-crystalline polymer under complex cyclic mechanical loading and unloading conditions. Mech. Time-Depend. Mater. 11, 47–60 (2007)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Danish Technological Institute, Gregersensvej 1, 2630, Taastrup, Denmark

    A. D. Drozdov

Authors
  1. A. D. Drozdov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to A. D. Drozdov.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Drozdov, A.D. Constitutive model for cyclic deformation of perfluoroelastomers. Mech Time-Depend Mater 13, 275–299 (2009). https://doi.org/10.1007/s11043-009-9085-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11043-009-9085-x

Keywords

Search

Navigation