Skip to main content
SpringerLink
Log in

The Initial-boundary Value Problem for the Ostrovsky-Vakhnenko Equation on the Half-line

  • Published:
Mathematical Physics, Analysis and Geometry Aims and scope Submit manuscript

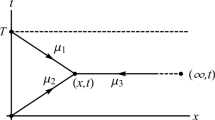
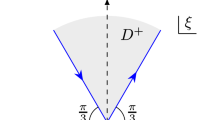
Abstract

We analyze an initial-boundary value problem for the Ostrovsky-Vakhnenko equation on the half-line. This equation can be viewed as the short wave model for the Degasperis-Procesi (DP) equation. We show that the solution u(x,t) can be recovered from its initial and boundary values via the solution of a vector Riemann-Hilbert problem formulated in the plane of a complex spectral parameter z.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Degasperis, A., Procesi, M.: Asymptotic integrability. In: Symmetry and Perturbation Theory (Rome 1998). World Scientific Publishers, New Jersey (1999)

  2. Kraenkel, R. A., Leblond, H., Manna, M. A.: An integrable evolution equation for surface waves in deep water. J. Phys. A Math. Theor. 47, 025208 (2014)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  3. Vakhnenko, V. O.: Solitons in a nonlinear model medium. J. Phys. A Math. Gen. 25, 4181–7 (1992)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  4. Parkes, E. J.: The stability of solutions of Vakhnenkos equation. J. Phys. A Math. Gen. 26, 6469–75 (1993)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  5. Vakhnenko, V. O.: The existence of loop-like solutions of a model evolution equation. Ukr. J. Phys. 42, 104–10 (1997)

    Google Scholar 

  6. Vakhnenko, V. O.: High-frequency soliton-like waves in a relaxing medium. J. Math. Phys. 40, 2011–20 (1999)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  7. Stepanyants, Y. A.: On stationary solutions of the reduced Ostrovsky equation: periodic waves, compactons and compound solitons. Chaos, Solitons Fractals 28, 193–204 (2006)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  8. Ostrovsky, L. A.: Nonlinear internal waves in a rotating ocean. Oceanology 18, 181–91 (1978)

    Google Scholar 

  9. Brunelli, J. C., Sakovich, S.: Hamiltonian structures for the Ostrovsky-Vakhnenko equation. Commun. Nonlinear Sci. Numer. Simul. 18, 56–62 (2013)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  10. Davidson, M.: Continuity properties of the solution map for the generalized reduced Ostrovsky equation. J. Differ. Equ. 252, 3797–815 (2013)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  11. Khusnutdinova, K. R., Moore, K. R.: Initial-value problem for coupled Boussinesq equations and a hierarchy of Ostrovsky equations. Wave Motion 48, 738–52 (2011)

    Article  MathSciNet  Google Scholar 

  12. Linares, F., Milan, A.: Local and global well-posedness for the Ostrovsky equation. J. Differ. Equ. 222, 325–40 (2006)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  13. Stefanov, A., Shen, Y., Kevrekidis, P. G.: 2010 Well-posedness and small data scattering for the generalized Ostrovsky equation. J. Differ. Equ. 249, 2600–17 (2010)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  14. Varlamov, V., Liu, Y.: Cauchy problem for the Ostrovsky equation, Discrete Contin. Dyn. Syst. 10, 731–53 (2004)

    MathSciNet  MATH  Google Scholar 

  15. Hone, A. N. W., Wang, J. P.: Prolongation algebras and Hamiltonian operators for peakon equations. Inverse Prob. 19, 129–45 (2003)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  16. Boutet de Monvel, A., Shepelsky, D.: The Ostrovsky-Vakhnenko equation by a Riemann-Hilbert approach. J. Phys. A: Math. Theor. 48, 035204 (2015)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  17. Fokas, A. S.: A unified transform method for solving linear and certain nonlinear PDEs. Proc. R. Soc. Lond. A 453, 1411–1443 (1997)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  18. Fokas, A. S.: Integrable nonlinear evolution equations on the half-line. Commun. Math. Phys. 230, 1–39 (2002)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  19. Fokas, A. S.: A unified approach to boundary value problems. In: CBMS-NSF Regional Conference Series in Applied Mathematics, SIAM (2008)

  20. Lenells, J.: Initial-boundary value problems for integrable evolution equations with 33 Lax pairs. Phys. D 241, 857–875 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  21. Lenells, J.: The Degasperis-Procesi equation on the half-line. Nonlinear Anal. 76, 122–139 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  22. Boutet de Monvel, A., Lenells, J., Shepelsky, D.: Long-time asymptotics for the Degasperis-Procesi equation on the half-line. arXiv:hep-th/1508:04097

  23. Xu, J., Fan, E.: The unified transform method for the Sasa-Satsuma equation on the half-line. Proc. R. Soc. A 469, 20130068 (2013)

    Article  ADS  MathSciNet  Google Scholar 

  24. Xu, J., Fan, E.: The three wave equation on the half-line. Mod. Phys. Lett. A 378, 26–33 (2014)

    Article  MathSciNet  Google Scholar 

  25. Xu, J.: Initial-boundary value problem for the two-component nonlinear schrödinger equation on the half-line. J. Non. Math. Phys. 23, 167–189 (2016)

    Article  ADS  Google Scholar 

  26. Xu, J., Fan, E.: Initial-boundary value problem for integrable nonlinear evolution equations with 33 Lax pairs on the interval. Stud. Appl. Math. 136, 321–354 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  27. Grimshaw, R., Pelinovsky, D.: Global existence of small-norm solutions in the reduced Ostrovsky equation. Discrete Contin. Dyn. Syst. 34, 557–66 (2014)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. College of Science, University of Shanghai for Science and Technology, Shanghai, 200093, People’s Republic of China

    Jian Xu

  2. School of Mathematical Sciences, Key Laboratory of Mathematics for Nonlinear Science, Fudan University, Shanghai, 200433, People’s Republic of China

    Engui Fan

Authors
  1. Jian Xu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Engui Fan
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jian Xu.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Xu, J., Fan, E. The Initial-boundary Value Problem for the Ostrovsky-Vakhnenko Equation on the Half-line. Math Phys Anal Geom 19, 20 (2016). https://doi.org/10.1007/s11040-016-9223-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11040-016-9223-z

Keywords

Mathematics Subject Classification (2010)

Search

Navigation