1 Introduction

In the last few decades many researcher explored different kinds of systems which self-organize and lead to pattern formation. Physical systems which display such phenomena include magnetization, Benard convective cells, cloud formations in stripes or rolls and many others. The challenge in all these instances is to find an appropriate mathematical model for the emergence of patterns in these self-organizing systems (Chandrasekhar 1946; Kiguchi et al. 1987; Lissauer 1993; Matsumoto and Hanawa 1999; Petigura et al. 2013; Spitzer 1968; Von Weizsacker 1944; Woolfson 2000).

One of the first conjecture about pattern formation under the action of gravity was formulated by Laplace (2010). He put forward the hypothesis that a primitive interstellar gas cloud evolve under gravity to form a system of isolated rings which may in turn lead to the formation of planetary systems. More recently Belrage (1968) and Prentice (1978) followed on Laplace hypothesis and explored possible mechanisms for the formation of ring structure in the solar nebula.

The issue of ring formation has become especially relevant and timely since the discovery of a remarkable pattern of bright and dark rings in the circumstellar disc surrounding the young solar analogue star HL Tau (ALMA Partnership 2015). It is also a relevant issue due to the large number of exoplanetary systems that have now been discovered. The orbital architecture of these systems is consistent with their condensation from dense rings of gas in proto-stellar disks, just as is believed to be the case for our solar system (Prentice 1978). The new astronomical data leads us to believe that there is a fundamental physical process at work, not yet fully understood, in which ring pattern formation is crucial to our understanding of the origin of planetary systems.

Many theories were put forward in the past about the origin of solar system. Currently the leading theory about the formation of planetary systems is the “Nebula Theory” whereby a cloud of interstellar gas accreted under it own gravitation, to form in stages, the protostar and the planets. Many of the results related to this theory were obtained through elaborate modeling and large scale numerical simulations. These involve, in general, thermodynamic considerations, magnetohydrodynamics modeling and turbulence (Ya Marov et al. 2013).

In a series of paper we addressed the issue of pattern formation under gravity from idealized analytical point of view (Humi 2006, 2009, 2016). In particular we showed that a self-gravitating incompressible rotating 2-dimensional gas admits steady states with ring structure in the matter distribution. It is our objective in this paper to examine this pattern formation problem under the assumption that the gas is isentropic. This is motivated by the desire to take into account (at least partially) the irreversible thermodynamic processes taking place in the disk which lead to the emission of radiation and heat. Under this idealized scenario the entropy produced within the disk due to the irreversible thermodynamic and turbulent processes taking place is removed by heat and radiation and the gas maintains a constant entropy. Obviously this assumption implies that this is a prototype “barebone” model that neglects many other important aspects of the processes taking place in an actual primitive cloud of interstellar gas.

The plan of the paper is as follows: In Sect. 2 we present the basic model equations. In Sect. 3 we consider the radial steady state of these equations and demonstrate that they admit solutions with patterns of density oscillations. In Sect. 4 we discuss radial time dependent states and establish again the existence of pattern forming solutions. We end up in Sect. 5 with some conclusions and observations.

2 Basic Equations

The basic equations that govern the time dependent state of non-relativistic self gravitating, compressible, inviscid two dimensional gas (in the \(x-y\) plane) are Prentice (1978), Humi (2006), Humi (2009) and Li et al. (2006)

$$\begin{aligned} \rho _t+(\rho u)_x + (\rho v)_y= & {} 0 \end{aligned}$$
(2.1)
$$\begin{aligned} (\rho u)_t + (\rho u^2 +p)_x + (\rho uv)_y= & {} -\rho \phi _x+\rho \omega ^2 x \end{aligned}$$
(2.2)
$$\begin{aligned} (\rho v)_t + (\rho uv)_x + (\rho v^2 +p)_y= & {} -\rho \phi _y+\rho \omega ^2 y \end{aligned}$$
(2.3)
$$\begin{aligned} \nabla ^2 \phi= & {} 4 \pi G \rho \end{aligned}$$
(2.4)

where subscripts indicate differentiation with respect to the indicated variable, \(\mathbf{u}=(u,v)\) is the gas velocity, \(\rho\) is its density, p is the pressure, \(\phi\) is the gravitational potential, G is the gravitational constant, t is time and \(\omega\) represents a solid body rotation of the gas.

In this set of Eq. (2.1) is the continuity equation, and (2.2–2.3) are the momentum equations. Finally (2.4) is the equation for the gravitational potential.

We can nondimensionalize these equations by introducing the following scalings

$$\begin{aligned}&t=\frac{L{\tilde{t}}}{U_0},\, x= L\tilde{x},\,\,\ y=L\tilde{y},\,\,\ u=U_0 \tilde{u} ,\,\,\ v=U_0 \tilde{v} ,\,\,\ \rho = \rho _0 \tilde{\rho },\nonumber \\&p=\rho _0 U_0^2\tilde{p},\,\,\ \phi = U_0^2 \tilde{\phi },\,\,\, G=\frac{U_0^2}{\rho _0 L^2}\tilde{G}, \,\,\,\omega =\frac{U_0}{L}\tilde{\omega }. \end{aligned}$$
(2.5)

where \(L,U_0,\rho _0\) are some characteristic length, velocity and mass density respectively that characterize the gas under consideration. Substituting these scalings in Eqs. (2.1–2.4) and dropping the tildes these equations remain unchanged (but the quantities that appear in these equations become non-dimensional).

3 Radial Steady States

In this section we consider radial steady states of the isentropic gas. In polar coordinates \((r,\theta )\) when all variables depend only on r. Eqs. (2.1–2.4) become;

$$\begin{aligned} u\frac{d\rho }{dr} + \rho \frac{du}{dr}+\frac{\rho u}{r}=0 \end{aligned}$$
(3.1)
$$\begin{aligned} \rho v\frac{du}{dr}+uv\frac{d\rho }{dr}+\frac{2\rho uv}{r}+\rho u\frac{dv}{dr}=0 \end{aligned}$$
(3.2)
$$\begin{aligned} 2\rho u\frac{du}{dr}+u^2\frac{d\rho }{dr}+\rho \frac{d\phi }{dr} +\frac{dp}{dr}+\frac{\rho (u^2-v^2)}{r}-\rho \omega ^2 r=0 \end{aligned}$$
(3.3)
$$\begin{aligned} \frac{d^2\phi }{dr^2}+\frac{1}{r}\frac{d\phi }{dr}-4\pi G\rho =0 \end{aligned}$$
(3.4)

Solving (3.2) algebraically for \(\frac{d\rho }{dr}\) and substituting in (3.1) it follows that

$$\begin{aligned} r\frac{dv}{dr}+v=0, \end{aligned}$$
(3.5)

and therefore

$$\begin{aligned} v=\frac{c_2}{r}. \end{aligned}$$
(3.6)

Similarly one can solve (3.1) for \(\rho u\) and obtain that

$$\begin{aligned} u=\frac{c_1}{\rho r}. \end{aligned}$$
(3.7)

(where \(c_1\), \(c_2\) are integration constants).

The classical relationship between pressure and density for isentropic gas is

$$\begin{aligned} p=A\rho ^{\alpha } \end{aligned}$$
(3.8)

where A is a constant which can be normalized to one and \(\alpha\) is the isentropy index. A more general functional relationship between the pressure and density will allow the isentropy coefficient A to be a function of r. That is

$$\begin{aligned} p=A(r)\rho ^{\alpha } \end{aligned}$$
(3.9)

This generalization is introduced to account for the possibility that the thermodynamic processes taking place within the gas are position dependent. An additional generalization can be obtained if we let \(\alpha\) to be a function of r. However in this paper we shall consider only the case where \(\alpha\) is a constant.

Substituting (3.6, 3.7 and 3.9) in (3.3) and assuming that \(\alpha\) is a constant we obtain (after differentiation to eliminate \(\phi\)) the following equation for \(\rho\)

$$\begin{aligned}&r^2(\alpha r^2A\rho ^{\alpha +2}-c_1^2\rho )\frac{d^2\rho }{dr^2}+ r^2[\alpha r^2\rho ^{\alpha +1}A(\alpha -2)+3c_1^2] \left( \frac{d\rho }{dr}\right) ^2 \\ \nonumber&\left\{ r^3\rho ^{\alpha +2}[r\frac{dA}{dr}(2\alpha -1)+ \alpha A]+3c_1^2r\rho \right\} \frac{d\rho }{dr}+ r^3\rho ^{\alpha +3}\frac{dA}{dr}+ \\ \nonumber&r^4\rho ^{\alpha +3}\frac{d^2A}{dr^2}+2\rho ^2 (2\pi Gr^4\rho ^3-r^4\omega ^2\rho ^2+c_2^2\rho ^2+c_1^2) =0 \end{aligned}$$
(3.10)

In the following we present analytic solutions of (3.10) with density pattern.

3.1 Steady State Analytic Solutions with Density Patterns

When \(\alpha =2\) and A is a constant (3.10) becomes

$$\begin{aligned}&r^2\rho (2Ar^2\rho ^3-c_1^2)\frac{d^2\rho }{dr^2}+3c_1^2r^2\left( \frac{d\rho }{dr}\right) ^2+ r\rho (2Ar^2\rho ^3+3c_1^2)\frac{d\rho }{dr}+ \nonumber \\&2\rho ^2(c_2^2\rho ^2+2\pi Gr^4\rho ^3-\omega ^2 r^4\rho ^2+c_1^2)=0 \end{aligned}$$
(3.11)

When \(c_1=c_2=0\) (i.e. \(u=v=0\)) this equation has an exact analytic solution,

$$\begin{aligned} \rho _0=C_1J_0(w r)+C_2Y_0(w r)+\frac{\omega ^2}{2\pi G} \end{aligned}$$
(3.12)

where \(w=\sqrt{\frac{2\pi G}{A}}\) and \(J_0\), \(Y_0\) are zero order Bessel functions of the first and second kind. Since \(Y_0\) is singular at the origin we let \(C_2=0\). Assuming that the disk has a “central body” of radius 1 and \(\rho (1)=0\) the solution becomes

$$\begin{aligned} \rho _0=\frac{\omega ^2}{2\pi G}\left[ 1-\frac{J_0(w r)}{J_0(w)}\right] \end{aligned}$$
(3.13)

For \(\omega =0.36\), \(A=0.02\) and \(G=0.05\) this solution is positive and have oscillatory pattern beyond the boundary of the central body viz. \(r >1\) (See Fig. 1).

Fig. 1
figure 1

Steady state density pattern

Full size image

Analytic solution of (3.11) in terms of hypergeometric functions can be obtained also when \(c_1=0\) and \(c_2 \ne 0\). To show the impact of this change on the solution we display the difference between this solution with \(c_2=0.02\) and the one above in Fig. 2 (Same values for A, G and \(\omega\) and same strategy to determine the integration constants). We see that the major difference between the two solutions happens in the region close to \(r=0\).

Fig. 2
figure 2

Difference in density pattern when \(c_2=0.02\)

Full size image

Another analytic oscillatory solution is obtained for \(A(r)=\beta r\), \(c_1=c_2=0\) where \(\beta\) is a constant. The general solution in this case can be written as

$$\begin{aligned} \rho =\frac{1}{\pi ^2 G^2 r} \left( C_1\sin \left( 2\sqrt{\frac{2\pi Gr}{\beta }}\right) +C_2\cos \left( 2\sqrt{\frac{2\pi Gr}{\beta }}\right) +\frac{\omega ^2(4\pi Gr-\beta )}{8}\right) \end{aligned}$$
(3.14)

To avoid the singularity in this expression at \(r=0\) we let \(C_1=0\) and \(C_2=\frac{\beta \omega ^2}{8}\). The solution is then

$$\begin{aligned} \rho =\frac{\omega ^2\left( \beta \cos \left( 2\sqrt{\frac{2\pi Gr}{\beta }}\right) + 4\pi Gr-\beta \right) }{8\pi ^2G^2r} \end{aligned}$$

For \(G=1\), \(\omega =1\), \(\beta =0.1\) this solution displays some density fluctuations whose amplitude decays as r increases.

One can obtain similar (but more complicated) analytic expressions for \(\rho\) when \(c_2 \ne 0\).

These solutions demonstrate that steady state density patterns do arise in isontropic gas under gravity.

For \(\alpha =1\) and \(\omega =c_1=c_2=0\) we obtain the following analytic solution for \(\rho\)

$$\begin{aligned} \rho =\frac{C_1 br^{-2+d}}{2\pi G(r^d+b)^2} \end{aligned}$$
(3.15)

where \(d=\sqrt{\frac{C_1}{A}}\) and \(b=C_2 d\) and \(C_1,\,C_2\) are integration constants.

4 Time Dependent Radial Solutions

When all the variables in (2.1–2.4) depend only on r and t we obtain the following system of equations:

$$\begin{aligned} \frac{\partial \rho }{\partial t}+ & {} u\frac{\partial \rho }{\partial r} + \rho \frac{\partial u}{\partial r}+\frac{\rho u}{r}=0 \end{aligned}$$
(4.1)
$$\begin{aligned} \rho v\frac{\partial u}{\partial r}+ & {} uv\frac{\partial \rho }{\partial r}+ \frac{2\rho uv}{r}+\rho u\frac{\partial v}{\partial r} + v\frac{\partial \rho }{\partial t}+\rho \frac{\partial v}{\partial t} =0 \end{aligned}$$
(4.2)
$$\begin{aligned} u\frac{\partial \rho }{\partial t}+ & {} 2\rho u\frac{\partial u}{\partial r}+ u^2\frac{\partial \rho }{\partial r}+\rho \frac{\partial \phi }{\partial r}+ \frac{\partial p}{\partial r}+\frac{\rho (u^2-v^2)}{r}-\rho \omega ^2 r +\rho \frac{\partial u}{\partial t}=0 \end{aligned}$$
(4.3)
$$\begin{aligned} \frac{\partial ^2\phi }{\partial r^2}+ & {} \frac{1}{r}\frac{\partial \phi }{\partial r} -4\pi G\rho =0 \end{aligned}$$
(4.4)

To simplify (somewhat) these equations we solve (algebraically) (4.2) for \(\frac{\partial \rho }{\partial t}\) and substitute in (4.1) to obtain

$$\begin{aligned} r\frac{\partial v}{\partial t}+ru\frac{\partial v}{\partial r}+uv=0. \end{aligned}$$
(4.5)

Similarly solving (4.3) algebraically for \(\frac{\partial \rho }{\partial t}\) and substituting in (4.1) yields,

$$\begin{aligned} r\rho \frac{\partial u}{\partial t}+\rho u\frac{\partial u}{\partial r} +r\frac{\partial p}{\partial r}+r\rho \frac{\partial \phi }{\partial r}- \rho \omega ^2 r^2-\rho v^2=0 \end{aligned}$$
(4.6)

4.1 Further Reductions for \(\boldsymbol{\alpha} \boldsymbol{=}{\bf 2}\)

Assuming that v(r, t) is independent of t it follows from (4.5) that

$$\begin{aligned} v(r)= \frac{c_1}{r} \end{aligned}$$
(4.7)

where \(c_1\) is a constant. Equation (4.4) can be used to express \(\phi\) in terms of \(\rho\).

$$\begin{aligned} \phi (r,t)=\int \frac{4\pi G\int \rho (r,t)r dr+f_1(t)}{r} dr \end{aligned}$$
(4.8)

where \(f_1(t)\) is some function of t.

Substituting (4.7) in (4.6) and using the fact that \(\alpha =2\) (viz. \(p=A\rho ^2\)) leads to

$$\begin{aligned} \frac{\partial u(r,t)}{\partial t}+ \frac{u(r,t)}{r}\frac{\partial u(r,t)}{\partial r}+ \frac{2A\partial (\rho (r,t)+\phi (r,t))}{\partial r}-r\omega ^2-c^2/r^3. \end{aligned}$$
(4.9)

Solving this equation for \(\rho (r,t)+\phi (r,t)\) yields,

$$\begin{aligned} 2A\rho (r,t)+\phi (r,t)=-\int \frac{r^3\frac{\partial u(r,t)}{\partial t}+ r^2u(r,t) \frac{\partial u(r,t)}{\partial r}-r^4\omega ^2- c^2}{r^3} dr+f_2(t) \end{aligned}$$
(4.10)

where \(f_2(t)\) is arbitrary. Using (4.8) to substitute for \(\phi (r,t)\), differentiating the result with respect to r, and then multiplying by r and differentiating again we obtain the following differential equation for \(\rho\)

$$\begin{aligned} 2Ar\frac{\partial ^2\rho (r,t)}{\partial r^2}+ 2A \frac{\partial \rho (r,t)}{\partial r}+ 4\pi Gr\rho (r, t)+H(r, t) =0 \end{aligned}$$
(4.11)

where

$$\begin{aligned} H(r,t)=u(r,t)\frac{\partial ^2 u(r,t)}{\partial r^2}+ r\frac{\partial ^2 u(r,t)}{\partial r\partial t}+ \frac{\partial u(r,t)}{\partial r}+ \left( \frac{\partial u(r,t)}{\partial r}\right) ^2-2r\omega ^2+ \frac{2c^2}{r^3}. \end{aligned}$$
(4.12)

The system (4.1), (4.11) for \(\rho\) and u can solved numerically. However it can be decoupled also by solving (4.11) for \(\rho\)

$$\begin{aligned} \rho (r,t)&= J_0(ar)f_3(t)+Y_0(ar)f_4(t)\nonumber \\ &\quad - \frac{\pi }{4A} \left( J_0(ar)\int Y_0(ar)H(r,t) dr +Y_0(ar)\int J_0(ar)H(r,t) dr\right) \end{aligned}$$
(4.13)

where \(a=\sqrt{\frac{2\pi G}{A}}\) and \(f_3,\,f_4\) are arbitrary. However since \(Y_0\) is singular at the origin we let \(f_4=0\). Substituting this result in (4.1) yields a (complicated) intgro-differential equation for u(r, t) . However the key point here is that \(\rho (r,t)\) in (4.13) is expressed in terms of Bessel functions whose values are modulated by H(r, t). Since Bessel functions are oscillatory it is plausible to assume that for proper functional values of u(r, t) this may lead to time dependent pattern formations in the density.

4.2 Time Dependent Perturbations to a Steady State

The system (4.1–4.4) admits a steady state \(\rho =\rho _0(r)\) and \(u=v=0\). We consider here a perturbation from this steady state with \(\alpha =2\) in the form

$$\begin{aligned} \rho (r,t)=\rho _0(r)+\epsilon \rho _1(r,t),\;\;\; u=\epsilon u_1(r,t),\;\; v=0. \end{aligned}$$
(4.14)

Since \(\alpha =2\) the relevant equations to satisfy are (4.1) and (4.11). Substituting the expressions in (4.14) in these equations we obtain to first order in \(\epsilon\) the following equations

$$\begin{aligned} \frac{\partial \rho _1}{\partial t}+u_1\frac{\partial \rho _0}{\partial r} + \rho _0\frac{\partial u_1}{\partial r}+\frac{\rho _0 u_1}{r}=0 \end{aligned}$$
(4.15)
$$\begin{aligned} 2Ar\frac{\partial ^2 \rho _1}{\partial r^2}+2A\frac{\partial \rho _1}{\partial r}+ r\frac{\partial ^2 u_1}{\partial r\partial t}+\frac{\partial u_1}{\partial t}+ 4\pi Gr\rho _1=0. \end{aligned}$$
(4.16)

Introducing the unsatz

$$\begin{aligned} \rho _1=g_1(r)e^{\nu t},\;\;\; u_1=g_2(r)e^{\nu t}. \end{aligned}$$
(4.17)

we find that the time dependence in (4.15), (4.16) factors out and we obtain the following two equations for \(g_1\) and \(g_2\)

$$\begin{aligned} r\frac{\partial \rho _0}{\partial r}g_2+\rho _0\frac{\partial g_2}{\partial r} +\nu r g_1+\rho _0 g_2=0 \end{aligned}$$
(4.18)
$$\begin{aligned} 2Ar\frac{\partial ^2 g_1}{\partial r^2}+2A\frac{\partial g_1}{\partial r}+ \nu r\frac{\partial g_2}{\partial r}+\nu g_2+4\pi Grg_1=0. \end{aligned}$$
(4.19)

It is possible to reduce the system (4.18), (4.19) to a single equation by solving (4.18) algebraically for \(g_1\) and substituting the result in (4.19). For the steady state \(\rho _0=\frac{1}{2}\frac{\omega ^2}{G\pi }\) this procedure leads to the equation for \(g_2\),

$$\begin{aligned} \frac{A\omega ^2 r}{\pi G\nu }\frac{d^3g_2}{dr^3}+ & {} \frac{A\omega ^2}{\pi G\nu }\frac{d^2g_2}{dr^2}- \left[ \nu r-\frac{2\omega ^2 r}{\nu }+\frac{A\omega ^2}{\pi G\nu r}\right] \frac{dg_2}{dr}\nonumber \\+ & {} \left( \frac{A\omega ^2}{\pi G\nu r^2}+\frac{2\omega ^2}{\nu } -\nu \right) g_2=0 \end{aligned}$$
(4.20)

The solutions for \(g_2\) and \(g_1\) (which have no singularity at \(r=0\)) are

$$\begin{aligned} g_2=C_1 J_1\left( \frac{\sqrt{\pi }\mu r}{\omega }\right) ,\;\;\; g_1=-C_1\frac{\omega \mu }{2G\nu \sqrt{\pi }} J_0\left( \frac{\sqrt{\pi }\mu r}{\omega }\right) , \end{aligned}$$
(4.21)

Where \(J_0\) and \(J_1\) are Bessel functions of the first kind and

$$\begin{aligned} \mu =\sqrt{\frac{G}{A}(2\omega ^2-\nu ^2)}. \end{aligned}$$

This solution demonstrates that perturbations from the steady state \(\rho =\frac{1}{2}\frac{\omega ^2}{G\pi }\) yield patterns of density oscillations within the cloud.

4.3 Explicit Solutions

To provide some explicit time dependent pattern forming solutions we consider the system (4.1), (4.5), (4.6) and (4.4) under the assumption that \(p(r,t)=A\rho (r,t)^{\alpha (r)}\), viz. we let the isentropy index to vary with r. To derive these solutions we use separation of variables on this system of equations. That is we look for solutions in the form

$$\begin{aligned}&u(r,t)=f_1(r)g_1(t),\,\,\,v(r,t)=f_2(r)g_2(t),\,\,\, \rho (r,t)=f_3(r)g_3(t)\nonumber \\&\phi (r,t)=f_4(r)g_4(t),\,\,\,p(r,t)=f_5(r)g_5(t) \end{aligned}$$
(4.22)

From (4.1) we have

$$\begin{aligned}&\frac{\frac{dg_3}{dt}}{g_1g_3}=\mu _1, \end{aligned}$$
(4.23)
$$\begin{aligned}&\frac{f_1\frac{df_3}{dr}}{f_3}+\frac{df_1}{dr}+\frac{f_1}{r}=-\mu _1. \end{aligned}$$
(4.24)

(Here and in the following \(\mu _i\) are separation of variables constants). Similarly (4.5) leads to

$$\begin{aligned}&\frac{\frac{dg_2}{dt}}{g_1g_2}=\mu _2, \end{aligned}$$
(4.25)
$$\begin{aligned}&\frac{f_1\frac{df_2}{dr}}{f_2}+\frac{f_1}{r}=-\mu _2. \end{aligned}$$
(4.26)

Equation (4.4) yields that \(g_3(t)=g_4(t)\) (up to a multiplicative constant which can be normalized to 1) and \(f_4(r),\,f_3(r)\) must satisfy

$$\begin{aligned} r\frac{d^2f_4}{dr^2}+\frac{df_4}{dr}-4\pi Grf_3=0 \end{aligned}$$
(4.27)

Finally substituting the ansatz (4.22) in (4.6) leads to

$$\begin{aligned} \frac{df_1}{dr}+\frac{\frac{df_5}{dr}g_5}{f_1f_3g_3g_1^2}+ \frac{\frac{df_4}{dr}g_3}{f_1g_1^2}-\frac{f_2^2g_2^2}{rf_1g_1^2}- \frac{r\omega ^2}{f_1g_1^2}+\frac{\frac{dg_1}{dt}}{g_1^2}=0 \end{aligned}$$
(4.28)

To perform separation of variables on this equation it is obvious that additional assumptions are needed. We explore here two of these possibilities.

  1. 1.

    Assume that \(g_5=g_3g_1^2\), \(g_3=g_1^2\), \(\omega =0\) and \(g_1=g_2\). (Here and in the following we ignore possible multiplicative constants which can be normalized to 1).

Using these assumptions we can perform separation of variables on (4.28) and obtain;

$$\begin{aligned}&\frac{\frac{dg_1}{dt}}{g_1^2}=\mu _3 \end{aligned}$$
(4.29)
$$\begin{aligned}&\frac{df_1}{dr}+\frac{\frac{df_5}{dr}}{f_1f_3}+ \frac{\frac{df_4}{dr}}{f_1}-\frac{f_2^2}{rf_1}=-\mu _3. \end{aligned}$$
(4.30)

To solve for \(g_1,\,g_2\) and \(g_3\) we use (4.23) and (4.25). Since we assumed that \(g_1=g_2\) we can solve (4.25) to obtain

$$\begin{aligned} g_2=\frac{1}{C_1-\mu _2 t} \end{aligned}$$
(4.31)

Substituting this result in (4.23) leads to

$$\begin{aligned} g_3=C_2(C_1-\mu _2 t)^{-\mu _1/\mu _2}. \end{aligned}$$
(4.32)

where \(C_1\), \(C_2\) are an integration constants. However since we assumed that \(g_3=g_1^2\) we must have \(C_2=1\) and \(\mu _1=2\mu _2\) These results will be consistent with (4.29) if we let \(\mu _2=\mu _3\).

This completes the determination of all the functions \(g_i(t)\,\,i=1,\ldots ,5\) (since \(g_4=g_3\) and \(g_5=g_3g_1^2\)).

Equations (4.24), (4.26), (4.28) and (4.30) form a redundant system of four equations for five functions \(f_i(r),\,i=1,\ldots ,5\). Therefore we can choose one of these functions as a “parameter function”. For example if we let

$$\begin{aligned} f_1=\frac{-1}{a-\cos \,kr},\,\,\, a > 1, \end{aligned}$$

then from (4.24) and (4.26) we have

$$\begin{aligned} f_2=\frac{C_4}{r}\exp \left( \frac{\mu _2(akr-\sin \,kr)}{k}\right) ,\,\,\, f_3=\frac{C_5(a-\cos \,kr)}{r}\exp \left( \frac{\mu _1(akr+\sin \,kr)}{k}\right) \end{aligned}$$

where \(C_4\), \(C_5\) are constants. The expressions for \(g_3\) and \(f_3\) imply that \(\rho\) is oscillatory in r but the amplitude of these oscillations decays in time.

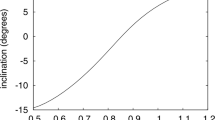
Substituting \(f_3\) in (4.27) we can solve for \(f_4\). Using all these results (4.29) becomes a first order differential equation for \(f_5\) which can be solved numerically. A plot of the isentropic index (at \(t=0\)) in this case with \(a=2\), \(k=5\pi\), \(C_4=C_5=1\), \(\mu _2=\mu _3=-1\) \(\mu _1=-2\) is shown in Fig. 3. In this figure the isentropic index has an approximate value of 2 at \(r=0\) but then rises rapidly to a constant value of 2.6.

Fig. 3
figure 3

Isentropic index for a time dependent solution

Full size image
  1. 2.

    Assume that \(g_5=g_3g_1^2\), \(g_3=g_1^2\), \(\omega =0\) and \(f_2^2=rf_1\).

Under present assumptions, separation of variables for (4.28) yields,

$$\begin{aligned}&-\frac{g_2^2}{g_1^2}+\frac{\frac{dg_1}{dt}}{g_1^2}=\mu _3, \end{aligned}$$
(4.33)
$$\begin{aligned}&\frac{df_1}{dr}+\frac{\frac{df_5}{dr}}{f_1f_3}+\frac{\frac{df_4}{dr}}{f_1}= -\mu _3. \end{aligned}$$
(4.34)

To determine the functions \(g_i(t)\) \(i=1...5\) in this case we use first (4.23) and (4.25) to obtain

$$\begin{aligned} \frac{\frac{dg_3}{dt}}{g_3}=\frac{\mu _1}{\mu _2}\frac{\frac{dg_2}{dt}}{g_2}. \end{aligned}$$
(4.35)

Hence

$$\begin{aligned} g_3 = C_1g_2^{\mu _1/\mu _2}. \end{aligned}$$
(4.36)

However since we assumed that \(g_3=g_1^2\) it follows that

$$\begin{aligned} g_1 = \sqrt{C_1g_2^{\mu _1/\mu _2}}. \end{aligned}$$
(4.37)

Substituting this result in (4.25) and solving for \(g_2\) yields

$$\begin{aligned} g_2 = 4^{\mu _2/\mu _1}(2C_2-\sqrt{C_1}\mu _1 t)^{-2\mu _2/\mu _1} \end{aligned}$$
(4.38)

where \(C_1,\, C_2\) are integration constants. Therefore

$$\begin{aligned} g_3=\frac{4C_1}{(2C_2-\sqrt{C_1}\mu _1 t)^2} \end{aligned}$$
(4.39)

These results will be consistent with (4.33) if we impose the constraints,

$$\begin{aligned} \mu _1=2\mu _2\;\;\; \mu _3=-\frac{1+C_1\mu _2}{C_1} \end{aligned}$$

To solve for the functions \(f_i(r)\), \(i=1...5\) we use the assumption \(f_2^2=rf_1\) in (4.26) and solve for \(f_1\). This yields

$$\begin{aligned} f_1=\frac{E_1-\mu _2 r^4}{2r^3} \end{aligned}$$

where \(E_1\) is a constant. However to avoid the singularity at the origin we let \(E_1=0\) and hence \(f_1=-\frac{\mu _2}{2}r\). Substituting this in (4.24) and solving for \(f_3\) we find that

$$\begin{aligned} f_3=E_2r^{2\frac{\mu _1-\mu _2}{\mu _2}} \end{aligned}$$

where \(E_2\) is a constant. However \(\mu _1=2\mu _2\), hence,

$$\begin{aligned} f_3=E_2r^2. \end{aligned}$$

From (4.27) we obtain then (after applying the boundary conditions at \(r=0\))

$$\begin{aligned} f_4=\frac{1}{4}E_2\pi Gr^4. \end{aligned}$$

Similarly (4.34) yields a polynomial expression for \(f_5\).

5 Conclusions

In this paper we explored the solutions of a simple two dimensional hydrodynamic model for a self gravitating and rotating isentropic gas cloud. Our main objective was to establish the existence of solutions with density oscillations (viz. pattern formation).

It might be argued that this paper does not present a general theory for pattern formation under gravity. However it should be pointed out that the existence and classification of periodic solutions for a system of nonlinear differential equations is an open problem (Wayne 1997). Nevertheless our results establish analytically the existence of such solutions for the steady and time dependent states of a rotating, isentropic self-gravitating gas in two dimensions.

For the radial steady state case we were able to reduce the original set of four partial differential equations to one differential equation for the density of the gas. The steady state solutions of this equation were explored with constant isentropic index and isentropic coefficient that is a function of r.

For the time dependent case we showed, perturbations from a steady state lead to oscillation in the the density. Furthermore using separation of variables, we showed explicitly that analytic oscillatory closed form solutions of the equations exist.