Skip to main content
SpringerLink
Log in

Blade cutting of thin walled structures by explicit dynamics finite elements

  • Novel Computational Approaches to Old and New Problems in Mechanics
  • Published:
Meccanica Aims and scope Submit manuscript

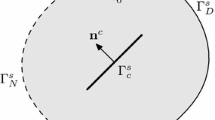
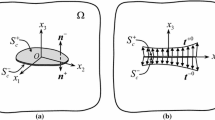
Abstract

In cohesive crack propagation induced by blade cutting, it is necessary to consider the blade radius of curvature as a characteristic length additional to the shell thickness and to the cohesive process zone length, which usually characterize crack propagation in thin walled structures. When the finite element simulation of a blade cutting process is considered, these three lengths need to be properly resolved. The blade radius of curvature can be orders of magnitude smaller than the shell thickness and the cohesive process zone. Furthermore, the transition from a continuous mesh to a mesh containing a crack with a cohesive interface is well known to be critical for solution accuracy. Nodal equilibrium is in general violated during the transition, with subsequent generation of spurious stress oscillations that, in view of the non-reversible nature of the problem, can lead to significant inaccuracies in the stress response. The smallest length, i.e. the blade radius of curvature, is here resolved using the so called directional cohesive element model as in Pagani and Perego (CMAME 285:515–541, 2015), while the structural thickness is modeled using solid-shell elements. The concept of directional cohesive elements is here extended for application to the case of cutting by scissors. As for the cohesive process zone length, different modeling options are discussed in terms of their capability to reduce the spurious oscillations and to provide an accurate estimate of the cutting parameters. Numerical tests are presented to validate the proposed modeling strategies.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22

Similar content being viewed by others

References

  1. Alfano G (2006) On the influence of the shape of the interface law on the application of cohesive-zone models. Compos Sci Technol 66(6):723–730. doi:10.1016/j.compscitech.2004.12.024

    Article  Google Scholar 

  2. Alfano G, Crisfield MA (2001) Finite element interface models for the delamination analysis of laminate composites: mechanical and computational issues. Int J Numer Methods Eng 50:1701–1736. doi:10.1002/nme.93

    Article  MATH  Google Scholar 

  3. Ambati M, De Lorenzis L (2016) Phase-field modeling of brittle and ductile fracture in shells with isogeometric NURBS-based solid-shell elements. Comput Methods Appl Mech Eng. doi:10.1016/j.cma.2016.02.017

    MathSciNet  Google Scholar 

  4. Amiri F, Millán D, Shen Y, Rabczuk T, Arroyo M (2014) Phase-field modeling of fracture in linear thin shells. Theor Appl Fract Mech 69:102–109. doi:10.1016/j.tafmec.2013.12.002

    Article  Google Scholar 

  5. Areias PMA, Belytschko T (2005) Non-linear analysis of shells with arbitrary evolving cracks using XFEM. Int J Numer Methods Eng 62(3):384–415. doi:10.1002/nme.1192

    Article  MATH  Google Scholar 

  6. Atkins AG, Mai YW (1979) On the guillotining of materials. J Mater Sci 14(11):2747–2754. doi:10.1007/bf00610649

    Article  ADS  Google Scholar 

  7. Carpinteri A, Colombo G (1989) Numerical analysis of catastrophic softening behaviour (snap-back instability). Comput Struct 31:607–636. doi:10.1016/0045-7949(89)90337-4

    Article  Google Scholar 

  8. Cirak F, Ortiz M, Pandolfi A (2005) A cohesive approach to thin-shell fracture and fragmentation. Comput Methods Appl Mech Eng 194:2604–2618. doi:10.1016/j.cma.2004.07.048

    Article  ADS  MATH  Google Scholar 

  9. Do B, Liu W, Yang Q, Su X (2013) Improved cohesive stress integration schemes for cohesive zone elements. Eng Fract Mech 107:14–28. doi:10.1016/j.engfracmech.2013.04.009

    Article  Google Scholar 

  10. Dolbow J, Moës N, Belytschko T (2000) Modeling fracture in Mindlin-Reissner plates with the extended finite element method. Int J Solids Struct 37(48–50):7161–7183. doi:10.1016/s0020-7683(00)00194-3

    Article  MATH  Google Scholar 

  11. Frangi A, Pagani M, Perego U, Borsari R (2010) Directional cohesive elements for the simulation of blade cutting of thin shells. Comput Model Eng Sci 57:205–224. doi:10.3970/cmes.2010.057.205

    MATH  Google Scholar 

  12. Ghisi A, Confalonieri F, Perego U (2015) Blade cutting simulation with crack propagation through thin-walled structures via solid-shell finite elements in explicit dynamics. In: Idelsohn S, Sonzogni V, Coutinho A, Cruchaga M, Lew A, Cerrolaza M (eds) PANACM 2015 - 1st Pan-American congress on computational mechanics, in conjunction with the 11th Argentine Congress on Computational Mechanics, MECOM 2015, pp 304–313

  13. Gilormini P, Diani J (2015) Testing some implementations of a cohesive-zone model at finite strain. Eng Fract Mech 148:97–109. doi:10.1016/j.engfracmech.2015.09.013

    Article  Google Scholar 

  14. Harper PW, Hallett SR (2008) Cohesive zone length in numerical simulations of composite delamination. Eng Fract Mech 75(16):4774–4792. doi:10.1016/j.engfracmech.2008.06.004

    Article  Google Scholar 

  15. Hu N, Zemba Y, Okabe T, Yan C, Fukunaga H, Elmarakbi AM (2008) A new cohesive model for simulating delamination propagation in composite laminates under transverse loads. Mech Mater 40(11):920–935. doi:10.1016/j.mechmat.2008.05.003

    Article  Google Scholar 

  16. Lu G, Calladine C (1990) On the cutting of a plate by a wedge. Int J Mech Sci 32(4):293–313. doi:10.1016/0020-7403(90)90095-Z

    Article  Google Scholar 

  17. Mahvash M, Voo L, Kim D, Jeung K, Wainer J, Okamura AM (2008) Modeling the forces of cutting with scissors. IEEE Trans Biomed Eng 55(3):848–856. doi:10.1109/TBME.2007.908069

    Article  Google Scholar 

  18. Menouillard T, Belytschko T (2009) Correction force for releasing crack tip element with xfem and only discontinuous enrichment. Eur J Comput Mech 18(5–6):465–483. doi:10.3166/ejcm.18.465-483

    Article  MATH  Google Scholar 

  19. Menouillard T, Belytschko T (2010) Smoothed nodal forces for improved dynamic crack propagation modeling in XFEM. Int J Numer Methods Eng 84:47–72. doi:10.1002/nme.2882

    Article  MathSciNet  MATH  Google Scholar 

  20. Mostofizadeh S, Fagerström M, Larsson R (2013) Dynamic crack propagation in elastoplastic thin-walled structures: modelling and validation. Int J Numer Methods Eng 96(2):63–86. doi:10.1002/nme.4524

    Article  MathSciNet  MATH  Google Scholar 

  21. Needleman A (2014) Some issues in cohesive surface modeling. Proc IUTAM 10:221–246. doi:10.1016/j.piutam.2014.01.020

    Article  Google Scholar 

  22. Pagani M, Perego U (2015) Explicit dynamics simulation of blade cutting of thin elastoplastic shells using “directional” cohesive elements in solid-shell finite element models. Comput Methods Appl Mech Eng 285:515–541. doi:10.1016/j.cma.2014.11.027

    Article  ADS  MathSciNet  Google Scholar 

  23. Pandolfi A, Ortiz M (2012) An eigenerosion approach to brittle fracture. Int J Numer Methods Eng 92(8):694–714. doi:10.1002/nme.4352

    Article  MathSciNet  MATH  Google Scholar 

  24. Planas J, Elices M (1991) Nonlinear fracture of cohesive materials. In: Bažant ZP (ed) Current trends in concrete fracture research. Springer, Netherlands, pp 139–157. doi:10.1007/978-94-011-3638-9_10

    Chapter  Google Scholar 

  25. Qiao H, Chen WQ, Yang Q, Lua J (2011) Augmented cohesive elements for efficient delamination analyses of composite laminates. J Eng Mater Technol 133(4):041010. doi:10.1115/1.4004694

    Article  Google Scholar 

  26. Schellekens JCJ, De Borst R (1993) On the numerical integration of interface elements. Int J Numer Methods Eng 36:43–66. doi:10.1002/nme.1620360104

    Article  MATH  Google Scholar 

  27. Schwarze M, Reese S (2011) A reduced integration solid-shell finite element based on the eas and the ans concept: large deformation problems. Int J Numer Methods Eng 85(3):289–329. doi:10.1002/nme.2966

    Article  MathSciNet  MATH  Google Scholar 

  28. Shet C, Chandra N (2004) Effect of the shape of t cohesive zone curves on the fracture response. Mech Adv Mater Struct 11(3):249–275. doi:10.1080/15376490490427207

    Article  Google Scholar 

  29. Simonsen BC, Wierzbicki T (1998) Plasticity, fracture and friction in steady state plate cutting. Int J Impact Eng 19(8):667–691. doi:10.1016/S0734-743X(98)90004-5

    Article  Google Scholar 

  30. Smith E (1999) The effect of the stress-relative displacement law on failure predictions using the cohesive zone model. Int J Fract 99(1–2):41–51. doi:10.1023/a:1018320714262

    Google Scholar 

  31. Soto A, González EV, Maimí P, Turon A, Sainz de Aja JR, de la Escalera FM (2016) Cohesive zone length of orthotropic materials undergoing delamination. Eng Fract Mech 159:174–188. doi:10.1016/j.engfracmech.2016.03.033

    Article  Google Scholar 

  32. Turon A, Camanho PP, Costa J, Renart J (2010) Accurate simulation of delamination growth under mixed-mode loading using cohesive elements: definition of interlaminar strengths and elastic stiffness. Compos Struct 92(8):1857–1864. doi:10.1016/j.compstruct.2010.01.012

    Article  Google Scholar 

  33. Turon A, Costa J, Camanho P, Maimí P (2008) Analytical and numerical investigation of the length of the cohesive zone in delaminated composite materials. In: Mechanical response of composites. Computational methods in applied sciences, vol. 10, pp. 77–97. Springer, Netherlands. doi:10.1007/978-1-4020-8584-0_4

  34. Turon A, Dávila CG, Camanho PP, Costa J (2007) An engineering solution for mesh size effects in the simulation of delamination using cohesive zone models. Eng Fract Mech 74(10):1665–1682. doi:10.1016/j.engfracmech.2006.08.025

    Article  Google Scholar 

  35. Tvergaard V, Hutchinson JW (1992) The relation between crack growth resistance and fracture process parameters in elastic–plastic solids. J Mech Phys Solids 40(6):1377–1397. doi:10.1016/0022-5096(92)90020-3

    Article  ADS  MATH  Google Scholar 

  36. Ulmer H, Hofacker M, Miehe C (2012) Phase field modeling of fracture in plates and shells. PAMM Proc Appl Math Mech 12(1):171–172. doi:10.1002/pamm.201210076

    Article  Google Scholar 

  37. Volokh KY (2004) Comparison between cohesive zone models. Commun Numer Methods Eng 20(11):845–856. doi:10.1002/cnm.717

    Article  MATH  Google Scholar 

  38. Yang Q, Cox B (2005) Cohesive models for damage evolution in laminated composites. Int J Fract 133(2):107–137. doi:10.1007/s10704-005-4729-6

    Article  MATH  Google Scholar 

  39. Yang QD, Fang XJ, Shi JX, Lua J (2010) An improved cohesive element for shell delamination analyses. Int J Numer Methods Eng 83(5):611–641. doi:10.1002/nme.2848

    MATH  Google Scholar 

Download references

Acknowledgements

The financial support by Tetra Pak Packaging Solutions is kindly acknowledged. Moreover, we acknowledge the CINECA and the Regione Lombardia award under the LISA initiative 2016–2018, for the availability of high performance computing resources and support.

Funding

This study was partly funded by Tetra Pak Packaging Solutions Spa (Order n. 800120646, 2016, Company code 0102) and partly by the CINECA and the Regione Lombardia award under the LISA initiative 2016-2018.

Author information

Authors and Affiliations

  1. Department of Civil and Environmental Engineering, Politecnico di Milano, piazza L. da Vinci, 32, Milano, 20133, Italy

    Federica Confalonieri, Aldo Ghisi & Umberto Perego

Authors
  1. Federica Confalonieri
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Aldo Ghisi
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Umberto Perego
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Umberto Perego.

Ethics declarations

Conflict of interest

Author Umberto Perego has received a research grant from Company Tetra Pak Packaging Solutions Spa. The remaining authors declare that they have no conflict of interest.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Confalonieri, F., Ghisi, A. & Perego, U. Blade cutting of thin walled structures by explicit dynamics finite elements. Meccanica 53, 1271–1289 (2018). https://doi.org/10.1007/s11012-017-0779-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11012-017-0779-x

Keywords

Search

Navigation