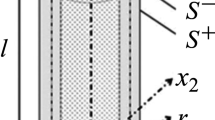
The dynamic problem of an elastic two-layer hollow cylinder whose surfaces are loaded by arbitrary forces is solved by the method based on the use of finite differences solely with respect to time. The numerical calculations of the stress concentration on the inner and outer surfaces and the surface of conjugation as functions of times are carried out for cylinders with different thicknesses of the layers under impact loads. The dependences of stresses on the radial variable r and time parameter τ are illustrated for two-layer cylinders subjected to impacts on the inner and outer surfaces.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
X. C. Yin and Z. Q. Yue, “Transient plane-strain response of multilayered elastic cylinders to axisymmetric impulse,” J. Appl. Mech., 69, No. 6, 825–835 (2002).
G. Cinelli, “Dynamic vibrations and stresses in elastic cylinders and spheres,” J. Appl. Mech., 33, 825–830 (1966).
Y. N. Gong and X. Wang, “Radial vibrations and dynamic stress in elastic hollow cylinders,” in: Structural Dynamics: Recent Advances, Elsevier, London (1991), pp. 137–147.
X. Wang and Y. N. Gong, “A theoretical solution for axially symmetric problem in elastodynamics,” Acta Mech. Sin., 7, 275–282 (1992).
X. C. Yin, “Multiple impacts of two concentric hollow cylinders with zero clearance,” Int. J. Solids Struct., 34, 4597–4616 (1997).
X. C. Yin and L. G. Wang, “The effect of multiple impacts on the dynamics of an impact system,” J. Sound Vibr., 228, 995–1015 (1999).
M. P. Savruk, “New method for the solution of dynamic problems of the theory of elasticity and fracture mechanics,” Fiz.-Khim. Mekh. Mater., 39, No. 4, 7–12 (2003).
M. P. Savruk, L. I. Onyshko, and M. M. Senyuk, “A plane dynamic axisymmetric problem for a hollow cylinder,” Fiz.-Khim. Mekh. Mater., 44, No. 1, 7–14 (2008).
A. Ya. Sagomonyan, Stress Waves in Continuous Media [in Russian], Moscow University, Moscow (1985).
Author information
Authors and Affiliations
Corresponding author
Additional information
Translated from Fizyko-Khimichna Mekhanika Materialiv, Vol. 45, No. 1, pp. 55–61, January–February, 2009.
Rights and permissions
About this article
Cite this article
Onyshko, L.I., Senyuk, M.M. Stressed state of a hollow two-layer cylinder under dynamic loads. Mater Sci 45, 57–65 (2009). https://doi.org/10.1007/s11003-009-9155-x
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11003-009-9155-x