Dynamic analysis of a functionally graded thick truncated cone with finite length

Abstract

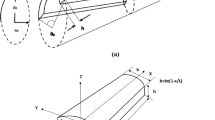
In this article dynamic analysis of a functionally graded thick truncated cone with finite length under axisymmetric internal impact loading is studied. The cone is made of a combined ceramic–metal material and the material composition varying continuously along its thickness according to the power law distribution. Finite Element Method based on Rayleigh–Ritz energy formulation and Newmark direct integration methods are applied to find the responses in time and space domain. The time histories of displacements, stresses, wave propagation in two directions and natural frequencies for various values of volume fraction exponent and semi-vertex angle of the cone for a cone with clamped end conditions have been studied. The results obtained in the present paper are compared with the result of an infinite FG thick hollow cylinder.

Appendix 1

For the simplex linear triangular element the following formulation is used.

$$ N_{i} = {\frac{{a_{i} + b_{i} r + c_{i} z}}{2A}} $$

(26)

$$ N_{j} = {\frac{{a_{j} + b_{j} r + c_{j} z}}{2A}} $$

(27)

$$ N_{k} = {\frac{{a_{k} + b_{k} r + c_{k} z}}{2A}} $$

(28)

$$ a_{i} = r_{j} z_{k} - r_{k} z_{j} $$

(29)

$$ b_{i} = z_{j} - z_{k} $$

(30)

$$ c_{i} = r_{k} - r_{j} $$

(31)

$$ a_{j} = r_{k} z_{i} - r_{i} z_{k} $$

(32)

$$ b_{j} = z_{k} - z_{i} $$

(33)

$$ c_{j} = r_{i} - r_{k} $$

(34)

$$ a_{k} = r_{i} z_{j} - r_{j} z_{i} $$

(35)

$$ b_{k} = z_{i} - z_{j} $$

(36)

$$ c_{k} = r_{j} - r_{i} $$

(37)

$$A=\frac{1}{2}\det \left[ \begin{array}{lll} 1 & 1 & 1\\ {r_{i} }& {r_{j} } & {r_{k}} \\ {z_{i} } & {z_{j}} & {z_{k}} \end{array}\right] $$

(38)