Abstract
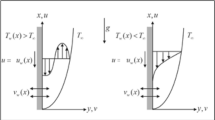
Sequel to the fact that hybrid nanofluidic systems (e.g. scalable micro-/nanofluidic device) exhibit greater thermal resistance with increasing nanoparticle concentration, little is known on the significance of thermal radiation, surface roughness and linear stability of water conveying alumina and copper oxide nanoparticles. This study presents the effects of thermal radiation and surface roughness on the complex dynamics of water conveying alumina and copper oxide nanoparticles, in the case where the thermophysical properties of the resulting mixture vary meaningfully with the volume fraction of solid nanomaterials, as well as with the Brownian motion and thermophoresis microscopic phenomena. Based on the linear stability theory and normal mode analysis method, the basic partial differential equations governing the transport phenomenon were non-dimensionalized to obtain the simplified stability equations. The optimum values of the critical thermal Rayleigh number depicting the onset of thermo-magneto-hydrodynamic instabilities were obtained using the power series method and the Chock–Schechter numerical integration. The increase in the strength of Lorentz forces, thermal radiation and surface roughness has a stronger stabilizing impact on the appearance of convection cells. On the contrary, the stability diminishes with the increasing values of the volumetric fraction and diameter of nanomaterials. The partial substitution of the alumina nanoparticles by the copper oxide nanomaterials in the mixture stabilizes importantly the hybrid nanofluidic medium.












Similar content being viewed by others
References
Zhao J-J, Duan Y-Y, Wang X-D, Wang B-X. Effects of superheat and temperature-dependent thermophysical properties on evaporating thin liquid films in microchannels. Int J Heat Mass Transf. 2011;54:1259–67.
Esfe MH, Afrand M. An updated review on the nanofluids characteristics. J Therm Anal Calorim. 2019;138:4091–101.
Żyła G. Viscosity and thermal conductivity of MgO–EG nanofluids. J Therm Anal Calorim. 2017;129:171–80.
Moldoveanu GM, Minea AA, Huminic G, Huminic A. Al2O3/TiO2 hybrid nanofluids thermal conductivity. J Therm Anal Calorim. 2019;137:583–92.
Animasaun IL, Koriko OK, Adegbie KS, Babatunde HA, Ibraheem RO, Sandeep N, et al. Comparative analysis between 36 and 47 nm alumina–water nanofluid flows in the presence of Hall effect. J Therm Anal Calorim. 2019;135:873–86.
Mahanthesh B, Lorenzini G, Oudina FM, Animasaun IL. Significance of exponential space- and thermal-dependent heat source effects on nanofluid flow due to radially elongated disk with Coriolis and Lorentz forces. J Therm Anal Calorim. 2019. https://doi.org/10.1007/s10973-019-08985-0.
Kumar KA, Sandeep N, Sugunamma V, Animasaun IL. Effect of irregular heat source/sink on the radiative thin film flow of MHD hybrid ferrofluid. J Therm Anal Calorim. 2020;139:2145–53.
Khodadadi H, Aghakhani S, Majd H, Kalbasi R, Wongwises S, Afrand M. A comprehensive review on rheological behavior of mono and hybrid nanofluids: effective parameters and predictive correlations. Int J Heat Mass Transf. 2018;127:997–1012.
Huminic G, Huminic A. Hybrid nanofluids for heat transfer applications—a state-of-the-art review. Int J Heat Mass Transf. 2018;125:82–103.
Babar H, Ali HM. Towards hybrid nanofluids: Preparation, thermophysical properties, applications, and challenges. J Mol Liq. 2019;281:598–633.
Das PK. A review based on the effect and mechanism of thermal conductivity of normal nanofluids and hybrid nanofluids. J Mol Liq. 2017;240:420–46.
Esfe MH, Esfandeh S, Saedodin S, Rostamian H. Experimental evaluation, sensitivity analyzation and ANN modeling of thermal conductivity of ZnO–MWCNT/EG–water hybrid nanofluid for engineering applications. Appl Therm Eng. 2017;125:673–85.
Sajid MU, Ali HM. Thermal conductivity of hybrid nanofluids: a critical review. Int J Heat Mass Transf. 2018;126:211–34.
Hussien AA, Abdullah MZ, Yusop NM, Al-Nimr MA, Atieh MA, Mehrali M. Experiment on forced convective heat transfer enhancement using MWCNTs/GNPs hybrid nanofluid and mini-tube. Int J Heat Mass Transf. 2017;115:1121–31.
Suresh S, Venkitaraj KP, Selvakumar P, Chandrasekar M. Effect of Al2O3–Cu/water hybrid nanofluid in heat transfer. Exp Therm Fluid Sci. 2012;38:54–60.
Syam Sundar L, Singh MK, Sousa ACM. Heat transfer and friction factor of nanodiamond–nickel hybrid nanofluids flow in a tube with longitudinal strip inserts. Int J Heat Mass Transf. 2018;121:390–401.
Mashali F, Languri EM, Davidson J, Kerns D, Johnson W, Nawaz K, et al. Thermo-physical properties of diamond nanofluids: a review. Int J Heat Mass Transf. 2019;129:1123–35.
Minea AA. Hybrid nanofluids based on Al2O3, TiO2 and SiO2: numerical evaluation of different approaches. Int J Heat Mass Transf. 2017;104:852–60.
Yarmand H, Zulkifli NWBM, Gharehkhani S, Shirazi SFS, Alrashed AAAA, Ali MAB, et al. Convective heat transfer enhancement with graphene nanoplatelet/platinum hybrid nanofluid. Int Commun Heat Mass Transf. 2017;88:120–5.
Shah TR, Ali HM. Applications of hybrid nanofluids in solar energy, practical limitations and challenges: a critical review. Sol Energy. 2019;183:173–203.
Yang C, Wu X, Zheng Y, Qiu T. Heat transfer performance assessment of hybrid nanofluids in a parallel channel under identical pumping power. Chem Eng Sci. 2017;168:67–77.
Buongiorno J. Convective transport in nanofluids. J Heat Transf. 2006;128:240–50.
Hayat T, Nadeem S. An improvement in heat transfer for rotating flow of hybrid nanofluid: a numerical study. Can J Phys. 2018;96:1420–30.
Subhani M, Nadeem S. Numerical analysis of micropolar hybrid nanofluid. Appl Nanosci. 2018;9:1–13.
Hayat T, Nadeem S. Heat transfer enhancement with Ag–CuO/water hybrid nanofluid. Results Phys. 2017;7:2317–24.
Ashorynejad HR, Shahriari A. MHD natural convection of hybrid nanofluid in an open wavy cavity. Results Phys. 2018;9:440–55.
Izadi M, Mohebbi R, Delouei AA, Sajjadi H. Natural convection of a magnetizable hybrid nanofluid inside a porous enclosure subjected to two variable magnetic fields. Int J Mech Sci. 2019;151:154–69.
Mehryan SAM, Sheremet MA, Soltani M, Izadi M. Natural convection of magnetic hybrid nanofluid inside a double-porous medium using two-equation energy model. J Mol Liq. 2019;277:959–70.
Mehryan SAM, Izadi M, Namazian Z, Chamkha AJ. Natural convection of multi-walled carbon nanotube–Fe3O4/water magnetic hybrid nanofluid flowing in porous medium considering the impacts of magnetic field-dependent viscosity. J Therm Anal Calorim. 2019;138:1541–55.
Ghalambaz M, Mehryan SAM, Izadpanahi E, Chamkha AJ, Wen D. MHD natural convection of Cu–Al2O3 water hybrid nanofluids in a cavity equally divided into two parts by a vertical flexible partition membrane. J Therm Anal Calorim. 2019;138:1723–43.
Rejvani M, Saedodin S, Vahedi SM, Wongwises S, Chamkha AJ. Experimental investigation of hybrid nano-lubricant for rheological and thermal engineering applications. J Therm Anal Calorim. 2019;138:1823–39.
Mehryan SAM, Izadpanahi E, Ghalambaz M, Chamkha AJ. Mixed convection flow caused by an oscillating cylinder in a square cavity filled with Cu–Al2O3/water hybrid nanofluid. J Therm Anal Calorim. 2019;137:965–82.
Ghalambaz M, Doostani A, Izadpanahi E, Chamkha AJ. Conjugate natural convection flow of Ag–MgO/water hybrid nanofluid in a square cavity. J Therm Anal Calorim. 2019;139:1–16.
Wakif A, Boulahia Z, Sehaqui R. Numerical analysis of the onset of longitudinal convective rolls in a porous medium saturated by an electrically conducting nanofluid in the presence of an external magnetic field. Results Phys. 2017;7:2134–52.
Wakif A, Boulahia Z, Sehaqui R. Numerical study of the onset of convection in a Newtonian nanofluid layer with spatially uniform and non-uniform internal heating. J Nanofluids. 2017;6:136–48.
Wakif A, Boulahia Z, Mishra SR, Rashidi MM, Sehaqui R. Influence of a uniform transverse magnetic field on the thermo-hydrodynamic stability in water-based nanofluids with metallic nanoparticles using the generalized Buongiorno’s mathematical model. Eur Phys J Plus. 2018;133:1–16.
Wakif A, Boulahia Z, Ali F, Eid MR, Sehaqui R. Numerical analysis of the unsteady natural convection MHD Couette nanofluid flow in the presence of thermal radiation using single and two-phase nanofluid models for Cu–water nanofluids. Int J Appl Comput Math. 2018;4(81):1–27.
Wakif A, Boulahia Z, Amine A, Animasaun IL, Afridi MI, Qasim M, et al. Magneto-convection of alumina–water nanofluid within thin horizontal layers using the revised generalized Buongiorno’s model. Front Heat Mass Transf. 2019;12:1–15.
Rosseland S. Astrophysik und Atom-Theoretische Grundlagen. Berlin: Springer; 1931.
Chandrasekhar S. Hydrodynamic and hydromagnetic stability. Oxford: Oxford University Press; 1961.
Nield DA, Kuznetsov AV. Thermal instability in a porous medium layer saturated by a nanofluid: a revised model. Int J Heat Mass Transf. 2014;68:211–4.
Nield DA, Kuznetsov AV. The onset of convection in a horizontal nanofluid layer of finite depth: a revised model. Int J Heat Mass Transf. 2014;77:915–8.
Celli M, Kuznetsov AV. A new hydrodynamic boundary condition simulating the effect of rough boundaries on the onset of Rayleigh–Bénard convection. Int J Heat Mass Transf. 2018;116:581–6.
Corcione M. Empirical correlating equations for predicting the effective thermal conductivity and dynamic viscosity of nanofluids. Energy Convers Manag. 2011;52:789–93.
Hayat T, Nawaz S, Alsaedi A, Rafiq M. Mixed convective peristaltic flow of water based nanofluids with joule heating and convective boundary conditions. PLoS ONE. 2016;11:1–28.
Haynes WM, Lide DR, Bruno TJ. CRC handbook of chemistry and physics. Boca Raton: CRC Press; 2016.
Izadi M, Maleki NM, Pop I, Mehryan SAM. Natural convection of a hybrid nanofluid subjected to non-uniform magnetic field within porous medium including circular heater. Int J Numer Methods Heat Fluid Flow. 2019. https://doi.org/10.1108/HFF-08-2018-0428.
Kashyap D, Dass AK. Effect of boundary conditions on heat transfer and entropy generation during two-phase mixed convection hybrid Al2O3–Cu/water nanofluid flow in a cavity. Int J Mech Sci. 2019;157–158:45–59.
Garnett JCM. Colours in metal glasses, in metallic films and in metallic solutions. Proc R Soc Lond A R Soc. 1905;76:370–3.
Sihvola AH, Lindell IV. Effective permeability of mixtures. Espoo: Helsinki University of Technology; 1989.
Jana S, Salehi-Khojin A, Zhong W-H. Enhancement of fluid thermal conductivity by the addition of single and hybrid nano-additives. Thermochim Acta. 2007;462:45–55.
Shah NA, Animasaun IL, Ibraheem RO, Babatunde HA, Sandeep N, Pop I. Scrutinization of the effects of Grashof number on the flow of different fluids driven by convection over various surfaces. J Mol Liq. 2018;249:980–90.
Animasaun IL, Ibraheem RO, Mahanthesh B, Babatunde HA. A meta-analysis on the effects of haphazard motion of tiny/nano-sized particles on the dynamics and other physical properties of some fluids. Chin J Phys. 2019;60:676–87.
Wakif A, Animasaun IL, Satya Narayana PV, Sarojamma G. Meta-analysis on thermo-migration of tiny/nano-sized particles in the motion of various fluids. Chin J Phys. 2019.
Wakif A, Boulahia Z, Sehaqui R. A semi-analytical analysis of electro-thermo-hydrodynamic stability in dielectric nanofluids using Buongiorno’s mathematical model together with more realistic boundary conditions. Results Phys. 2018;9:1438–54.
Chand R. Electro-thermal convection in a Brinkman porous medium saturated by nanofluid. Ain Shams Eng J. 2015;8:633–41.
Platten JK, Legros JC. Convection in liquids. Berlin: Springer; 1984.
Acknowledgements
We would like to express our profound gratefulness to the editor and reviewers and for all of their insightful recommendations that assisted us to improve considerably this research work and its scientific content.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The authors declare that they have no known conflict of interest in the present manuscript.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Wakif, A., Chamkha, A., Thumma, T. et al. Thermal radiation and surface roughness effects on the thermo-magneto-hydrodynamic stability of alumina–copper oxide hybrid nanofluids utilizing the generalized Buongiorno’s nanofluid model. J Therm Anal Calorim 143, 1201–1220 (2021). https://doi.org/10.1007/s10973-020-09488-z
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10973-020-09488-z



