Abstract
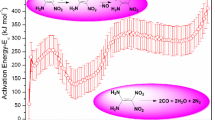
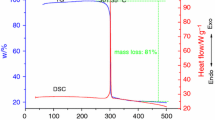
N-Nitrodihydroxyethyl dinitrate (DINA) is a useful energetic plasticizer in double-base propellant. To analyze the potential hazards of its thermal decomposition, the differential scanning calorimetry (DSC) was used to test the thermal behavior of DINA under non-isothermal and isothermal conditions. It is found from the non-isothermal DSC results, that the melting point of DINA is about 50 °C, the initial exothermic decomposition temperature (T onset) is between 177.46 and 189.60 °C with the heating rate 2, 4, 8 and 10 °C min−1, and its decomposition enthalpy (ΔH d) is about 3235.63 J g−1. Both the shapes of heat flow curves and activation energy curves, calculated by Friedman method, indicate the exothermic decomposition of DINA contains at least four reactions (P1–P4), which were separated into four curves by AKTS software according to the four peaks. The relatively constant E(α) of P1, P2, P3 and P4 verifies this four peaks are likely to be described by a single reaction model. The method proposed by ICTAC was used to determine the most suitable reaction function and kinetic parameters of DINA decomposition, the results show that P1 and P2 follow the Z–L–T model and the Avrami–Erofeev model, respectively, both of them are autocatalytic models, which is consistent with the isothermal DSC results. The reaction model of P3 cannot be obtained, while P4 corresponds to n-order reaction, f(α) = (1 − α)1.67.












Similar content being viewed by others
Abbreviations
- Β/°C min−1 :
-
Heating rate
- T melt/°C:
-
Melt temperature
- T onset/°C:
-
Onset temperature of decomposition
- T peak/°C:
-
Peak temperature of decomposition
- ΔH d/J g−1 :
-
Enthalpy of decomposition
- θ/s:
-
Isothermal induction period
- t/s:
-
Reaction time
- T/K:
-
Temperature
- α :
-
Reaction progress the extent of conversion
- E α/J mol−1 :
-
Activation energy
- A (α)/s−1 :
-
Pre-exponential factor
- f(α):
-
Differential form of the reaction model
- g(α):
-
Integral of the reaction model
References
Pi WF, Song XD, Zhang CH, Xie B, Wang JN, Zhao FQ. Combustion performance of double-based propellant with a lead free catalyst Gal-BiCu. Chin J Energ Mater. 2011;19(4):405–9.
Ksiqzczak A, Ostrowski M, Tomaszewski W. Thermochemistry of the binary system nitrocellulose+ N-nitrodiethanolamine dinitrate. J Therm Anal Calorim. 2008;94:275–9.
Ma YY. Effect of DINA on the performance of double base propellant. Chin J Energ Mater. 1995;3(2):31–6.
Zayed MA, Soliman AAW, Hassan MA. Evaluation of malonanilides as new stabilizers for double base propellants (I). J Hazard Mater. 2000;73:237–44.
Pouretedal HR, Damiri S, Ravanbod M, Haghdost M, Masoudi S. The kinetic of thermal decomposition of PETN, Pentastite and Pentolite by TG/DTA non-isothermal methods. J Therm Anal Calorim. 2017;129:521–9.
Liu S-H, Cao C-R, Lin Y-C, Shu C-M. Using thermal analysis and kinetic calculation method to assess the thermal stability of 2,20-azobis-(2-methylbutyronitrile). J Therm Anal Calorim. 2017;1:1–9.
Singh A, Sharma TC, Kishore P. Thermal degradation kinetics and reaction models of 1,3,5-triamino-2,4,6-trinitrobenzene-based plastic-bonded explosives containing fluoropolymer matrices. J Therm Anal Calorim. 2017;129:1403–14.
Campos MST, Fialho SL, Pereira BG, Yoshida MI, Oliveira MA. Kinetics studies of the degradation of sirolimus in solid state and in liquid medium. J Therm Anal Calorim. 2017;1:1–9.
Roduit B, Borgeat C, Berger B, Folly P, Andres H, Schadeli U, Vogelsanger B. Up-scaling of DSC data of high energetic materials. J Therm Anal Calorim. 2006;85(1):195–202.
Singh G, Pandey DK. Thermal studies on energetic compounds. J Therm Anal Calorim. 2004;76(2):507–19.
Pourmortazavi SM, Rahimi-Nasrabadi M, Kohsari I, Hajimirsadeghi SS. Non-isothermal kinetic studies on thermal decomposition of energetic materials. J Therm Anal Calorim. 2012;110(2):857–63.
Roduit B, Xia L, Folly P, et al. The simulation of the thermal behavior of energetic materials based on DSC and HFC signals. J Therm Anal Calorim. 2008;93(1):143–52.
Friedman HL. Kinetics of thermal degradation of char-forming plastics from thermogravimetry. Application to a phenolic plastic. J Polym Sci C Polym Symp. 1964;6(1):183–95.
Kissinger HE. Reaction kinetics in differential thermal analysis. Anal Chem. 1957;29(11):1702–6.
Flynn JH, Wall LA. A quick, direct method for the determination of activation energy from thermogravimetric data. J Polym Sci C Polym Lett. 1966;4(5):323–8.
AKTS. AKTS-thermokinetics and AKTS-thermal safety software. http://www.akts.com; Advances Kinetics and Technology Solutions. 2013.
Chen JR, Cheng SY, Yuan MH, Kossoy AA, Shu CM. Hierarchical kinetic simulation for autocatalytic decomposition of cumene hydroperoxide at low temperatures. J. Thermal Anal Calorim. 2009;96(3):751–8.
Li XR, Koseki H. SADT prediction of autocatalytic material using isothermal calorimetry analysis. Thermochim Acta. 2005;431(1/2):113–6.
Stoessel F. Thermal safety of chemical processes. Weinheim: Wiley-VCH; 2008. p. 279–82.
Tseng JM, Wu TC, Hsieh TF, Huang PJ, Lin CP. The thermal hazard evaluation of 1,1-di(tert-butylperoxy) cyclohexane by DSC using non-isothermal and isothermal kinetic simulation. J Loss Prev Process Ind. 2012;25(4):703–8.
Advanced Kinetics and Technology Solutions [EB/OL]. [2012-05-04]. http://www.akts.com.
Vyazovkin S, Burnham AK, Criado JM, et al. ICTAC kinetics committee recommendations for performing kinetic computations on thermal analysis data. Thermochim Acta. 2011;520:1–19.
Vyazovkin S, Chrissafis K, Lorenzo MLD, Koga N, Pijolat M, Roduit B, Sbirrazzuoli N, Suñol JJ. ICTAC kinetics committee recommendations for collecting experimental thermal analysis data for kinetic computations. Thermochim Acta. 2014;590:1–23.
Lu G, Yang T, Chen L, Zhou Y, Chen W. Thermal decomposition kinetics of 2-ethylhexyl nitrate under nonisothermal and isothermal conditions. J Therm Anal Calorim. 2015;124:471.
Malek J. The applicability of Johnson–Mehl–Avrami model in the thermal analysis of the crystallization kinetics of glasses. Thermochim Acta. 1995;267:61–73.
Hu RZ, Gao SL, Zhao FQ, Shi QZ, Zhang TL, Zhang JJ. Thermal analysis kinetics. 2nd ed. Beijing: Science Press; 2008. p. 149–66.
Criado JM, Malek J, Ortega A. Applicability of the master plots in kinetic analysis of non-isothermal data. Thermochim Acta. 1989;147:377–85.
Malek J. The kinetic-analysis of nonisothermal data. Thermochim Acta. 1992;200:257–69.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Zhang, J., Xue, B., Rao, G. et al. Thermal decomposition characteristic and kinetics of DINA. J Therm Anal Calorim 133, 727–735 (2018). https://doi.org/10.1007/s10973-017-6732-3
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10973-017-6732-3