Skip to main content
SpringerLink
Log in

Thermal (kinetic) stability of inclusion compounds on the basis of porous metal–organic frameworks

Dependence on the guest and framework properties

  • Published:
Journal of Thermal Analysis and Calorimetry Aims and scope Submit manuscript

Abstract

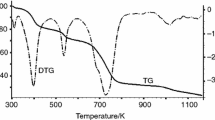
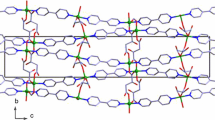
Metal–organic frameworks (MOFs) have promising practical applications in gas storage, in separation and purification of substances, and in catalysis. The standard process of the MOF production begins from the synthesis of the inclusion compound; the molecules of the used organic solvent are caught in the channels and caves of the MOF structure. These primary included guest molecules are excluded further by the evacuation or by the heating. We studied the correlation between the thermal (kinetic) stability of the inclusion compounds and the framework and guest molecules properties. The thermogravimetric curves were used for the kinetic studies; kinetic parameters of decomposition were estimated within the approaches of the non-isothermal kinetics (“model-free” kinetics and nonlinear regression methods). Studied compounds series: [Zn2(bdc)2(dabco)]·4DMF and [Zn4(DMF)(ur)2(ndc)4]·5DMF, [Zn4(DMF)(ur)2(ndc)4]·6benzene and [Zn4(DMF)(ur)2(ndc)4]·5toluene (bdc = bdc2− = terephthalate, dabco = 1,4-diazabicyclo[2.2.2]octane, DMF = dimethylformamide; ur = hexamethylenetetramine, ndc2− = 2,6-naphthalenedicarboxylate); [Mn(HCOO)2]·0.33dioxane and [Li2(H2btc)]·dioxane (H4btc = 1,2,4,5-benzenetetracarboxylic acid). The values of commonly used molecular kinetic diameters do not take into consideration the real molecular form and the size (e.g. benzene and toluene molecules have the same kinetic diameters 5.85 Å). Therefore, the ease of removal of guest molecules does not correlate with them directly.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. Hosny NM. Solvothermal synthesis, thermal and adsorption properties of metal–organic frameworks Zn and CoZn (DPB). J Therm Anal Calorim. 2015;122:89–95.

    Article  CAS  Google Scholar 

  2. Kirillov AM. Hexamethylenetetramine: an old new building block for design of coordination polymers. Coord Chem Rev. 2011;255:1603–22.

    Article  CAS  Google Scholar 

  3. Mani-Biswas M, Cagin T. Insights from theoretical calculations on structure, dynamics, phase behavior and hydrogen sorption in nanoporous metal organic frameworks. Comput Theor Chem. 2012;987:42–56.

    Article  CAS  Google Scholar 

  4. Lyszczek R, Iwan M. Investigation of desolvation process in lanthanide dinicotinates. J Therm Anal Calorim. 2011;103:633–9.

    Article  CAS  Google Scholar 

  5. Jiang C-H, Song L-F, Jiao C-L, Zhang J, Sun L-X, Xu F, Du Y, Cao Z. Exceptional thermal stability and thermodynamic properties of lithium based metal–organic framework. J Therm Anal Calorim. 2011;103:373–80.

    Article  CAS  Google Scholar 

  6. Sha J-Q, Wu L-H, Li S-X, Yang X-N, Zhang Yu, Zhang Q-N, Zhu P-P. Synthesis and structure of new carbohydrate metal–organic frameworks and inclusion complexes. J Mol Struct. 2015;1101:14–20.

    Article  CAS  Google Scholar 

  7. Dybtsev DN, Yutkin MP, Peresypkina EV, Virovets AV, Serre C, Ferey G, Fedin VP. Isoreticular homochiral porous metal–organic structures with tunable pore sizes. Inorg Chem. 2007;46:6843–5.

    Article  CAS  Google Scholar 

  8. Zhou Y-X, Sun L-X, Cao Z, Zhang J, Xu F, Song L-F, Zhao Z-M, Zou Y-J. Heat capacities and thermodynamic properties of M(HBTC)(4,4′-bipy)·3DMF (M = Ni and Co). J Therm Anal Calorim. 2012;110:949–54.

    Article  CAS  Google Scholar 

  9. Jang X, Jiao C-L, Sun Y-J, Li Z-B, Liu S, Zhang J, Wang Z-Q, Sun L-X, Xu F, Zhou H, Sawada Y. Heat capacities and thermodynamic properties of Co(3,5-PDC)(H2O). J Therm Anal Calorim. 2013;112:1579–85.

    Article  Google Scholar 

  10. Deb N. An investigation on solid-state pyrolytic decomposition of barium trihemiaquatris(oxalato)lanthanate(III)decahydrate using TG–DTA in air and DSC in nitrogen. J Therm Anal Calorim. 2013;112:1415–21.

    Article  CAS  Google Scholar 

  11. Yang Q, Xie G, Chen S, Gao S. Synthesis, structure and thermodynamics of supermolecular compound [Ni3(Hdatrz)6(sca)2(H2O)4]sca·11H2O (Hdatrz = 3,5-diamino-1,2,4-triazole, H2sca = succinic acid). J Therm Anal Calorim. 2012;110:979–84.

    Article  CAS  Google Scholar 

  12. Logvinenko VA, Yutkin MP, Zavakhina MS, Fedin VP. Porous metal–organic frameworks (MOFs) as matrices for inclusion compounds: kinetic stability under heating. J Therm Anal Calorim. 2012;109:555–60.

    Article  CAS  Google Scholar 

  13. Dybtsev DN, Yutkin MP, Samsonenko DG, Fedin VP, Nuzhdin AL, Bezrukov AA, Bryliakov KP, Talsi EP, Belosludov RV, Mizuseki H, Kawazoe Y, Subbotin OS, Belosludov VR. Modular homochiral porous coordination polymers: rational design, enantioselective guest exchange sorption and ab initio calculations of host–guest interactions. Chem Eur J. 2010;10:348–56.

    Google Scholar 

  14. Sapchenko SA, Samsonenko DG, Dybtsev DN, Melgunov MS, Fedin VP. Microporous sensor: gas sorption, guest exchange and guest-dependent luminescence of metal–organic framework. Dalton Trans. 2011;40:2196–203.

    Article  CAS  Google Scholar 

  15. Dybtsev DN, Chun H, Kim K. Rigid and flexible: a highly porous metal–organic framework with unusual guest-dependent dynamic behavior. Angew Chem Int Ed. 2004;43:5033–6.

    Article  CAS  Google Scholar 

  16. Dybtsev DN, Chun H, Yoon SH, Kim D, Kim K. Microporous manganese formate: a simple metal–organic porous material with high framework stability and highly selective gas sorption properties. J Am Chem Soc. 2004;126:32–3.

    Article  CAS  Google Scholar 

  17. Logvinenko V, Dybtsev D, Fedin V, Drebushchak V, Yutkin M. The stability of inclusion compounds under heating. Part I. Inclusion compounds of micro-porous manganese formate with included dioxane Mn(HCOO)2·1/3C4H8O2 and tetra-hydrofurane {Mn(HCOO)2·1/3C4H8O}. J Therm Anal Calorim. 2007;90:463–7.

    Article  CAS  Google Scholar 

  18. Logvinenko V. Stability of supramolecular compounds under heating. Thermodynamic and kinetic aspects. J Therm Anal Calorim. 2010;101:577–83.

    Article  CAS  Google Scholar 

  19. Aliev SB, Samsonenko DG, Rakhmanova MI, Dybtsev DN, Fedin VP. Syntheses and structural characterization of lithium carboxylate frameworks and guest-dependent photoluminescence study. Cryst Growth Des. 2014;14:4355–63.

    Article  CAS  Google Scholar 

  20. Netzsch Thermokinetics. In: Netzsch Thermokinetics. http://www.netzsch-thermal-analysis.com/us/products-%20%20solutions/advanced-software/thermokinetics.html.

  21. Moukhina E. Determination of kinetic mechanisms for reactions measured with thermoanalytical instruments. J Therm Anal Calorim. 2012;109:1203–14.

    Article  CAS  Google Scholar 

  22. Kissinger HE. Reaction kinetics in differential thermal analysis. Anal Chem. 1957;29:1702–6.

    Article  CAS  Google Scholar 

  23. Friedman HL. Kinetics of thermal degradation of char-forming plastics from thermogravimetry. J Polym Sci. 1963;6:183–95.

    Google Scholar 

  24. Ozawa T. A new method of analyzing thermogravimetric data. Bull Chem Soc Jpn. 1965;38:1881–6.

    Article  CAS  Google Scholar 

  25. Ozawa T. Estimation of activation energy by isoconversion methods. Thermochim Acta. 1992;203:159–65.

    Article  CAS  Google Scholar 

  26. Flynn JH, Wall LA. General treatment of the thermogravimetry of polymers. J Res Natl Bur Stand. 1966;70:478–523.

    Google Scholar 

  27. Opfermann J, Kaisersberger E. An advantageous variant of the Ozawa–Flynn–Wall analysis. Thermochim Acta. 1992;203:167–75.

    Article  CAS  Google Scholar 

  28. Opfermann JR, Kaisersberger E, Flammersheim HJ. Model-free analysis of thermo-analytical data-advantages and limitations. Thermochim Acta. 2002;391:119–27.

    Article  CAS  Google Scholar 

  29. Vyazovkin S. Model-free kinetics: staying free of multiplying entities without necessity. J Therm Anal Calorim. 2006;83:45–51.

    Article  CAS  Google Scholar 

  30. Simon P. Single-step kinetics approximation employing non-Arrhenius temperature functions. J Therm Anal Calorim. 2005;79:703–8.

    Article  CAS  Google Scholar 

  31. Simon P. The single-step approximation: attributes, strong and weak sides. J Therm Anal Calorim. 2007;88:709–15.

    Article  CAS  Google Scholar 

  32. Borchard HJ, Daniels F. The application of differential thermal analysis to the study of reaction kinetics. J Am Chem Soc. 1957;79:41–6.

    Article  Google Scholar 

  33. Vyazovkin S, Burnham AK, Criado JM, Luis A, Perez-Maqueda LA, Popescu C, Sbirrazzuoli N. ICTAC Kinetics Committee recommendations for performing kinetic computations on thermal analysis data. Thermochim Acta. 2011;520:1–19.

    Article  CAS  Google Scholar 

  34. Vyazovkin S, Chrissafis K, Di Lorenzo M-R, Koga N, Pijolat M, Roduit B, Sbirrazzuoli N, Suñol J-J. ICTAC Kinetics Committee recommendations for collecting experimental thermal analysis data for kinetic computations. Thermochim Acta. 2014;590:1–23.

    Article  CAS  Google Scholar 

  35. Vyazovkin S. Isoconversional kinetics of thermally stimulated processes. Berlin: Springer; 2015.

    Book  Google Scholar 

  36. Simon P, Thomas P, Dubaj T, Cibulkova Z, Peller A, Veverka M. The mathematical incorrectness of the integral isoconversional methods in case of variable activation energy and the consequences. J Therm Anal Calorim. 2014;115:853–9.

    Article  CAS  Google Scholar 

  37. Simon P, Dubaj T, Cibulkova Z. Equivalence of the Arrhenius and non-Arrhenian temperature functions in the temperature range of measurement. J Therm Anal Calorim. 2015;120:231–8.

    Article  CAS  Google Scholar 

  38. Sestak J. Is the original Kissinger equation obsolete today: not obsolete the entire non-isothermal kinetics? J Therm Anal Calorim. 2014;117:3–7.

    Article  CAS  Google Scholar 

  39. Galwey AK. What theoretical and/or chemical significance is to be attached to the magnitude of an activation energy determined for a solid-state decomposition by thermal analysis? J Therm Anal Calorim. 2006;86:267–86.

    Article  CAS  Google Scholar 

  40. Roura P, Farjas J. Analytical solution for the Kissinger equation. J Mater Res. 2009;24:3095–8.

    Article  CAS  Google Scholar 

  41. Holba P, Šesták J. Imperfections of Kissinger evaluation method and crystallization kinetics. Glass Physics Chem. 2014;40:486–91 (translation from Russian journal).

    Article  CAS  Google Scholar 

  42. Dubaj T, Cibulková Z, Šimon P. An incremental isoconversional method for kinetic analysis based on the orthogonal distance regression. J Comput Chem. 2015;36:392–8.

    Article  CAS  Google Scholar 

  43. Logvinenko V. Stability and reactivity of coordination and inclusion compounds in the reversible processes of thermal dissociation. Thermochim Acta. 1999;340–341:293–9.

    Article  Google Scholar 

  44. Logvinenko V, Drebushchak V, Pinakov D, Chekhova G. Thermodynamic and kinetic stability of inclusion compounds under heating. J Therm Anal. 2007;90:23–30.

    Article  CAS  Google Scholar 

  45. Logvinenko VA, Sapchenko SA, Fedin VP. Thermal decomposition of inclusion compounds on the base of the metal–organic framework [Zn4(dmf)(ur)2(ndc)4]. Part I. J Therm Anal Calorim. 2014;117:747–53.

    Article  CAS  Google Scholar 

  46. Solomons TWG, Fryhle CB. Organic Chemistry. 9th ed. New York: Wiley; 2006.

    Google Scholar 

  47. Hauptmann S, Graefe J, Remane H. Lehrbuch der organischen Chemie. Leipzig: VEB Deutscher Verlag fur Grundstoffindustrie; 1976.

    Google Scholar 

  48. Poling BE, Prausnitz JM, O’Connell JP. The properties of gases and liquids. New York: McGraw-Hill; 2001.

    Google Scholar 

  49. Logvinenko VA, Sapchenko SA, Fedin VP. Thermal decomposition of inclusion compounds on the base of the metal–organic framework [Zn4(dmf)(ur)2(ndc)4]. Part II. J Therm Anal Calorim. 2016;123:697–702.

    Article  CAS  Google Scholar 

  50. Logvinenko V, Dybtsev D, Fedin V, Drebushchak V, Yutkin M. The stability of inclusion compounds under heating. Part 2. Inclusion compounds of layered zinc camphorate, linked by linear N-donor ligands. J Therm Anal Calorim. 2010;100:183–93.

    Article  CAS  Google Scholar 

  51. Serin B, Ellickson RT. Determination of diffusion coefficients. J Chem Phys. 1941;9:742–7.

    Article  CAS  Google Scholar 

  52. Logvinenko VA, Aliev SB, Fedin VP. Thermal (kinetic) stability of the inclusion compound on the base of Li-containing MOF [Li2(H2btc)]·dioxane. J Therm Anal Calorim. 2015;120:53–8.

    Article  CAS  Google Scholar 

  53. Breck DW. Zeolite molecular sieves: structure, chemistry and use. New York: Wiley; 1974.

    Google Scholar 

  54. Matteucci S, Yampolskii Y, Freeman BD, Pinnau I. Transport of gases and vapors in glassy and rubbery polymers. In: Freeman BD, Yampolskii Y, Pinnau I, editors. Materials science of membranes for gas and vapor separation. New York: Wiley; 2006. p. 1–47.

    Chapter  Google Scholar 

  55. Elshof JE, Abadal CR, Sekulic J, Chowdhury SR, Blank DHA. Transport mechanisms of water and organic solvents through microporous silica in pervaporization of binary liquids. Microporous Mesoporous Mater. 2003;65:197–208.

    Article  Google Scholar 

  56. Freude D. Molecular physics. Letter notes. Chapter 2. Size, mass and kinetics of molecules. Version October 2004. http://www.uni-leipzig.de/~energy/pdf/freume2.pdf.

  57. Mehio N, Dai Sh, Jiang D. Quantum mechanical basis for kinetic diameters of small gaseous molecules. J Phys Chem A. 2014;118:1150–4.

    Article  CAS  Google Scholar 

Download references

Acknowledgements

This work was partially supported by the Russian Foundation for Basic Research (Grant 14-03-00291).

Author information

Authors and Affiliations

  1. Nikolaev Institute of Inorganic Chemistry, Siberian Branch of Russian Academy of Sciences, Ac. Lavrentyev Ave 3, Novosibirsk-90, Russia, 630090

    Vladimir A. Logvinenko, Sokhrab B. Aliev, Vsevolod A. Bolotov, Danil N. Dybtsev & Vladimir P. Fedin

  2. Novosibirsk State University, Pirogova St 2, Novosibirsk-90, Russia, 630090

    Vladimir A. Logvinenko, Danil N. Dybtsev & Vladimir P. Fedin

Authors
  1. Vladimir A. Logvinenko
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Sokhrab B. Aliev
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Vsevolod A. Bolotov
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Danil N. Dybtsev
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Vladimir P. Fedin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Vladimir A. Logvinenko.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Logvinenko, V.A., Aliev, S.B., Bolotov, V.A. et al. Thermal (kinetic) stability of inclusion compounds on the basis of porous metal–organic frameworks. J Therm Anal Calorim 127, 779–787 (2017). https://doi.org/10.1007/s10973-016-5398-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10973-016-5398-6

Keywords

Search

Navigation