Skip to main content
SpringerLink
Log in

Axially Symmetric Temperature Field of a Truncated Conic Shell with Variable Heat-Transfer Coefficients

  • Published:
Journal of Mathematical Sciences Aims and scope Submit manuscript

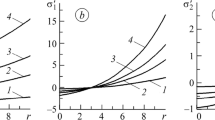
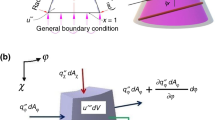
A method for reducing the heat-conduction problem for a truncated conic shell to the solution of a system of integral equations with Volterra and Fredholm integral operators of the second kind is proposed for the case of coordinate-dependent heat-transfer coefficients and ambient temperature. The system is solved numerically by the method of quadrature formulas. The numerical analyses of the distributions of mean temperature and temperature moment are performed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. A. F. Verlan’ and V. S. Sizikov, Methods for the Solution of Integral Equations with Computer Programs, [in Russian], Naukova Dumka, Kiev, 1978.

    Google Scholar 

  2. V. M. Gembara, “Axially symmetric stressed state of a conic shell under nonstationary thermal conditions,” Zbir. Robit Aspirant. Mekh.-Mat. Fiz. Fak. Lviv. Ord. Len. Derzh. Univ. Im. I. Franka, Issue 1, 11–18 (1961).

  3. A. D. Kovalenko, Ya. M. Grigorenko, and L. A. Il’in, Theory of Thin Conic Shells and Its Application to Machine Building [in Russian], Izd. Akad. Nauk Ukr. SSR, Kiev (1963).

    Google Scholar 

  4. R. M. Kushnir, “Key equations for a composite cylindrical shell with internal stresses and structural defects,” Mat. Met. Fiz.-Mekh. Polya, 44, No. 4, 77–84 (2001).

    MATH  MathSciNet  Google Scholar 

  5. B. V. Nerubailo, L. G. Smirnov, and O. A. Strukova, “On the solution of the problem of thermoelasticity of conic shells,” Izv. RAN. Mekh. Tverd. Tela, No. 4, 107–121 (2008).

  6. Ya. S. Pidstryhach and Yu. M. Kolyano, “Influence of heat transfer in the local heating of thin-walled structural elements,” Dokl. Akad. Nauk SSSR, 225, No. 4, 778–781 (1975).

    Google Scholar 

  7. Ya. S. Pidstryhach, Yu. M. Kolyano, V. I. Gromovyk, and V. L. Lozben’, Thermoelasticity of Bodies with Variable Coefficients of Heat Transfer [in Russian], Naukova Dumka, Kiev (1977).

    Google Scholar 

  8. Ya. S. Pidstryhach and R. N. Shvets, Thermoelasticity of Thin Shells [in Russian], Naukova Dumka, Kiev (1978).

    Google Scholar 

  9. B. S. Khapko, “Nonsteady temperature fields in a conic shell with cuts when the heat sources are distributed along an arbitrary curve,” in: Proc. of the Ninth Conf. of Young Scientists at the Institute for Applied Problems in Mechanics and Mathematics, Acad. Sci. Ukr. SSR, Lvov, 1982 [in Russian], Pt. 1, pp. 190–193, Dep. at VINITI 10.01.84; No. 323-84, VINITI, Moscow (1984).

  10. B. S. Khapko, “On the solution of the boundary-value problem for partial differential equations with impulsive coefficients,” Mat. Met. Fiz.-Mekh. Polya, 49, No. 3, 47–55 (2006).

    MATH  MathSciNet  Google Scholar 

  11. B. S. Khapko, and A. I. Chyzh, “Thermal displacements of a round plate with radius-dependent coefficients of heat transfer,” Mashynoznavstvo, No. 11, 19–23 (2009).

  12. A. I. Chyzh, “Thermoelastic state in a round plate with concentric hole for the radius-dependent coefficients of heat transfer from the faces,” in: Abstr. of the Pidstryhach Conference of Young Scientists on Contemporary Problems of Mechanics and Mathematics [in Ukrainian], Pidstryhach Institute for Applied Problems in Mechanics and Mathematics, Ukrainian National Academy of Sciences, Lviv (2009), p. 46.

  13. R. N. Shvets, B. S. Khapko, and A. I. Chyzh, “Heat-conduction equation for shells with breaks in the case of variable coefficients of heat transfer,” Teor. Prikl. Mekh., Issue 1 (47), 69–76 (2010).

    Google Scholar 

  14. K. C. Jane and Y. H. Wu, “A generalized thermoelasticity problem of multilayered conical shells,” Int. J. Solids Struct., 41, No. 9-10, 2205–2233 (2004).

    Article  MATH  Google Scholar 

  15. Wang Yong-gang and Dai Shi-liang, “Thermoelastically coupled axisymmetric nonlinear vibrations of shallow spherical and conical shells,” Appl. Math. Mech., 25, No. 4, 430–439 (2004).

    Article  Google Scholar 

  16. X. Zhao and K. M. Liew, “An element-free analysis of mechanical and thermal buckling of functionally graded conical shell panels,” Int. J. Numer. Meth. Eng., 86, No. 3, 269–285 (2011).

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Pidstryhach Institute for Applied Problems in Mechanics and Mathematics, Ukrainian Academy of Sciences, Lviv, Ukraine

    B. S. Khapko, A. I. Chyzh & R. M. Shvets’

Authors
  1. B. S. Khapko
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. A. I. Chyzh
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. R. M. Shvets’
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Translated from Matematychni Metody ta Fizyko-Mekhanichni Polya, Vol. 55, No. 4, pp. 161–170, October–December, 2012.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Khapko, B.S., Chyzh, A.I. & Shvets’, R.M. Axially Symmetric Temperature Field of a Truncated Conic Shell with Variable Heat-Transfer Coefficients. J Math Sci 198, 204–216 (2014). https://doi.org/10.1007/s10958-014-1784-4

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10958-014-1784-4

Keywords

Search

Navigation