Abstract
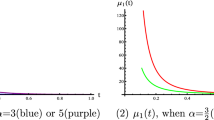
In this paper we study the gap probability problem in the Gaussian unitary ensembles of \(n\) by \(n\) matrices : The probability that the interval \(J := (-a,a)\) is free of eigenvalues. In the works of Tracy and Widom, Adler and Van Moerbeke, and Forrester and Witte on this subject, it has been shown that two Painlevé type differential equations arise in this context. The first is the Jimbo–Miwa–Okomoto \(\sigma \)-form and the second is a particular Painlevé IV. Using the ladder operator technique of orthogonal polynomials we derive three quantities associated with the gap probability, denoted as \(\sigma _n(a)\), \(R_n(a)\) and \(r_n(a)\). We show that each one satisfies a second order Painlevé type differential equation as well as a discrete Painlevé type equation. In particular, in addition to providing an elementary derivation of the aforementioned \(\sigma \)-form and Painlevé IV we are able to show that the quantity \(r_n(a)\) satisfies a particular case of Chazy’s second degree second order differential equation. For the discrete equations we show that the quantity \(r_n(a)\) satisfies a particular form of the modified discrete Painlevé II equation obtained by Grammaticos and Ramani in the context of Backlund transformations. We also derive second order second degree difference equations for the quantities \(R_n(a)\) and \(\sigma _n(a)\).
Similar content being viewed by others
References
Adler, M., Van Moerbeke, P.: Hermitian, symmetric and symplectic random ensembles : PDEs for the distribution of the spectrum. Ann. Math. 153, 149–189 (2001)
Basor, E., Chen, Y.: Painlevé V and the distribution function of a discontinuous linear statistic in the Laguerre unitary ensembles. J. Phys. A: Math. Theor. 42, 035203 (2009)
Basor, E., Chen, Y., Ehrhardt, T.: Painlevé V and time-dependent Jacobi polynomials. J. Phys. A: Math. Theor. 43, 015204 (2010)
Bertola, M., Eynard, B., Harnad, J.: Semiclassical orthogonal polynomials, matrix models and isomonodromic tau functions. Comm. Math. Phys. 263, 401–437 (2006)
Yang Chen, Z.D. Bai, Y-C. Liang, Random Matrix Theory and its Applications : Multivariate Statistics and Wireless Communications. Lecture Note Series, vol. 18, World Scientific Publishing 2009. Institute for Mathematical Sciences, National University of Singapore.
Chen, Y., Ismail, M.E.H.: Ladder operators and differential equations for orthogonal polynomials. J. Phys. A 30, 7817–7829 (1997)
Chen, Y., Ismail, M.: Ismail, Jacobi polynomials from compatibility conditions. Proc. Am. Math. Soc. 133, 465–472 (2005). (electronic)
Chen, Y., Its, A.: Painlevé III and a singular linear statistics in Hermitian random matrix ensembles. I. J. Approx. Theory 162, 270–297 (2010)
Chen, Y., McKay, M.R.: Coulumb fluid, Painlevé transcendents, and the information theory of MIMO systems. IEEE Trans. Inf. Theory 58, 4594–4634 (2012)
Chen, Y., Pruessner, G.: Orthogonal Polynomials with discontinuous weight. J. Phys. A 38(12), L191–L198 (2005)
Chen, Y., Zhang, L.: Painlevé VI and the unitary Jacobi ensembles. Stud. Appl. Math. 125, 91–112 (2010)
Cosgrove, C.M.: Chazy’s second-degree Painlevé equations. J. Phys. A 39, 11955 (2006)
Dai, D., Zhang, L.: Painlevé VI and Hankel determinants for the generalized Jacobi weight. J. Phys. A: Math. Theor. 43, 055207 (2010)
Fokas, A.S., Its, A.R., Kitaev, A.V.: The isomonodromy approach to matrix models in 2D quantum gravity. Comm. Math. Phys. 147(2), 395–430 (1992)
Forrester, P.J., Witte, N.S.: Gap probabilities in the finite and scaled Cauchy random matrix ensembles. Nonlinearity 13, 1965 (2000)
Haine, L., Vanderstichlen, D.: A centerless representation of the Virasoro algebra associated with the unitary circular ensemble. J. Comput. Appl. Math. 236(1), 19–27 (August 2011)
Jimbo, M., Miwa, T., Mori, Y., Sato, M.: Density matrix of an impenetrable Bose gas and the fifth Painlevé transcendent. Physica D 1(1), 80–158 (1980)
Magnus, A.P.: Painlevé-type differential equations for the recurrence coefficients of semi-classical orthogonal polynomials. J. Comput. Appl. Math. 57, 215–237 (1995)
Marcellán, F., Rocha, I.A.: On semiclassical linear functionals: integral representations. J. Comput. Appl. Math. 57(1–2), 239–249 (1995)
Mehta, M.L.: Random Matrices. Pure and Applied Mathematics Series, 3rd edn. Elsevier Academic Press, Amsterdam (2004)
Ramani, A., Grammaticos, B.: Miura transforms for discrete Painlevé equations. J. Phys. A 25, L633–L637 (1992)
G. Szegö, Orthogonal Polynomials, American Mathematical Society, New York, 1939. American Mathematical Society Colloquium Publications, vol 23 (1939)
Tracy, C.A., Widom, H.: Fredholm determinants, differential equations and matrix models. Comm. Math. Phys. 163, 33–72 (1994)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Cao, M., Chen, Y. & Griffin, J. Continuous and Discrete Painlevé Equations Arising from the Gap Probability Distribution of the Finite \(n\) Gaussian Unitary Ensembles. J Stat Phys 157, 363–375 (2014). https://doi.org/10.1007/s10955-014-1076-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10955-014-1076-x