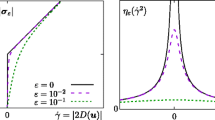
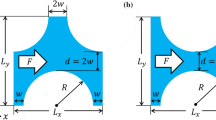
Abstract
It is shown how shear-thinning flow can be simulated without the need for numerical differentiation by following a lattice Boltzmann approach. The basic idea of is to combine the Cross model of viscosity with a 3D multiple relaxation time lattice Boltzmann method and to extract the required velocity derivatives from intrinsic quantities of the lattice Boltzmann scheme. Computational results are presented for a simple benchmark and for the simulation of liquid composite moulding.
Similar content being viewed by others
References
E. Aharonov D.H. Rothman (1993) ArticleTitleNon-Newtonian flow (through porous media): A lattice Boltzmann method Geophys. Res Lett. 20 679–682 Occurrence Handle1993GeoRL..20..679A
J.M. Buick C.A. Greated (2000) ArticleTitleGravity in a lattice Boltzmann model Phys. Rev. E 61 5307–5320 Occurrence Handle10.1103/PhysRevE.61.5307 Occurrence Handle2000PhRvE..61.5307B
M.M. Cross (1965) ArticleTitleRheology of non-Newtonian fluids: A new flow equation for pseudo-plastic systems J. Colloid Sci. 20 417–437 Occurrence Handle10.1016/0095-8522(65)90022-X
H.P. Darcy (1856) Les fontaines publiques de la ville de Dijon Dalmont Paris
U. Frisch D. d’Humières B. Hasslacher P. Lallemand Y. Pomeau J.-P. Rivet (1987) ArticleTitleLattice gas hydrodynamics in two and three dimensions Complex Systems 1 649–707 Occurrence Handle926931 Occurrence Handle0662.76101
I. Ginzbourg P.M. Adler (1994) ArticleTitleBoundary flow condition analysis for the three-dimensional lattice Boltzmann model J. Phys. II France 4 191–214 Occurrence Handle10.1051/jp2:1994123
I. Ginzburg K. Steiner (2002) ArticleTitleA free-surface lattice Boltzmann method for modelling the filling of expanding cavities by Bingham fluids., Phil Trans. R. Soc. Lond. A 360 453–466 Occurrence Handle2002RSPTA.360..453S Occurrence Handle1001.76083
I. Ginzburg K. Steiner (2003) ArticleTitleLattice Boltzmann model for free-surface flow and its application to filling process in casting J. Comput. Phys. 185 61–99 Occurrence Handle10.1016/S0021-9991(02)00048-7 Occurrence Handle2003JCoPh.185...61G Occurrence Handle2010158 Occurrence Handle1062.76554
L. Giraud D. d’Humières P. Lallemand (1997) ArticleTitleA lattice Boltzmann model for visco-elasticity Int. J. Mod. Phys. C 8 805–815 Occurrence Handle1997IJMPC...8..805G
L. Giraud D. d’Humières P. Lallemand (1998) ArticleTitleA lattice Boltzmann model for Jeffrey’s visco-elastic fluid Europhys. Lett. 42 625–630 Occurrence Handle10.1209/epl/i1998-00296-0 Occurrence Handle1998EL.....42..625G
X. He L.-S. Luo (1997) ArticleTitleLattice Boltzmann model for the incompressible Navier–Stokes equation J. Stat. Phys. 88 927–944 Occurrence Handle10.1023/B:JOSS.0000015179.12689.e4 Occurrence Handle1467637 Occurrence Handle0939.82042 Occurrence Handle1997JSP....88..927H
D. d’Humières, Generalized lattice Boltzmann equations, in Rarefied Gas Dynamics: Theory and Simulations (Progress in Astronautics and Aeronautics 159 (1992), AIAA, Washington DC.), pp. 450–458
D. d’Humières P. Lallemand (1993) ArticleTitleGaz sur réseau pour la représentation des ondes transverses C. R. Acad. Sci. Paris II 317 997–1001
M. Junk (2001) ArticleTitleA finite difference interpretation of the lattice Boltzmann method Numer. Methods Partial Differ. Equations 17 383–402 Occurrence Handle0987.76082 Occurrence Handle1833496 Occurrence Handle10.1002/num.1018
M. Junk Z. Yang (2003) ArticleTitleAnalysis of lattice Boltzmann boundary conditions PAMM 3 76–79 Occurrence Handle10.1002/pamm.200310320
Kehrwald D., Parallel lattice Boltzmann simulation of complex flows, in Proceedings of the NAFEMS Seminar “Simulation of Complex Flows (CFD) – Applications and Trends” (Niedernhausen bei Wiesbaden, Germany, 3–4 May 2004)
P. Lallemand D. d’Humières L.-S. Luo R. Rubinstein (2003) ArticleTitleTheory of the lattice Boltzmann equation: Three-dimensional model for linear visco-elastic fluids Phys. Rev. E 67 021203 Occurrence Handle10.1103/PhysRevE.67.021203 Occurrence Handle2003PhRvE..67b1203L Occurrence Handle1974620
R.G. Owens T.N. Phillips (2002) Computational Rheology Imperial College Press London Occurrence Handle1015.76002
Repsch M., Huber U., Maier M., Rief S., Kehrwald D., and Steiner K., Process simulation of LPM (Liquid Polymer Moulding) in special consideration of fluid velocity and viscosity characteristics, accepted for publication in the Proceedings of FPCM-7 (Newark, DE, 7–9 July 2004)
Repsch M., Huber U., Rief S., Kehrwald D., and Steiner K., New perceptions regarding the influence of RTM-process parameters on the microstructure of a non-crimp fibre fabric bed, submitted to Composites Part A
D.H. Rothman S. Zaleski (1997) Lattice-Gas Cellular Automata Cambridge University Press Cambridge Occurrence Handle0931.76004
S. Succi (2001) The Lattice Boltzmann Equation for Fluid Dynamics and Beyond Clarendon Press Oxford Occurrence Handle0990.76001
D. Wolf-Gladrow (2000) Lattice-Gas Cellular Automata and Lattice Boltzmann Models Springer Berlin Occurrence Handle0999.82054
K. Yasuda R.C. Armstrong R.E. Cohen (1981) ArticleTitleShear flow properties of concentrated solutions of linear and star branched polysterones Rheol. Acta. 20 163–178 Occurrence Handle10.1007/BF01513059
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Kehrwald, D. Lattice Boltzmann Simulation of Shear-Thinning Fluids. J Stat Phys 121, 223–237 (2005). https://doi.org/10.1007/s10955-005-5963-z
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1007/s10955-005-5963-z