Skip to main content
SpringerLink
Log in

Glass Formation in Binary Solutions of Acetaminophen with Guaifenesin and Mephenesin

  • Published:
Journal of Solution Chemistry Aims and scope Submit manuscript

Abstract

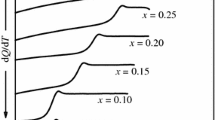
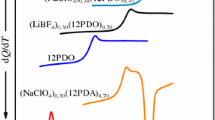
Dielectric behavior and glass formation in supercooled liquid guaifenesin (GFN) and its binary liquids with acetaminophen (ACT), and of ACT with mephenesin (MP), are reported using dielectric spectroscopy (10−3 Hz–2 MHz) and differential scanning calorimetry down to liquid nitrogen temperature. Solid–liquid phase equilibria of ACT + GFN and ACT + MP exhibit a simple eutectic of complete miscibility in the liquid state with eutectic points at 344.6 (±1) K and 337.5 (±1.4) K, respectively, and separate into two crystalline components in the solid state. The glass transition temperature (T g) measured for quenched binary liquids obeys a mixture rule. The primary relaxation process (α-process), can be well described by the Havriliak–Negami equation. A secondary relaxation process (β-process), found below T g, is Arrhenius in its temperature dependence, but may not be of inter-molecular or Johari–Goldstein (JG) type in nature. The “fragility” of the samples is discussed in the context of a coupling model. The supercooled liquid samples and binary liquids studied here are found to be “very fragile” in nature.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13

Similar content being viewed by others

References

  1. Wang, J., Angell, C.A.: Glass Structure by Spectroscopy. Marcel Dekkar, New York (1976)

    Google Scholar 

  2. Johari, G.P., Goldstein, M.: Viscous liquids and the glass transition III. Secondary relaxations in aliphatic alcohols and other non-rigid molecules. J. Chem. Phys. 55, 4245–4252 (1971)

    Article  CAS  Google Scholar 

  3. Johari, G.P., Goldstein, M.: Viscous liquids and the glass transition II. Secondary relaxations in glasses of rigid molecules. J. Chem. Phys. 53, 2372–2388 (1970)

    Article  CAS  Google Scholar 

  4. Okamoto, N., Oguni, M.: Discovery of crystal nucleation preceding much below the glass transition temperature in a supercooled liquid. Solid State Commun. 99, 53–56 (1996)

    Article  CAS  Google Scholar 

  5. Hikima, T., Hanaya, M., Oguni, M.: β-Molecular rearrangement process, but not an α-process, as governing the homogeneous crystal-nucleation rate in a supercooled liquid. Bull. Chem. Soc. Jpn. 69, 1863–1868 (1996)

    Article  CAS  Google Scholar 

  6. Hikima, T., Hanaya, M., Oguni, M.: Microscopic observation of a peculiar crystallization in the glass transition region and β-process as potentially controlling the growth rate in triphenylethylene. J. Mol. Struct. 479, 245–250 (1999)

    Article  CAS  Google Scholar 

  7. Paladi, F., Oguni, M.: Anomalous generation and extinction of crystal nuclei in nonequilibrium supercooled liquid o-benzylphenol. Phys. Rev. B. 65, 144202–144208 (2002)

    Article  Google Scholar 

  8. Paladi, F., Oguni, M.: Generation and extinction of crystal nuclei in an extremely nonequilibrium glassy state of salol. J. Phys. Condens. Matter. 15, 3909–3917 (2003)

    Article  CAS  Google Scholar 

  9. Hatase, M., Hanaya, M., Hikima, T., Oguni, M.: Discovery of homogeneous-nucleation-based crystallization in simple glass-forming liquid of toluene below its glass-transition temperature. J. Non-Cryst. Solids 307–310, 257–263 (2002)

    Article  Google Scholar 

  10. Murthy, S.S.N., Nayak, S.K.: Experimental study of the nature of the glass transition process in monohydroxy alcohols. J. Chem. Phys. 99, 5362–5368 (1983)

    Article  Google Scholar 

  11. Johari, G.P.: Glass transition and secondary relaxations in molecular liquids and crystals. Ann. New York Acad. Sci. 279, 117–140 (1976)

    Article  CAS  Google Scholar 

  12. Reid, C.J., Evans, M.W.: Dielectric and far infrared study of solutions in the glassy state from 100 Hz to 10 THz: discovery and characterization of the universal γ process. J. Chem. Phys. 76, 2576–2584 (1982)

    Article  CAS  Google Scholar 

  13. Wu, L., Nagel, S.R.: Secondary relaxation in o-terphenyl glass. Phys. Rev. D. 46, 11198–11200 (1992)

    Article  CAS  Google Scholar 

  14. Gangasharan, Murthy, S.S.N.: Study of α-, β-, and γ-relaxation processes in some supercooled liquids and supercooled plastic crystals. J. Chem. Phys. 99, 9865–9873 (1993)

    Article  CAS  Google Scholar 

  15. Shahin, Md, Murthy, S.S.N.: Sub-T g relaxations due to dipolar solutes in nonpolar glass-forming solvents. J. Chem. Phys. 122, 014507 (15) (2005)

    Article  Google Scholar 

  16. Johari, G.P., Kim, S., Shanker, R.M.: Dielectric relaxation and crystallization of ultraviscous melt and glassy states of aspirin, ibuprofen, progesterone, and quinidine. J. Pharm. Sci. 96, 1159–1175 (2007)

    Article  CAS  Google Scholar 

  17. Barro, M., Espeau, P., Tamarit, J.L., Perrin, M.A., Veglio, N., Ceolin, R.: Polymorphism of progesterone: relative stabilities of the orthorhombic phases I and II inferred from topological and experimental pressure temperature phase diagrams. J. Pharm. Sci. 98, 1657–1670 (2009)

    Article  Google Scholar 

  18. Kerc, J., Srcic, S., Mohar, M., Korbar, J.S.: Some physicochemical properties of glassy felodipine. Int. J. Pharm. 68, 25–33 (1991)

    Article  CAS  Google Scholar 

  19. Sailaja, U., Thayyil, M.S., Kumar, N.S.K., Govindaraj, G.: Molecular dynamics in liquid and glassy states of non-steroidal anti-inflammatory drug: ketoprofen. Eur. J. Pharm. Sci. 49, 333–340 (2013)

    Article  CAS  Google Scholar 

  20. Mahe, N., Perrin, M.A., Barrio, M., Nicolai, B., Rietveld, I.B., Tamarit, J.L., Ceolin, R.: Solid state studies of the triclinic (Z = 2) antiprotozoal drug ternidazole. J. Pharm. Sci. 100, 2258–2266 (2011)

    Article  CAS  Google Scholar 

  21. Mooter, G.V., Augustijins, P., Kinget, R.: Stability prediction of amorphous benzodiazepines by calculation of the mean relaxation time constant using the Williams Watts decay function. Eur. J. Pharm. Biopharm. 48, 43–48 (1999)

    Article  Google Scholar 

  22. Johari, G.P., Kim, S., Shanker, R.M.: Dielectric studies of molecular motions in amorphous solid and ultraviscous acetaminophen. J. Pharm. Sci. 94, 2207–2223 (2005)

    Article  CAS  Google Scholar 

  23. Espeau, P., Ceolin, R., Tamarit, J.L., Perrin, M.A., Gauchi, J.P., Leveiller, F.: Polymorphism of paracetamol: relative stabilities of the monoclinic and orthorhombic phase inferred from topological pressure temperature and temperature volume phase diagram. J. Pharm. Sci. 94, 524–539 (2005)

    Article  CAS  Google Scholar 

  24. Rengarajan, G.T., Beiner, M.: Relaxation behaviour and crystallization kinetics of amorphous acetaminophen. Lett. Drug Design Discov. 3, 723–730 (2006)

    Article  CAS  Google Scholar 

  25. Saini, M.K., Murthy, S.S.N.: Study of glass transition phenomenon in the supercooled liquid phase of methocarbamol, acetaminophen and mephenesin. Thermchim. Acta 575, 195–205 (2014)

    Article  CAS  Google Scholar 

  26. Johari, G.P., Kim, S., Shanker, R.M.: Dielectric study of equimolar acetaminophen–aspirin, acetaminophen–quinidine, and benzoic acid–progesterone molecular alloys in the glass and ultraviscous states and their relevance to solubility and stability. J. Pharm. Sci. 99, 1358–1374 (2010)

    Article  CAS  Google Scholar 

  27. Stott, P.W., Williams, A.C., Barry, B.W.: Transdermal delivery from eutectic systems: enhanced permeation of a model drug, ibuprofen. J. Control. Release 50, 297–308 (1998)

    Article  CAS  Google Scholar 

  28. Tu, W., Chen, Z., Gao, Y., Li, Z., Zhang, Y., Liu, R., Tian, Y., Wang, L.M.: Glass transition mixing thermodynamics of a binary eutectic system. Phys. Chem. Chem. Phys. 16, 3586–3592 (2014)

    Article  CAS  Google Scholar 

  29. Liu, D., Fei, X., Wang, S., Jiang, T., Su, D.: Increasing solubility and dissolution rate of drugs via eutectic mixture: itraconazole–poloxamer 188 system. Asian J. Pharm. Sci. 1, 213–221 (2006)

    CAS  Google Scholar 

  30. Gala, U., Pham, H., Chauhan, H.: Pharmaceutical applications of eutectic mixtures. J. Dev. Drugs. (2013). doi:10.4172/2329-6631.1000e130

    Google Scholar 

  31. McCrum, N.G., Read, D.E., Williams, G.: Inelastic and Dielectric Effects in Polymeric Solids. Wiley, New York (1967)

    Google Scholar 

  32. Jonscher, A. K.: Dielectric Relaxation in Solids. Chelsea, London (1983) (The dielectric loss in the frequency range 10−0.5–10−3 is obtained from the Hamon’s approximation: ε″(f) = i(t) * t/0.63C 0 V 0 with ft = 0.l, where i(t) is the discharging current at a time t, C 0 is the empty cell capacitance, and V 0 is the applied voltage)

  33. Hamon, B.V.: An approximation method for deducing dielectric loss factor from direct-current measurements. Inst. Monogr. 99, 151–155 (1952)

    Google Scholar 

  34. Kita, Y., Koizumi, N.: Remarks on the Hamon approximation. Adv. Mol. Relax. Process. 7, 13–20 (1975)

    Article  Google Scholar 

  35. Bredikhin, A.A., Gubaidullin, A.T., Bredikhina, Z.A., Krivolapov, D.B., Pashagin, A.V., Litvinov, I.A.: Absolute configuration and crystal packing for three chiral drugs prone to spontaneous resolution: guaifenesin, methocarbamol and mephenesin. J. Mol. Struct. 920, 377–382 (2009)

    Article  CAS  Google Scholar 

  36. Brittain, H.G.: Analytic Profiles of Drug Substances and Excipients, vol. 25, pp. 121–164. Acute Therapeutics, Inc, New Jersey (1998)

    Book  Google Scholar 

  37. Havriliak, S., Negami, S.: A complex plane analysis of α-dispersions in some polymer systems. J. Polym. Sci. Part C. Polym. Symp. 14, 99–117 (1966)

    Article  Google Scholar 

  38. Murthy, S. S. N.: Phase behavior of the supercooled aqueous solutions of dimethyl sulfoxide, ethylene glycol, and methanol as seen by dielectric spectroscopy, J. Phys. Chem. B. 101, 6043–6049 (1997) {The exact relation between f m and f 0 is f m  = f 0 {k′/[cos(απ/2) − sin(απ/2).k′]}1/(1 –α) where k′ = tan [(1–α)π/(2(1 + β))]}

  39. Murthy, S.S.N., Kumar, D.: Glass formation in organic binary liquids studied using differential scanning calorimetry. J. Chem. Soc. Faraday Trans. 89, 2423–2427 (1993)

    Article  CAS  Google Scholar 

  40. Murthy, S.S.N.: Temperature dependence of the dielectric relaxation process in glass-forming materials. J. Chem. Soc. Faraday Trans. 84, 671–677 (1988)

    Article  CAS  Google Scholar 

  41. Hill, N.E., Vaughan, W.E., Price, A.H., Davies, M.: Dielectric Properties and Molecular Behaviour. Van Nostrand Reinhold, London (1969)

    Google Scholar 

  42. Angell, C.A.: Relaxation in liquids, polymers and plastic crystals—strong/fragile patterns and problems. J. Non-Cryst. Solids 131–133, 13–31 (1991)

    Article  Google Scholar 

  43. Zhou, D., Zhang, G.G.Z., Law, D., Grant, D.J.W., Schmitt, E.A.: Physical stability of amorphous pharmaceuticals. Importance of configurational thermodynamic quantities and molecular mobility. J. Pharm. Sci. 91, 1863–1872 (2002)

    Article  CAS  Google Scholar 

  44. Espeau, P., Ceolin, R., Tamarit, J.L., Perrin, M.A., Gauchi, J.P., Leveiller, F.: Polymorphism of paracetamol. Relative stabilities of the monoclinic and orthorhombic phases inferred from topological pressure–temperature and temperature–volume phase diagrams. J. Pharm. Sci. 94, 524–539 (2005)

    Article  CAS  Google Scholar 

  45. Martino, P.D., Palmieri, G.F., Martelli, S.: Molecular mobility of the paracetamol amorphous form. Chem. Pharm. Bull. 48, 1105–1108 (2000)

    Article  Google Scholar 

  46. Klimova, K., Leitner, J.: DSC study and phase diagrams calculation of binary systems of paracetamol. Thermochim. Acta 550, 59–64 (2012)

    Article  CAS  Google Scholar 

  47. Capacioli, S., Nagai, K.L.: Relation between the α-relaxation and Johari–Goldstein β-relaxation of a component in binary miscible mixtures of glass-formers. J. Phys. Chem. B. 109, 9727–9735 (2005)

    Article  Google Scholar 

  48. Ngai, K.L., Capacioli, S.: Relation between the activation energy of the Johari–Goldstein β-relaxation and Tg of glass formers. Phys. Rev. E. 69, 031501 (5) (2004)

    Article  Google Scholar 

  49. Alvarez, F., Alegria, A., Colmenero, J.: Relationship between the time-domain Kohlrausch–Williams–Watts and frequency-domain Havriliak–Negami relaxation functions. Phys. Rev. B., Condens. Matter. 44, 7306–7312 (1991)

    Article  Google Scholar 

  50. Murthy, S.S.N., Gangasharan, Nayak, S.K.: Noval differential scanning calorimetric studies of supercooled organic liquids. J. Chem. Soc. Faraday Trans. 89, 509–514 (1993)

    Article  CAS  Google Scholar 

  51. Rodrigues, A.C., Viciosa, M.T., Danede, F., Affouard, F., Correia, N.T.: Molecular mobility of amorphous S-flurbiprofen: a dielectric relaxation spectroscopy approach. Mol. Pharm. 11, 112–130 (2013)

    Article  Google Scholar 

  52. Wang, L.M., Angell, C.A., Richert, R.: Fragility and thermodynamic in nonpolymeric glass-forming liquids. J. Chem. Phys. 125, 074505–074513 (2006)

    Article  Google Scholar 

  53. Wang, L.M., Angell, C.A.: Response to comment on direct determination of the fragility indices of glass forming liquids by differential scanning calorimetry: kinetic versus thermodynamic fragilities. J. Chem. Phys. 118, 10353–10355 (2003)

    Article  CAS  Google Scholar 

  54. Bohmer, R., Ngai, K.L., Angell, C.A., Plazek, D.J.: Nonexponential relaxations in strong and fragile glass formers. J. Chem. Phys. 99, 4201–4209 (1993)

    Article  Google Scholar 

  55. Shamblin, S.L., Hancock, B.C., Dupuis, Y., Pikal, M.J.: Interpretation of relaxation time constants for amorphous pharmaceutical systems. J. Pharm. Sci. 89, 417–427 (2000)

    Article  CAS  Google Scholar 

  56. Ngai, K.L.: Relation between some secondary relaxations and the α relaxations in glass-forming materials according to the coupling model. J. Chem. Phys. 109, 6982–6994 (1998)

    Article  CAS  Google Scholar 

  57. Colby, R.H.: Dynamic scaling approach to glass formation. Phys. Rev. E 61, 1783–1792 (2000)

    Article  CAS  Google Scholar 

  58. Drozd-Rzoska, A., Rzoska, S.J., Pawlus, S., Garcia, M., Tammarit, J.L.: Evidence for critical like behavior in ultra-slowing glass forming systems. Phys. Rev. E 82, 031501–031509 (2010)

    Article  Google Scholar 

  59. Martinez Garcia, J.C., Tamrit, J.L., Rzoska, S.J.: Enthalpy space analysis of the evolution of the primary relaxation time in ultra-slowing system. J. Chem. Phys. 134, 024512–024519 (2011)

    Article  CAS  Google Scholar 

Download references

Acknowledgments

The authors like to thank Dept. of Science & Technology & UGC, Govt. of India for the financial support. One of the authors (Manoj K. Saini) acknowledges the Research fellowship from UGC, India.

Author information

Authors and Affiliations

  1. School of Physical Sciences, Jawaharlal Nehru University, New Delhi, 110067, India

    Manoj K. Saini & S. S. N. Murthy

Authors
  1. Manoj K. Saini
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. S. S. N. Murthy
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. S. N. Murthy.

Electronic supplementary material

Below is the link to the electronic supplementary material.

Supplementary material 1 (PDF 285 kb)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Saini, M.K., Murthy, S.S.N. Glass Formation in Binary Solutions of Acetaminophen with Guaifenesin and Mephenesin. J Solution Chem 44, 1723–1748 (2015). https://doi.org/10.1007/s10953-015-0364-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10953-015-0364-7

Keywords

Search

Navigation