Skip to main content
SpringerLink
Log in

Effect of sample’s length on flow properties of open-cell metal foam and pressure-drop correlations

  • Published:
Journal of Porous Materials Aims and scope Submit manuscript

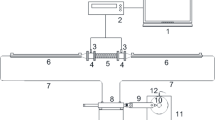
Abstract

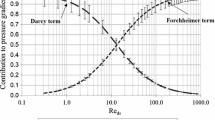
Many applications require fluid flow through the open pores of metal foam. The foam is usually treated as a porous medium for which the Darcy law and the Hazen-Dupuit-Darcy (or Forchheimer) equation are used to describe the pressure drop, and for obtaining the two important flow properties, i.e., the permeability and the form drag coefficient. Little or no attention is paid to the length (or thickness) of the porous medium in the flow direction. This paper establishes a minimum length necessary for the foam to have length-independent (or bulk) permeability and form drag coefficient. This minimum length is obtained experimentally for various types of open-cell aluminum foam subjected to airflow in the Forchheimer regime. Below this thickness values of the two key flow properties are not constant, and they include entrance/exit effects, which may explain some of the discrepancies in the reported values in the literature. The Forchheimer equation was recast in two different manners, which resulted in new non-dimensional numbers- one representing the form drag and the other the viscous drag. These numbers correlated very well with the thickness of the porous medium. The obtained correlations allow for determining the pressure drop given only the velocity and the thickness of an aluminum foam sample.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

Abbreviations

C :

Form drag coefficient (m−1)

C D :

Dimensionless form drag coefficient

C V :

Dimensionless viscous drag coefficient

F :

Universal drag coefficient (dimensionless)

f :

Friction factor (dimensionless)

f ND :

Viscous friction factor (dimensionless)

K :

Permeability (m2)

L :

Thickness of foam sample in the flow direction (m or cells)

p :

Static pressure (Pa)

p i :

Inlet pressure (Pa)

p o :

Exit pressure (Pa)

Re:

Reynolds number

V :

Darcy velocity (m/s)

δ:

Uncertainty (%)

Δ:

Change

ε:

Porosity (%)

μ:

Kinematic viscosity of air (kg/m.s)

ρ:

Density of air (kg/m3)

References

  1. J. Zhou, W.O. Soboyejo, C. Mercer, Metall. Mater. Trans. 33A(5), 413–1427 (2002)

    Google Scholar 

  2. M.F. Ashby, A.G. Evans, N.A. Fleck, L.J. Gibson, J.W. Hutchinson, H.N.G. Wadley, Metal Foams, a Design Guide (Butterworth-Heinemann, Woburn, 2000)

    Google Scholar 

  3. W. Azzi, W.L. Roberts, A. Rabiei, Mater. Des. 28, 569–574 (2007)

    Article  Google Scholar 

  4. J.J. Hwang, G.J. Hwang, R.H. Yeh, C.H. Chao, J. Heat Trans. 124, 120–129 (2002)

    Article  CAS  Google Scholar 

  5. J.L. Lage, B.V. Antohe, D.A. Nield, J. Fluids Eng. 119, 700–706 (1997)

    Article  CAS  Google Scholar 

  6. D. Seguin, A. Montillet, J. Comiti, Chem. Eng. Sci. 53(21), 3751–3761 (1998)

    Article  CAS  Google Scholar 

  7. L. Tadrist, M. Miscevic, O. Rahli, F. Topin, Exp. Therm. Fluid Sci. 28, 193–199 (2004)

    Article  CAS  Google Scholar 

  8. S.Y. Kim, J.W. Paek, B.H. Kang, J. Heat Trans. 122, 572–578 (2000)

    Article  CAS  Google Scholar 

  9. J.W. Paek, B.H. Kang, S.Y. Kim, J.M. Hyun, Int. J. Thermophys. 21(2), 453–464 (2000)

    Article  CAS  Google Scholar 

  10. J.S. Noh, K.B. Lee, C.G. Lee, Int. Commun. Heat Mass. Trans. 33, 434–444 (2006)

    Article  Google Scholar 

  11. A. Bhattacharya, V.V. Calmidi, R.L. Mahajan, Int. J. Heat Mass. Trans. 45, 1017–1031 (2002)

    Article  CAS  Google Scholar 

  12. J.P. du Plessis, A. Montillet, J. Comiti, J. Legrand, Chem. Eng. Sci. 49, 3545–3553 (1994)

    Article  CAS  Google Scholar 

  13. J.G. Fourie, J.P. du Plessis, Chem. Eng. Sci. 57, 2781–2789 (2002)

    Article  CAS  Google Scholar 

  14. J.F. Despois, A. Mortensen, Acta Mater. 53, 1381–1388 (2005)

    Article  CAS  Google Scholar 

  15. K. Boomsma, D. Poulikakos, Y. Ventikos, Int. J. Heat Fluid Flow 24, 825–834 (2003)

    Article  Google Scholar 

  16. K. Boomsma, D. Poulikakos, J. Fluids Eng. 124, 263–272 (2002)

    Article  CAS  Google Scholar 

  17. B. Antohe, J.L. Lage, D.C. Price, R.M. Weber, J. Fluids Eng. 11, 404–412 (1997)

    Article  Google Scholar 

  18. C. Naakteboren, P.S. Krueger, J.L. Lage, in Proceedings of the International Conference on Porous Media and Applications, Evora, Portugal, 24–27 May 2004

  19. C. Naakteboren, P.S. Krueger, J.L. Lage, in Proceedings of the ASME Fluids Engineering Summer Meeting and Exhibit, Houston, TX, 19–20 June 2005

  20. M. Medraj, E. Baril, V. Loya, L.P. Lefebvre, J. Mat. Sci. 42, 4372–4383 (2007)

    Article  CAS  Google Scholar 

  21. M.D.M. Innocentini, L.P. Lefebvre, R. V. Meloni, E. Baril (2009) J. Porous Mat. doi: 10.1007/s10934-009-9312-5

  22. E. Baril, A. Mostafid, L.P. Lefebvre, M. Medraj, Adv. Eng. Mat. 10(9), 889–894 (2008)

    Article  CAS  Google Scholar 

  23. K.C. Leong, L.W. Jin, Int. J. Ht. Fld. Fl. 27, 144–153 (2006)

    Article  CAS  Google Scholar 

  24. A. Bhattacharya, R.L. Mahajan, J. Elec. Pack 124, 155–163 (2002)

    Article  CAS  Google Scholar 

  25. K. Boomsma, D. Poulikakos, F. Zwick, Mech. Mat. 35, 1161–1176 (2003)

    Article  Google Scholar 

  26. J.F. Liu, W.T. Wu, W.C. Chiu, W.H. Hsieh, Exp. Therm. Fld. Sci. 30, 329–336 (2006)

    Article  Google Scholar 

  27. O. Reutter, E. Smirnova, J. Sauerhering, S. Angel, T. Fend, R. Pitz-Paal, J. Fluids Eng. 130, 201–205 (2008)

    Article  Google Scholar 

  28. J.P. Bonnet, F. Topin, L. Tadrist, Transp. Porous Media. 73, 233–254 (2008)

    Article  CAS  Google Scholar 

  29. ISO 4638:1984—Polymeric materials, cellular flexible—Determination of air flow permeability

  30. ISO 7231:1984—Polymeric materials, cellular flexible—Method of assessment of air flow value at constant pressure-drop

  31. ASTM D737 Standard test method for air permeability of textile fabrics

  32. ASTM F 778–88 Standard methods for gas flow resistance testing of filtration media

  33. ASTM D3574-03 Standard test methods for flexible cellular Materials—slab, bonded, and molded urethane foams

  34. J.P. du Plessis, S. Woudberg, Chem. Eng. Sci. 63, 2576 (2008)

    Article  CAS  Google Scholar 

  35. J.L. Lage, B. Antohe, J. Fluids Eng. 122, 619–625 (2000)

    Article  CAS  Google Scholar 

  36. S. Ergun, Chem. Eng. Progress 48(2), 89–94 (1952)

    CAS  Google Scholar 

  37. N. Dukhan, P. Patel, Exp. Therm. Fluid Sci. 32, 1059–1067 (2008)

    Article  CAS  Google Scholar 

  38. ERG Materials and Aerospace, Oakland, CA. www.ergaerospace.com. Accessed March 2010

  39. R. Figliola, D. Beasly, Theory and Design for Mechanical Measurements (Wiley, New York, 2000)

    Google Scholar 

  40. K. Vafai, C.L. Tien, Int. J. Heat Mass. Trans. 25(8), 1183–1190 (1982)

    Article  Google Scholar 

  41. I.H. Shams, Mechanics of Fluids (Wiley, New York, 1992), p. 674

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, University of Detroit Mercy, Detroit, MI, 48221, USA

    Nihad Dukhan & Krunal Patel

Authors
  1. Nihad Dukhan
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Krunal Patel
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Nihad Dukhan.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Dukhan, N., Patel, K. Effect of sample’s length on flow properties of open-cell metal foam and pressure-drop correlations. J Porous Mater 18, 655–665 (2011). https://doi.org/10.1007/s10934-010-9423-z

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10934-010-9423-z

Keywords

Search

Navigation