Inversion of Elastic Constants of Anisotropic (100) Silicon Based on Surface Wave Velocity by Acoustic Microscopy Using Particle Swarm-Based-Simulated Annealing Optimization

Abstract

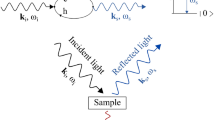
In this paper, a new inversion model of elastic constants of anisotropic (100) silicon is established by inducing the hybrid particle swarm optimization-based-simulated annealing optimization. Theoretical analysis on the surface acoustic wave (SAW) velocities of silicon has been carried out to construct the novel objective error function. The SAW velocities along different azimuthal angles are obtained by the V(f,z) analysis based on a lens-less line-focus acoustic microscopy. And the inversed results agree well with the reported data, which proves that this method shows high accuracy and strong reliability for the inversion of elastic constants of anisotropic materials.

Appendix

The coefficient matrix of the Christoffel equations can be described as the matrix [$K_{ij}$]$_{3\times 3}$ in Eq. (3). So the equations are written as:

$$\begin{aligned} \left[ {{\begin{array}{*{20}c} &{} &{} \\ &{} {K_{ij} } &{} \\ &{} &{} \\ \end{array} }} \right] _{3\times 3} \cdot \left[ {{\begin{array}{*{20}c} {U_1 } \\ {U_2 } \\ {U_3 } \\ \end{array} }} \right] =0 \end{aligned}$$

(13)

where the elements of the matrix [$K_{ij}$]$_{3\times 3}$ are listed as:

$$\begin{aligned} \begin{array}{l} K_{{11}} ={C}'_{11} {+2}{C}'_{{15}} \alpha +{C}'_{{55}} \alpha ^2-\rho c^{2} \\ K_{{12}} =K_{{21}} ={C}'_{{16}} +{C}'_{{14}} \alpha +{C}'_{{56}} \alpha +{C}'_{{54}} \alpha ^2 \\ K_{{13}} =K_{{31}} ={C}'_{{15}} +{C}'_{{13}} \alpha +{C}'_{{55}} \alpha +{C}'_{{53}} \alpha ^2 \\ K_{{22}} ={C}'_{{66}} {+2}{C}'_{{46}} \alpha +{C}'_{{44}} \alpha ^2-\rho c^{2} \\ K_{{23}} =K_{{32}} ={C}'_{{65}} +{C}'_{{63}} \alpha +{C}'_{{45}} \alpha +{C}'_{{43}} \alpha ^2 \\ K_{{33}} ={C}'_{{55}} {+2}{C}'_{{35}} \alpha +{C}'_{{33}} \alpha ^2-\rho c^{2} \\ \end{array} \end{aligned}$$

(14)

where ${C}'_{IJ}$ are the elastic constants in the local coordinate system (${x}'_1$,${x}'_2$,${x}'_3 )$. When the density $\rho$ and phase velocity c are given, three different solutions $\alpha$ $_{q }(q$=1$\sim$3) of the undetermined component $\alpha$ will be obtained. And [$K_{ij}$($\alpha$)]$_{3\times 3}$ will be calculated by substituting $\alpha$ $_{q }$into the matrix [$K_{ij}$]$_{3\times 3}$, the expression of $U_{iq}$ in Eq. (6) are:

$$\begin{aligned} U_{1q}= & {} 1 \nonumber \\ U_{2q}= & {} \frac{K_{\text{11 }} (\alpha _q )K_{\text{23 }} (\alpha _q )-K_{13} (\alpha _q )K_{12} (\alpha _q )}{K_{13} (\alpha _q )K_{22} (\alpha _q )-K_{\text{12 }} (\alpha _q )K_{\text{23 }} (\alpha _q )} \\ U_{3q}= & {} \frac{K_{\text{11 }} (\alpha _q )K_{\text{23 }} (\alpha _q )-K_{13} (\alpha _q )K_{\text{12 }} (\alpha _q )}{K_{12} (\alpha _q )K_{33} (\alpha _q )-K_{23} (\alpha _q )K_{13} (\alpha _q )} \nonumber \end{aligned}$$

(15)

Substituting Eq. (6) into the general Hooke’s law leads to the stress ($\sigma$ $_{33}$, $\sigma$ $_{13}$, $\sigma$ $_{23})$ components, and on the surface of material we have:

$$\begin{aligned} ( {\sigma _{33} ,\sigma _{13} ,\sigma _{23} })= & {} \sum \limits _{q=1}^3 ( {D_{1q} ,D_{2q} ,D_{3q} })A_q \cdot \sqrt{-1} \xi \nonumber \\&\cdot \exp \left[ {\sqrt{-1} \xi ( {x_1 -ct})} \right] \end{aligned}$$

(16)

where the expressions of $D_{iq}$ are:

$$\begin{aligned} D_{1q}= & {} {C}'_{13} {+{C}'}_{36} \cdot U_{2q} +{C}'_{35} \cdot U_{3q} +{C}'_{35} \cdot \alpha _q\nonumber \\&+{C}'_{34} \cdot U_{2q} \cdot \alpha _q +{C}'_{33} \cdot U_{3q} \cdot \alpha _q \nonumber \\ D_{2q}= & {} {C}'_{15} +{C}'_{56} \cdot U_{2q} +{C}'_{55} \cdot U_{3q} +{C}'_{55} \cdot \alpha _q \nonumber \\&+{C}'_{54} \cdot U_{2q} \cdot \alpha _q +{C}'_{53} \cdot U_{3q} \cdot \alpha _q \nonumber \\ D_{3q}= & {} {C}'_{14} +{C}'_{46} \cdot U_{2q} +{C}'_{45} \cdot U_{3q} +{C}'_{45} \cdot \alpha _q \nonumber \\&+{C}'_{44} \cdot U_{2q} \cdot \alpha _q +{C}'_{43} \cdot U_{3q} \cdot \alpha _q \end{aligned}$$

(17)

Finally, the coefficient matrix [M]$_{3\times 3 }$ will be derived:

$$\begin{aligned} \left[ M \right] _{3\times 3} =\left[ {{\begin{array}{*{20}c} {D_{11} } &{} {D_{12} } &{} {D_{13} } \\ {D_{21} } &{} {D_{22} } &{} {D_{23} } \\ {D_{31} } &{} {D_{32} } &{} {D_{33} } \\ \end{array} }} \right] \cdot \sqrt{-1} \xi \cdot \exp \left[ {\sqrt{-1} \xi ( {x_1 -ct})} \right] \end{aligned}$$

(18)