Abstract
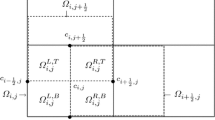
In this paper, the block-centered finite difference method is introduced and analyzed to solve the compressible wormhole propagation. The coupled analysis approach to deal with the fully coupling relation of multivariables is employed. By this, stability analysis and error estimates for the pressure, velocity, porosity, concentration and its flux in different discrete norms are established rigorously and carefully on non-uniform grids. Finally, some numerical experiments are presented to verify the theoretical analysis and effectiveness of the given scheme.









Similar content being viewed by others
References
Kou, J., Sun, S., Wu, Y.: Mixed finite element-based fully conservative methods for simulating wormhole propagation. Comput. Methods Appl. Mech. Eng. 298, 279–302 (2016)
Panga, M.K., Ziauddin, M., Balakotaiah, V.: Two-scale continuum model for simulation of wormholes in carbonate acidization. AIChE J. 51, 3231–3248 (2005)
Wu, Y., Salama, A., Sun, S.: Parallel simulation of wormhole propagation with the Darcy–Brinkman–Forchheimer framework. Comput. Geotech. 69, 564–577 (2015)
Fredd, C.N., Fogler, H.S.: Influence of transport and reaction on wormhole formation in porous media. AIChE J. 44, 1933–1949 (1998)
Golfier, F., Zarcone, C., Bazin, B., Lenormand, R., Lasseux, D., Quintard, M.: On the ability of a Darcy-scale model to capture wormhole formation during the dissolution of a porous medium. J. Fluid Mech. 457, 213–254 (2002)
Liu, M., Zhang, S., Mou, J., Zhou, F.: Wormhole propagation behavior under reservoir condition in carbonate acidizing. Transp. Porous Media 96, 203–220 (2013)
Zhao, C., Hobbs, B., Hornby, P., Ord, A., Peng, S., Liu, L.: Theoretical and numerical analyses of chemical-dissolution front instability in fluid-saturated porous rocks. Int. J. Numer. Anal. Methods Geomech. 32, 1107–1130 (2008)
Li, X., Rui, H.: Characteristic block-centered finite difference method for simulating incompressible wormhole propagation. Comput. Math. Appl. 73, 2171–2190 (2017)
Raviart, P.-A., Thomas, J.-M.: A Mixed Finite Element Method for 2-nd Order Elliptic Problems. Springer, Berlin (1977)
Arbogast, T., Wheeler, M.F., Yotov, I.: Mixed finite elements for elliptic problems with tensor coefficients as cell-centered finite differences. SIAM J. Numer. Anal. 34, 828–852 (1997)
Rui, H., Pan, H.: A Block-centered finite difference method for the Darcy–Forchheimer model. SIAM J. Numer. Anal. 50, 2612–2631 (2012)
Li, X., Rui, H.: Characteristic block-centred finite difference methods for nonlinear convection-dominated diffusion equation. Int. J. Comput. Math. 94, 384–404 (2015)
Li, X., Rui, H.: A two-grid block-centered finite difference method for nonlinear non-Fickian flow model. Appl. Math. Comput. 281, 300–313 (2016)
Rui, H., Pan, H.: Block-centered finite difference methods for parabolic equation with time-dependent coefficient. Japan J. Ind. Appl. Math. 30, 681–699 (2013)
Rui, H., Liu, W.: A two-grid block-centered finite difference method for Darcy–Forchheimer flow in porous media. SIAM J. Numer. Anal. 53, 1941–1962 (2015)
Liu, Z., Li, X.: A parallel CGS block-centered finite difference method for a nonlinear time-fractional parabolic equation. Comput. Methods Appl. Mech. Eng. 308, 330–348 (2016)
Li, X., Rui, H.: A two-grid block-centered finite difference method for the nonlinear time-fractional parabolic equation. J. Sci. Comput. (2017). doi:10.1007/s10915-017-0380-4
Mauran, S., Rigaud, L., Coudevylle, O.: Application of the Carman–Kozeny correlation to a high-porosity and anisotropic consolidated medium: the compressed expanded natural graphite. Transp. Porous Media 43, 355–376 (2001)
Nédélec, J.-C.: Mixed finite elements in \({\mathbb{R}}\) 3. Numer. Math. 35, 315–341 (1980)
Weiser, A., Wheeler, M.F.: On convergence of block-centered finite differences for elliptic problems. SIAM J. Numer. Anal. 25, 351–375 (1988)
Dawson, C.N., Wheeler, M.F., Woodward, C.S.: A two-grid finite difference scheme for nonlinear parabolic equations. SIAM J. Numer. Anal. 35, 435–452 (1998)
Durán, R.: Superconvergence for rectangular mixed finite elements. Numer. Math. 58, 287–298 (1990)
Douglas, J., Roberts, J.E.: Numerical methods for a model for compressible miscible displacement in porous media. Math. Comput. 41, 441–459 (1983)
Guo, Q., Zhang, J.Wang: Error analysis of the semi-discrete local discontinuous Galerkin method for compressible miscible displacement problem in porous media. Appl. Math. Comput. 259, 88–105 (2015)
Acknowledgements
The authors would like to thank the editor and referees for their valuable comments and suggestions which helped us to improve the results of this paper. This work is supported by the National Natural Science Foundation of China Grant No. 11671233, the Science Challenge Project No. JCKY2016212A502.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Li, X., Rui, H. Block-Centered Finite Difference Method for Simulating Compressible Wormhole Propagation. J Sci Comput 74, 1115–1145 (2018). https://doi.org/10.1007/s10915-017-0484-x
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10915-017-0484-x
Keywords
- Block-centered finite difference
- Compressible wormhole propagation
- Non-uniform grids
- Error estimates
- Numerical experiments