Uniform Convergence of V-cycle Multigrid Algorithms for Two-Dimensional Fractional Feynman–Kac Equation

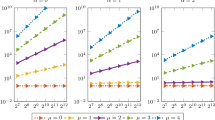
Abstract

When solving large linear systems stemming from the approximation of elliptic partial differential equations (PDEs), it is known that the V-cycle multigrid method (MGM) can significantly lower the computational cost. Many convergence estimates already exist for the V-cycle MGM: for example, using the regularity or approximation assumptions of the elliptic PDEs, the results are obtained in Bank and Douglas (SIAM J Numer Anal 22:617–633, 1985), Bramble and Pasciak (Math Comp 49:311–329, 1987); in the case of multilevel matrix algebras (like circulant, tau, Hartely) (Aricò et al. in SIAM J Matrix Anal Appl 26:186–214, 2004; Aricò and Donatelli in Numer Math 105:511–547, 2007), special prolongation operators are provided and the related convergence results are rigorously developed, using a functional approach. In this paper we derive new uniform convergence estimates for the V-cycle MGM applied to symmetric positive definite Toeplitz block tridiagonal matrices, by also discussing few connections with previous results. More concretely, the contributions of this paper are as follows: (1) It tackles the Toeplitz systems directly for the elliptic PDEs. (2) Simple (traditional) restriction operator and prolongation operator are employed in order to handle general Toeplitz systems at each level of the recursion. Such a technique is then applied to systems of algebraic equations generated by the difference scheme of the two-dimensional fractional Feynman–Kac equation, which describes the joint probability density function of non-Brownian motion. In particular, we consider the two coarsening strategies, i.e., doubling the mesh size (geometric MGM) and Galerkin approach (algebraic MGM), which lead to the distinct coarsening stiffness matrices in the general case: however, several numerical experiments show that the two algorithms produce almost the same error behaviour.

Appendix

Proof of Lemma 2.3

Since $A^{(k)}$ is the symmetric matrix, we denote $A^{(k)}=\{a_{i,j}^{(k)}\}_{i,j=1}^{\infty }$ with $a_{i,j}^{(k)}=a_{|i-j|}^{(k)}~~~~\forall k \ge 1.$ Using the relation $A^{(k)}=L_h^{H}A^{(k-1)}L_{H}^{h}$, there exists

$$\begin{aligned} \{b_{j,l}^{(k)}\}_{j,l=1}^{\infty }=A^{(k-1)}L_{H}^{h}~~~~\mathrm{and}~~~~\{a_{i,l}^{(k)}\}_{i,l=1}^{\infty }=L_h^{H}A^{(k-1)}L_{H}^{h} \end{aligned}$$

with $b_{j,l}^{(k)}=a_{2l-j-1}^{(k-1)}+2a_{2l-j}^{(k-1)}+a_{2l-j+1}^{(k-1)}$ and $a_{i,l}^{(k)}=b_{2i-1,l}^{(k)}+2b_{2i,l}^{(k)}+b_{2i+1,l}^{(k)}$. Then for the Toeplitz matrix $A^{(k)}$, it holds

$$\begin{aligned} a_0^{(k)}&=6a_0^{(k-1)}+8a_1^{(k-1)}+2a_2^{(k-1)} \quad \forall k \ge 2; \nonumber \\ a_j^{(k)}&=a_{2j-2}^{(k-1)} +4a_{2j-1}^{(k-1)}+6a_{2j}^{(k-1)}+4a_{2j+1}^{(k-1)}+a_{2j+2}^{(k-1)} \quad \forall j \ge 1. \end{aligned}$$

(A.1)

We prove (2.13) by mathematical induction. For $k=2$, Eq. (2.13) holds obviously. Suppose (2.13) holds for $k=2,3,\ldots s$. In particular, for $k=s$, we have

$$\begin{aligned} a_0^{(s)} =&(4C_s+2^{s-1})a_0^{(1)}+\sum _{m=1}^{2\cdot 2^{s-1}-1}{_0}C_m^sa_m^{(1)};\nonumber \\ a_1^{(s)} =&C_sa_0^{(1)}+\sum _{m=1}^{3\cdot 2^{s-1}-1}{_1}C_m^s a_m^{(1)};\nonumber \\ a_j^{(s)} =&\sum _{m=(j-2)2^{s-1}}^{(j+2)2^{s-1}-1} {_j}C_m^s a_m^{(1)} \quad \forall j\ge 2. \end{aligned}$$

(A.2)

Next we need to prove that (2.13) holds for $k=s+1$.

According to (A.1), (A.2) and the coefficients ${_j}C_m^s$, $j\ge 0$ in (2.13), we can check that

$$\begin{aligned} a_0^{(s+1)}&=6a_0^{(s)}+8a_1^{(s)}+2a_2^{(s)}\\&=\left( 32c_s+6\cdot 2^{s-1}\right) a_0^{(1)}+\sum _{m=1}^{2^{s-1}-1}\left( 6\cdot {_0}C_m^{s} +8\cdot {_1}C_m^{s}+2\cdot {_2}C_m^{s} \right) a_m^{(1)}\\&\quad +\sum _{m=2^{s-1}}^{2\cdot 2^{s-1}-1}\left( 6\cdot {_0}C_m^{s} +8\cdot {_1}C_m^{s}+2\cdot {_2}C_m^{s} \right) a_m^{(1)}\\&\quad +\sum _{m=2\cdot 2^{s-1}}^{3\cdot 2^{s-1}-1}\left( 8\cdot {_1}C_m^{s}+2\cdot {_2}C_m^{s} \right) a_m^{(1)} +\sum _{m=3\cdot 2^{s-1}}^{4\cdot 2^{s-1}-1}2\cdot {_2}C_m^{s} a_m^{(1)}\\&=(4C_{s+1}+2^{s})a_0^{(1)}+\sum _{m=1}^{4\cdot 2^{s-1}-1}{_0}C_m^{s+1}a_m^{(1)};\\ a_1^{(s+1)}&=a_0^{(s)}+4a_1^{(s)}+6a_2^{(s)}+4a_3^{(s)}+a_4^{(s)}\\&=\left( 8c_s+ 2^{s-1}\right) a_0^{(1)}+\sum _{m=1}^{2^{s-1}-1}\left( {_0}C_m^{s} +4\cdot {_1}C_m^{s}+6\cdot {_2}C_m^{s} \right) a_m^{(1)}\\&\quad +\sum _{m=2^{s-1}}^{2\cdot 2^{s-1}-1}\left( {_0}C_m^{s} +4\cdot {_1}C_m^{s}+6\cdot {_2}C_m^{s} +4\cdot {_3}C_m^{s} \right) a_m^{(1)}\\&\quad +\sum _{m=2\cdot 2^{s-1}}^{3\cdot 2^{s-1}-1}\left( 4\cdot {_1}C_m^{s}+6\cdot {_2}C_m^{s} +4\cdot {_3}C_m^{s}+{_4}C_m^{s} \right) a_m^{(1)}\\&\quad +\sum _{m=3\cdot 2^{s-1}}^{4\cdot 2^{s-1}-1}\left( 6\cdot {_2}C_m^{s} +4\cdot {_3}C_m^{s}+{_4}C_m^{s} \right) a_m^{(1)}\\&\quad +\sum _{m=4\cdot 2^{s-1}}^{5\cdot 2^{s-1}-1}\left( 4\cdot {_3}C_m^{s}+{_4}C_m^{s} \right) a_m^{(1)} +\sum _{m=5\cdot 2^{s-1}}^{6\cdot 2^{s-1}-1}{_4}C_m^{s} a_m^{(1)}\\&=C_{s+1}a_0^{(1)}+\sum _{m=1}^{6\cdot 2^{s-1}-1}{_1}C_m^{s+1} a_m^{(1)};\\ \end{aligned}$$

and

$$\begin{aligned} a_j^{(s+1)}&=a_{2j-2}^{(s)} +4a_{2j-1}^{(s)}+6a_{2j}^{(s)}+4a_{2j+1}^{(s)}+a_{2j+2}^{(s)}\\&=\sum _{m=(2j-4)2^{s-1}}^{(2j-3)2^{s-1}-1} {_{2j-2}}C_m^{s} a_m^{(1)} +\sum _{m=(2j-3)2^{s-1}}^{(2j-2)2^{s-1}-1} \left( {_{2j-2}}C_m^{s} +4\cdot {_{2j-1}}C_m^{s} \right) a_m^{(1)}\\&\quad +\sum _{m=(2j-2)2^{s-1}}^{(2j-1)2^{s-1}-1} \left( {_{2j-2}}C_m^{s} +4\cdot {_{2j-1}}C_m^{s}+6\cdot {_{2j}}C_m^{s} \right) a_m^{(1)}\\&\quad +\sum _{m=(2j-1)2^{s-1}}^{2j\cdot 2^{s-1}-1} \left( {_{2j-2}}C_m^{s} +4\cdot {_{2j-1}}C_m^{s}+6\cdot {_{2j}}C_m^{s}+4\cdot {_{2j+1}}C_m^{s} \right) a_m^{(1)}\\&\quad +\sum _{m=2j\cdot 2^{s-1}}^{(2j+1)\cdot 2^{s-1}-1} \left( 4\cdot {_{2j-1}}C_m^{s}+6\cdot {_{2j}}C_m^{s}+4\cdot {_{2j+1}}C_m^{s}+{_{2j+2}}C_m^{s} \right) a_m^{(1)}\\&\quad +\sum _{m=(2j+1)\cdot 2^{s-1}}^{(2j+2)\cdot 2^{s-1}-1} \left( 6\cdot {_{2j}}C_m^{s}+4\cdot {_{2j+1}}C_m^{s}+{_{2j+2}}C_m^{s} \right) a_m^{(1)}\\&\quad +\sum _{m=(2j+2)\cdot 2^{s-1}}^{(2j+3)\cdot 2^{s-1}-1} \left( 4\cdot {_{2j+1}}C_m^{s}+{_{2j+2}}C_m^{s} \right) a_m^{(1)} +\sum _{m=(2j+3)\cdot 2^{s-1}}^{(2j+4)\cdot 2^{s-1}-1} {_{2j+2}}C_m^{s} a_m^{(1)}\\&=\sum _{m=(2j-4)2^{s-1}}^{(2j+4)2^{s-1}-1} {_j}C_m^{s+1} a_m^{(1)}. \end{aligned}$$

The proof is completed. $\square$